1. Introduction

2. Oscillatory Properties of Noncanonical Neutral DDEs of Second-Order

We begin with the following notations: $U^{+}$ is the set of all eventually positive solutions of (1), $V\left(t\right):=a_0^{1/\beta }\left(t\right)v^{\prime }\left(t\right)$ ,

Lemma 1. Assume that $v\in U^{+}$ and there exists a  ${\delta }_0\in \left(0,1\right)$ such that:

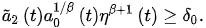
(3)

Then, v eventually satisfies:

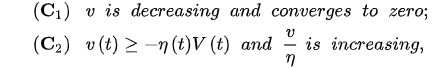
and:

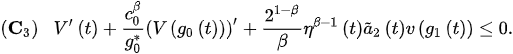
Proof.  Let $u\in U^{+}$ . Then, we have that $u\left(g_0\left(t\right)\right)$ , and $u\left(g_1\left(t\right)\right)$ are positive for $t\ge t_1$ , for some $t_1\ge t_0$ . Therefore, it follows from (1) that:

Using (1) and Lemma 1 in ^[1], we see that:

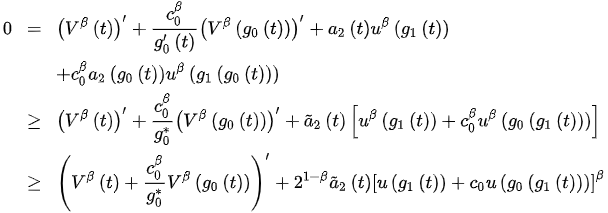
and so:

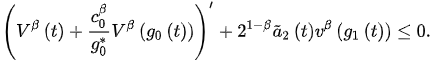
(4)

Integrating this inequality from $t_1$ to t and using the fact  ${\left(V^{\beta }\left(t\right)\right)}^{\prime }\le 0$ , we find:

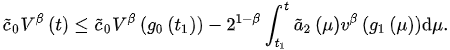
(5)

$\left({\mathbf{C}}_1\right)$ Assume the contrary, that $v^{\prime }\left(t\right)>0$ for $t\ge t_1$ . Thus, from (5), we have:

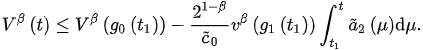
This, from (3), implies:

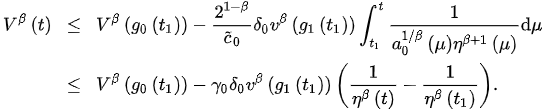
Letting $t\to \infty$ and taking the fact that $\eta \left(t\right)\to 0$ as $t\to \infty$ ,  we obtain $V^{\beta }\left(t\right)\to -\infty$ , which contradicts the positivity of  $V\left(t\right)$ .

Next, since v is positive decreasing, we have that ${lim}_{t\to \infty }v\left(t\right)=v_0\ge 0$ . Assume the contrary, that $v_0>0$ . Then, $v\left(t\right)\ge v_0$ for all $t\ge t_2$ , for some $t_2\ge t_1$ . Thus, from (3) and (5), we have:

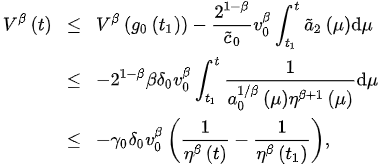
or

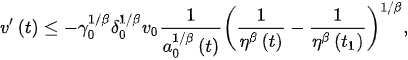
and so,

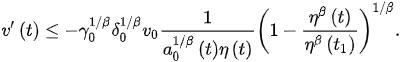
(6)

Using the fact that ${\eta }^{\prime }\left(t\right)<0$ , we obtain that $\eta \left(t\right)<{\eta }^{\prime }\left(t_2\right)<{\eta }^{\prime }\left(t_1\right)$ for all $t\ge t_2\ge t_1$ . Hence, by integrating (6) from $t_1$ to t, we obtain:

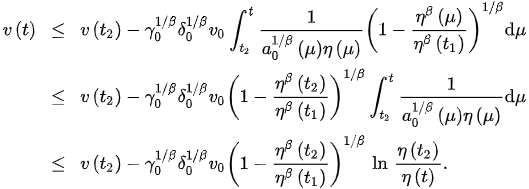
Letting $t\to \infty$ and taking the fact that $\eta \left(t\right)\to 0$ as $t\to \infty$ , we obtain $v\left(t\right)\to -\infty$ , which contradicts the positivity of $v\left(t\right)$ . Therefore, $v_0=0$ .

$\left({\mathbf{C}}_2\right)$ Since $V\left(t\right)$ is decreasing, we obtain:

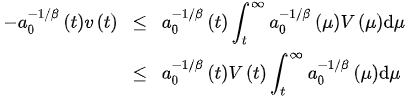
and:

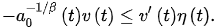
(7)

Then, ${\left(v/\eta \right)}^{\prime }\ge 0$ .

$\left({\mathbf{C}}_3\right)$ From (7), we obtain:

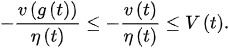
Thus, from (4) and the fact  $V^{\prime }\left(t\right)\le 0$ , we obtain:

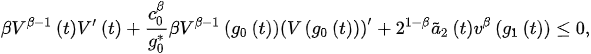
and then:

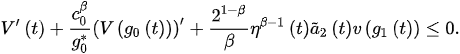
The proof is complete.

Lemma 2. Assume that $u\in U^{+}$ and there exists a ${\delta }_0\in \left(0,1\right)$ such that (3) holds. Then:

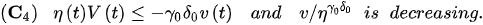
Proof.  Let $u\in U^{+}$ . From Lemma 1, we have that $\left({\mathbf{C}}_1\right)$ – $\left({\mathbf{C}}_3\right)$ hold for $t\ge t_1$ .

Integrating $\left({\mathbf{C}}_3\right)$ from $t_1$ to t, we arrive at:

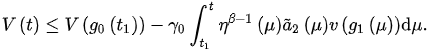
From (3), we obtain:

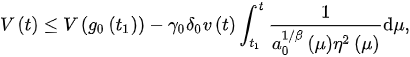
and:

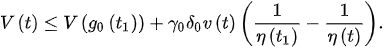
(8)

Using $\left({\mathbf{C}}_1\right)$ , we eventually have:

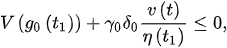
Hence, (8) becomes:

This implies that $v/{\eta }^{{\gamma }_0{\delta }_0}$ is a decreasing function.
The proof is complete.

2.2. Oscillation Theorems

is oscillatory, then every solution of (1) is oscillatory.

Proof.  Assume the contrary, that (1) has a solution $u\in U^{+}$ . Then, we have that $u\left(t\right),$ and $u\left(g_1\left(t\right)\right)$ are positive for $t\ge t_1$ , for some $t_1\ge t_0$ . From Lemmas 1 and 2, we have that $\left({\mathbf{C}}_1\right)$ – $\left({\mathbf{C}}_4\right)$ hold for $t\ge t_1$ .

Next, we define:

From $\left({\mathbf{C}}_1\right)$ , $w\left(t\right)>0$ for $t\ge t_1$ . Thus,

Thus, it follows from $\left({\mathbf{C}}_3\right)$ that:

(10)

Using $\left({\mathbf{C}}_4\right)$ , we obtain that:

which with (10) gives:

(11)

Now, we set:

Then, $W\left(t\right)\le {\tilde{c}}_{0}w\left(g_0\left(t\right)\right)$ , and so,  (11) becomes:

which has a positive solution. In view of ^[2] (Theorem 1), (9) also has a positive solution, which is a contradiction.

The proof is complete.

Corollary 1. Assume that $g_1\left(t\right)\le g_0\left(t\right)$ and there exists a ${\delta }_0\in \left(0,1\right)$ such that (3) holds. If:

(12)

then every solution of (1) is oscillatory.

Proof.  It follows from Theorem 2 in ^[3] that the condition (12) implies the oscillation of (9).

Next, by introducing two Riccati substitution, we obtain a new oscillation criterion for (1).

Theorem 7. Assume that $g_1\left(t\right)\le g_0\left(t\right)$ and there exists a ${\delta }_0\in \left(0,1\right)$ such that (3) holds. If:

(13)

then every solution of (1) is oscillatory.

Proof.  Assume the contrary, that (1) has a solution $u\in U^{+}$ . Then, we have that $u\left(t\right),$ and $u\left(g_1\left(t\right)\right)$ are positive for $t\ge t_1$ , for some $t_1\ge t_0$ . From Lemmas 1 and 2, we have that $\left({\mathbf{C}}_1\right)$ – $\left({\mathbf{C}}_4\right)$ hold for $t\ge t_1$ .

Now, we define the functions:

$${\Theta }_1:=\frac{V}{v},$$

and:

$${\Theta }_2:=\frac{V\circ g_0}{v\circ g_0}.$$

Then, ${\Theta }_1$ and ${\Theta }_2$ are negative for $t\ge t_1$ . From $\left({\mathbf{C}}_4\right)$ , we obtain:

Hence,

and:

Then:

(14)

and:

(15)

Combining (14) and (15), we obtain:

Integrating this inequality from $t_1$  to t, we have:

From $\left({\mathbf{C}}_2\right),$ we obtain $\eta \left(t\right){\Theta }_1\left(t\right)\ge -1$ . Therefore,

where:

Since ${\eta }^{\prime }\left(t\right)<0$ and $a^{\prime }\left(t\right)\ge 0$ , we find:

Taking $lim{sup}_{t\to \infty }$ and using (13), we arrive at a contradiction.

The proof is complete.

2.3. Applications and Discussion

Remark 1. It is easy to see that the previous works that dealt with the noncanonical case required either $a_1\left(t\right)<1$ or $a_1\left(t\right)<\eta \left(t\right)/\eta \left(g_0\left(t\right)\right)$ . Since η is decreasing and $g_0\left(t\right)\le t$ , we have that $\eta \left(g_0\left(t\right)\right)\ge \eta \left(t\right)$ . Then, the results of these works only apply when $a_1\left(t\right)\in \left(0,1\right)$ .

Example 1. Consider the DDE:

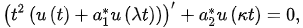
(16)

where $t\ge 1,$ $a_1^{*}>0,$ and $\kappa <\lambda \in \left(0,1\right)$ . By choosing ${\delta }_0=a_2^{*},$ the condition (12) becomes:

(17)

Using Corollary 1, Equation (16) is oscillatory if (17) holds.

Remark 2. To apply Theorems 3 and 4 on (16), we must stipulate that $a_1^{*}<1$ . Let a special case of (16), namely,

A simple computation shows that (16) is oscillatory if:

(18)

or:

(19)

or:

(20)

Consider the following most specific special case:

(21)

Note that (18)–(20) fail to apply. However, (17) reduces to:

which ensures the oscillation of (21).