Topic review

Calculation of the transition temperature of superconducting elements

Subjects: Solid state physics, condensed matter physics, superconductivity 

Submitted by:

Michael Koblischka and Anjela Koblischka-Veneva

 Definition

Using the Roeser-Huber formalism, we establish a non-trivial relation between the crystal structure and the transition temperature, T_c, to the superconducting state. By means of this relation, we can calculate T_c for practically all superconducting elements quite accurately within a small error margin. It is shown that this works well also for polymorphic elements and elements under pressure. Furthermore, the Roeser-Huber formalism implies that all calculated data fall on a common line with the slope m₁ = h²/(2πk_B) = 5.061 × 10⁻⁴⁵ m² kg K, when plotting log(Σ((2x)^-2n₁^-1M_L^-1))^-1 versus 1/T_c, which can be employed as a test when predicting T_c of unknown superconductors.

1. Introduction

Up to now, there are 53 superconducting elements known (in ambient conditions and under pressure, [1,2], and three more are superconductors under special conditions (i.e., in thin film form (Cr), after irradiation (Pd), or C in several modifications [3], e.g., diamond films, alkali-doped fullerene, and carbon nanotubes). A periodic table of the elements with the transition temperature (T_c) data from the literature is presented in Figure 1. From all reviews and teaching books covering this field [2—9], it is clear that there is no simple relation between T_c and the respective crystal structure. Moreover, some elements are polymorphic superconductors with different crystal structures, e.g., La, Hg and Ga, and some elements show changes of the crystal structure under pressure (e.g., Fe becomes superconducting under pressure in the non-magnetic phase (hexgonally close-packed (hcp) ε-Fe phase) [10—12]. Commonly, bandstructure calculations are performed to obtain T_c requiring many parameters (see, e.g., Refs. [13,14] and using given crystal structures as a base, which is, however, not straightforward to enable comparison of a large variety of elements and different crystal structures.

All this demonstrates clearly that the link between the crystal structure and T_c, if existing, must be a much more subtle and non-trivial~one. Exactly this task is fulfilled by the Roeser--Huber (from now on, we use the abbreviation RH) formalism, establishing a truely non-trivial relation between T_c and the given crystal structures.

For a successful application of the RH formalism, one needs the crystallographic information of the possible crystal structures, and about the electronic configuration to count the number N_L of electrons involved in superconductivity (the rules for this are similar to the valence electron count used by Matthias [15] and the number of passed (or near) atoms in the crystal unit cell, N_atoms as determined from the given crystal structure using the relation l/x < 0.5, which is described in Sec. 2 below.

Thus, a relatively simple calculation procedure like the RH formalism, which requires only knowledge of the crystal structure and the basic electronic configuration with no free parameters, has clear advantages when being incorporated as a test in machine learning approaches to find new superconducting materials [16—18].

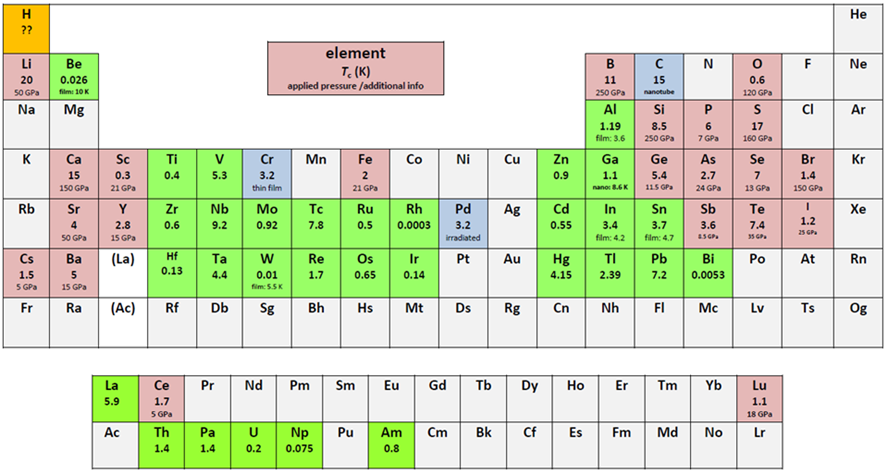
Figure 1. Superconducting elements in the periodic table (light green = superconductor in ambient conditions, light brown = superconductor under applied pressure, and light blue = superconductor with special conditions). Given are the abbreviations of the names, the transition temperatures, T_c, and the applied pressure or some extra info for each element. Hydrogen H is marked in orange concerning the prediction to be a room-temperature superconductor under high pressure. All data given are taken from [1—9]

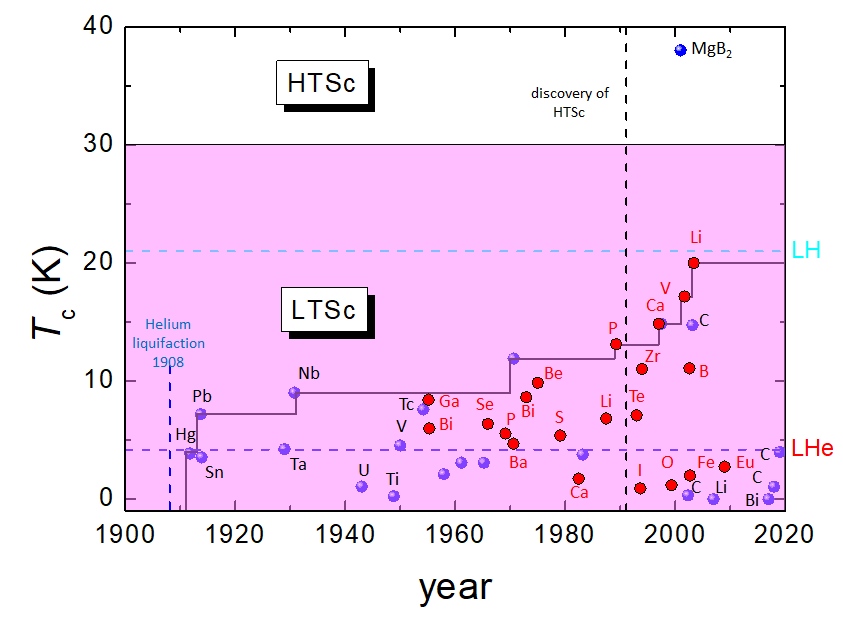
Figure 2. (a) T_c as a function of the year of discovery of the superconducting elements (● – ambient conditions, ● – under pressure). Note the highest T_c of all elements at 20 K for Li under pressure. The borderlines for liquid He (LHe) and liquid H (LH) are also presented. The 30 K-line marks the border between LTSc and HTSc materials, crossed by the alloy superconductor MgB₂, which is given for comparison. (b) Logarithmic plot of T_c of various elements in ambient conditions with respect to their crystal structure (body-centered cubic (bcc), face-centered cubic (fcc), hexagonally close-packed (hcp), tetragonal (tetra), monoclinic (mono), orthorhombic (ortho), fcc* and double hexagonally close-packed (dhcp)). The highest T_c in ambient conditions is obtained for Nb with bcc structure, the lowest T_c to date has Bi with a monoclinic structure.

2. Details of the RH formalism

The starting point is the view of a superconducting transition as an integrated resonance curve between the charge carrier wave (Cooper pairs with the deBroglie wavelength λ_cc) and a characteristic length within the crystal unit cell.

As described already in Refs. [19,20], the Roeser--Huber formula is given as

\begin{equation} \Delta_{(0){\rm ges}}= \frac{h^2}{2 M_{\rm L}} \cdot \left(\sum_{R_1}^{R_n} \frac{1}{(2 x_{R_i})^2} \cdot n_0^{2/3} \cdot \frac{n_{2R_i}}{n_{1R_i}}\right) = \pi k_B T_{c(0)} ~~~, \label{eq:1} \end{equation} with Δ₍₀₎ describing the lowest level energy of the PiB. h denotes the Planck constant, k_B the Boltzmann constant, M_L is a parameter with the unit of a mass, and the sum is taken for all possible directions, R_i, as explained below. For all unit cells of metallic elemental superconductors, there are no 2D-like superconducting planes, thus n₀ (describing the number of superconducting planes of 2D superconductors) is set to n₀ = 1, and the parameter n₂ is also set to 1 as the simple crystal structures of the elements do not have multiple superconducting paths as in the case of alloys [20,21].

The characteristic interatomic distance x now depends on each crystallographic direction (which will be called superconducting path hereafter), R_i, and the correction factor, n₁, the determination of which is discussed below.

It is to the credit of the late Prof. Roeser and his students to have established a set of rules which provide the essential input for the calculations.

Following the works of Moritz [19] and [21] Stepper, there are four important points to be considered when calculating T_c for metallic elements:

# The distance x corresponds to an interatomic distance similar to the particle-in-box (PiB) approach applied in [22,23]. The distance x is obtained from possible symmetric paths (also called superconducting directions in the following) for the movement of the charge carrier wave within the crystal structure, as discussed below. The crystallographic data come from respective databases [24,25], which is an important issue for application of the RH formalism in machine-learning calculation approaches.

# The parameter M_L for high-T_c superconductor (HTSc) compounds was taken as 2 m_e (= electron mass, 2 for a Cooper pair). For element superconductors, M_L= η m_e is much higher as all the phononic interactions (Fermi temperature, Debye temperature, effective mass, charge carrier density) are incorporated in the parameter η. In a first approximation, η m_e ~1900, which corresponds closely to the mass of a proton (m_p/m_e = 1836.15). Regarding the location of metallic superconductors in a Uemura plot (T_c as function of the Fermi temperature, T_F = (m* v_F²)/(2 k_B) [26,27] and v_F denoting the Fermi velocity, m* is the effective mass) in the lower right corner with TF ~10⁴.10⁵ K, the high value for η is reasonable. This significant difference between elemental metallic superconductors and the HTSc materials was also pointed out by Emery and Kievelson [28] mentioning the substantial phase rigidity of the superconducting state in, e.g., lead at all temperatures below T_c.

# A first correction factor is required to account for more complex crystal structures. Atoms being close to a superconducting direction may have an influence on the moving charge carriers via the phonon interaction. Therefore, the number of atoms passed within a unit cell is counted. This correction was originally added to the parameter M_L via:

\begin{equation} M_L = \frac{N_L}{N_{\rm atoms}} \cdot m_p ~~~, \label{eq:2} \end{equation}

which we keep here for consistency. Regarding the definition of η given above, the relation N_L/N_atoms is thus incorporated in η.

Here, N_L represents the number of the charge carriers and Natoms denotes the number of the near, passed atoms along each superconducting path. A correction factor n₁ can be then defined as n₁ = N_L/N_atoms. In case there are no (near) passed atoms, then n₁ = 1.

As the symmetry of the superconducting path plays an important role for our considerations, the passed atoms must be symmetrically arranged along the superconducting path, as otherwise the charge carriers would be not in phase due to the unsymmetric forces. This implies that superconductivity cannot exist in directions with unsymmetrically arranged passed atoms. As test for the influence of the passed atoms, we define a relation l/x, with l being a distance perpendicular to the direction of the moving charge carriers. If l/x ≤ 0.5, the passed atoms show an influence on the superconductivity and must be counted in N_atoms.

Here, it is important to point out that N_L and N_atoms are not free parameters, but are given from the respective crystal structure being investigated. A special case for determining N_L will be encountered for hcp Fe under pressure as discussed below.

# A second correction factor is necessary to account for anisotropic superconductivity, which can even lead to so-called multimode superconductivity. The factor n₂ gives a relation between the specific directions for the charge carrier wave, R_i, in the given crystal structure.

The energy Δ₍₀₎ and the transition temperature T_c(0) are then calculated for each existing superconducting path R_i, and the results must be summed up according to Equation (1). If one of the directions R_i gives a reasonable value for T_c(R_i) to compare with the experimentally determined T_c, this direction is taken as the superconducting path. However, the T_c(R_i)-values of the other directions and the complete sum of all T_c(R_i) may also have important implications as, e.g., in the case of Al, which was discussed in Ref. [20], the experimental value of T_c is reached with 2 of the possible 4 directions in the fcc structure, but the total sum of all 4 directions is strikingly close to the increased T_c, when measuring thin films. A similar situation is given for the energies, Δ₍₀₎(R_i). Δ₍₀₎(R_i) may be compared to the pairing energy or energy gap as determined experimentally.

Thus, all Δ₍₀₎(R_i)- and T_c(R_i)-values must be calculated for a given material.

For most elemental superconductors, n₂ = 1, in contrast to the metallic alloys Nb₃Sn or MgB₂, where superconducting directions can exist several times within one unit cell, as already discussed in Ref. [20].

The Roeser--Huber formalism (1) does not contain any free parameters, as all the required inputs are given via the crystal unit cell (i.e., x and N_atoms) and N_L from the basic electronic configuration of the material. It must be noted here that the RH formalism does not describe how the Cooper pairs are formed, and so it is not possible to determine if a given material is a superconductor. However, regarding the limits of the parameter η (η_min = 2, and η_max is set by the demand of the BCS-theory that the effective charge carrier mass, m* cannot be smaller than m*< 0.1 m_e for isotropic, spherical Fermi surfaces [29], one may deduce a possibility to judge if superconductivity for a given material and crystal structure exists. This will be elaborated in future works.

Figure 3 presents the Roeser--Huber plot with many calculated superconducting materials, high-T_c superconductors (HTSc, marked with a red circle), metallic alloys (blue circle) and elements (green circle). Practically all superconducting elements can be calculated using the RH formalism with only small error margins. The only exceptions are the very low-T_c materials Li, Be and Bi, where the low values of T_c can only be reproduced with an adaptation of η, which is due to either an extremely small charge carrier mass (Li, Be) or a large electron mean free path (Bi). In contrast, the RH formalism works well to calculate the respective T_c-values of the same materials under applied pressure.

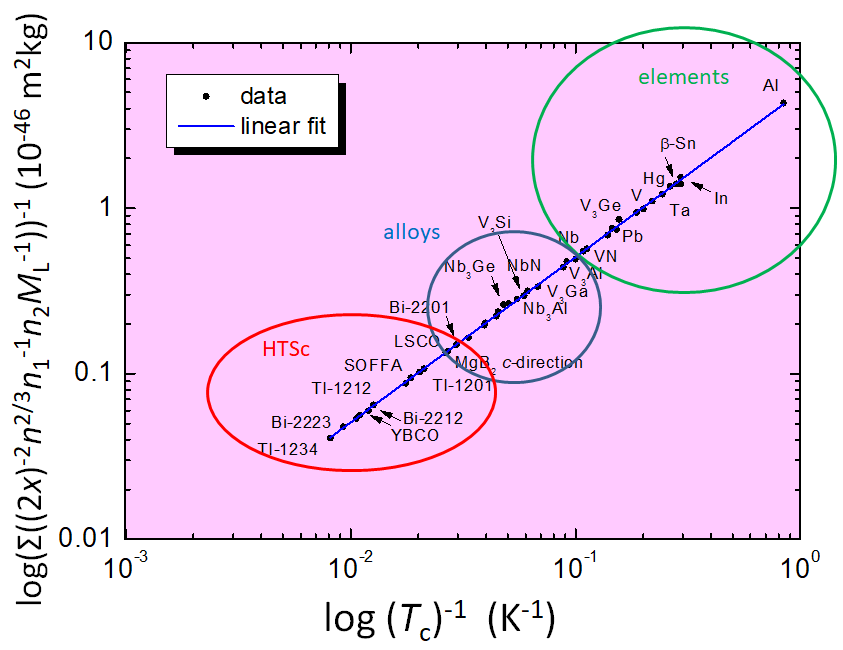
Figure 3. The Roeser--Huber plot for a large number of superconducting materials. Red circle: HTSc, blue circle: alloys, and green circle: elemental superconductors. Some overlaps between these regions do exist. For clarity, only some of our collected data are included.

A very important point is here that all the data of T_c obtained fall on a common, straight line (blue), which follows the equation for a particle in a box [23] with the slope h²/(2π k_B) = 5.061 × 10^-45 m² kg K. This result enables the RH formalism to act as a test for given predictions of T_c, e.g., for the case of metallic hydrogen as was done recently in Ref. [30].

3. Conclusions

To conclude, in our article [31] and before in Ref. [20], we have presented calculations of the superconducting transition temperature, T_c, for two polymorphic superconducting elements (Hg, La) using the RH formalism and for ε-Fe (hcp) under pressure. The principles of this formalism enable to reproduce the different T_c-values of various crystal structures of the same elements, which directly proofs the validity of the concept. This will enable us to apply this formalism for the prediction of T_c of still unknown superconducting materials, e.g., in machine learning approaches.

This entry is adapted from 10.3390/met12020337 

References

1. Flores-Livas, J.A.; Boeri, L.; Sanna, A.; Profeta, G.; Arita, R.; Eremets, M. A perspective on conventional high-temperature superconductors at high pressure: Methods and materials. Phys. Rep. 2020, 856, 1—78.
2. Buzea, C.; Robbie K. Assembling the puzzle of superconducting elements: A review. Supercond. Sci. Technol. 2005, 18, R1—R8.
3. Buckel, W., Kleiner, R. Supraleitung. Grundlagen und Anwendungen, 7^th edition, Wiley-VCH, Weinheim, 2013.
4. Eisenstein, J. Superconducting elements. Rev. Mod. Phys. 1954, 26, 277—291.
5. Matthias, B. T. Chapter V: Superconductivity in the Periodic System. Prog. Low Temp. Phys. 1957, 2, 138—150.
6. Roberts, B. W. Survey of superconductive materials and critical evaluation of selected properties. J. Phys. Chem. Ref. Data 1976, 5, 581—821.
7. Poole, C.P. (Ed.) Handbook of Superconductivity, 1^st ed.; Elsever: Amsterdam, The Netherlands, 1995.
8. Narlikar, A. V. Superconductors. Oxford University Press, Oxford, U.K., 2014.
9. Savitskii, E.M.; Baron, V.V.; Efimov, Y.V.; Bychkova, M.I.; Myzenkova, L.F. Superconducting Materials, 1st ed.; Plenum Press: New York, NY, USA; London, UK, 1973.
10. Shimizu, K.; Kimura, T.; Furomoto, S.; Takeda, K.; Kontani, K.; Onuki, Y.; Amaya, K. Superconductivity in the non-magnetic state of iron under pressure. Nature 2001, 427, 316—318.
11. Steinle-Neumann, G.; Stixrude, L.; Cohen, R. E. Magnetism in dense hexagonal iron. Proc. Natl. Acad. Sci. 2004, 101, 33—36.
12. Roth, S. Theoretical Investigation of One-Component-Superconductors and their Relationship between Transition Temperature and Ionization Energy, Crystal Parameters and Further Parameters. Ph.D. Thesis, Institute of Space Systems, University of Stuttgart, 2018. (in German)
13. Nowotny, H.; Hittmair, O. Calculation of the transition temperature T_c of superconductors. Phys. Stat. Solidi (B) 1979, 91, 647—656.
14. Arita, R.; Koretsune, T.; Sakai, S.; Akashi, R.; Nomura, Y.; Sano, W. Nonempirical Calculation of Superconducting Transition Temperatures in Light-Element Superconductors. Adv. Mater. 2017, 29, 1602421.
15. Matthias, B. T., Geballe, T. H., Compton, V. B. Superconductivity. Rev. Mod. Phys. 1963, 35, 1—22.
16. Stanev, V., Oses, C., Kusne, A. G., Rodriguez, E., Paglione, J., Curtarolo, S., Takeuchi, I. Machine learning modeling of superconducting critical temperature. npj Computational Materials 2018, 4, 29.
17. Lee, D.; You, D.; Lee, D.; Li, X.; Kim, S. Machine-Learning-Guided Prediction Models of Critical Temperature of Cuprates. J. Phys. Chem. Lett. 2021, 12, 6211—6217.
18. Konno, T.; Kurokawa, H.; Nabeshima, F.; Sakishita, Y.; Ogawa, R.; Hosako, I.; Maeda, A. Deep learning model for finding new superconductors. Phys. Rev. B 2021, 103, 014509.
19. Moritz, A. Calculation of the transition temperature of one-component-superconductors. Master thesis IRS 09-S33, Institute of Space Systems, University of Stuttgart, Stuttgart, Germany, 2009. (in German)
20. Koblischka, M. R., Roth, S., Koblischka-Veneva, A., Karwoth, T., Wiederhold, A., Zeng, X. L., Fasoulas, S., and Murakami, M. Relation between Crystal Structure and Transition Temperature of Superconducting Metals and Alloys. Metals 2020, 10, 158.
21. Stepper, M. Master thesis IRS 08-S23, Institute of Space Systems, University of Stuttgart, Germany, 2008. (in German)
22. Roeser, H. P., Hetfleisch, F.; Huber, F. M., Stepper, M., von Schoenermark, M. F., Moritz, A., Nikoghosyan, A. S. A link between critical transition temperature and the structure of superconducting YBa₂Cu₃O_7-δ. Acta Astronautica 2008, 62, 733—736.
23. Rohlf, J. W.: Modern Physics from α to Z⁰. Wiley, New York (1994).
24. ICDD. ICDD PDF Data Base; ICDD: 12 Campus Blvd, Newtown Square, PA 19073, USA (accessed on 12. 02. 2022).
25. Materials Project Database V2019.05. Available online: https://materialsproject.org/ (accessed on 12. 02. 2022).
26. Uemura, Y. J.; Le, L. P.; Luke, G. M.; Sternlieb, B. J.; Wu, W. D.; Brewer, J. H.; Riseman, T. M.; Seaman, C. L.; Maple, M. B.; Ishikawa, M.; Hinks, D. G.; Jorgensen, J. D.; Saito, G.; Yamochi, H. Basic Similarities among Cuprate, Bismuthate, Organic, Chevrel-Phase, and Heavy-Fermion Superconductors Shown by Penetration Depth Measurements. Phys. Rev. Lett. 1991, 66, 2665—2668.
27. Uemura, Y. J. Condensation, excitation, pairing, and superfluid density in high-T_c superconductors: the magnetic resonance mode as a roton analogue and a possible spin-mediated pairing. J. Phys.: Condens. Matter 2004, 16, S4515—S4540.
28. Emery, V. J.; Kivelson, S. A. Importance of phase fluctuations in superconductors with small superfluid density. Nature 1995, 374, 434—437.
29. Talantsev, E. F.; Mataira, R. C.; Crump, W. P. Classifying superconductivity in Moiré graphene superlattices. Sci. Rep. 2020, 10, 212.
30. Ghosh, K. J. B.; Kais, S.; Herschbach, D. R. Dimensional interpolation for metallic hydrogen. Phys. Chem. Chem. Phys. 2021, 23, 7841—7848.
31. Koblischka, M. R.; Koblischka-Veneva, A. Calculation of T_c of superconducting elements with the Roeser-Huber formalism. Metals