Energy Harvesting from Fluid Flow Using Piezoelectric Materials

Version	Summary	Created by	Modification	Content Size	Created at	Operation
1		Ahsan Ali	--	4686	2022-12-15 08:57:58	\|
2	Format correction	Sirius Huang	Meta information modification	4686	2022-12-16 04:55:25	\|

This entry is adapted from the peer-reviewed paper 10.3390/en15197424

The use of piezoelectric-energy harvesting has grown in popularity during the previous two decades. Several implantable energy-harvesting technologies are being developed by scientists to power portable gadgets and medical equipment. Thanks to new technologies, researchers have been able to fabricate highly flexible piezoelectric devices and electronics, paving the way for the development of biocompatible and long-lasting harvesters.

energy harvesting piezoelectric materials fluid flow wind flow

1. Introduction

Power and electricity generation from piezoelectric materials has been studied for the last few decades ^[1]. To improve energy harvesting, different techniques have been reviewed. Piezoelectric devices are most broadly used for their simplicity and for being lightweight ^[2]. In energy harvesting, the energy production from fluid movement is essential. The mechanical energy of fluids is transformed into electrical energy through piezoelectric materials. Different piezoelectric materials can operate and accumulate this energy directly ^[3]. High-density kinetic energy is relatively available in flowing media, so the K.E of fluids can provide an opportunity to be the power source of electronic devices. Piezoelectric transducers are widely used to transform fluid kinetic energy into valuable electrical energy. Due to the importance of fluid movement in daily life, such as wind and water, energy harvesting from these sources is essential. Energy harnessing from fluid flow has a prominent probability in channels and pipelines ^[4]^[5]. Below, the energy harvesting from water and wind is discussed.

2. Wind Flow

Renewable and green energy resources are significant provocations we face nowadays. Vibrations very commonly produce electrical energy by using piezoelectric transducers ^[6]. Within the energy sources, wind is the encouraging source that is massively used on a large scale for energy production, but less attention is paid on a small scale ^[7]. Wind energy is inextinguishable and exists almost everywhere in the surroundings. Wind helps in alternative power supplies such as self-driven or automatic wireless sensor networks (WSNs) that need sophisticated monitoring software. Maximizing wind energy usage can generate more power and, hence, reduce the energy crisis. Windmills and wind turbines are commonly used for power generation. 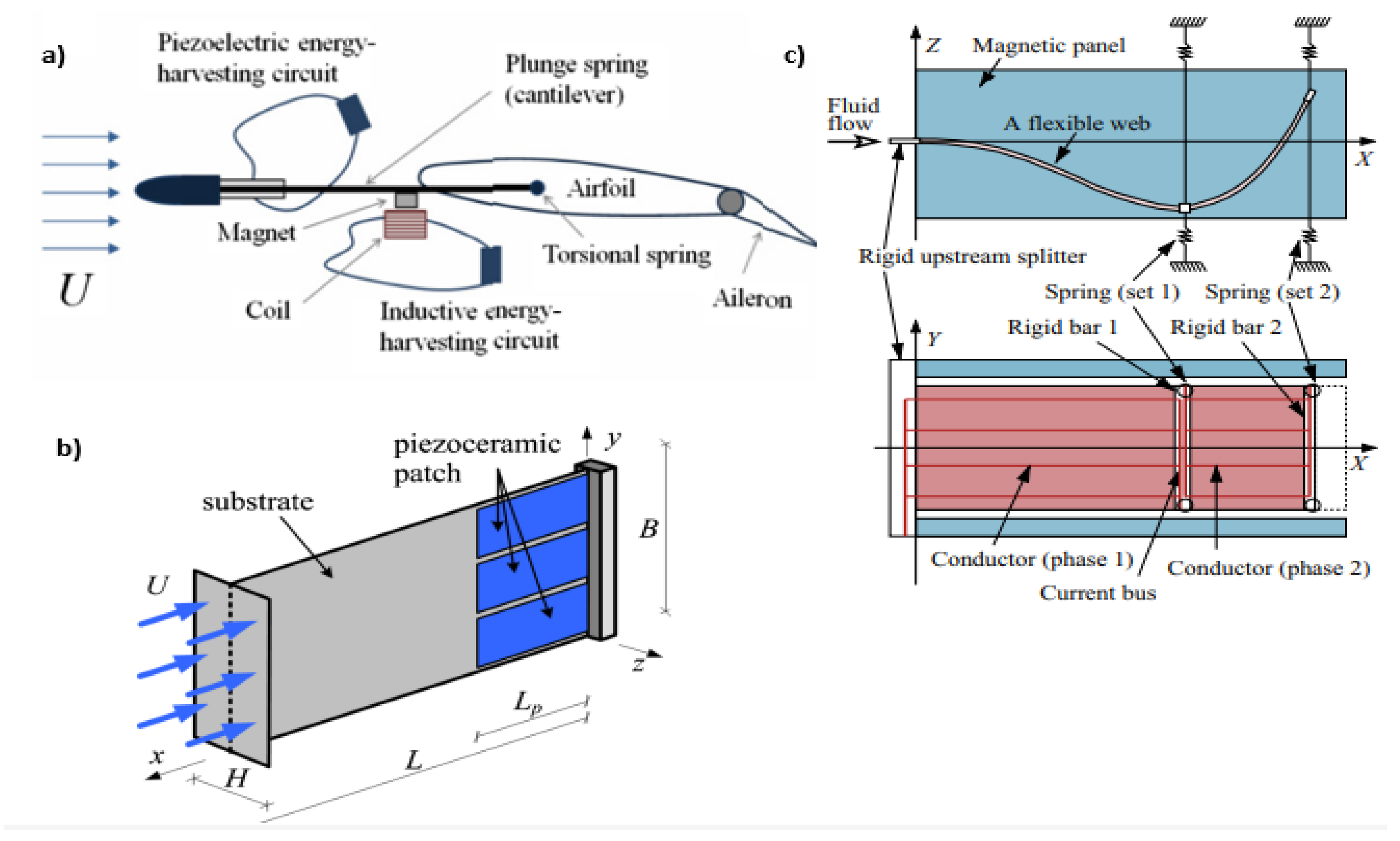The boundary-layer effect and physical hindrances like trees, buildings, and smooth surfaces cause a correspondingly low speed of wind near the surface and act as limitations of energy harvesting ^[8]^[9]. In ^[10], the power generation from wind is illustrated as the impact force generated on the piezo film when power-carrying wind strikes it. An induced electric charge is produced when the wind force generates stress waves in piezo film and a resulting strain in the piezoelectric components. Furthermore, this rectified electric current can be stored in the capacitor, and the stress that produces the current is time dependent.

\frac{P_{w i n d}}{S} = \frac{1}{2} ρ_{a i r} ν^{3}

where

ρ_{a i r}

is the density of air which is approximately equal to 1.225 kg/m³ at room temperature. At wind speed ν, suppose that the flow is laminar ^[10].

Some challenges were encountered while recording the wind energy on a micro-power scale for generating sensor nodes: (i) in the region with shunted speed and periodic wind patterns, sensor nodes can be distributed; (ii) sometimes, wind energy harvesters are required to be utilized at a tiny cut-in wind speed (e.g., lower than 2 m/s); and (iii) the voltage level required by electronic devices so that from 1 to 3 V should be delivered by low-speed wind power energy harvesters ^[11]. The above-indicated challenges can be addressed by the small voltage and maximized power density vibrational frequencies of a piezoelectric energy harvester rather than the low voltage and speed of electromagnetic energy harvesters ^[12]^[13]^[14]. There are several ways by which energy can be harvested from wind, including windmills and wind-turbine-style harvesters, flutter-style harvesters, vortex-induced vibrations, and galloping.

2.1. Windmills and Wind-Turbine-Style Harvesters

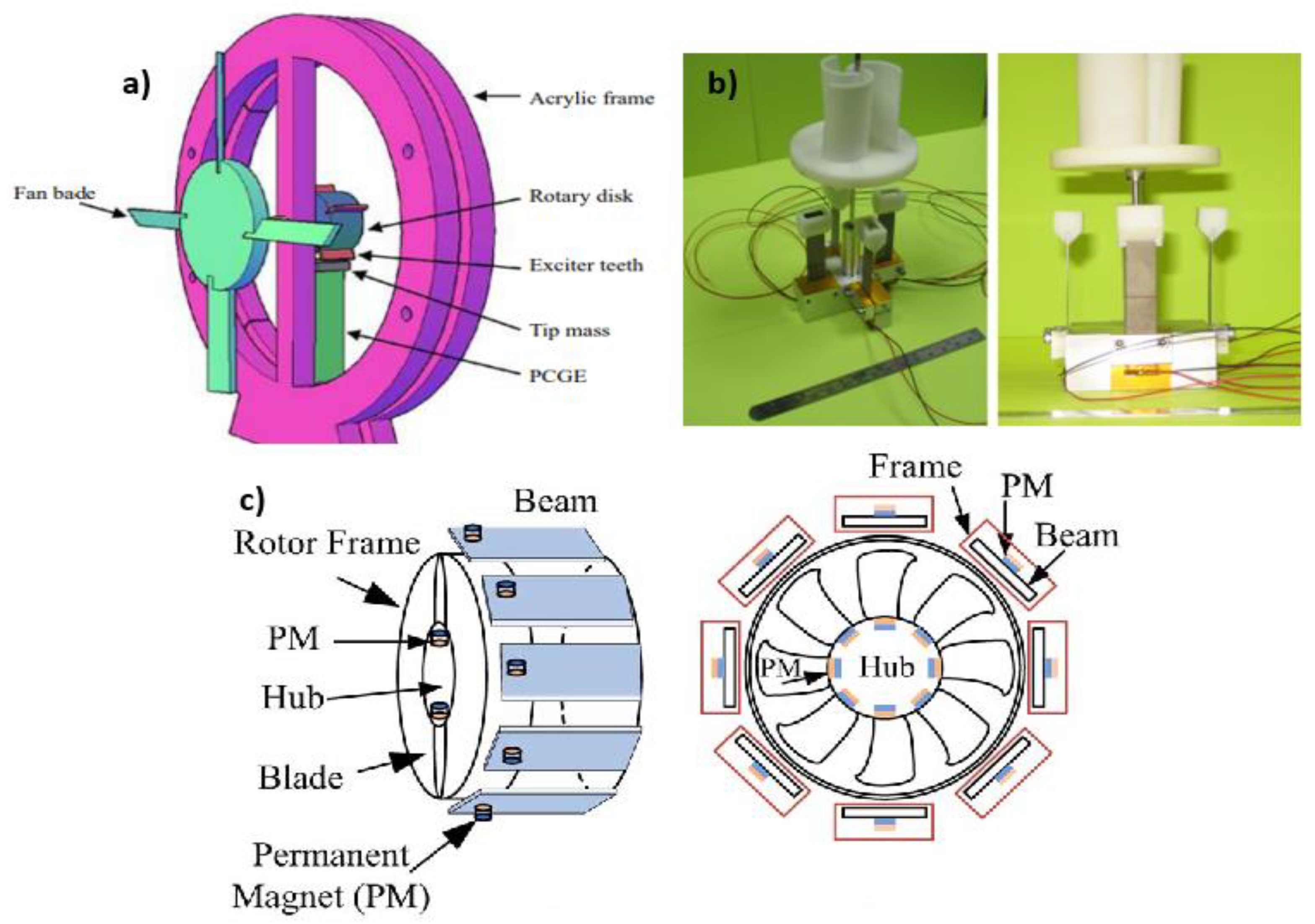
Because of wind availability and its continuous mechanical energy, harvesting energy from wind seems more attractive than from many other sources. Tien and Goo proposed a single PZT composite cantilever windmill harvester concept in 2010 ^[15]. A standard fan with exciter teeth was attached to the turbine’s shaft to gather energy. A different harvester model was built in a wind tunnel with several bimorphs installed. Yang et al. ^[16] also presented a novel windmill harvester design with a rotating fan polygonal arrangement. It consists of 12 piezoelectric bimorphs and 3 steel balls inside the polygon. As shown in Figure 1, power will be produced when the wind rotates the windmill, resulting in the piezoelectric energy harvesters being stuck with the steel balls.

Figure 1. (a) Windmill with exciter teeth; (b) piezomagnetic windmill with no contact; (c) multi-magnet windmill with no connection ^[17].

Power generation by centimeter-scale windmills was inspected by Rancourt et al. ^[17]. An electromagnetic transduction mechanism was used to produce power. Three propellers with a diameter of 4.2 cm and four blades having different pitch angles were tested as prototypes in the wind tunnel. This experiment’s result shows that the “Schmitz theory” of substantial wind turbines applies to the smallest-scale wind turbines, but there is a sharp decline in the power generation at low wind speed because of frictional losses in internal electric resistance and the generator. Similarly, several other studies on windmills and wind turbines were accomplished by Bansal et al. ^[18], Howey et al. ^[19], Priya et al. ^[20], Karami et al. ^[21], Bressers et al. ^[22], Chen et al. ^[23], and numerous others.

Their studies show that all of them use windmills and turbines having different numbers of bimorphs. Some of them change the alignment of these bimorphs from circular to perpendicular. Compared to circular arrays, they were far easier to fabricate and were space efficient. Still, their drawback was that dissipation of kinetic energy occurs and mechanical damage is caused by the fatigue issues of piezoelectric cantilevers. So, to reduce this issue, a few introduced magnetic interaction by using a magnet at the tips of transducers. High output power, small cut-in wind speed, and a hefty operative range of wind speed can be obtained by both conventional and non-uniform parametric excitation techniques. Figure 2 and Figure 3 show the schematics and graphs of small-scale windmills and wind turbines.

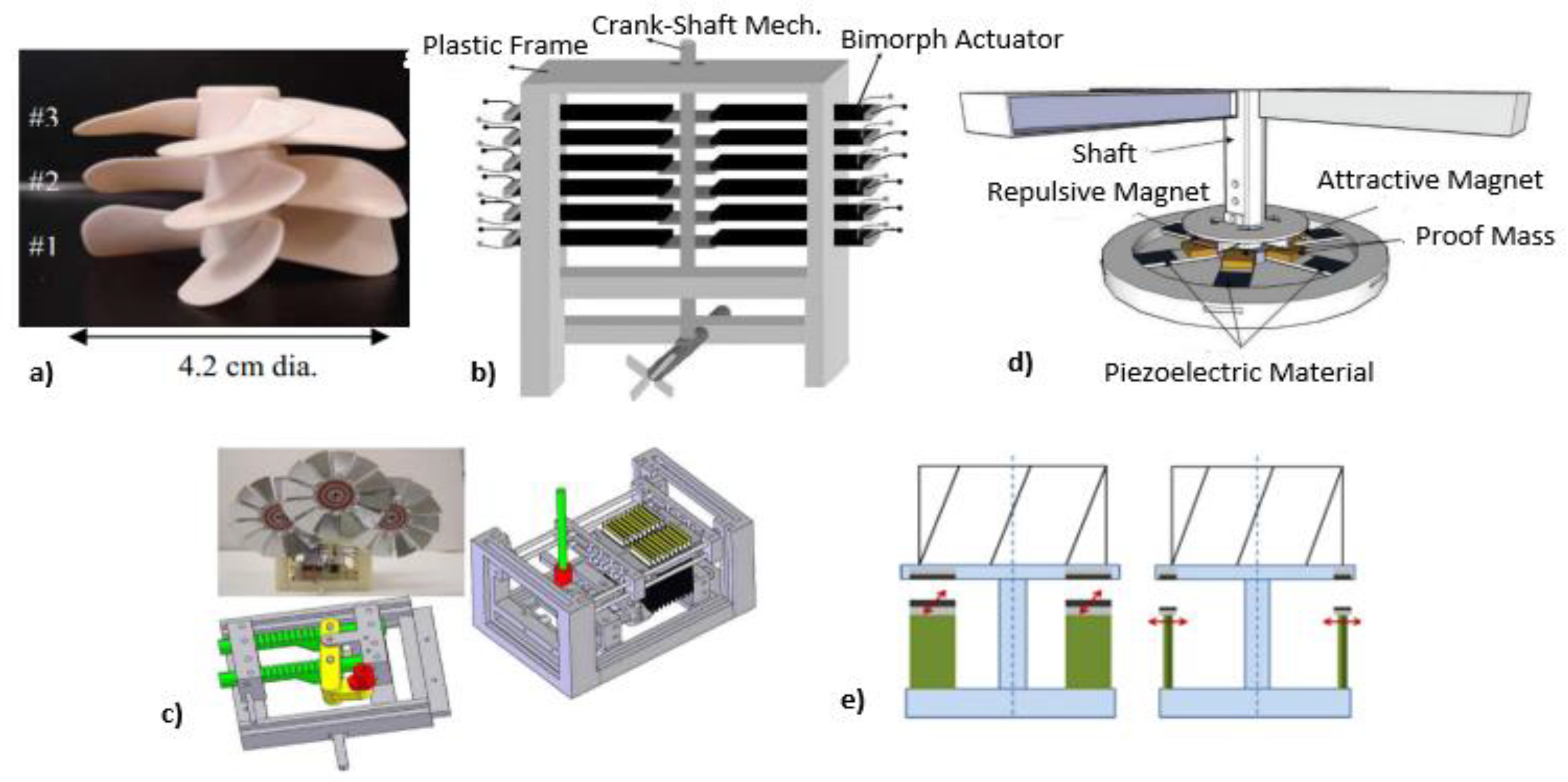
Figure 2. (a) Testing of three propellers with different pitch angles; (b) pictorial view of rectangular piezoelectric windmill; (c) schematic of optimized three-fan blades in rectangular piezoelectric windmill; (d) contactless piezoelectric wind-turbine schematic featuring magnetically driven piezoelectric elements; (e) unregular piezoelectric wind-turbine schematic with parallel and circular configurations ^[24].

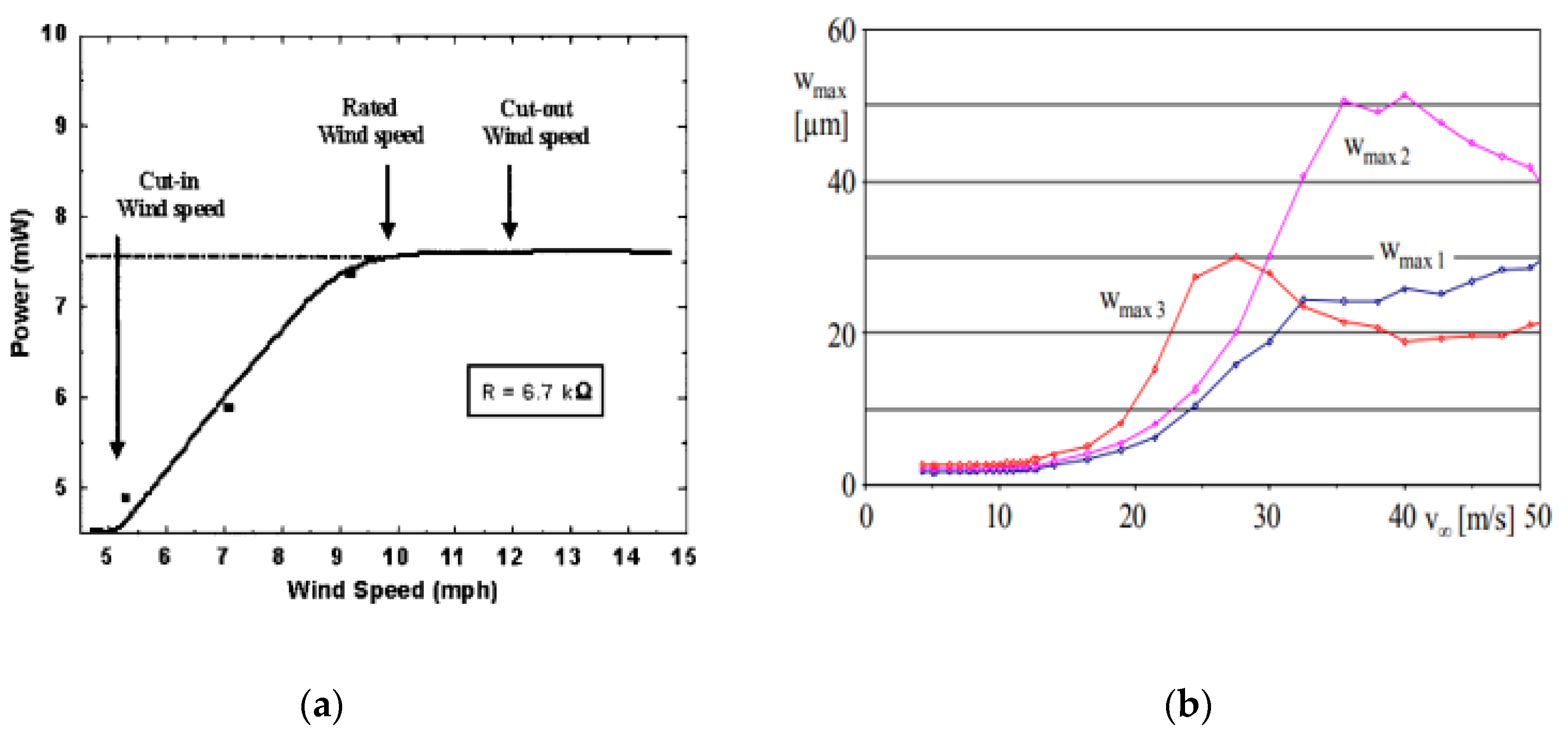
Figure 3. (a) Wind speed for the piezoelectric windmill with the variation in power; (b) at a wind speed of 40 m/s, measured voltage and power vs. external load using Series 1 and 2 cantilevers, with index “short” indicating L/D = 2.125 and “long” showing L/D = 5.057 ^[24].

2.2. Flutter Style Harvesters

Flutter is an evanescent structure aeroelastic instability in an exceedingly fluid flow. Different kinds of flutters can depend on categorizing their excitement and nourishment mechanisms ^[25]. Flutter-style harvesters are the second most detailed layouts from energy harvesting to the flowing fluid. Several problems related to windmill-style harvesters using harvesters containing the flutter style were observed, including intricacy, high production, and maintenance price, and small-scale loss of scalability because of both friction and viscous drag ^[26]. There might be different types by which flutter-style harvesters can be possible, such as model convergence flutter, crossflow flutter, dual cantilever flutter, movement-induced excitation, and extraneously induced excitation.

Model convergence flutter is based on airfoil and aeroelastic flutter with coupled pitch-plunge movements. Using the electromagnetic transduction mechanism, Bade, McKinney, and Delauries studied energy harvesting based on airfoils a few decades ago ^[27]. Bryant and Garcia ^[28]^[29] and co-authors are one of the initial companies to study the viability of capturing piezoelectric energy using flapping wings ^[30]^[31]^[32]. Bryant and Garcia ^[29] initially characterized the wing flutter-based ecological generator in both practical and theoretical wind tunnel findings, and it was then examined in a more comprehensive theoretical modeling manner ^[32]^[33]. The impacts of different system architectural aspects on wind velocity were evaluated using experimental and analytical parameter assessments. Reference ^[33] released research on energy harvesting performance that discovered that peak power intensities and power collection efficiencies of flutter energy harvesting devices emerge at the smallest winds evaluated owing to the device’s restricted sweep range. Resultant load, area of the device, and power accessible in the flow rose as the wind speed maximize, while power density and power separation efficiency declined. Erturk and his colleagues ^[34]^[35] were also among the first to investigate the utilization of flow energy in aeroelastic wing hinges. Both theoretical and experimental evidence has shown that optimal demand corresponds to the most incredible chatter rate because of the related maximum damping effect during the power extraction process. Bryant and Garcia’s ^[36] aerodynamic nonlinearity enables oscillations of the large-amplitude limit cycle that surpass flutter velocities and energy acquisition throughout a broader spectrum of wind speeds. Sousa et al. studied the conceptual and applied benefits of leveraging nonlinearities in the geometry of the piezo aeroelastic energy harvesting technique. Free-play nonlinearity has been studied theoretically and empirically and was proven to lower by 2 m/s in reduced wind speed and maximize electricity production ^[37]. Dias and colleagues then developed a three-dimensional airfoil-based hybrid aeroelastic harvester with integrated inductive and piezoelectric couplings. The 3DOF architecture has improved harvester productivity by implementing a wider design domain and a broader operational optimization parameter ^[37]. Boragno et al. also discussed their model convergence-based flutter-based energy harvesting experiment. The investigation was carried out on wing mass, elastic constants, the center of gravity’s position, and the elastomers’ elastic adhesion point that were used to support the two wings to give them bending and torsion rigidity simultaneously. 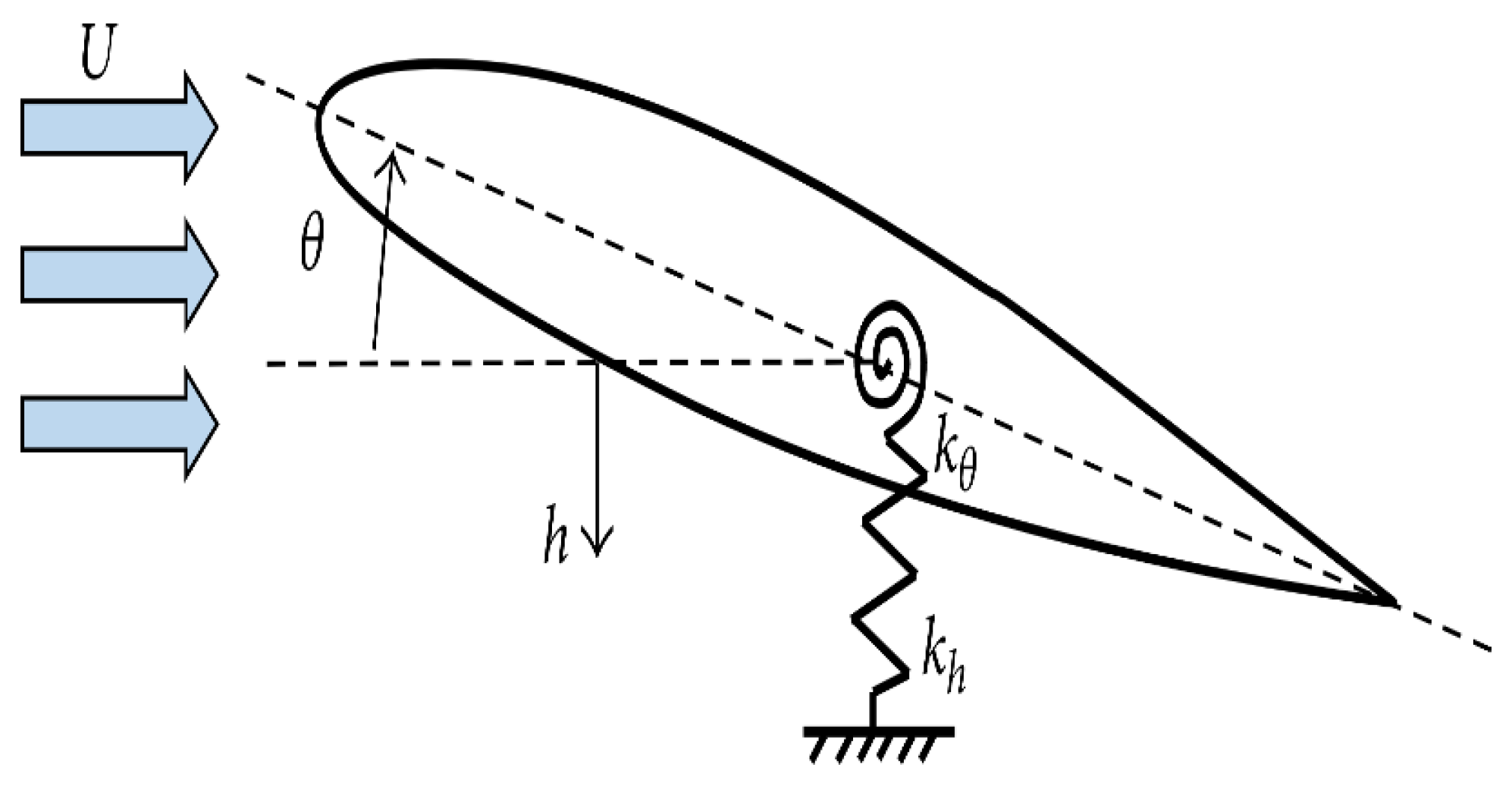It was concluded that self-sustained oscillation with properly tunned parameters is suitable for energy harvesting, as shown in Figure 4.

Figure 4. Schematic of an airfoil going through model convergence flutter.

Energy harvesters rely on crossflow flutter. The genuine fluttering leaves of a tree subjected to background fluxes inspired this piece. Li and Lipson ^[38] proposed a device consisting of a polyvinylidene fluoride or polyvinylidene difluoride stock, a plastic pivot, and a three-dimensional polymer “leaf”. The vertical and the horizontal stalk leaf were examined as two alternative layouts with various stalk axis orientations. The highest power density of 615 W was attained with a linked dual-membrane stock of 72 × 16 × 0.41 mm at 8 m/s on a 5 M power, while the highest power density of 2036 W/m³ was attained at 7 m/s on a 30 M force, using a unimorph-limited stem measuring 41 × 8 × 0.205 mm. Like the airfoil-based piezo aeroelastic energy harvester, the upright stalk bends as the leaf experiences paired twisting and torsional movements around the joint, resulting in modal convergence flutter. Piezoelectric materials like PVDF and their copolymers are significant and promising. However, their applications are restricted because of the much-decreased piezoelectricity. As a result, several initiatives to enhance piezoelectricity have been made, broadening the range of applications for it. Other preparation techniques include electrospinning, hot pressing, and solution casting. Processes such as stretching, adding fillers, heat treatment, poling, and nanofiller alignment by electric field/magnetic field have been developed, and the impact of the parameters has been examined to enhance the development of piezoelectric β-phase and the α-to-β phase transition. However, since it is still unclear how crystallinity arises, further research is required ^[39]. Different leaf shapes, leaf areas, short, long, and narrow-short stalk scales, monolayer, adherent dual-layer, and air gaps dual-layer PVDF arrangements were assessed and contrasted. The leaf’s equilateral triangle, square, and circular forms showed equivalent and maximum power, and the cut-in wind speed rose with the performance of the area of the leaf ^[40]. It has a parallel-flow asymmetric structure in which a torsional motion of the stalk around axis x was generated by the distance between the stalk and leaf axes. It is an energy extractor dependent on modal convergence flutter; however, because of Li and Lipson’s and Li’s constructs’ similarities, this part of the crossflow flutter contains its recommendations. Because the PVDF functioned in part of the d32 mode, wherein has a less piezoelectric transformation rate, it was determined that electrical d32 power from bending in a linear flow arrangement served solely as an auxiliary small-value gain as a result of folding. Although substantially less than a crossflow equivalent harvester, the production was comparable to a parallel-flow flapping-leaf harvester. De Marqui Jr. et al. also conducted studies about energy gathering from crossflow flutter ^[41]. For divided electrodes, torsional movements of the linked modes became very considerable, which was related with enhanced broadband productivity and greater flutter speed.

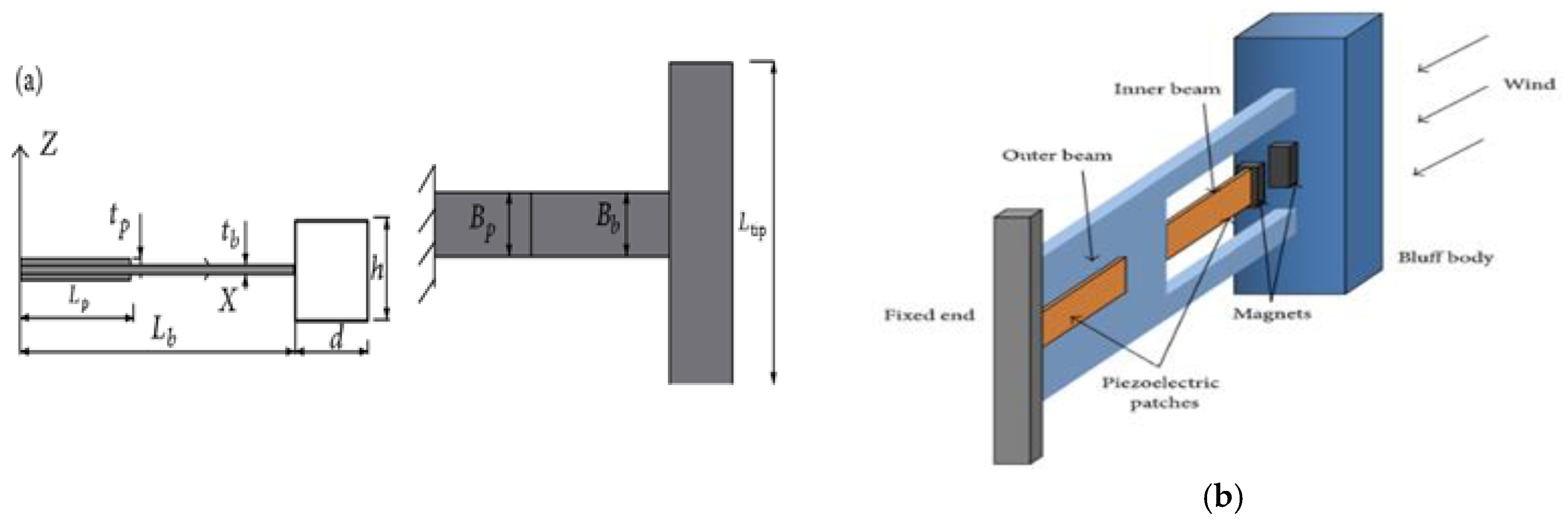
Hobeck et al. proposed another study on a novel type of flutter called a “dual-cantilever flutter”. Two cantilevers of 14.6 × 2.54 × 0.0254

{cm}^{3}

were exposed to wind flow to experience the large amplitude and persistent vibration in the experimental setup of a wind tunnel. The highest power of 0.967 mW was obtained at 13 m/s. If the distance is small, between 0.25 cm and 1 cm, the cantilever produces enough power in the range of 3 m/s to 15 m/s. This determined that the “dual-cantilever flutter” is responsible for strong energy-scavenging methods for nonlinear flowing fluid ^[42].

Movement-induced excitation (MIE) is an immersed-shape self-excited flutter followed by twisting fragility at resonance, as shown in Figure 5. Negative damping and structural deformation through divergence occur at critical flutter speed. The seminal work demonstrates that self-generated MIE flutter and self-supported flutter. The seminal work was further illustrated in ^[43]^[44]. Critical flutter speed, the relation of flutter frequency, and a two-dimensional complaint beam in viscous flow were explained based on scaling laws, as shown below in the equation.

ω ~ \sqrt{\frac{ρ f U^{2}}{ρ_{s} h L^{'}}}

U_{c} ~ \sqrt{\frac{Y h^{3}}{ρ_{f} L^{3}}}

where ω is flutter frequency,

U_{c}

is the critical flutter speed,

ρ

_f is the density of the fluid, and

ρ

_s is the density of the beam. Similarly,

U_{c}

is the flow speed, h is the thickness of the beam, L is the beam length, and Y is the elastic modulus of the beam. Recently, experimental verification on scalable rules for rectangular, tri-directional beams was followed in ^[45].

µ = \frac{ρ_{s} h}{ρ_{f} L^{'}}

where µ is the mass ratio, the above Equation (1) shows that the fraction of the non-dimensional weight is enormous. For an adequately minor mass ratio, dense effect and added mass are responsible for controlling the beam flutter motions compared to the estimated model displacement ^[46].

Figure 5. (a) Airfoil-based hybrid harvester; (b) T-shaped harvester; (c) electric current induction having a magnetic field in the flutter mill.

2.3. Vortex Induces Vibrations

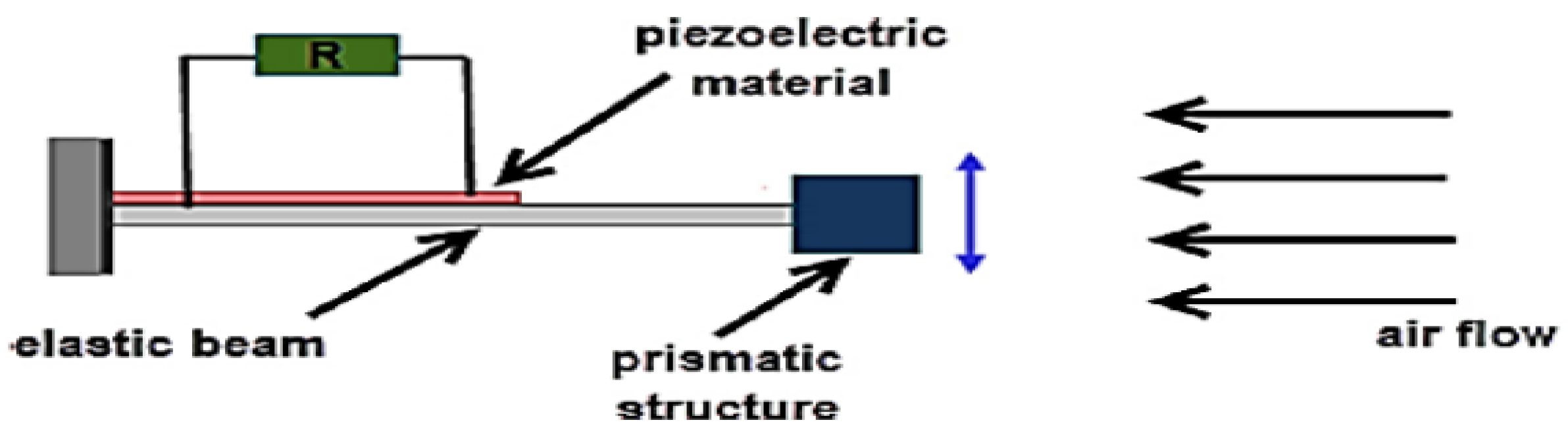
Vortex-induced vibration (VIV) was priorly investigated in water flow rather than wind flow. When bluff bodies are subjected to an external force resulting in periodic abnormalities in the flow, vortex-induced vibration (VIV) emerges. The oscillations in the underwater cylinder are an excellent illustration of vortex-induced vibration. Because of its considerable curvature, this limit layer might segregate from the body at numerous locations. The pressure distribution along the surface is then changed by the generation of vortices ^[47]. Akaydin et al. ^[48] suggested a novel self-excited vortex-induced vibrational energy harvester to boost output power. Unlike in earlier occurrences, PZT was used to cover the end area of the cylinder, which was an aluminum cantilever’s free end connected. The aeroelastic efficiency was raised from 0.032% to 2.8% compared to the prior design. The finding was that the altered arrangement of the cylinder connection to the cantilever end and the substitution of PZT for PVDF considerably improved the power extraction. Due to vortex shedding, regular oscillations are generated in the path perpendicular to the flowing wind.

Weinstein et al. ^[49] developed an energy scavenger with tunable abilities for resonance to overcome the short operating range of VIV-based harvesters and presented a resonance-tuning-capable energy harvester. An aerodynamic fin was used to affix the piezoelectric cantilever to the head of a cylinder body. The resonant frequencies of the harvester might be fine-tuned by directly altering the mass locations. Weinstein et al. ^[49] proposed an energy-harvesting device ranging from 2 to 5 m/s. Their research was centered on the means of a piezoelectric beam affixed to the tip of a cylinder to capture energy from the VIV phenomena. Gao et al. reported a spherical prolongation to a piezoelectric cantilever (PEC) is used in a wind-energy collecting device. This model was constructed to generate electricity from VIV using a nonlinear distributed 192-parameter model, confirmed by the experimental data of Akaydin et al. Using a flexible piezoelectric beam, Goushcha et al. ^[50] collected the vibration energy based on the VIV. The topic of fluid flow energy extraction through the VIV of a circular cylinder in a dual-mass configuration was examined by Xu-Xu et al. ^[51]. Wang with his colleagues ^[52]^[53]^[54] created energy harvesters in the shape of a flow path, which they claimed were operated by a bluff body in the stream producing a vortex and shedding it. The diaphragm is attached to a piezoelectric patch or a magnet and coil for energy transmission. The effects of different bluff body shapes on wind harvesters were studied by Tam Nguyen et al. ^[55]. A 0.2 mm broad polydimethylsiloxane (PDMS) diaphragm was implanted in the prototype, and a PVDF sheet was bonded up to the flow network’s peak. The prototype measured an average power of 0.59 nW and a 14 mV open-circuit voltage at a wind velocity of 20.7 m/s. Wang et al. ^[56] utilized computational fluid dynamics modeling to highlight the importance of establishing the area of synchronization for VIV energy harvesting. Lately, the so-called vortex bladeless wind generator has been used in conjunction with VIV to capture wind energy on a big scale.

3. Galloping

Various investigations have proven the likelihood of extracting meaningful energy from the surrounding flow for the significant galloping vibration. The studies of mainly transverse and torsional energy collection during galloping are discussed. The aeroelastic instability phenomena of transverse galloping have only recently been used to get vibrations inside the structure for energy gathering. Galloping, in comparison to VIV, has the characteristics of enormous oscillation amplitude and the capability to oscillate in an unbounded range of wind speeds, which is desirable for energy harnessing ^[57]. Several research studies have shown transverse galloping energy extraction’s analytical and theoretical feasibility. Barrero-Gil et al. published analytical research to demonstrate the feasibility of cross-country galloping as an energy source. Using a 1DOF model, theoretically, Barrero-Gil et al. looked at the possibility of using galloping to generate energy. There was no particular type of energy harvesting suggested. The aerodynamic force was calculated using a cubic polynomial depending on the quasi-steady hypothesis. Theoretically, a very effective bluff body was determined to have a higher A1 aerodynamic coefficient and A3’s small significance level. The mass-damper parameter must be set to a minimal value for the mechanical damping ^[58].

Rather than utilizing a polynomial fitting, Sorribes-Palmer and Sanz-Andres ^[59] extend the research by accumulating the aerodynamics curvature parameter straight-forwardly by investigation records. Sirohi and Mahadik ^[60] presented a galloping energy harvester with two 161 × 38 × 0.635 mm³ cantilever beams and a prism 251 mm in length linked to the outer end and a 40 mm symmetrical triangular cross-section in every boundary. Directly acquiring from the experiment has eliminated the difficulties involved with polynomial fitting, such as incorrect dynamic reactions caused by erroneous polynomials. Sirohi and Mahadik ^[61] devised alternative galloping with an energy harvester, such as a D-shaped cross-section coupled to the composite piezoelectric beam. The tip body has a diameter of 30 mm and a length of 235 mm, while the cantilever has a volume of 90 38 0.635 mm³. An axial fan was used to create the wind flow, which had a highly turbulent profile. With a 10.5 mph wind velocity, the power density of 1.14 mW was produced.

Abdelkefi et al. ^[62]^[63] examined the topic with an energy harvester of a galloping square cylinder in a theoretical study. They discovered that when the load resistance grew, the commencement of galloping and output electrical power improved, but the displacement was reduced. An ideal load for higher Reynolds provided maximum power output, the maximum beginning of galloping, and minimizing distance simultaneously. According to Yang et al. ^[64], and Daqaq ^[65], once all energy harvesters are configured effectively, a bluff body with a squared section generally outperforms D-shaped and equilateral three-sided objects at high wind speeds. Using the Weibull probability density function, wind data were fitted, and Bibo and Daqaq ^[65]^[66]^[67] included actual wind-data figures in the results of energy harvesters that are in motion (PDF). Vicente-Ludlam et al. ^[68] discovered that by adjusting the output tolerance to loads in the appropriate adjustable, the power and efficiency for each wind-velocity generation productivity might be maintained throughout a broader range of wind speeds, as shown in Figure 6. Putting an impact bump stop onto a galloping harvester was discovered to extend its durability significantly during electromagnetic energy collection by a dual-mass galloping.

Figure 6. (a) Schematic of galloping with an energy harvester square bluff body; (b) at low wind speed, power enhancement through 2DOF piezoelectric galloping energy harvester ^[69].

Figure 7 below shows that when the speed of flow increases from the critical value, transverse fluctuation becomes the cause to interrupt the bluff body exposed through the airflow, and this phenomenon is known as galloping. There are twin galloping bluff bodies in the wake form of PEH; one is connected to the loose at the beam’s tip, and the other is attached in its front. A primary rule for the front bluff body depends on the certainty that variations are produced in the spacing distances ^[70]^[71]^[72]. Much research has been taking place to investigate the impact of various factors on galloping manufacturing ^[73]^[74].

Figure 7. Example of PEH-based Galloping ^[75].

All the methods and techniques of energy harvesting from wind are described in Table 1 below:

Table 1. Piezoelectric Devices in Wind Flow.

Windmill and Wind Turbine Energy Harvesting Devices
Sr No.	Device	Transducer Type	$Power Density (mW / m m^{3})$	Dimensions	Cut-In Wind Speed (m/s)	Cut-Out Wind Speed (m/s)	Wind Speed (m/s)	Relevant Information	References
1.	Fan-type windmill	Piezoelectric bimorph	0.01042	$114 mm diameter \times 60 mm width, 60 \times 20 \times 0.6 {mm}^{3}$ each beam	-	-	4.47	-	^[75]
2.	Vane-type vertical windmill	Piezoelectric bimorph	7.38 × 10⁻⁶	96 × 107 × 66 mm³ generator, 178 mm vane diameter	-	-	4.47	-	^[76]
3.	Fan-type windmill	Piezomagnetic generator PZT-5A	-	31 mm diameter	0.9	-	0.9	-	^[77]
4.	Fan-type windmill using impact-induced resonance	Piezoelectric unimorph PZT ceramic	-	31 mm diameter	-	-	Not specified (fan with the speed of 200 RPM rotation)	-	^[78]
5.	Contactless windmill, Savonius turbine	Piezomagnetic generator	1.9 × 10⁻⁷	$165 \times 165 \times 229 {mm}^{3}$	-	-	4.02	Fabrication is easy. Comparatively much less power than circular windmill. Due to vibration phase of all bimorphs combined circuit can be used Have enough space because of rectangular-array arrangement of transducers	^[78]
6.	Small-scale windmill and turbine	Piezoelectric material. Contact via mechanical stopper	0.00267	$5.08 \times 11.6 \times 7.62 {cm}^{3}$	2.1	6.2	5.4		^[79]
Flutter Energy Harvesting Devices in Wind Flow
Sr No.	Device	Transducer Type	$Power Density (mW / m m^{3}$ )	Wind Speed (m/s)	Cut-In Wind Speed (Flutter speed) (m/s)	Cut-Out Wind Speed (m/s)	Dimensions	Relative Information	References
1.	Rigid flap with revolute joint	Bimorph_ PZT	6.3 × 10⁻⁵	7.9	2.6	-	$283.7 \times 136 \times 0.9 {mm}^{3}$	-	^[79]
2.	The piezoelectric film with plastic extension	Rectangular film PVDF	$0.0524 µ W / {mm}^{3}$	2	-	-	$22 \times 13 \times 0.2 {mm}^{3}$	-	^[79]
3.	Piezoelectric grass	Bimorph	0.0038	11.5	-	-	$Four 101.6 \times 25.4 \times 0.406 {mm}^{3}$ beams	-	^[80]
4.	Inverted flag	Piezoelectric membrane	0.00347	9	-	-	$60 \times 120 \times 0.2 {mm}^{3}$	-	^[80]
5.	Modal convergence flutter with flat plate	Piezoelectric	Stiff host: 6.078 Compliant host: 4.696	Stiff host: 26 Compliant host: 25	Stiff host: 17.3 Compliant host: 15.2	Stiff host: 29 Compliant host: 29	Flat plate tip: span 6 cm, chord 3 cm, thickness 0.79 mm. $Cantilever : 7.6 \times 2.5 \times 0.0381 {cm}^{3}$	Wind speed and intensity have decreased, reduced in strong host as opposed to a compliant host In the conforming host architecture, the power peak is shifted toward lower wind speed	^[80]
6.	Dual cantilever flutter	Piezoelectric	0.845	13 approx.	3 approx.	-	$Two identical cantilevers : 14.6 \times 2.54 \times 0.0254 {cm}^{3}$	Efficient power generation with high operational wind speed	^[81]
Micro Scale Piezoelectric Wind Energy Harvesters
Sr No.	Transducer	Mechanical Mechanism	Size (mm)	Peak Power	Voltage	Wind Speed(m/s)	Power Coefficient Cp (%)	Normalized Power Density (μW × s/ (mm³ × m))	References
1.	Piezoelectric	Aeroelastic	2 × 1.65 × 0.005	34 nW	25 mW	5.2	0.012	0.39	^[81]
2.	Piezoelectric	Rotational	47 × 20 × 0.5	613 µW	13 V	200 r/min	-	-	^[82]
3.	Piezoelectric	Aeroelastic	75 × 20 × 0.004	0.98 µW	1.2 V	3.9	0.002	0.041	^[82]
4.	Piezoelectric	Rotational	ϕ = 53	7.5 mW	5 V	4.47	6.32	-	^[83]
5.	Piezoelectric	Aeroelastic	23 × 4 × 0.130	0.64 µW	1.6 V	15	$3.4 \times 10^{- 4}$	0.004	^[84]
6.	Piezoelectric	Aeroelastic	58 × 10 × 0.202	30 µW	4.3 V	5	0.06	0.05	^[85]
7.	Piezoelectric	Aeroelastic	3 × 8 × 0.035	2.27 µW	965 mV	16.3	0.004	0.17	^[85]
8.	Piezoelectric	Aeroelastic	69 × 37 × 0.24	3.3 V	1 mW	2	8.1	0.81	^[86]
Macro Scale Piezoelectric Wind Energy Harvesters
Sr No.	Transducer	Mechanical Mechanism	Size (mm)	Voltage	Power Peak	Wind Speed(m/s)	Power Co-efficient Cp (%)	Normalized Power Density (μW × s/ (mm³ × m))	References
1.	Piezoelectric	Aeroelastic	76.7 × 12.7 × 2.2	8.8 V	155 µW	6.7	0.09	0.01	^[86]
2.	Piezoelectric	Rotational	ϕ = 80, t = 170	80 mV	2 µW	14	$2.4 \times 10^{- 5}$	$1.7 \times 10^{- 7}$	^[87]
3.	PZ	Aeroelastic	90 × 10 × 0.6	12 V	145 µW	3.5	0.63	0.08	^[88]
4.	PZ	Rotational	325 × 36.2 × 0.267	30 V	1.14 mW	4.96	0.15	0.077	^[89]

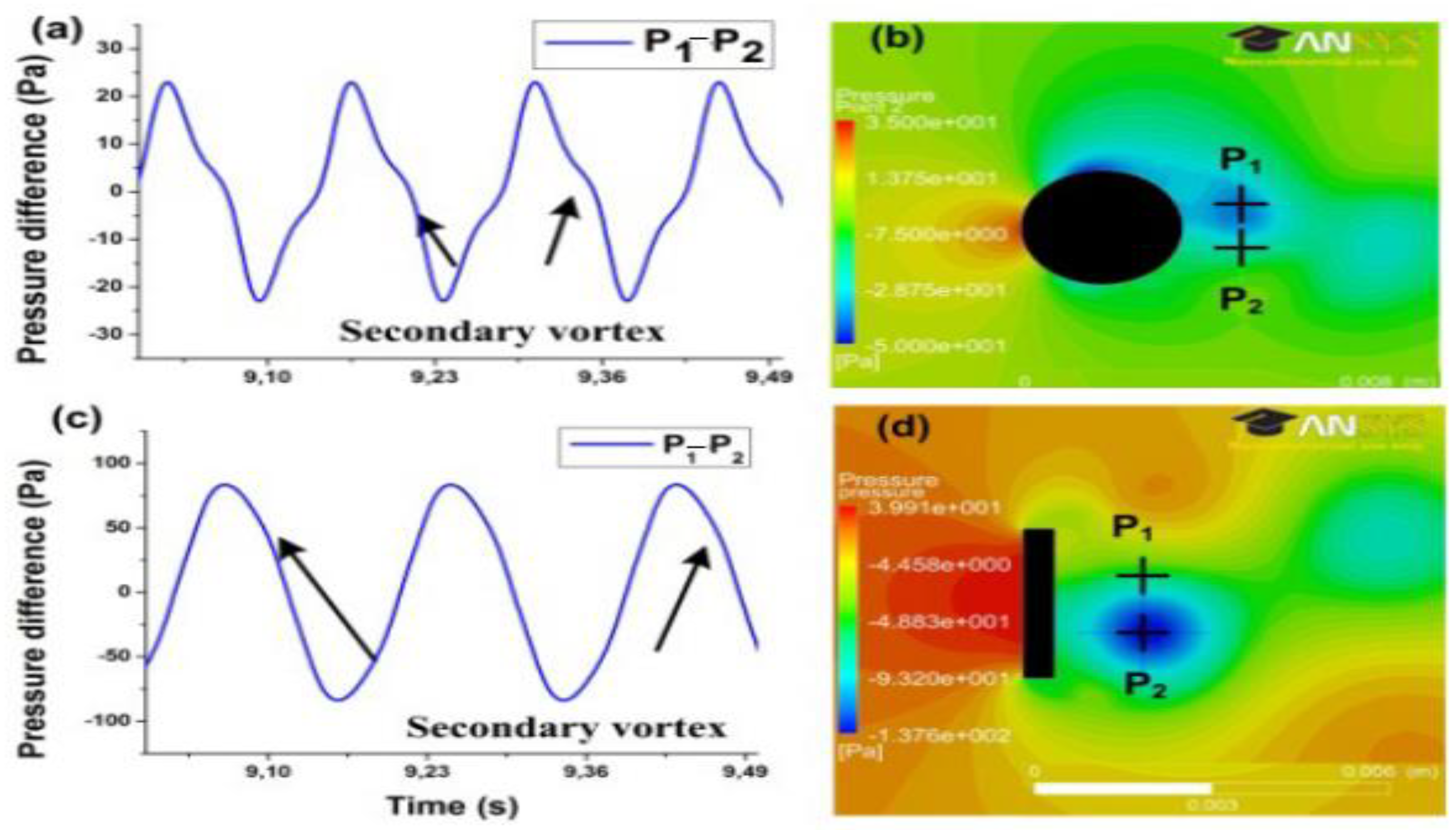
Many bluff body designs were studied to increase the wake flow’s pressure from the vortex. Researchers conducted computational fluid dynamics (CFD) models to understand better vortex formation and how it affects the harvester efficiency. When a half-reversal velocity occurs when the river passes, the bluff body velocity difference occurs because of fluid pulls causing the vortex to form. Viscous shearing between the solid object and the fluid layer generates friction drag. Maximizing the velocity ratio, k, generates an inner kinetic energy reduction throughout the bluff body’s longitudinal distance.

As a result, the length is reduced by using a bluff body with a cuboid form. Because of the steep flow detachment point, the primary edge might move toward the inflow area, increasing vortex pressure. According to CFD simulation results, the vortex pressure in the wake of a cuboid-shaped bluff body reaches 84 Pa at 2 m/s air flow (Figure 8c). This is roughly four times larger than a cylinder-shaped bluff body, as seen in Figure 8a. The simulation’s outcomes indicate the shedding of an additional vortex that flows following the primary vortex (Figure 8a,c). It was supposed to be more dampening, which would result in poor harvester performance ^[89]. Table 2 shortlists all the techniques that help in energy harvesting in wind flows from piezoelectric devices.

Figure 8. (a) Comparison of vortex pressure in the cylindrical bluff body wake; and (b) associated pressure difference; (c) when a vortex is present in the cubical bluff body wake; and (d) associated pressure difference ^[89].

Table 2. Techniques of PEH in Wind Flow.

Ref.	Technique	Brief Title	Highlights	Authors
^[90]	VIV	Flow-induced based on PEH	Utilizing a one-dimensional approach to investigate the function of an adaptable piezoelectric cylinder, the produced power	Xie et al.
^[91]	VIV	Tree-simulated piezoelectric	Four cylindrical linear arrays connected to piezoelectric actuator were tested in the wind.	Hobbs and Hu.
^[92]	VIV	Designing of fluid K.E harvesters	The harvester was optimized using a cuboid-shaped bluff body and a 1 μ W output power.	Wen et al.
^[92]	VIV	Air conditioners containing piezoelectric	A piezoelectric energy-harvesting system was offered based on VIV with a fin for use in turbulent airflow.	Weinstein et al.
^[92]	VIV	Cylindrical extension on piezoelectric cantilevers	A wind-driven cylinder with a piezoelectric beam attached to the edge has been described as an energy harvester.	Gao et al.
^[92]	VIV	Piezoelectric analysis with non-uniformity	Investigating a piezoelectric cantilever ray connected to the radial cylinder subjected to nonuniform distribution structure.	Dai et al.
^[93]	VIV	Fluidic energy with flexible beam	By the usage of flexible beam piezoelectric harvesting vibrational energy construct of VIV.	Dunnmon et al.
^[93]	VIV	Circular cylinder of VIV	Enhancing the efficiency of dual-mass development compromises on a circular cylinder.	Dunnmon et al.
^[93]	Flapping	Oscillations in the aeroelastic limit cycle	In a wind s velocity of 27 m.s-1, piezoelectric plates were put on a floppy sheet.	Dunnmon et al.
^[94]	Flapping	Cross flow fluttering by the usage of wind harvesters	Wind energy was turned into electrical energy by a swinging leaf dependent on aeroelastic fluttering.	Li et al.
^[95]	TIV	TIV comprising of artificial piezoelectric grass	TIV was studied using a piezoelectric grass and a variable structure model.	Hobeck and Inman
^[95]	TIV	TIV with a mathematical framework	TIV force of a unimorph cantilever was given by scattered a computational model.	Hobeck and Inman
^[96]	TIV	Micromachined piezoelectric harvesting	2W of energy is produced by a MEMS piezoelectric and a Helmholtz oscillator that includes a packed harvester.	Matova et al.
^[97]	TIV	Unsteady flows for piezoelectric	Flexible piezoelectric cantilever beams were positioned in turbulent boundary layers to generate 0.06 W.	Akaydin et al.
^[97]	TIV	Wake of a cylinder	Applying piezoelectric beams, the greater Reynolds values were used to study the effects of radial cylinders.	Akaydin et al.
^[98]	TIV	Turbulent boundary layers in piezoelectric transducers	In a turbulent boundary layer, several piezoelectric beams were examined.	Goushcha
^[99]	FIV	In axial flows, the performance of piezoelectric flags	A nonlinear variant of the FIV was proposed for a moving plate in an axial flow using piezoelectric patches.	Michelin and Doare.
^[100]	FIV	Piezoelectric cantilever of T-shaped	Depending on the aeroelastic oscillation, a T-shaped piezoelectric cantilever was demonstrated.	Kwo
^[101]^[102]	FIV	Single piezoelectric generator of aerodynamic	A piezo-aeroelastic energy harvesting system that depends on flutter was studied.	Bibo and Daqaq.
^[103]	FIV	Aeroelasticity nonlinearity	Piezoelectric transmission and airfoil form nonlinear investigation methods.	Dowell et al.
^[104]	FIV	Piezoaeroelastics analysis	To prevent pressurized collapse of piezo-aeroelastic harvesters, a nonlinear model was investigated.	Abdelkefi et al.
^[105]	Galloping	Low-power sensors containing wind harvesters	In an air velocity of 11.6 miles per hour, a galloping-based piezoelectric yielded a captured energy of moreover 50 mW.	Sirohi and Mahadi.

References

Shan, X.; Song, R.; Liu, B.; Xie, T. Novel energy harvesting: A macro fiber composite piezoelectric energy harvester in the water vortex. Ceram. Int. 2015, 41 (Suppl. S1), S763–S767.
Safaei, M.; Sodano, H.A.; Anton, S.R. A review of energy harvesting using piezoelectric materials: State-of-the-art a decade later (2008–2018). Smart Mater. Struct. 2019, 28, 113001.
Abrol, S.; Chhabra, D. Harvesting piezoelectricity using different structures by utilizing fluid flow interactions. Int. J. R D Eng. Sci. Manag. 2017, 5, 24–36.
Chen, Z.; Guo, B.; Yang, Y.; Cheng, C. Metamaterials-based enhanced energy harvesting: A review. Phys. B Condens. Matter 2014, 438, 1–8.
Anton, S.R.; Sodano, H.A. A review of power harvesting using piezoelectric materials (2003–2006). Smart Mater. Struct. 2007, 16, R1–R21.
Tien, C.M.T.; Goo, N.S. Use of a piezo-composite generating element for harvesting wind energy in an urban region. Aircr. Eng. Aerosp. Technol. 2010, 82, 376–381.
Zeng, P.; Khaligh, A. A Permanent-Magnet Linear Motion Driven Kinetic Energy Harvester. IEEE Trans. Ind. Electron. 2013, 60, 5737–5746.
Tang, Q.; Li, X. Two-Stage Wideband Energy Harvester Driven by Multimode Coupled Vibration. IEEE ASME Trans. Mechatron. 2015, 20, 115–121.
Sankman, J.; Ma, D. A 12-μW to 1.1-mW AIM Piezoelectric Energy Harvester for Time-Varying Vibrations with 450-nA. IEEE Trans. Power Electron. 2015, 30, 632–643.
Dallago, E.; Barnabei, A.L.; Liberale, A.; Malcovati, P.; Venchi, G. An Interface Circuit for Low-Voltage Low-Current Energy Harvesting Systems. IEEE Trans. Power Electron. 2015, 30, 1411–1420.
Ziegler, S.; Woodward, R.C.; Iu, H.H.-C.; Borle, L.J. Current Sensing Techniques: A Review. IEEE Sens. J. 2009, 9, 354–376.
Tan, Y.K.; Panda, S.K. Self-Autonomous Wireless Sensor Nodes with Wind Energy Harvesting for Remote Sensing of Wind-Driven Wildfire Spread. IEEE Trans. Instrum. Meas. 2011, 60, 1367–1377.
Zhao, L.; Yang, Y. Toward Small-Scale Wind Energy Harvesting: Design, Enhancement, Performance Comparison, and Applicability. Shock Vib. 2017, 2017, 3585972.
Ovejas, V.J.; Cuadras, A. Multimodal piezoelectric wind energy harvesters. Smart Mater. Struct. 2011, 20, 85030.
Yadav, N.; Baredar, P. A review on piezoelectric energy harvesting system using vortex induced vibration. AIP Conf. Proc. 2018, 2039, 020054.
Yang, Y.; Shen, Q.; Jin, J.; Wang, Y.; Qian, W.; Yuan, D. Rotational piezoelectric wind energy harvesting using impact-induced resonance. Appl. Phys. Lett. 2014, 105, 53901.
Rancourt, D.; Tabesh, A. Evaluation of centimeter-scale micro windmills: Aerodynamics and electromagnetic power generation. Dig. Power MEMS 2017, 2007, 93–96.
Bansal, A.; Howey, D.A.; Holmes, A.S. CM-scale air turbine and generator for energy harvesting from low-speed flows. In Proceedings of the TRANSDUCERS 2009—2009 International Solid-State Sensors, Actuators and Microsystems Conference, Denver, CO, USA, 21–25 June 2009; pp. 529–532.
Howey, D.A.; Bansal, A.; Holmes, A.S. Design and performance of a centimetre-scale shrouded wind turbine for energy harvesting. Smart Mater. Struct. 2011, 20, 85021.
Priya, S.; Chen, C.-T.; Fye, D.; Zahnd, J. Piezoelectric Windmill: A Novel Solution to Remote Sensing. Jpn. J. Appl. Phys. 2004, 44, L104–L107.
Karami, M.A.; Farmer, J.R.; Inman, D.J. Parametrically excited nonlinear piezoelectric compact wind turbine. Renew. Energy 2013, 50, 977–987.
Bressers, S.; Avirovik, D.; Lallart, M.; Inman, D.J.; Priya, S. Contact-less Wind Turbine Utilizing Piezoelectric Bimorphs with Magnetic Actuation. Struct. Dyn. 2011, 3, 233–243.
Chen, C.-T.; Islam, R.A.; Priya, S. Electric energy generator. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2006, 53, 656–661.
Liya, Z. Small-Scale Wind Energy Harvesting Using Piezoelectric Materials. Nanyan Techonol. Univ. 2021, 2015.
Allen, J.J.; Smits, A.J. Energy Harvesting EEL. J. Fluids Struct. 2001, 15, 629–640.
Gao, X.; Shih, W.-H.; Shih, W.Y. Flow Energy Harvesting Using Piezoelectric Cantilevers with Cylindrical Extension. IEEE Trans. Ind. Electron. 2013, 60, 1116–1118.
McKinney, W.; DeLaurier, J. Wingmill: An Oscillating-Wing Windmill. J. Energy 1981, 5, 109–115.
Bryant, M.; Garcia, E. Modeling and Testing of a Novel Aeroelastic Flutter Energy Harvester. J. Vib. Acoust. 2011, 133, 011010.
Bryant, M.; Garcia, E. Energy harvesting: A key to wireless sensor nodes. In Proceedings of the Second International Conference on Smart Materials and Nanotechnology in Engineering, Weihai, China, 8–11 July 2009; Volume 7493, pp. 523–530.
Bryant, M.; Tse, R.; Garcia, E. Investigation of Host Structure Compliance in Aeroelastic Energy Harvesting. Smart Mater. Adapt. Struct. Intell. Syst. 2012, 2, 769–775.
Bryant, M.; Pizzonia, M.; Mehallow, M.; Garcia, E. Energy harvesting for self-powered aerostructure actuation. In Proceedings of the Active and Passive Smart Structures and Integrated Systems, San Diego, CA, USA, 10–13 March 2014; Volume 9057, pp. 121–129.
Bryant, M.; Schlichting, A.D.; Garcia, E. Toward efficient aeroelastic energy harvesting: Device performance comparisons and improvements through synchronized switching. In Proceedings of the Active and Passive Smart Structures and Integrated Systems, San Diego, CA, USA, 10–14 March 2013; Volume 8688, pp. 33–42.
Bryant, M.; Wolff, E.; Garcia, E. Parametric design study of an aeroelastic flutter energy harvester. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 8–12 March 2011; Volume 7977.
Erturk, A.; Vieira, W.G.R.; De Marqui, C.; Inman, D.J. On the energy harvesting potential of piezoaeroelastic systems. Appl. Phys. Lett. 2010, 96, 184103.
Erturk, A.; Bilgen, O.; Fontenille, M.; Inman, D.J. Piezoelectric energy harvesting from macro-fiber composites with an application to morphing-wing aircrafts. In Proceedings of the 19th International Conference on Adaptive Structures and Technologies, Ascona, Switzerland, 6–9 October 2008; pp. 339–359.
Boragno, C.; Festa, R.; Mazzino, A. Elastically bounded flapping wing for energy harvesting. Appl. Phys. Lett. 2012, 100, 253906.
Sousa, V.C.; Anicézio, M.D.M.; De Marqui, C., Jr.; Erturk, A. Enhanced aeroelastic energy harvesting by exploiting combined nonlinearities: Theory and experiment. Smart Mater. Struct. 2011, 20, 94007.
Li, S.; Lipson, H. Vertical-Stalk Flapping-Leaf Generator for Wind Energy Harvesting. Smart Mater. Adapt. Struct. Intell. Syst. 2009, 21, 611–619.
Wu, L.; Jin, Z.; Liu, Y.; Ning, H.; Liu, X.; Hu, N. Recent advances in the preparation of PVDF-based piezoelectric materials. Nanotechnol. Rev. 2022, 11, 1386–1407.
Deivasigamani, A.; McCarthy, J.M.; John, S.; Watkins, S.; Trivailo, P.; Coman, F. Piezoelectric energy harvesting from wind using coupled bending-torsional vibrations. Mod. Appl. Sci. 2014, 8, 106–126.
De Marqui Junior, C.; Erturk, A.; Inman, D.J. An electromechanical finite element model for piezoelectric energy harvester plates. J. Sound Vib. 2009, 327, 9–25.
Hobeck, J.D.; Geslain, D.; Inman, D.J. The dual cantilever flutter phenomenon: A novel energy harvesting method. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 9–13 March 2014; Volume 9061.
Naudascher, E.; Rockwell, D. Oscillator-Model Approach to Theidentification and Assessment Offlow-Induced Vibrations in a System. J. Hydraul. Res. 1980, 18, 59–82.
Argentina, M.; Mahadevan, L. Fluid-flow-induced flutter of a flag. Proc. Natl. Acad. Sci. USA 2005, 102, 1829–1834.
McCarthy, J.M.; Watkins, S.; Deivasigamani, A.; John, S.J. Fluttering energy harvesters in the wind: A review. J. Sound Vib. 2016, 361, 355–377.
Yamaguchi, N.; Yokota, K.; Tsujimoto, Y. Flutter Limits and Behaviors of a Flexible Thin Sheet in High-Speed Flow—I: Analytical Method for Prediction of the Sheet Behavior. J. Fluids Eng. 1999, 122, 65–73.
Kashyap, A.S.; Vidya Shankar, K.V.; Vignesh, S. Renewable energy from vortex induced vibrations in a slow moving fluid. In Proceedings of the 2010 International Conference on Environmental Engineering and Applications, Singapore, 10–12 September 2010; pp. 263–266.
Akaydin, H.D.; Elvin, N.; Andreopoulos, Y. The performance of a self-excited fluidic energy harvester. Smart Mater. Struct. 2012, 21, 25007.
Weinstein, L.A.; Cacan, M.R.; So, P.M.; Wright, P.K. Vortex shedding induced energy harvesting from piezoelectric materials in heating, ventilation and air conditioning flows. Smart Mater. Struct. 2012, 21, 45003.
Goushcha, O.; Elvin, N.; Andreopoulos, Y. Interactions of vortices with a flexible beam with applications in fluidic energy harvesting. Appl. Phys. Lett. 2014, 104, 21919.
Xu-Xu, J.; Barrero-Gil, A.; Velazquez, A. Dual mass system for enhancing energy extraction from Vortex-Induced Vibrations of a circular cylinder. Int. J. Mar. Energy 2016, 16, 250–261.
Wang, D.-A.; Ko, H.-H. Piezoelectric energy harvesting from flow-induced vibration. J. Micromech. Microeng. 2010, 20, 25019.
Wang, D.-A.; Chang, K.-H. Electromagnetic energy harvesting from flow induced vibration. Microelectron. J. 2010, 41, 356–364.
Williamson, J.D.B.S.J.; Stark, B.H. Low Head Pico Hydro Turbine Selection using a Multi-Criteria Analysis. In Proceedings of the World Renewable Energy Congress, Linköping, Sweden, 8–13 May 2011; Volume 6, pp. 1337–1385. Available online: https://ep.liu.se/ecp/057/vol6/ecp057vol6.pdf#page=5 (accessed on 11 August 2022).
Tam Nguyen, H.-D.; Pham, H.-T.; Wang, D.-A. A miniature pneumatic energy generator using Kármán vortex street. J. Wind Eng. Ind. Aerodyn. 2013, 116, 40–48.
Wang, J.; Ran, J.; Zhang, Z. Energy Harvester Based on the Synchronization Phenomenon of a Circular Cylinder. Math. Probl. Eng. 2014, 2014, 567357.
Petrini, F.; Gkoumas, K. Piezoelectric energy harvesting from vortex shedding and galloping induced vibrations inside HVAC ducts. Energy Build. 2018, 158, 371–383.
Barrero-Gil, A.; Alonso, G.; Sanz-Andres, A. Energy harvesting from transverse galloping. J. Sound Vib. 2010, 329, 2873–2883.
Sorribes-Palmer, F.; Sanz-Andres, A. Optimization of energy extraction in transverse galloping. J. Fluids Struct. 2013, 43, 124–144.
Sirohi, J.; Mahadik, R. Piezoelectric wind energy harvester for low-power sensors. J. Intell. Mater. Syst. Struct. 2011, 22, 2215–2228.
Sirohi, J.; Mahadik, R. Harvesting Wind Energy Using a Galloping Piezoelectric Beam. J. Vib. Acoust. 2011, 134, 011009.
Abdelkefi, A.; Hajj, M.R.; Nayfeh, A.H. Power harvesting from transverse galloping of square cylinder. Nonlinear Dyn. 2012, 70, 1355–1363.
Abdelkefi, A.; Yan, Z.; Hajj, M.R. Performance analysis of galloping-based piezoaeroelastic energy harvesters with different cross-section geometries. J. Intell. Mater. Syst. Struct. 2013, 25, 246–256.
Yang, Y.; Zhao, L.; Tang, L. Comparative study of tip cross-sections for efficient galloping energy harvesting. Appl. Phys. Lett. 2013, 102, 64105.
Daqaq, M.F. Characterizing the response of galloping energy harvesters using actual wind statistics. J. Sound Vib. 2015, 357, 365–376.
Bibo, A.; Daqaq, M.F. An analytical framework for the design and comparative analysis of galloping energy harvesters under quasi-steady aerodynamics. Smart Mater. Struct. 2015, 24, 94006.
Bibo, A.; Daqaq, M.F. On the optimal performance and universal design curves of galloping energy harvesters. Appl. Phys. Lett. 2014, 104, 23901.
Vicente-Ludlam, D.; Barrero-Gil, A.; Velazquez, A. Enhanced mechanical energy extraction from transverse galloping using a dual mass system. J. Sound Vib. 2015, 339, 290–303.
Pellegrini, S.P.; Tolou, N.; Schenk, M.; Herder, J.L. Bistable vibration energy harvesters: A review. J. Intell. Mater. Syst. Struct. 2013, 24, 1303–1312.
Tang, L.; Yang, Y.; Soh, C.K. Toward broadband vibration-based energy harvesting. J. Intell. Mater. Syst. Struct. 2010, 21, 1867–1897.
Zhao, L.; Tang, L.; Yang, Y. Small Wind Energy Harvesting from Galloping Using Piezoelectric Materials. Smart Mater. Adapt. Struct. Intell. Syst. 2012, 2, 919–927.
Erturk, A. Electromechanical Modeling of Piezoelectric Energy Harvesters. Ph.D. Dissertation, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 2009.
Stanton, S.C.; Erturk, A.; Mann, B.P.; Inman, D.J. Nonlinear piezoelectricity in electroelastic energy harvesters: Modeling and experimental identification. J. Appl. Phys. 2010, 108, 074903.
Renno, J.M.; Daqaq, M.F.; Inman, D.J. On the optimal energy harvesting from a vibration source. J. Sound Vib. 2009, 320, 386–405.
Hamlehdar, M.; Kasaeian, A.; Safaei, M.R. Energy Harvesting from Fluid Flow Using Piezoelectrics: A Critical Review. Renew. Energy 2019, 143, 1826–1838.
Abdelkefi, A.; Najar, F.; Nayfeh, A.H.; Ben Ayed, S. An energy harvester using piezoelectric cantilever beams undergoing coupled bending-torsion vibrations. Smart Mater. Struct. 2011, 20, 115007.
Myers, R.; Vickers, M.; Kim, H.; Priya, S. Small scale windmill. Appl. Phys. Lett. 2007, 90, 54106.
Rezaei-Hosseinabadi, N.; Tabesh, A.; Dehghani, R.; Aghili, A. An Efficient Piezoelectric Windmill Topology for Energy Harvesting from Low-Speed Air Flows. IEEE Trans. Ind. Electron. 2015, 62, 3576–3583.
Stamatellou, A.-M.; Kalfas, A.I. Experimental investigation of energy harvesting from swirling flows using a piezoelectric film transducer. Energy Convers. Manag. 2018, 171, 1405–1415.
Hobeck, J.D.; Inman, D.J. Artificial piezoelectric grass for energy harvesting from turbulence-induced vibration. Smart Mater. Struct. 2012, 21, 105024.
Orrego, S.; Shoele, K.; Ruas, A.; Doran, K.; Caggiano, B.; Mittal, R.; Kang, S.H. Harvesting ambient wind energy with an inverted piezoelectric flag. Appl. Energy 2017, 194, 212–222.
Jun Li, D.; Hong, S.; Gu, S.; Choi, Y.; Nakhmanson, S.; Heinonen, O.; Karpeev, D.; No, K. Polymer piezoelectric energy harvesters for low wind speed. Appl. Phys. Lett. 2014, 104, 12902.
Priya, S. Modeling of electric energy harvesting using piezoelectric windmill. Appl. Phys. Lett. 2005, 87, 184101.
Wang, K.X.; Bu, L.; Chen, J.M.; Song, L. Frequency-tunable airflow energy harvester using variable aperture helmholtz resonator and piezoelectric cantilever. In Proceedings of the 2015 Transducers—2015 18th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Anchorage, AK, USA, 21–25 June 2015; pp. 1945–1948.
He, X.; Shang, Z.; Cheng, Y.; Zhu, Y. A micromachined low-frequency piezoelectric harvester for vibration and wind energy scavenging. J. Micromech. Microeng. 2013, 23, 125009.
Jung, H.J.; Song, Y.; Hong, S.K.; Yang, C.H.; Hwang, S.J.; Jeong, S.Y.; Sung, T.H. Design and optimization of piezoelectric impact-based micro wind energy harvester for wireless sensor network. Sens. Actuators A Phys. 2015, 222, 314–321.
Matova, S.P.; Elfrink, R.; Vullers, R.J.M.; van Schaijk, R. Harvesting energy from airflow with a michromachined piezoelectric harvester inside a Helmholtz resonator. J. Micromech. Microeng. 2011, 21, 104001.
Dai, H.L.; Abdelkefi, A.; Yang, Y.; Wang, L. Orientation of bluff body for designing efficient energy harvesters from vortex-induced vibrations. Appl. Phys. Lett. 2016, 108, 53902.
Wen, Q.; Schulze, R.; Billep, D.; Otto, T.; Gessner, T. Modeling and optimization of a vortex induced vibration fluid kinetic energy harvester. Procedia Eng. 2014, 87, 779–782.
Xie, J.; Yang, J.; Hu, H.; Hu, Y.; Chen, X. A piezoelectric energy harvester based on flow-induced flexural vibration of a circular cylinder. J. Intell. Mater. Syst. Struct. 2012, 23, 135–139.
Hobbs, W.B.; Hu, D.L. Tree-inspired piezoelectric energy harvesting. J. Fluids Struct. 2012, 28, 103–114.
Dai, H.L.; Abdelkefi, A.; Wang, L. Theoretical modeling and nonlinear analysis of piezoelectric energy harvesting from vortex-induced vibrations. J. Intell. Mater. Syst. Struct. 2014, 25, 1861–1874.
Dunnmon, J.A.; Stanton, S.C.; Mann, B.P.; Dowell, E.H. Power extraction from aeroelastic limit cycle oscillations. J. Fluids Struct. 2011, 27, 1182–1198.
Li, S.; Yuan, J.; Lipson, H. Ambient wind energy harvesting using cross-flow fluttering. J. Appl. Phys. 2011, 109, 2–5.
Hobeck, J.D.; Inman, D.J. A distributed parameter electromechanical and statistical model for energy harvesting from turbulence-induced vibration. Smart Mater. Struct. 2014, 23, 115003.
Alben, S.; Shelley, M.J. Flapping States of a Flag in an Inviscid Fluid: Bistability and the Transition to Chaos. Phys. Rev. Lett. 2008, 100, 74301.
Akaydın, H.D.; Elvin, N.; Andreopoulos, Y. Wake of a cylinder: A paradigm for energy harvesting with piezoelectric materials. Exp. Fluids 2010, 49, 291–304.
Goushcha, O.; Akaydin, H.D.; Elvin, N.; Andreopoulos, Y. Energy harvesting prospects in turbulent boundary layers by using piezoelectric transduction. J. Fluids Struct. 2015, 54, 823–847.
Michelin, S.; Doaré, O. Energy harvesting efficiency of piezoelectric flags in axial flows. J. Fluid Mech. 2013, 714, 489–504.
Kwon, S.-D. A T-shaped piezoelectric cantilever for fluid energy harvesting. Appl. Phys. Lett. 2010, 97, 164102.
Bibo, A.; Daqaq, M.F. Energy harvesting under combined aerodynamic and base excitations. J. Sound Vib. 2013, 332, 5086–5102.
Bibo, A.; Daqaq, M.F. Investigation of concurrent energy harvesting from ambient vibrations and wind using a single piezoelectric generator. Appl. Phys. Lett. 2013, 102, 243904.
Dowell, E.; Edwards, J.; Strganac, T. Nonlinear Aeroelasticity. J. Aircr. 2003, 40, 857–874.
Abdelkefi, A.; Hajj, M.R.; Nayfeh, A.H. Phenomena and modeling of piezoelectric energy harvesting from freely oscillating cylinders. Nonlinear Dyn. 2012, 70, 1377–1388.
Yin, J.; Li, X.; Yu, J.; Zhang, Z.; Zhou, J.; Guo, W. Generating electricity by moving a droplet of ionic liquid along graphene. Nat. Nanotechnol. 2014, 9, 378–383.

© Text is available under the terms and conditions of the Creative Commons Attribution (CC BY) license; additional terms may apply. By using this site, you agree to the Terms and Conditions and Privacy Policy.

Upload a video for this entry

Information

Subjects: Energy & Fuels

Contributors MDPI registered users' name will be linked to their SciProfiles pages. To register with us, please refer to https://encyclopedia.pub/register :

Areeba Naqvi

Ahsan Ali

Wael A. Altabey

Sallam A. Kouritem

View Times: 1.4K

Update Date: 16 Dec 2022

Table of Contents

Video Upload Options

Confirm