Submitted Successfully!
To reward your contribution, here is a gift for you: A free trial for our video production service.
Thank you for your contribution! You can also upload a video entry or images related to this topic.
Version Summary Created by Modification Content Size Created at Operation
1
Ningyuan Zhang
 -- 1320 2022-06-14 07:52:13 |
2 layout
Camila Xu
 Meta information modification 1320 2022-06-14 08:01:19 | |
3 layout
Camila Xu
 + 6 word(s) 1326 2022-06-15 11:13:33 |

Video Upload Options

We provide professional Video Production Services to translate complex research into visually appealing presentations. Would you like to try it?
No, upload directly Yes

Confirm

Are you sure to Delete?
Yes No
Cite
If you have any further questions, please contact Encyclopedia Editorial Office.
Zhang, N.; Luo, B.; , . Cable-Supported Grid Structures. Encyclopedia. Available online: https://encyclopedia.pub/entry/23997 (accessed on 21 December 2024).
Zhang N, Luo B,  . Cable-Supported Grid Structures. Encyclopedia. Available at: https://encyclopedia.pub/entry/23997. Accessed December 21, 2024.
Zhang, Ningyuan, Bin Luo,  . "Cable-Supported Grid Structures" Encyclopedia, https://encyclopedia.pub/entry/23997 (accessed December 21, 2024).
Zhang, N., Luo, B., & , . (2022, June 14). Cable-Supported Grid Structures. In Encyclopedia. https://encyclopedia.pub/entry/23997
Zhang, Ningyuan, et al. "Cable-Supported Grid Structures." Encyclopedia. Web. 14 June, 2022.
Cable-Supported Grid Structures
Edit
This entry is adapted from the peer-reviewed paper 10.3390/buildings12060749

The cable-supported grid structure (CSGS) is a hybrid structure combined with rigidity and flexibility. Its formed state is closely related to the zero-stress state, construction process, and prestress distribution. 

cable-supported grid structure force-finding zero-stress state form-finding

1. Cable-Supported Grid Structures

Cable-supported grid structure (CSGS) is an emerging structure that combines rigidity and flexibility, similar to the suspendome [1][2]. The difference between them is that CSGS generally has an opening in the center of the structure. It is composed of the upper rigid grid, providing rigid support and reducing the flexibility [3], and the lower cable-strut system, which effectively increases the structural span and takes full advantage of material strength [4]. CSGS can obtain the geometric stiffness and the bearing capacity by applying the prestress in the cables, which is identical to other flexible structures. There is a compression ring in the upper rigid grid to supply constraints. The compression ring in CSGS can be assigned to three categories in accordance with its position: external compression ring, internal compression ring, and upper grid as a whole as a compression ring, as shown in Figure 1. Due to its tremendous advantages of light weight, good aesthetic, large-span high bearing efficiency, and good economy, CSGS has gradually aroused widespread attention in the engineering field, especially in the applications of the stadium [5][6]. In comparison, few projects adapt CSGS with an internal compression ring.
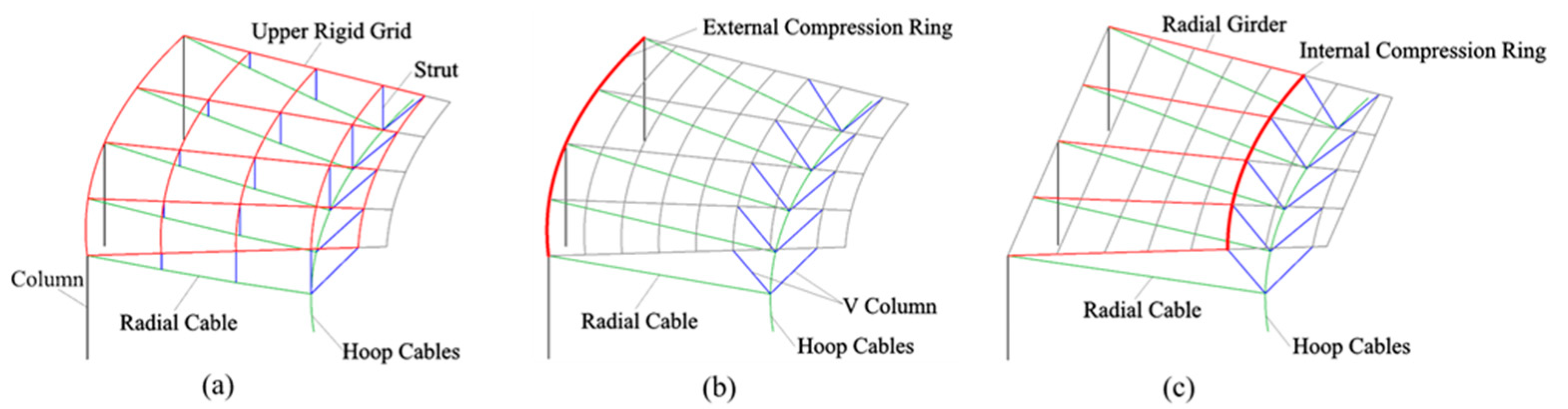Figure 1. Three categories of CSGS: (a) upper grid as a whole compression ring; (b) external compression ring; (c) internal compression ring.
In contrast to rigid structures, the configuration of CSGS changes significantly during the construction process. Structural configuration is unstable and dynamic in the construction stage until the design configuration is reached [7]. For CSGS, it is important to find equilibrium configuration and feasible prestress distribution [8][9]. Therefore, it is an essential issue to find a stable self-equilibrium configuration in the design of various structures, including cable-net structures [10][11][12], cable-membrane structures [13][14][15][16], tensegrities [17][18][19][20][21], compressive structures [22], suspension bridges [23][24], and even submerged floating tunnels [25]. As a consequence, form-finding is a research hotspot and has been intensively studied by many scholars. The form-finding methods can be classified into two types: physical hanging models and numerical form-finding methods. The physical hanging model is used to establish a physical model to determine the structural equilibrium state under external loads [26]. Among numerous numerical form-finding methods, the force density method, dynamic relaxation method, and nonlinear finite element method are preponderant. The force density method, first put forward by Linkwitz and Scheck [27][28], defined the ratio of the internal force of components to its corresponding length as the force density, linearizing the nonlinear equations, which is concise and effective. Later researchers also devoted considerable efforts to the force density method [10][29][30]. The dynamic relaxation method transforms a nonlinear static problem into a pseudodynamic one [22] with fictitious mass and damping to calculate the structural equilibrium state, which is proposed by Otter [31] and Day [32]. Barnes [33] introduced the dynamic relaxation method into the form-finding analysis of cable-net and membrane structures. The nonlinear finite element method [34] applies the finite element method [35][36] to nonlinear form-finding analysis and solves nonlinear equations by iteration [37]. In addition to the three numerical methods mentioned above, the evolutionary and intelligent form-finding method has been developed to seek specific equilibrium configurations, genetic algorithms [38][39], group theory [40], particle swarm optimization algorithms [41][42], and the Monte Carlo method [43].
The configuration of CSGS can be defined as the following three states: the zero-stress state is the state before applying prestress where the structure is lofted; the prestress state, also known as the initial state, is the self-equilibrium state under its self-weight and prestress [44]; the load state refers to the state in which external loads are applied to the structure in the prestress state. The above form-finding methods generally only pay attention to the equilibrium configuration in the prestress state. Nevertheless, it is indispensable to determine the zero-stress state in practical engineering and the basis and starting point for subsequent construction steps, which will profoundly impact on the prestress state and the load state. If the prestress state is improperly taken as the starting point of the forming process, the formed structure will deviate from the design requirements, i.e., the configuration “drift” phenomenon [13]. The “drift” has less influence on small-scale structures with a low prestress level. However, it cannot be neglected and is inevitable to solve this problem for large-scale structures with a high prestress level and high precision requirements [45]. Structural components should be processed and assembled in accordance with the configuration of the zero-stress state.
In actual projects, the desired structural configuration is determined by architects or clients in general [21]. Prestress distribution in the desired structural configuration still needs further discussion in theory. Consequently, form-finding problems turn into force-finding problems when the shapes are given in advance [40], because “force” interacts with “form”. Pellegrino and Calladine [46][47][48] proposed the singular value decomposition (SVD) technique to obtain the self-stress modes of cable-strut structures. Yuan and Dong [49] introduce the state of integral feasible prestress to the optimal prestress design. Then, Yuan [50] proposed a general method, referred to as the double singular value decomposition (DSVD), to determine the prestress modes of cable domes. Chen and Dong [9] obtain multioverall self-stress modes from the equilibrium matrix with SVD. Many scholars have also carried out further research on force-finding [20][39][40][51].
The forming process of CSGS is also called the construction process. The construction process is the bridge between the zero-stress state and the prestress state [21]. Valid and rational construction techniques can guarantee the accuracy of prestress distribution and configuration of the formed structure [52]. The structural state of CSGS during the construction process, where the structural configuration and internal force are constantly changing, could be strikingly different from that in the service stage [3]. Hence, it is considerable to track the structural state during the construction process so as to ensure structural safety, which sets higher requirements for form-finding and force-finding in the construction stage. Construction analysis can be divided as follows: direct analysis is to analyze structural displacement and internal force during the construction process in conformity to the actual construction sequence [53]; inverse analysis takes the prestress state as the construction starting point and removes the components step by step to obtain the structural behavior in the construction stage [54]. Moreover, Luo [55] proposed the nonlinear dynamic finite element method to solve form-finding problems of the cable-strut system during the construction process. The ideal control parameters of each construction step can be determined by analysis to adjust the structural state so that the formed state is consistent with the design state.

2. Engineering Background of Cable-Supported Grid Structures

Pudong Football Stadium is the world’s first engineering application of a large-span CSGS with an internal compression ring. It is located in Shanghai, China, with a total construction area of 13,500 m2 and a 33,000 seat capacity. The structural plane is a rounded rectangle, and there are straight lines on the boundary. The long axis of the stadium is 211 m and the short axis is 173 m, as shown in Figure 2. The roof structure is about 11.8 m in height. There are 46 radial cables in total, of which the diameter of the radial cables at the corner is 110 mm and 120 mm, and those on the long side and short side are 95 mm and 110 m, respectively. The hoop cables are arranged in parallel with eight cables with a diameter of 100 mm. As a combination of rigid and flexible structures, CSGS is shaped by tensioning the lower radial cables so that it has the ability to bear loads itself. The primary constituent unit of the structure is composed of radial cable, radial girder, internal compression ring, V column outer branch, and hoop cables, forming the prestress self-balancing triangle area, as shown in Figure 2e. The specifications of the main steel components are listed in Table 1.
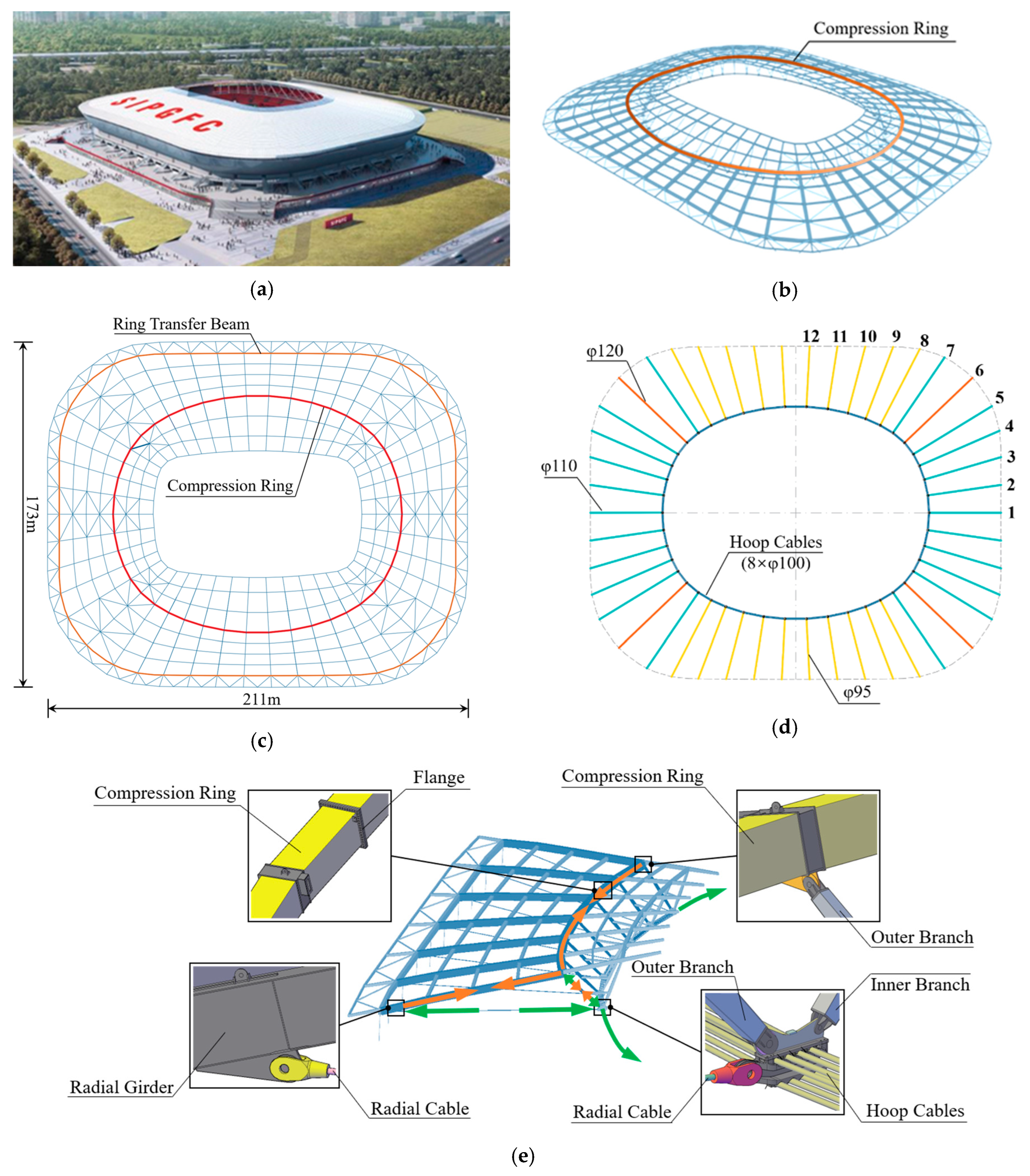Figure 2. Schematic of Pudong Football Stadium: (a) effect picture; (b) three-dimensional view; (c) plan view; (d) radial cable distribution; (e) node detail diagram.
Table 1. Component specifications.
Section Type Component Sectional Dimension (mm)
Rectangular tube Internal compression ring 1500 × 1500 × 50 × 50
Radial girder 650 × 650 × 14 × 22
Ring beam 1400 × 700 × 10 × 48
V column 600 × 350 × 12 × 12
Circular tube Column 400 × 10

References

  1. Kawaguchi, M.; Abe, M.; Tagemichi, I. Design, tests and realization of “suspen-dome” system. J. Int. Assoc. Shell Spat. Struct. 1999, 40, 179–192.
  2. Kang, W.; Chen, Z.; Lam, H.F.; Zuo, C. Analysis and design of the general and outmost-ring stiffened suspendome structures. Eng. Struct. 2003, 25, 1685–1695.
  3. Liu, H.; Zhang, W.; Yuan, H.; Zhu, J.; Zheng, J. Modified double-control form-finding analysis for suspendomes considering the construction process and the friction of cable–strut joints. Eng. Struct. 2016, 120, 75–81.
  4. Krishnan, S. Cable-stayed columns and their applications in building structures. J. Build. Eng. 2020, 27, 100984.
  5. Feng, Y.; Xiang, X.; Wang, H.; Chen, W. Mechanical behavior and form-finding research on large opening spoke-wheel-type cable supported grid structure. J. Build. Struct. 2019, 40, 69–80.
  6. Feng, Y.; Xiang, X.; Wang, H. Static performance parameter analysis of large opening spoke-wheel-type cable supported grid structure. J. Build. Struct. 2019, 40, 81–91.
  7. Zhang, Z.; Cao, Q.; Dong, S.; Fu, X. Structural design of a practical suspendome. Adv. Steel Constr. 2008, 4, 323–340.
  8. Wang, Y.; Xu, X.; Luo, Y. A unifying framework for form-finding and topology-finding of tensegrity structures. Comput. Struct. 2021, 247, 106486.
  9. Chen, L.; Dong, S. Optimal Prestress Design and Construction Technique of Cable-Strut Tension Structures with Multi-Overall Selfstress Modes. Adv. Struct. Eng. 2013, 16, 1633–1644.
  10. Li, X.; Xue, S.; Liu, Y. A novel form finding method for minimum surface of cable net. J. Build. Eng. 2022, 48, 103939.
  11. Nie, R.; He, B.; Hodges, D.H.; Ma, X. Form finding and design optimization of cable network structures with flexible frames. Comput. Struct. 2019, 220, 81–91.
  12. Deng, H.; Jiang, Q.; Kwan, A.S.K. Shape finding of incomplete cable-strut assemblies containing slack and prestressed elements. Comput. Struct. 2005, 83, 1767–1779.
  13. Zhao, J.; Chen, W.; Fu, G.; Li, R. Computation method of zero-stress state of pneumatic stressed membrane structure. Sci. China Technol. Sci. 2012, 55, 717–724.
  14. Tang, Y.; Li, T.; Ma, X.; Hao, L. Extended Nonlinear Force Density Method for Form-Finding of Cable-Membrane Structures. J. Aerosp. Eng. 2017, 30, 4016101.
  15. Tang, Y.; Li, T. Equivalent-force density method as a shape-finding tool for cable-membrane structures. Eng. Struct. 2017, 151, 11–19.
  16. Shi, J.; Zhao, J.; Tsukimoto, S.; Shimoda, M. Design optimization of cable–membrane structures for form-finding and stiffness maximization. Compos. Struct. 2020, 206, 9–22.
  17. Su, Y.; Zhang, J.; Ohsaki, M.; Wu, Y. Topology optimization and shape design method for large-span tensegrity structures with reciprocal struts. Int. J. Solids Struct. 2018, 192, 528–536.
  18. Zhang, L.; Zhu, S.; Li, S.; Xu, G. Analytical form-finding of tensegrities using determinant of force-density matrix. Compos. Struct. 2018, 189, 87–98.
  19. Pagitz, M.; Mirats Tur, J.M. Finite element based form-finding algorithm for tensegrity structures. Int. J. Solids Struct. 2009, 46, 3235–3240.
  20. Yuan, S.; Zhu, W. Optimal self-stress determination of tensegrity structures. Eng. Struct. 2021, 238, 112003.
  21. Dong, W.; Stafford, P.J.; Ruiz-Teran, A.M. Inverse form-finding for tensegrity structures. Comput. Struct. 2019, 215, 27–42.
  22. Bagrianski, S.; Halpern, A.B. Form-finding of compressive structures using Prescriptive Dynamic Relaxation. Comput. Struct. 2014, 132, 65–74.
  23. Domaneschi, M.; Limongelli, M.G.; Martinelli, L. Multi-site damage localization in a suspension bridge via aftershock monitoring. Ing. Sismica 2013, 30, 56–72.
  24. Wang, X.; Wang, H.; Zhang, J.; Sun, Y.; Bai, Y.; Zhang, Y.; Wang, H. Form-finding method for the target configuration under dead load of a new type of spatial self-anchored hybrid cable-stayed suspension bridges. Eng. Struct. 2021, 227, 11407.
  25. Martinelli, L.; Domaneschi, M.; Shi, C. Submerged Floating Tunnels under Seismic Motion: Vibration Mitigation and Seaquake effects. Procedia Eng. 2016, 166, 229–246.
  26. Zhang, P.; Zhou, J.; Chen, J. Form-finding of complex tensegrity structures using constrained optimization method. Compos. Struct. 2021, 268, 113971.
  27. Schek, H.J. The force density method for form finding and computation of general networks. Comput. Methods Appl. Mech. Eng. 1974, 3, 115–134.
  28. Linkwitz, K.; Schek, H.J. Einige Bemerkungen zur Berechnung von vorgespannten Seilnetzkonstruktionen. Ing. Arch. 1971, 40, 145–158.
  29. Cai, J.; Wang, X.; Deng, X.; Feng, J. Form-finding method for multi-mode tensegrity structures using extended force density method by grouping elements. Compos. Struct. 2018, 187, 1–9.
  30. Koohestani, K. Innovative numerical form-finding of tensegrity structures. Int. J. Solids Struct. 2020, 206, 304–313.
  31. Otter, J.R.H.; Cassell, A.C.; Hobbs, R.E. Poisson, Dynamic relaxation. Proc. Inst. Civ. Eng. 1966, 35, 633–656.
  32. Day, A.S.; Bunce, J.H. Analysis of cable networks by dynamic relaxation. Civ. Eng. Public Work. Rev. 1970, 4, 383–386.
  33. Barnes, M.R. Form Finding and Analysis of Tension Structures by Dynamic Relaxation. Int. J. Space Struct. 1999, 14, 89–104.
  34. Argyris, J.H.; Angelopoulos, T.; Bichat, B. A general method for the shape finding of lightweight tension structures. Comput. Methods Appl. Mech. Eng. 1974, 3, 135–149.
  35. Domaneschi, M. Experimental and numerical study of standard impact tests on polypropylene pipes with brittle behavior. Proc. Inst. Mech. Eng. Part B 2012, 226, 2035–2046.
  36. Domaneschi, M.; Perego, U.; Borgqvist, E.; Borsari, R. An industry-oriented strategy for the finite element simulation of paperboard creasing and folding. Packag. Technol. Sci. 2017, 30, 269–294.
  37. Tanarrok, B.; Qin, Z. Nonlinear analysis of tension structures. Comput. Struct. 1992, 45, 973–984.
  38. Lee, S.; Lee, J. Form-finding of tensegrity structures with arbitrary strut and cable members. Int. J. Mech. Sci. 2014, 85, 55–62.
  39. Quagliaroli, M.; Albertin, A.; Pollini, N. The role of prestress and its optimization in cable domes design. Comput. Struct. 2015, 161, 17–30.
  40. Zhang, Q.; Wang, X.; Cai, J.; Yang, R.; Feng, J. Prestress design for cable-strut structures by grouping elements. Eng. Struct. 2021, 244, 112010.
  41. Sun, F.; Zhu, D.; Liang, M.; Zhang, D. Study on Form-Finding of Cable-Membrane Structures Based on Particle Swarm Optimization Algorithm. Math. Probl. Eng. 2020, 2020, 1281982.
  42. Chen, Y.; Yan, J.; Feng, J.; Sareh, P. A hybrid symmetry–PSO approach to finding the self-equilibrium configurations of prestressable pin-jointed assemblies. Acta Mech. 2020, 231, 1485–1501.
  43. Li, Y.; Feng, X.; Cao, Y.; Gao, H. A Monte Carlo form-finding method for large scale regular and irregular tensegrity structures. Int. J. Solids Struct. 2010, 47, 1888–1898.
  44. Ye, J.; Feng, R.; Zhao, X.; Liu, B. A form-finding method of beam string structures-Offload by steps method. Int. J. Steel Struct. 2012, 12, 267–283.
  45. Fan, L.; Sun, Y.; Fan, W.; Chen, Y.; Feng, J. Determination of active members and zero-stress states for symmetric prestressed cable–strut structures. Acta Mech. 2020, 231, 3607–3620.
  46. Pellegrino, S. Analysis of prestressed mechanisms. Int. J. Solids Struct. 1990, 26, 1329–2350.
  47. Pellegrino, S. Structural computations with the singular value decomposition of the equilibrium matrix. Int. J. Solids Struct. 1993, 30, 3025–3035.
  48. Pellegrino, S.; Calladine, C.R. Matrix analysis of statically and kinematically indeterminate frameworks. J. Solids Struct. 1986, 22, 409–428.
  49. Yuan, X.; Dong, S. Integral feasible prestress of cable domes. Comput. Struct. 2003, 81, 2111–2119.
  50. Yuan, X.; Chen, L.; Dong, S. Prestress design of cable domes with new forms. Int. J. Solids Struct. 2007, 44, 2773–2782.
  51. Guo, J.; Jiang, J. An algorithm for calculating the feasible pre-stress of cable-struts structure. Eng. Struct. 2016, 118, 228–239.
  52. Chen, L.; Hu, D.; Deng, H.; Cui, Y.; Zhou, Y. Optimization of the construction scheme of the cable-strut tensile structure based on error sensitivity analysis. Steel Compos. Struct. 2016, 21, 1031–1043.
  53. Li, Y.; Zhang, G.; Yang, Q. Determination of cable forces during construction for cable-supported lattice shells. J. Build. Struct. 2004, 24, 76–81.
  54. Yuan, X.; Dong, S. Inverse Analysis of Construction Process of Cable Dome. J. Build. Struct. 2001, 22, 75–80.
  55. Luo, B.; Guo, Z.; Chen, X.; Gao, F.; Wang, K. Static equilibrium form-finding analysis of cable-strut system based on nonlinear dynamic finite element method. Adv. Steel Constr. 2005, 11, 452–468.
More
© Text is available under the terms and conditions of the Creative Commons Attribution (CC BY) license; additional terms may apply. By using this site, you agree to the Terms and Conditions and Privacy Policy.
Upload a video for this entry
Information
Subjects: Engineering, Civil
Contributors MDPI registered users' name will be linked to their SciProfiles pages. To register with us, please refer to https://encyclopedia.pub/register :
Ningyuan Zhang
,
Bin Luo
,
View Times: 1.1K
Revisions: 3 times (View History)
Update Date: 15 Jun 2022
Table of Contents
    1000/1000
    Hot Most Recent
    Video Production Service
    Feedback