Oscillation is one of the important branches in applied mathematics and can be induced or destroyed by the introduction of nonlinearity, delay, or a stochastic term. The oscillation of differential and difference equations contributes to many realistic applications, such as torsional oscillations, the oscillation of heart beats, sinusoidal oscillation, voltage-controlled neuron models, and harmonic oscillation with damping.
1. Introduction
Over many years, the process of describing natural or real-life phenomena has been carried out using the integer-order differential equations. However, the factors involved in the phenomena are very complicated and of different natures, all of which cannot be incorporated by the ordinary differential equations. This gap in the construction of the models is covered up by arbitrary-order calculus. Fractional calculus has its origin during the same period of time as that of the classical calculus in the 17th Century. The insufficient geometrical and unsatisfactory physical interpretation of the arbitrary-order derivatives has slowed down the progress of the field. It was in the 20th Century with the development of high-speed computers and computational techniques that researchers began to understand the importance and the meaningful representation to construct and apply a certain type of nonlocal operator to real-life problems. Now, fractional calculus has turned out to be a hot topic in the fields of science and engineering. The rapid growth and inspiration of fractional calculus have been greatly due to anticipation of the memory and hereditary features that are incorporated in many phenomena by the so-called fractional differential operators
[1][2][3]. As a result of this, the subject of fractional calculus and its widespread applications have become of great interest for the relevant audience
[4]. For the same justifications that led to the investigation of the discrete analogue of integer-order differential operators, the discrete analogue of fractional differential operators, which is called fractional difference operators, has gained considerable attention, and thus, they have been significantly adopted due to their extensive applications in computations and simulations. The study of fractional difference equations was led by the pioneering works of Agarwal, Atici, Eloe, Anastassiou, Holm, Goodrich, and Peterson, who introduced a complete counterpart theory that adopts all the essential preliminaries needed to set forth similar results relevant to the qualitative theory of solutions for several types of fractional difference equations
[5][6][7][8][9][10][11].
Every phenomenon in the world in one way or other is nonlinear in nature. Thus, the better understanding of these phenomena can be obtained from models constructed via nonlinear equations. The analytical solution of nonlinear equations is not always possible to obtain as in the case of linear equations. However, approximate solutions can be obtained for the nonlinear equations, which provide a better understanding of the behavior of the equations. In the case of nonlinear equations, without actually solving the equations, one can very well answer questions such as the existence of solutions, whether the system is stable, whether it can be controlled, whether the system is chaotic, or whether it exhibits periodicity. Thus, this direct method of analyzing the system behavior can be useful and help engineers in their research. Scientists and researchers are very much interested in the qualitative properties such as the oscillation, stability, controllability, bifurcation, chaos, and so on.
2. Preliminaries
The empty sums and products were taken to be zero and one, respectively. Denote by he set of all natural numbers, the set of all real numbers, and the set of all positive real numbers. Define by and for any a, such that .
Definition 1 ([12][13]). The Euler gamma function is defined by:
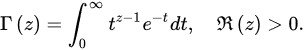
Using its reduction formula, the Euler gamma function can also be extended to the half-plane except for
Definition 2 ([14]). The generalized falling function is defined by:
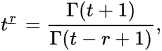
for those values of t and r such that the right-hand side of this equation makes sense. If is a nonpositive integer and is not a nonpositive integer, then we use the convention that . The generalized rising function is defined by:
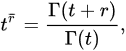
for those values of t and r so that the right-hand side of this equation is sensible. If t is a nonpositive integer, but is not a nonpositive integer, then we use the convention that
Definition 3 ([15]). Let and . The first-order forward (delta) and backward (nabla) differences of u are defined by:
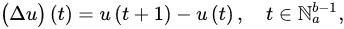
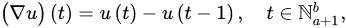
respectively. The -order delta and nabla differences of u are defined recursively by
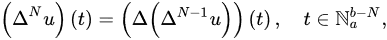
and:
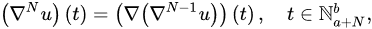
respectively.
Definition 4 ([14]). Let and . Then, the -order delta fractional sum of u based at a is defined by:
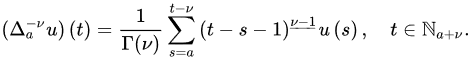
Definition 5 ([14]). Let and . Then, the -order nabla fractional sum of u based at a is defined by:
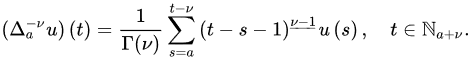
Definition 6 ([14]). Let , and choose such that . The -order Riemann–Liouville delta fractional difference of u is defined by:
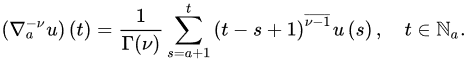
Definition 7 ([14]). Let , and choose such that . Then, the -order Riemann–Liouville nabla fractional difference of u is defined by:
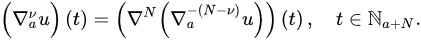
Definition 8 ([16]). Let , and . Then, the -order Caputo delta fractional difference of u is defined by:
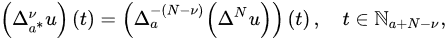
where . If , then:
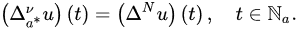
Definition 9 ([17]). Let and . Then, the -order Caputo nabla fractional difference of u is defined by:
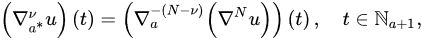
where .
3. Oscillation
3.1. Oscillatory Behavior of Delta Fractional Difference Equations
Consider the following higher-order nonlinear delta fractional difference equations involving the Riemann–Liouville and the Caputo operators of arbitrary order:
and:
Here, and choose such that ; and are continuous. A solution u of (1) (or (2)) is said to be oscillatory if for every natural number M, there exists such that ; otherwise, it is called non-oscillatory. An equation is said to be oscillatory if all of its solutions are oscillatory.
Let be continuous and , be positive real numbers. We make the following assumptions:
(A1) The functions satisfy the sign condition , , , ;
(A2) ;
(A3) .
In [18], Senem et al. established some oscillation theorems given in the sequel.
Theorem 1 ([18]). Let (A1)–(A2) be satisfied with . If:
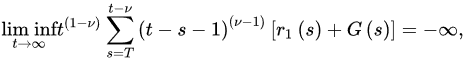
and:
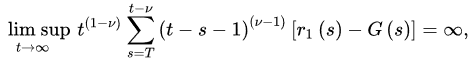
for every sufficiently large T, where:
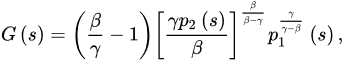
then Equation (1) is oscillatory.
Theorem 2 ([18]). Let and (A1)–(A3) be satisfied with . If
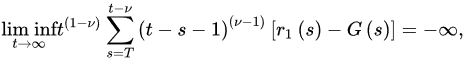
and:
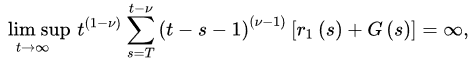
for every sufficiently large T, where G is defined as in Theorem 1, then every bounded solution of Equation (1) is oscillatory.
Theorem 3 ([18]). Let (A1) and (A2) be satisfied with . If:
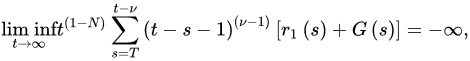
and:
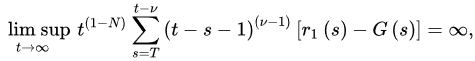
for every sufficiently large T, where G is defined as in Theorem 1, then Equation (2) is oscillatory.
Theorem 4 (
[18])
. Let and (A1)–(A3) be satisfied with . If:
and:
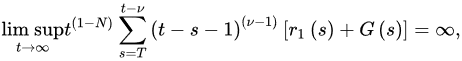
for every sufficiently large T, where G is defined as in Theorem 1, then every bounded solution of Equation (2) is oscillatory.
Following the work in
[18], Li et al.
[19] investigated the oscillation of forced delta fractional difference equations with the damping term of the form:
where ; , and such that:
and for .
Theorem 5 (
[19])
. For , suppose that:
and:
where M is a constant and:
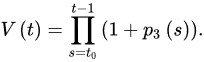
Then, Equation (3) is oscillatory.
Theorem 6 (
[19])
. For , suppose that:
and:
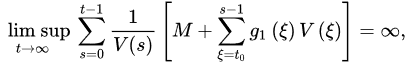
where M is a constant and V is defined as in Theorem 5. Then, Equation (3) is oscillatory.
In this line, Seçer et al.
[20] investigated the oscillation of the following nonlinear delta fractional difference equations:
for , Here, , and are the quotients of two odd positive numbers such that , , and are positive sequences,
is continuous, and:
Theorem 7 (
[20])
. If there exists a positive sequence ϕ such that:
then Equation (4) is oscillatory. Here:
and,
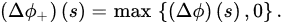
Theorem 8 (
[20])
. Let ϕ be a positive sequence. Furthermore, we assume that there exists a double sequence such that:
If:
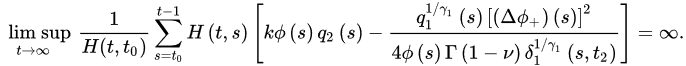
Then, Equation (4) is oscillatory.
If we choose the double sequence:
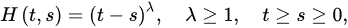
we have the following corollary.
Corollary 1 (
[20])
. Under the conditions of Theorem 8 and:
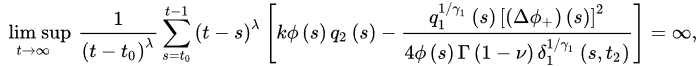
then Equation (4) is oscillatory.
In
[21], Chatzarakis et al. studied the oscillatory behavior of the delta fractional difference equation of the form:
where ; is a quotient of odd positive integers; is a positive sequence, and is a continuous function such that:
We also assume:
for some positive constants , and for all and , and:
for some positive constants and .
Theorem 9 (
[21])
. Assume:
Furthermore, assume that there exists a positive sequence r˜ such that:
where:
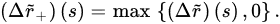
Then, Equation (5) is oscillatory.
Theorem 10 (
[21])
. Assume:
Furthermore, assume that there exists a positive sequence r˜ and a double positive sequence such that:
If:
where:
then Equation (5) is oscillatory.
Theorem 11 (
[21])
. Assume that there exists a positive sequence such that:
Then, Equation (5) is oscillatory.
Motivated by the above works, Adiguzel
[22][23] considered the oscillation behavior of the solutions of the following delta fractional difference equations:
and:
3.2. Oscillatory Behavior of Nabla Fractional Difference Equations
Let , and choose such that . Take p, , , ; , ; ; , ; w, ; q, ; x, ; ; y is a positive function defined on , , are positive real numbers; are the ratios of odd positive integers with .
We make the following assumptions:
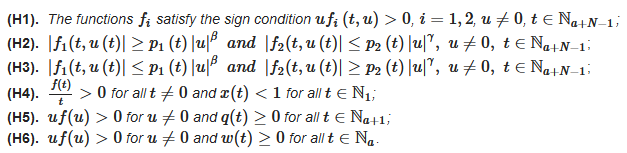
Alzabut et al. [24] initiated the study of the oscillation of solutions of nabla fractional difference equations. In [24], the authors established several oscillation criteria for the following nonlinear nabla fractional difference equations involving the Riemann–Liouville and Caputo operators of arbitrary order.
and:
A solution u of (30) (or (31)) is said to be oscillatory if for every natural number M, there exists such that ;otherwise, it is called non-oscillatory. An equation is said to be oscillatory if all of its solutions are oscillatory.
Theorem 37 ([24]). Let and Condition (H1) hold. If:
then Equation (30) is oscillatory.
Theorem 38 (
[24])
. Let Conditions (H1) and (H2) hold with and . If:
then Equation (30) is oscillatory.
Theorem 39 (
[24])
. Let Conditions (H1) and (H2) hold with and . If:
then Equation (30) is oscillatory.
Theorem 40 (
[24])
. Let Conditions (H1) and (H2) hold with
Following the work in
[24], Abdalla et al.
[25] established new oscillation criteria for (
30) and (
31) using the fractional Volterra sum equations and Young’s inequalities. The authors in
[25] observed that the cases
and
were not considered for (
30) in
[24]. The purpose of the paper
[25] was to cover this gap and establish new oscillation criteria that improve the results in
[24].
Theorem 46 ([25]).
Theorem 47 ([25]).
Theorem 48 ([25]).
for sufficiently large T, where H is defined in Theorem 45, then every bounded solution of Equation (31) is oscillatory.
In alignment with the above works, Abdalla et al. [26] investigated the oscillation of solutions for nabla fractional difference equations with mixed nonlinearities of the forms:
Theorem 49 ([26]). Let:
then Equation (32) is oscillatory.
Corollary 3 ([26]). Let in (32), then . Suppose , , .If (35) and (36) hold for some constant , then Equation (32) is oscillatory.
Corollary 4 ([26]). Let in (32), then . Suppose , , .If (35) and (36) hold for some constant , then Equation (32) is oscillatory.
Corollary 5 ([26]). Let:
Theorem 50 ([26]). Assume that Condition (34) holds. If:
Corollary 6 ([26]).
Corollary 7 ([26]).
Corollary 8 ([26]).
Following the above trend, in [27], Alzabut et al. considered the following forced and damped nabla fractional difference equation:
Motivated by the paper [24], the authors [28] investigated the oscillation of a nonlinear fractional nabla difference system of the form:
Theorem 55 ([29]). Let Condition (H6) hold. If the inequality: