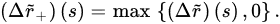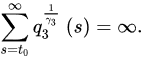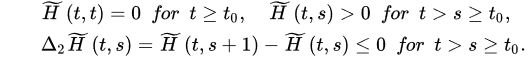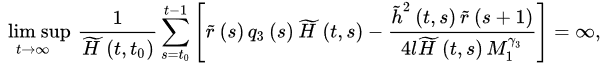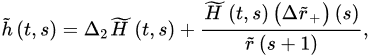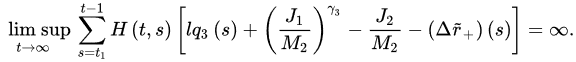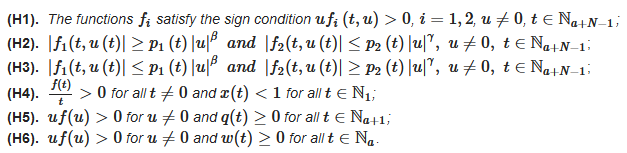Every phenomenon in the world in one way or other is nonlinear in nature. Thus, the better understanding of these phenomena can be obtained from models constructed via nonlinear equations. The analytical solution of nonlinear equations is not always possible to obtain as in the case of linear equations. However, approximate solutions can be obtained for the nonlinear equations, which provide a better understanding of the behavior of the equations. In the case of nonlinear equations, without actually solving the equations, one can very well answer questions such as the existence of solutions, whether the system is stable, whether it can be controlled, whether the system is chaotic, or whether it exhibits periodicity. Thus, this direct method of analyzing the system behavior can be useful and help engineers in their research. Scientists and researchers are very much interested in the qualitative properties such as the oscillation, stability, controllability, bifurcation, chaos, and so on.
2. Preliminaries
The empty sums and products were taken to be zero and one, respectively. Denote by he set of all natural numbers, the set of all real numbers, and the set of all positive real numbers. Define by and for any a, such that .
Definition 1 ([12][13][19,20]). The Euler gamma function is defined by:
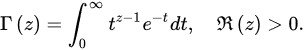
Using its reduction formula, the Euler gamma function can also be extended to the half-plane
Γ
ℜ
(
z
)
≤
0=
except for
∫
z
∈0
{ ∞
…
,
− t
2
,
−z
1
−
,
0
1
}
Definition 2 ([14]). The generalized falling function is defined by:
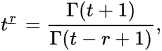
for those values of t and r such that the right-hand side of this equation makes sense. If
t
− e
r
+
1−
is a nonpositive integer and
t
t
+
1d
t
is not a nonpositive integer, then we use the convention that
,
ℜ
t
(
r
z
̲)
>
=0
0.
.
Using Theits generalized rising funreduction is defined by:
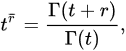
fformula, those values of t and r so that the right-hand side of this equation is sensible. If t is a nonpositive integer, but
t
+
r
is not a nonpositive Euler gamma function can also be integer, then we use the conventionxtended to the half-plane that t(
z
r)
¯≤
=
0
Definition 3 ([15]). Lexcept for N…
a,
b−
2
→,
R−
and
1
N,
∈0
}
N
1
.
Definition 2 ([21]). The first-ordgener forward (delta) and backward (nabla) differences of u are alized falling function is defined by:
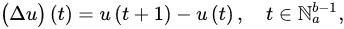
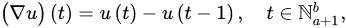
respectively. The-order delta and nabla differences of u are defined recursively by
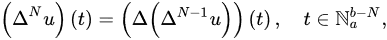
and:
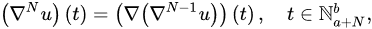
respectively.
Definition 4 ([14]). Let
u̲
:
N
a=
→
R
and
Γ
ν
>(
0
. Then, the
ν
t
h
+
-order delta fractional sum of u based at a is defined by:
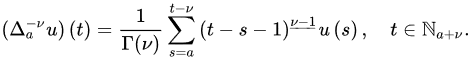
Definition 5 ([14]). Let
u1
:
)
N
aΓ
+(
1t
−
→
Rr
and
+
ν
>1
0
)
. Then, the
ν
t
h,
-
ford thoser nabla fractional sum of u based at a is defined by:
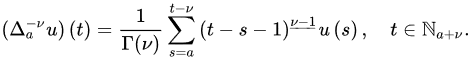
Definition 6 ([14]). Let values of t and r such that the right-hand side of this equation makes sense. If N+
a1
→
R
,
ν
>
0
is a nd choosonpositive integer and
N
1
isuch not a nonpositive integer, then we use the convention that − t
1
<
νr
≤
N̲
. The
ν
t=
h0
-ord. Ther Riemann–Liouville delta fractional difference of u generalized rising function is defined by:
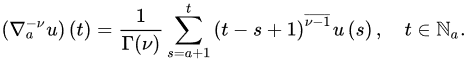
Definition 7 ([14]). Let ,
νΓ
>
0(
and choose
t
N
∈+
r
N
)
1
such that
N
−Γ
1
<(
ν
≤t
N
)
. Then, the
ν
t
h,
-
ford thoser Riemann–Liouville nabla fractional difference of u is defined by:
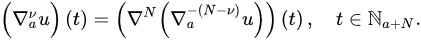
Definition 8 ([16]). Le values of t and r so that the right-hand side of this equation is sensible. If t is a nonpositive integer, but N
a
→
R
,
ν
>
0
ais nd
ν
∉
N
ot .a Thennonpositive integer, the
ν
t
h
-ordn wer Caputo delta fractional difference of u is defined by:
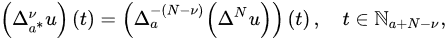
wuse the convention there at
[t
ν
]
+
1r
. If
¯
ν
=
N
∈=
N0
, then:
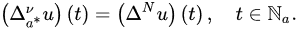
Definition 93 ([17][22]). Let
a
−b
N
+
1
→
R
and
. Then, the
N
ν
t1
h
. The first-order Caputo nabla fractionalforward (delta) and backward (nabla) differences of u isare defined by:
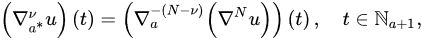
where
N
=
⌈
ν
⌉
.
3. Oscillation
3.1. Oscillatory Behavior of Delta Fractional Difference Equations
Consider the following higher-order nonlinear delta fractional difference equations involving the Riemann–Liouville and the Caputo operators of arbitrary order:
(
Δ
ν
u
)
(
t
)
+=
f
1
(
t
,
u
(
t
+
ν
)
)
=
r
1
(
t
)
+−
f
2
(
t
,
u
(
t
+
ν
)
)
,
t
∈
N
a
,
b
(
Δ
−
(
k
−
ν
)
u
)
(
t
)
|
t
=
a
=
u
k
∈
R
,
k
=
1
,
2
,
⋯
,
N
,
and:
(
∇
Δ
*
ν
u
)
(
t
)
+=
f
1
(
t
,
u
(
t
+
ν
)
)−
=
r
1
(
t
)
+
f
2
(
t
,
u
(
t
+−
ν1
)
)
,
t
>
a
≥
0
,
(
Δ
k
u
)
(
t
)
|
t
=
a
=
u
¯
k
∈
R
,
k
=
0
,
1
,
2
,
⋯
,
N
−
1
.
(3)
Here,
ν
>
0
and choose
N
∈
N
1
such that
N
−
1
<
ν
≤
N
;
f
2
:
[
a
,
∞
)
×
R
→
R
and
r
1
:
[
a
,
∞
)
→
R
are continuous. A solution u of (1) (or (2)) is said to be oscillatory if for every natural number M, there exists
t
≥
M
such that
u
(
t
)
u
(
t
+
1
)
≤
0
; otherwise, it is called non-oscillatory. An equation is said to be oscillatory if all of its solutions are oscillatory.
Let
p
2
:
[
a
,
∞
)
→
R
+
be continuous and
β
,
γ
be positive real numbers. We make the following assumptions:
(A1) The functions
f
i
satisfy the sign condition
u
f
i
(
t
,
u
)
>
0
,
i
=
1
,
2
,
b
u
≠
0
,
t
≥
a
;
(A2)
f
1
(
t
,
u
)
≥
p
1
(
t
)
u
β
and
f
2
(
t
,
u
)
≤
p
2
(
t
)
u
γ
,
u
≠
0
,
t
≥
a
;
(A3)
f
1
(
t
,
u
)
≤
p
1
(
t
)
u
β
and
f
2
(
t
,
u
)
≥
p
2
(
t
)
u
γ
,
u
≠
0
,
t
≥
a
.
In [18], Senem et al. established some oscillation theorems given in the sequel.
Theorem 1 ([18]). Lret (A1)–(A2) bspe satisfied with
β
>
γ
. If:
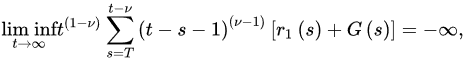
and:
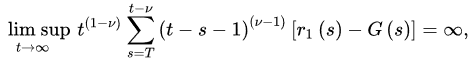
for ectivery sufficiently large T, wher. The:
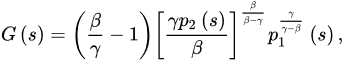
then Equation (1) is oscillatory.
Theorem 2 ([18]). Let
t
and (A1)–(A3) be satisfied with
βh
<
γ
. If
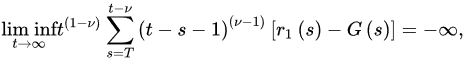
and:
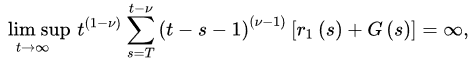
f-or devery sufficiently large T, where G is defined as in Theorem 1, then every bounded solution of Equation (1) is oscir delta and nabllatory.
Theorem 3 ([18]). Let (A1) and (A2) be satisfied with
β
>
γ
. If:
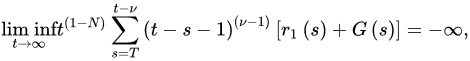
and:
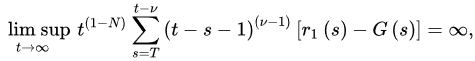
iffor every sufficiently large T, where G is defined as in Theorem 1, then Equation (2)rences of u is oscillatory.
Theorem 4 ([18]). Lret
ν
≥
1
an d (A1)–(A3) be satisfied with
β
<
γ
. If:
and:
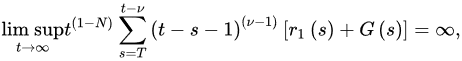
fofined r every sufficiently large T, where G is defined as in Theorem 1, then every bounded solution of Equation (2) is oscillatorcursively by.
Following the work in [18], Li et al. [19] investigated the oscillation of forced delta fractional difference equations with the damping term of the form:
(
1
+
p
3
(
t
)
)
(
Δ
ΔN
ν
u
)
(
t
)
+=
p
3
(
tΔ
)
(
Δ
ν
N
u−
)1
(
t
)
+
f
3
(
t
,
u
(
t
)
)
=
g
1
(
t
)
,
t
∈
N
0a
,b
−
N
(
,
Δ
(4)
and:
−
(
1
−
ν∇
)
N
u
)
(
t
)
=
|(
∇
t(
=
0∇
N
=−
1
u
0
u
∈)
R)
,
(
t
where
0
<,
ν
<t
1∈
;
pN
3
,
a
g
1+
:N
N
0b
→,
R
and
respectively.
Definition 4 ([21]). Let
f
3
:
N
0a
×
R
→
R
such that:
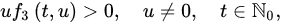
andand
p
3
(
t
)
>
−
1
for
t
∈
N
0
.
Theorem 5 ([19]). For. Then, the t
0h
∈
N
0
, supp-ose that:
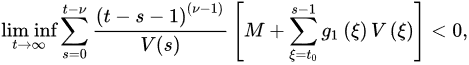
anrder d:
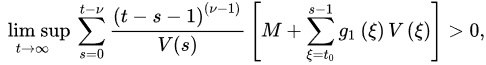
where M is a consltant and:
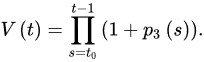
Then, Equa fractional (3) isum oscillatory.
Theorem 6 ([19]). For
t
0
∈
N
0
,f suppose that:
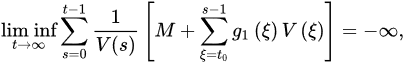
an based:
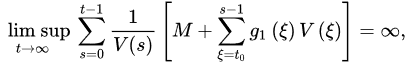
where M is a constant and V t a is defined as in Theorem 5. Then, Equation (3) is oscillatorby.
In this line, Seçer et al. [20] investigated the oscillation of the following nonlinear delta fractional difference equations:
:
p
4
(
t
)
Δ
qa
1
−
(ν
t
)
u
)
Δ
ν
u
(
t
)
=
γ
1
Γ
(
γν
2
+
q
2
(
t
)
f
4
∑
s
=
a
t
0
t
−
1ν
+
ν
(
t
−
s
−
1
)
(
−
ν
)
u
(
s
)
=
0
,
(5)
for
t
∈
N
t
0
+
1
−
ν
, Here,
0
<
ν
≤
1
,
γ
1̲
and
γ
2
are the quotients of two odd positive numbers such that
γ
1
γ
2
=
1
,
p
4
,
q
1
and
q
2
are positive sequences,
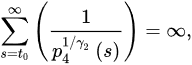
f
4
:
R
→
R
is continuous, and:
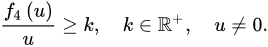
Theorem 7 ([20]). If there exists a positive sequence ϕ such that:
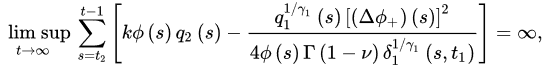
then Equation (4) is oscillatory. Here:
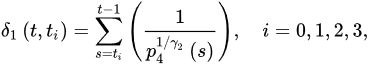
and,
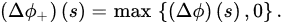
Theorem 8 ([20]). Let ϕ be a positive sequence. Furthermore, we assume that there exists a double sequence such that:
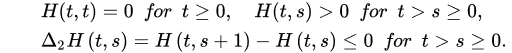 If:
If:
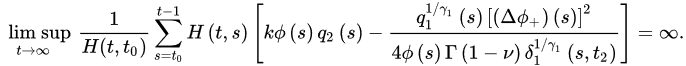
Then, Equation (4) is oscillatory.
If we choose the double sequence:
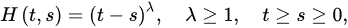 we have the following corollary.
we have the following corollary.
Corollary 1 ([20]). Under the conditions of Theorem 8 and:
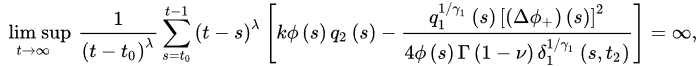
then Equation (4) is oscillatory.
In [21], Chatzarakis et al. studied the oscillatory behavior of the delta fractional difference equation of the form:
Δ
(
(
Δ
ν
u
)
(
ts
)
)
γ
3
+
q
3
(
t
)
f
5
(
u
(
t
)
)
=
0
,
t
∈
N
a
t
0
+
1
−
ν
,.
whereDefinition 5 ([21]). Let
1N
;
γa
3
+
>
01
is a quotient of odd positive integers;
q
3
is a positive sequence, and
f
5
:
R
→
R
is a continuous function such that:
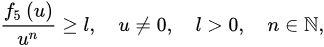
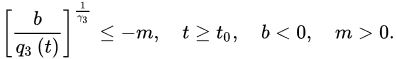 We also assume:
We also assume:
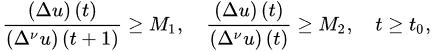 for some positive constants
M
1
,
for some positive constants
M
1
,and
2
and for all
(
Δ
ν
>
u
)
(
t
)
≠
0
and. Then, the
(
Δ
ν
u
)
(
t
+h
1
)
≠
0
, and:
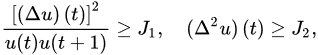 for some positive constants
J
1
and
J
2
.
Theorem 9 ([21]). Assume:
for some positive constants
J
1
and
J
2
.
Theorem 9 ([21]). Assume:
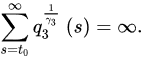 Fu-orthdermore, assume that there exists a positive sequence r˜ such r nabla fracthat:
Fu-orthdermore, assume that there exists a positive sequence r˜ such r nabla fracthat:
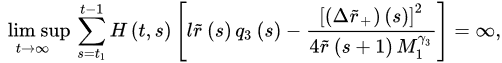 where:
where:
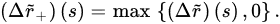
Then, Equation (5) is oscilalatory.
Theorem 10 ([21]). Assume:
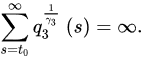
Furthermore, assume that there exists a positive sequence of u r˜ band a double positive sequence
H
˜
(
t
,
s
)
such thed at:
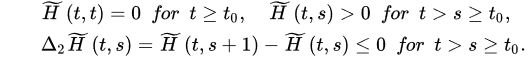
If:
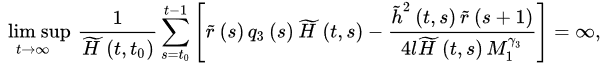
where:
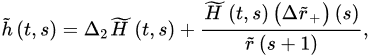
then Equation (5) is oscillatory.
Theorem 11 ([21]). Assumde that there exfists a positive sequencne
r
˜
suchd thatby:
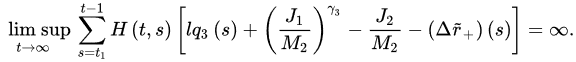
Then, Equation (5) is oscillatory.
Motivated by the above works, Adiguzel [22][23] considered the oscillation behavior of the solutions of the following delta fractional difference equations:
(
rΔ
2a
(
t−
)
ν
(
Δ
ν
u
)
(
t
)
)=
+
q1
4
Γ
(
t(
)
ν
f)
6
∑
s
=
a
t
0
t
−
1ν
+
ν
(
t
−
s
−
1
)
(
−
ν
)
u
(
s
)
=
0
,
t
∈
N
t
0
+
1
−
ν
,
(6)
and:
Δ
(
c
1
(
t̲
)
Δ
(
c
2
(
t
)
(
r
2
(
t
)
(
Δ
ν
u
)
(
t
)
)
)
)
+
q
4
(
t
)
∑
s
=
t
0
t
−
1
+
ν
(
t
−
s
−
1
)
(
−
ν
)
u
(
s
)
=
0
,
t
∈
N
a
t
0
+
1
−
ν
,.
3.2. Oscillatory Behavior of Nabla Fractional Difference Equations
Let
ν
>
0
,Definition 6 ([21]). and choose Let ∈
N
1
such that
N
−
1
<
ν
<
N
. Take p,
q
i
,
r
:
N
a
+
N
−
1
→
R
, ,
2
,
⋯
n
;and choose
,
∈
f
2
:
N
a
+
N
−
1
×
R
→
R
;
f
:
R
→
R
;
p
1
,such that
p
2
:
N
a
+
N
−
1
<
ν
→≤
R
+
N
; w,. The N
a
→
R
; q,
g
:
N
a
+
1
→
R
; x,
z
:
N
1
→
R
;
w
2
:
N
1
→
R
+
; -order Riemann–Liouville delta fractional difference of u is defined by is a positive function defined on:
N
1
,
β
,
γ
are positive real numbers;
1
≤
i
≤
n
are the ratios of odd positive integers with
λ
1
>
⋯
>
λ
l
>
1
>
λ
l
+
1
>
⋯
>
λ
n
.
We make the following assumptions:
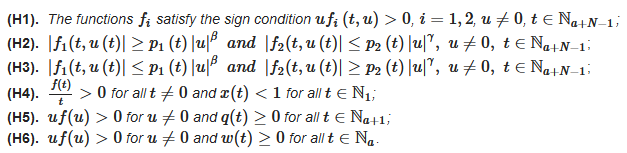
Alzabut et al. [24] initiated the study of the oscillation of solutions of nabla fractional difference equations. In [24], the authors established several oscillation criteria for the following nonlinear nabla fractional difference equations involving the Riemann–Liouville and Caputo operators of arbitrary order.
(
(
∇
a
+
N
−
2ν
ν
u
))
(
t
)
+
f
1
(
t
,
u
(
t
)
)
=
r
(
t
)
+
f
2
(
t
,
u
(
t
)
)
,
t
∈
N
a
+
N
−
1
Γ
,
(
∇
a
+
N
−
2
−
(
1
−
ν
)
u
)∑
(s
t
)
|
t
=
a
+
N
−
1
t
=
u
(
at
+
N
−
1s
)
=
c
,
c
∈
R
,
and:
(
∇
a
+
N
−
1
*
ν
u
)
(
t
)ν
−
+
f
1
¯
(
t
,
u
(
ts
)
)
=
r
(
t
)
+
f
2
(
t
,
u
(
t
)
)
,
t
∈
N
a
+
N
−
1
,
(
∇
k
u
(
a
+
N
−
1
)
=
b
k
,
b
k
∈
R
,
k
=
0
,
1
,
2
,
⋯
,
N
−
1
.
A solution u of
Definition 7 (30[21]). (or (31)) is said to be oscillatory if for every natural number M, there existsLet
such that
N
u
(
ta
)
u+
(
t1
+
1
)
≤→
0R
;otherwise, it is called non-oscillatory. An equation is said to be oscillatory if all of its solutions are oscillatory.
Theorem 37 ([24]). Let, f>
2
=
0
and Condition (H1) chold. If:
then Equation (30) is oscillatory.
Theorem 38 ([24]). Let Conditions (H1) and (H2) hold with
and
N
γ
=1
1
. If:
then Eqsuation (30) is oscillatory.
Theorem 39 ([24]). Leth Conditions (H1) and (H2) hold wihath
and
<
γν
<≤
1N
. If:
tThen, Equation (30) is oscillatory.
Theorem 40 ([24]). Lthet Conditions (H1) and (H2) hold with
Theorem 41 ([24]). Let
Theorem 42 ([24]).
Theorem 43 ([24]).
Theorem 44 ([24]).
Following the work in [24], Abdalla et al. [25] established new oscillation criteria for (30) and (31) using the fractional Volterra sum equations and Young’s inequalities. The authors in [25] observed that the cases >
1
and
γ
>
β
>
1
were not considered for (30) in [24]. The purpose of the paper [25] was to cover this gap and establish new oscillation criteria that improve the results in [24].
Theorem 45 ([25]).
Theorem 46 ([25]).
Theorem 47 ([25]).
Theorem 48 ([25]).
f-or sufficidently large T, where H is defined in Theorem 45, then every bounded solution of Equation (31) is oscir Riemann–Liouvillatory.
In alignment with the above works, Abdalla et al. [26] investigated the oscillation of solutions for nabla fractional difference equations with mixed nonlinearities of the forms:
Theorem 49 ([26]). Let:
then Eqof uation (32) is oscillatory.
Corollary 3 ([26]). Ldet
l
=
n
fin (32), then
λ
1
>
λ
2
>
⋯
λ
n
>
1
.d Supposeby:
,
ν
q
iu
)
(
t
)
≥
0
,
i
=
1(
,
2
,∇
⋯
nN
.If (35) and (36) hold for some constant
(
K
1∇
>
0
a
, then Equation (32) is oscillatory.
Corollary 4 ([26]). Let
l
=
0
in (32), then
−
1
>(
λN
1
−
>
ν
λ
2)
>
⋯
u
λ)
n
. Suppose
p
(
t
)
<
0
,
q
i
(
t
)
≤,
0
,
i∈
=
1
,N
2
,
⋯
na
.If (35) and (36) hold for some constant
+
KN
2
>.
0
, then Equation (32) is oscillatory.
Corollary 5 ([26]). Let:
Theorem 50 ([26]). Assume that Condition (34) holds. If:
Corollary 6 ([26]).
Corollary 7 ([26]).
Corollary 8 ([26]).
Following the above trend, in [27], Alzabut et al. considered the following forced and damped nabla fractional difference equation:
Motivated by the paper [24], the authors [28] investigated the oscillation of a nonlinear fractional nabla difference system of the form:
Theorem 55 ([29]). Let Condition (H6) hold. If the inequality:
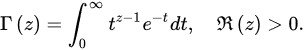
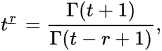
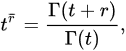
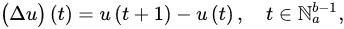
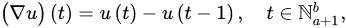
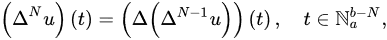
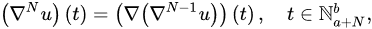
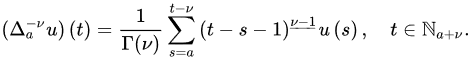
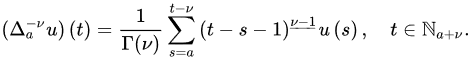
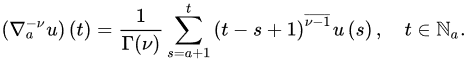
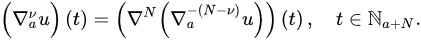
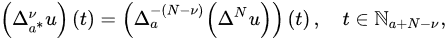
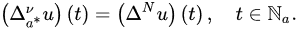
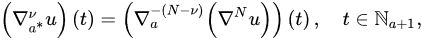
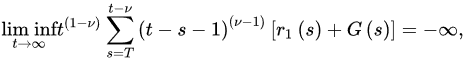
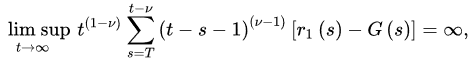
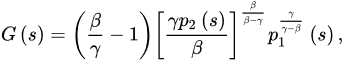
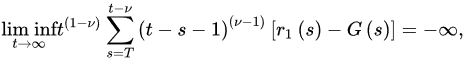
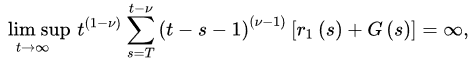
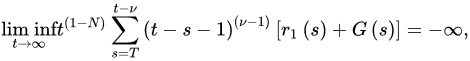
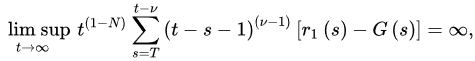

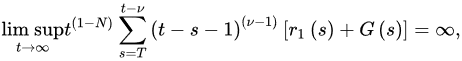
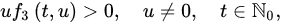
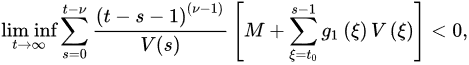
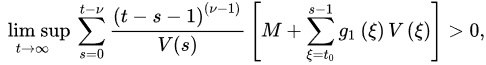
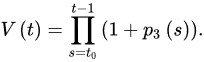
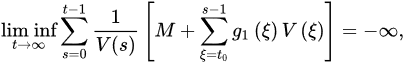
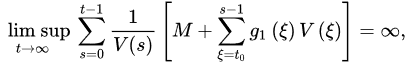
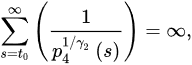
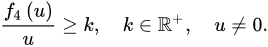
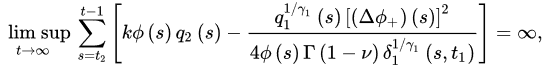
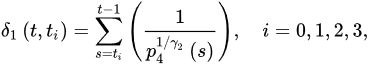
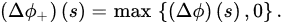
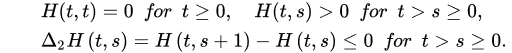 If:
If:
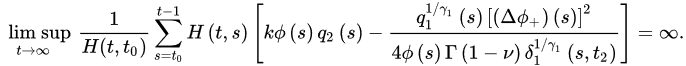
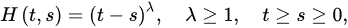 we have the following corollary.
we have the following corollary.
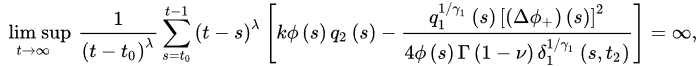
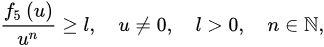
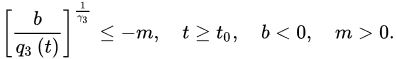 We also assume:
We also assume:
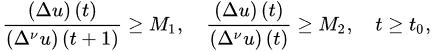 for some positive constants
for some positive constants 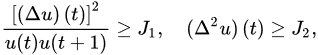 for some positive constants
for some positive constants 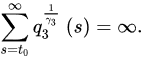 Fu-orthdermore, assume that there exists a positive sequence r˜ such r nabla fracthat:
Fu-orthdermore, assume that there exists a positive sequence r˜ such r nabla fracthat:
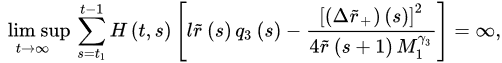 where:
where: