1. Introduction

Both OH and NH infrared (IR) stretching frequencies have been shown to be good descriptors of hydrogen bonds. Hydrogen bonding is a key parameter for important chemical and biological molecules, not the least in water. However, these resonances may be be difficult to identify in strongly hydrogen bonded cases. Density Functional Theory calculations have become a standard method to calculate IR frequencies. Coupled cluster calculations may predict frequencies accurately but only for small molecules due to the huge computer requirements. For larger molecules DFT methods produce results that with a scaling reproduce most of the infrared spectrum. The exception is in many cases the OH and NH stretching vibrations, as the potential energy well in these cases can be very asymmetric. The DFT calculations may be performed both in the harmonic or the anharmonic approximation. The aim is to give suggestions to the use of functionals, basis sets and the harmonic vs. anharmonic approximation in the calculations of OH and NH stretching frequencies in a practical manner. "static" in this context means non-tautomeric.

2. DFT  Calculations

Performing DFT calculations of XH (X=O or N) stretching frequencies in hydrogen bonded systems a number of decisions have to be made:  appropriate functional, basis set, harmonic vs. anharmonic approximation and computing time in relation to the size of the molecule. Examples of medium large and large molecules or systems see Figure 1.  A different type of large system is a binary alocohol + cyclohexane. ^[1]

Figure 1.   c From Ref. ^[2]

A recent paper has summarized the methods to calculate IR frequencies in general. ^[3]  In the regime of weak hydrogen bonds Futami et al.^[4] calculated the OH stretching frequencies of (R)-1,3-butanediol using the VPT2 approach ^[5] and the B3LYP/6-311++G(3df,3pd) functional and basis set.  

In the same frequency range Medel and Suhm ^[6] investigated a series of alcolhols as seen in Figure 2.  The frequency range is very narrow, but very accurate predictions were obtained dividing the alcohols according to primary, ceconday and tertiary (see Figure 2).

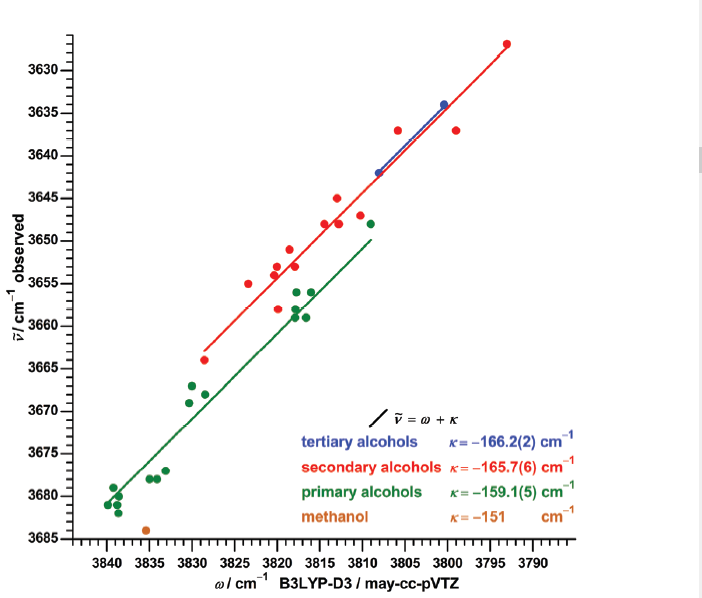Figure 2.  Plot of observed OH stretching frequencies vs. calculated (B3LYP-D3/may-cc-pVTZ) taken from Ref. 6 with permission from Royal Society of Chemistry.

Spanget-Larsen, Hansen and Hansen investigated two different types of hydrogen bonded systems either with OH or RNH groups as hydrogen bond donors. ^{[[7],[8],[9]]}.  The acceptors cover carboxylic acids, esters, aldehydes, ketones, amides, thioxoketones, nitroso groups and heterocyclic nitrogens of e.g. pyridines.  In case of oxygen as donors the compounds are both "static" and tautomeric.  The hydrogen bond types cover both intra and intermolecular ones.  Most of the former and many of these compounds are of RABH type.  ^[10]

Figure 3. Linear regression of observed OH stretching wavenumbers ν_OH (cm^-1) on those predicted within the harmonic approximation, B3LYP/6-31G(d). Taken from Ref. 8 with permission from Elsevier.

The regression line of Figure 3 is: ν_OH{Obsd} = –757 + 1.171*ν_OH{Harm} (R = 0.985, SD = 74 cm^-1).  Eq. 1

In case of the -NHR derivatives a non-linear relationship was found (see Figure 4). ^[9]

Figure 4.  Plot of observed NH stretching frequencies vs. calculated (B3LYP/6-311++G(d,p).  Data from Ref. 9 with permission from MDPI.

However, performing the same calculation but with B3LYP/6-31G* gave a correlation:

P(harm) =3470-6.714 x 10⁷  exp(-Harm/270.3).  R² = 0.965 and SD= 38.1 cm^-¹.  Eq.2

Figure 5.  Plot of NH stretching frequencies calculated with basis sets 6-311++G(d,p) vs. 6-31G(d).  Data from Ref. 9 with permission from MDPI.

This is very similar to the much higher basis set used in Figure  5.  As a matter of fact, the two types of calculations can be correlated with R²=0.991 and SD = 15 cm^-1.  Calculations were also performed with the B3PW91 functional.  These were linearly correlated to  the B3LYP calculations with a R² = 0.9999 and and SD of 2.6 cm^-1. 

Performing anharmoic calculations in case of the -OH stretching frequencies led to the plot seen in Fig. 6. A good correlatation is found between the harmonic and the anharmonic data as seen in legend of Figure 6.

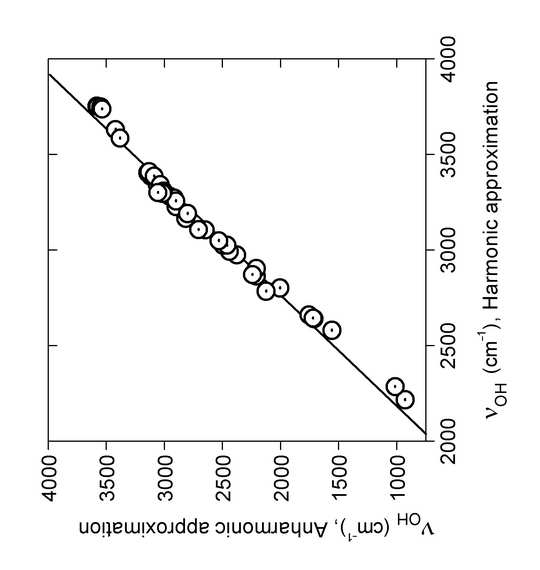Figure 6. Linear regression of OH stretching wavenumbers ν_OH (cm^-1) calculated in the PT2 anharmonic approximation on the corresponding harmonic wave numbers, B3LYP/6-31G(d). The regression line is ν_OH{Anh} = –2791 + 1.732*ν_OH{Harm} (R = 0.99, SD = 88 cm-1). From Ref. 8 with permission from Elsevier.

In case of the NH stretching frequencies a similar plot is found:

Figure 7.  Plot of NH stretching frequencies anharmonic (VPT2 B3LYP/6-31G(d) vs. the harmonic one.  Taken from Ref. 9 with permission from MDPI.

A calculation doing a B3LYP VPT2 calculation at molecule a in Figure 1, but with the higher basis set 311++G(d,p) took 28 days using 8 processors (this may of course be done quicker on some computers, but it is still a long time) and resulted in a predicted NH stretching frequency of 2930 cm-1 as compared to the experimental value of 2760 cm^-1.

Recently, DFT calculations using DVPT2 and GVPT2 funtionals and the B3LYP-D3 basis set gave very similar results to those described above.  This was ascribed to the inability to account accurately for large amplitude vibrational motions. http://dx.doi.org/10.2139/ssrn.4702777

Considering the use of the simple basis set G(d).  A strongly hydrogen bonded example is the enol form of nitromalonamide.  PT2 calculations gave 1428 cm^{-1 [11]}, whereas the P(harm) prediction gave 1920 cm^-1.  The latter result was confirmed by using Car-Parrinello and path integral molecular dynamics (CPMD) calculations.  ^[12]

In designing a scheme for prediction of XH stretching frequencies in hydrogen bonded systems, it is important to include molecules with extensive conjugation, steric interations and a range of hydrogen bond strengths varying from very weak to very strong.  This has led to the simple equations 1 and 2, which can predict OH and NH stretching frequencies in even quite large molecules based on simple calculations.  The use of these correlations also avoid the pitfall of the fortuitous correspondence between a single calculated XH frequency and an experimental value.  Furthermore, the calculations allow assignment of a large number of experimental XH stretching frequencies not so far assigned either because they are broad due to strong hydrogen bonding or in regions with many CH stretching frequency absorptions.