1. Introduction

A shape of geological discontinuities plays an important role in influencing the stability of rock masses. Many approaches have been used for its determination. The method of Barton and Choubey 1977 ^[1] is well known in geotechnical practice. A visual comparison is preferred way for determining values in geotechnical practice. A quick and easy estimate is probably one of the main reasons for this preference. However, this method is very subjective. Therefore, objective methods for estimation are searched. Most of them are based on measurement of diference between fracture surface and an appropriate reference surface. This contribution shows which reference surface is the right one.

2. Characteristics of Fracture Surfaces

Two height characteristics of the 3D profiles are obviously introduced as numerical indicators of a "distance" between surface 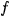f to be study and the reference surface :

where 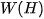is the surface width (height) in pixels. From a statistical point of view, is the average distance of and , is the standard deviation. From a functional analysis point of view, these expressions are the distances of these surfaces in Manhattan (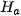) or Euclidean (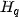) metrics. The question is how to construct a reference surface.

3. Monomial Base in Two Variables

Reference surface can be construct as a linear combination of monomials in two variables 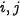, i.e. as a standard polynomial in two variables 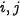, i. e.

using by least squares method. However, polynomial relatively high degree of polynomial is necessary for useable reference surface. This problem leads to a large system of linear equation. It is badly conditioned because monomial base is “very non-orthogonal” and solution is unuseable – see Figure 1.

Figure 1. Fracture surface (on the left) and its “reference approximation” constructed as standard polynomial of 19^th degree.

4. Standard Fourier base in two variables

The scanned relief ; for and is possible to expand into Fourier partial sums (Standard Fourier sum) combining both the sine and cosine harmonics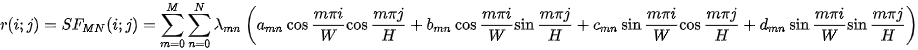

where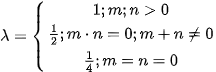

and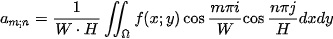

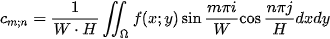
where

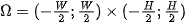
is a ground plan of the scanned relief ^[2].

This base is orthogonal and it is not necessary to solve any equation system. It means it is relatively easy to find very long expansion. However, there is an other problem.

Theorem (Dirichlet): The Fourier series 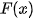of a piecewise continuous function 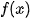on interval converges to the arithmetic mean of left-hand and right-hand limits of 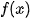for ; i. e.

If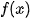is discontinuous in then and converges to the arithmetic mean of these limits (convergence in the mean). If is continuous in then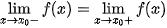and converges to the 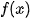in In the case of 2D by analogy.

The Fourier series converges in the same way to periodic extension of 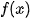on 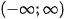. The periodic extension of continuous fracture surface which is expanded to (odd extension) is generally not continuous. In Figure 2 on the left, we can see the periodic extension of function 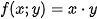 defined on 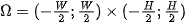. Due discontinuities on the surface boundary, does not converge to surface points here, but to the arithmetic mean of opposite boundary points (so-called convergence in the mean).

Figure 2. Different periodic extensions of the profile to be expanded (red perimeter). Left – discontinuous odd extension by ; Right – continuous even extension by .

5. Cosine Fourier Base in Two Variables

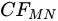The scanned relief 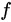; for  and  is possible to expand into cosine Fourier partial sums 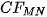defined as follows: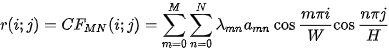

where  

is a ground plan of the scanned relief ^[3].

Periodic extension of continuous fracture surface which is expanded to 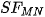is discontinuous (see Figure 2 on the left). It means it suffers from convergence in the mean (se above) and also by so called Gibbs effect, i. e. by ”fake waves” nearby discontinuities. Periodic extension of continuous fracture surface which is expanded to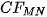is always continuous (see Figure 2 on the right). Therefore, it does not suffer from these defects. In Figure 3, comparing the  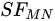and 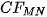of the same “profile” - function .

Figure 3. “Original profile” modelled by the function 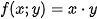(in the middle) was expanded by 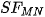(on the left) and by (on the right) in both cases.

6. Results

In Figure 4, expansion by for real surface. Convergence in the mean and also Gibbs effect are evident in this case.

Figure 4. Aproximation of a real profile (on the left) by 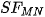(on the right).

Figure 5 illustrate expansion by 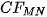. It has no defects. For 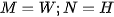, the 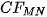goes precisely through the surface points.

Figure 5. Aproximation of a real profile with resolution (on the left) by(on the right).