2. Structure and Principle
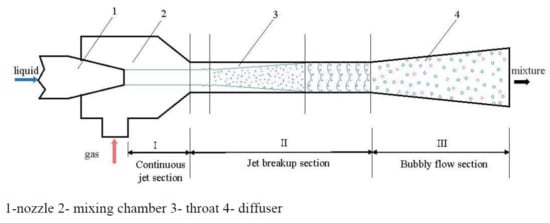
The LGJP is mainly composed of four parts: the nozzle, the mixing chamber, the throat and the diffuser (see
Figure 1). When the working fluid (liquid) is spewed out at high speed from the nozzle, the gas around it is sucked to form negative pressure in the mixing chamber, and the suction gas is sucked in. The two fluids are then mixed in the throat and exchange momentum, so that the velocity of the working fluid decreases and the velocity of the sucked gas increases. At the outlet of the throat, the velocities of the two fluids are basically the same. The velocity of the mixed fluid in the diffuser gradually decreases while the pressure gradually increases, converting kinetic energy into pressure energy.
Figure 1. Working principle and structure diagram of the LGJP.
The liquid and gas in the mixing chamber have different velocities, and when the two are mixed, a large energy loss occurs, which reduces the efficiency of the LGJP. At present, the methods to improve the mixing efficiency mainly include structural optimization
[2][4][5][6][5,7,8,9] and the use of pulsed jets
[16][19], that is, a pulsed liquid–gas jet pump (PLGJP). There are two main ways of pulse generation: one is to install the pulse generator at the front of the nozzle without changing the original liquid–gas jet pump structure, so that the working liquid has a certain pulse frequency
[15][18]; the other is the centrifugal jet vacuum pump
[6][9] (CJVP).
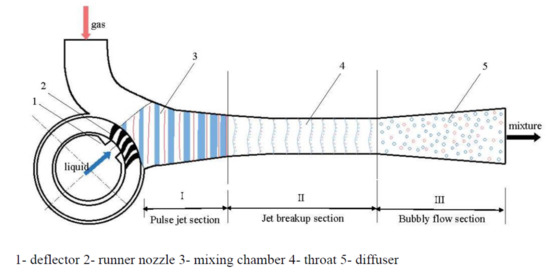
The CJVP adopts the structure of the runner nozzle and the deflector on the basis of retaining the LGJP mixing chamber, throat, diffuser and other structures (see
Figure 2). Only when the runner nozzle communicates with the deflector and the mixing chamber can the working fluid be thrown out into the mixing chamber through the runner nozzle, and the connection time of each runner nozzle is limited, thereby generating a pulsed jet.
Figure 2. Working principle and structure diagram of the CJVP.
Pulse jets can rapidly mix fluids, thereby reducing the length of the mixing section. The PLGJP has good suction performance because the inertial force of the pulse jet increases the working pressure of the jet pump, thereby improving the suction capacity. There are two ways for the pulsed jet to carry the gas into the suction chamber: one is the viscous effect between the fluids, and the other is that the gap between the pulsed jets ejected from the nozzle plays the role of the piston pump. The pulse frequency generated by a pulse generator is usually less than 10 Hz
[6][9], while the pulse frequency generated by a CJVP can reach more than 1000 Hz, so it has higher suction capacity and efficiency.
3. Research Status of the LGJP
Jet pump design theory has been studied for more than 150 years. As early as the 1860s, the German scholar Zeune
[17][1] established the theoretical basis of jet pump design according to the momentum theorem. Although the research of the jet pump has a long history, it was not until the 1930s that, with the continuous development of science and the continuous improvement of the knowledge system about fluid mechanics and aerodynamics, the theoretical research and practical application of the LGJP were further developed. The specific concept of the LGJP was first proposed by the scholar Hoeffer
[2][5] in 1922 and was successfully applied in the vacuum pumping experiment of a condenser. Subsequently, a large number of research papers on the LGJP were published in various magazines. Then, through a large number of experimental studies conducted by Rammingen
[18][2], they discovered the sudden rise in the pressure of the two-phase fluid after mixing at the throat. Then more scholars began to study the effect of changing the length of the throat on the mixing performance of the gas–liquid two-phase flow on this basis to obtain the approximate range of the optimal length. At the same time, Bonnington
[3][6] found in the gas–liquid two-phase mixing experiment of the transparent throat that as the flow rate of the LGJP increased, the entrainment efficiency of the LGJP decreased. The gas effect was obviously improved. Within a certain length of the throat, the longer the throat, the better the entrainment effect.
Witte
[17][1] was the first to combine the application of the Euler equation with the theoretical analysis of the LGJP and described the flow process of the two-phase fluid in the tube in detail, thereby defining the mixed shock wave. He found that the two-phase fluid in the flow mixing process showed a great influence on the compressed gas, and the structure of the LGJP was further optimized. Higgins
[4][7] deduced the unary relationship of the drag coefficient introduced by the LGJP and verified the correctness of the theoretical derivation through experiments. The results showed that the suction performance was the best when the two-phase fluid was mixed at the throat outlet of the LGJP. Lu
[5][6][8,9] derived the basic performance equation of the LGJP based on the simplified method of unitary flow, optimized some parameters, took into account the influence of different flow rates of each structural plane in the pump and verified the accuracy of the theoretical equation through experiments. On this basis, he comprehensively summarized the research results of experts and scholars at home and abroad on the jet pump. In 1989, he published a monograph on jet technology, the Theory and Application of Liquid–Gas Jet Pump Technology, and in 2004, he published again, the Theory and Application of Jet Technology. Long
[7][10] obtained the performance equation of the LGJP under the condition of constant determination based on the relevant theories of fluid mechanics on the premise of constant determination of the basic performance equation of the LGJP, laying a foundation for further study and analysis of the internal flow mechanism of the unsteady liquid–gas jet pump.
Betzler
[5][8] used experiments to analyze the influence of the size of the diffuser, and the results showed that the LGJP displayed better suction performance when the two-phase fluid was completely mixed before entering the diffuser. When optimizing the overall structure of the LGJP, it was found that when the length–diameter ratio of the throat was 23, the gas isothermal compression rate of the LGJP could reach about 19%. Based on a large number of experimental results, Cunningham
[18][2] analyzed that the gas isothermal compression efficiency of the LGJP could reach 40% or even higher under optimal working conditions as long as the structural parameters of the LGJP, such as the throat length–diameter ratio and throat diameter, were given reasonable values. When Neve
[4][7] tested the performance of the diffuser of the LGJP, he found that the performance of the diffuser was related to the degree of homogeneous mixing of the two-phase fluid in the diffuser and the inconsistency of velocity at the entrance of the diffuser.
Haidl
[8][11] used a converging nozzle to measure the suction capacity of a conventional liquid–gas jet pump while experimenting with the performance of the pump in less stable configurations, providing the best design suggestions for units with various geometric shapes, directions and operating conditions to minimize gas entrainment. Opletal
[10][13] studied the influence of the geometric parameters of the jet pump on the injection ratio and mass transfer coefficient, showed that the working structure parameters had an important influence on the injection performance of the LGJP and proposed a method to evaluate the mass transfer performance. Kim
[11][14] and others simulated and analyzed that the suction rate of a LGJP was proportional to the fluid circulation velocity in the pump, but as the nozzle diameter increased, the suction volume decreased. The Rahman
[9][12] experiment found that nozzle geometry affected the gas entrainment rate and pressure drop in the pump and that a low nozzle coefficient could form a higher vacuum in the pump. Sharma
[12][15] designed different shapes of nozzles for experiments and analyzed and compared the performance of LGJPs of various shapes. Sung
[1][4] compared the entrainment flow of the LGJP in three installation modes: horizontal, vertical upward and vertical downward and found that the gas entrainment rate installed vertically downward was the best. Liu
[13][16] observed the flow process in the pump and measured the pressure distribution in the pipe through experiments. Based on the expression of American scholars, he supplemented the expression for the optimal throat length and proposed that the isothermal compression efficiency during vacuum pumping could reach 20~50% of the optimal parameter design of the LGJP. Liao
[14][17] studied the performance of the LGJP through experiments and at the same time, used a method of numerical simulation to analyze the variation law and interaction of various parameters in the LGJP. He measured and recorded the pressure of the LGJP and flow data under different area ratios, and the influence of the area ratio on the inspiratory performance was determined by analysis. Gao
[15][16][18,19] used geometric parameters and working parameters, such as nozzles with different diameters, area ratio, throat distance and pulse frequency, to test the liquid–gas piston pulse jet pump and preliminarily mastered the stable conditions of the device operation. Ge
[19][20] proposed that when the throat-to-nozzle distance of the LGJP remained unchanged, the pressure ratio gradually decreases with the increase in the flow ratio. When the throat distance was 1.5 times the nozzle diameter, the efficiency of the LGJP was the highest, and the optimal throat distance range of the LGJP was determined to be 1.0 to 1.7 times the nozzle diameter.
Bhatkar
[20][21] experimentally studied the performance parameters of the LGJP. The results showed that the working efficiency increased with the increase in the flow ratio and decreased with the decrease in the pressure ratio. Wu
[21][22] conducted experiments under the same orifice Reynolds number and different liquid–gas flow ratio. The experiments showed that the bubble size in the pump was inversely proportional to the flow ratio, and the bubble diameter significantly changed at a low Reynolds number but changed little at a high Reynolds number. Eisallak
[22][23] experimentally analyzed the influence of inlet pressure on jet flow. The results showed that the performance of LGJP was improved when the inlet pressure was weak, and the pump efficiency was reduced when the inlet pressure was strong. Mikheev
[23][24] conducted an experimental analysis on the nozzle and capacity curve of the LGJP in the working environment where the inlet pressure was greater than 1 MPa. Zhang
[24][25] put forward the scheme of using the LGJP to recover casing gas and emphasized that the optimal distance between the nozzle and throat of the LGJP is different under different working fluid pressures.
Carvalho
[25][26] carried out numerical simulation research on the flow field of two-phase fluid in the throat of the LGJP with CFD software on the computer. Jiao
[26][27] analyzed the mathematical model of the LGJP, synthesized the empirical resistance coefficients of each structure of the LGJP as a dimensionless undetermined coefficient, determined its undetermined coefficient through a large number of experiments and obtained the correlation function expression. The mathematical model of single-phase flow was extended, and the mathematical model of two-phase flow of the LGJP was determined. At the same time, the results of multiple numerical simulations showed that compared with the single-phase flow model, the mathematical model of two-phase flow was more accurate in analyzing and comparing the performance of the LGJP. Ismagilov
[27][28] measured the performance of the LGJP and analyzed its characteristics by discussing the physics of the LGJP and establishing a mathematical model. Sharma
[28][29] conducted a simulation analysis on the influence of the turbulent flow effect in the CFD model on the internal flow and gas entrainment rate of the LGJP to optimize the design of the pump. Zhu
[29][30] studied the influence of the structure size on the suction performance of the LGJP and used the CFD numerical simulation method to study the relationship between the structural parameters of the nozzle area ratio, the throat, the nozzle distance, the throat length and the suction performance, respectively. Finally, the optimal range of each structure size under the highest efficiency was given. Semlitsch
[30][31] proposed that four alternative jet pump configurations have been explored, i.e., a chevron primary nozzle, an empirical primary nozzle, a primary nozzle with swilling inserts and a multiple injector nozzle. The simulation found that, with the chevron primary nozzle, the jet pump efficiency with the chevrons slightly increased (less than one percent). Using an elliptical primary nozzle, the diffuser was only partially better utilized with an elliptical primary jet pipe exit, but an improvement of the jet pump efficiency of approximately two percent was achieved. With the primary nozzle with swirling inserts, the ratio of flow momentum to mixing could be manipulated with an increase in guide vane height to optimize the primary jet structure. The efficiency of the jet pump increased by about 6% using a multiple injector nozzle. Zheng
[31][32] used a numerical simulation to simulate the internal flow field of the LGJP and found that with the increase in the liquid flow rate and the diameter of the diffuser outlet, the pressure dropped at the jet outlet and increased at the inlet of the jet pump accordingly. In addition, the wall flow effect increased, and the working efficiency decreased. Qin
[32][33] pointed out that the appropriate liquid inlet speed can stabilize the volume fraction of each phase in the LGJP, which is conducive to the normal injection of the ejector. The increase in the output pressure of the diffuser leads to steam liquefaction and affects the normal operation of the ejector. Gao
[33][34] conducted a three-dimensional numerical simulation calculation on the throat cavity contraction half angle of different LGJPs. It was found that the variation trend of the efficiency of the LGJP with the contraction half angle was similar to that of the pressure ratio, and there was a maximum value. The optimal suction efficiency interval was determined according to the reduction in the maximum suction efficiency by 5%, and the optimal range of the corresponding throat contraction half angle was 13.5°~17.1°. Wang
[34][35] simulated the mixed flow in a LGJP and divided the mixing process into three stages: coaxial flow, mixed shock flow and bubble flow. The mixed shock wave was the main factor affecting the mixed flow characteristics and the performance of the LGJP.
Yang
[35][36][37][38][36,37,38,39] designed the diffuser of the jet pump using the method of constant speed or constant pressure change, studied the influence of structural parameters on the internal flow field and found, through analysis that compared with the traditional conical diffuser, that the diffuser with constant speed or constant pressure change obtained a better performance and significantly shortened the length of the jet pump. There was an optimal combination of throat and diffuser. In order to improve the performance of an annular jet pump, the constant velocity/pressure rate of change method was used to design its diffuser, and the results showed that the prediction results of the RNG k-ε turbulence model were in better agreement with the experimental data than the standard, and this is an achievable k-ε turbulence model.
Sato
[39][40] used high-speed camera technology to observe the whole process of cavitation generation, development and collapse in the Venturi and observed the unstable sheet-like cavitation cloud in the diffusion section under a small cavitation number. Stutz
[40][41] observed the unstable sheet-like cavitating cloud of a Venturi tube with an X-ray device and dual light detector, found that the change in the cavitating cloud volume with time characterized the periodic law of cavitating cloud shedding and pointed out that the exit velocity had an important impact on the cavitating flow and the frequency of cavitating cloud shedding. Coutier
[41][42][42,43] carried out a numerical simulation of the unstable cavitating flow in rectangular Venturi, and the obtained results are in good agreement with the test results of Stutz
[40][41].
In order to improve the accuracy of the unsteady cavitation simulation of hydrofoil, Gu
[43][44][45][44,45,46] used the GCI evaluation method to study grid independence and dispersion error to determine the optimal number of grids and reveal the mechanism of instability and falling off of hydrofoil cavitation. Yazici
[46][47] studied the cavitating flow in the two-dimensional axisymmetric Venturi, found that the vibration frequency range of the cavitating flow was wide and observed that the high momentum bubbles eventually collapsed into small low momentum bubbles in the diffusion tube, and the vibration became smaller. Sayyaadi
[47][48] observed the fluctuating process of the cavitating flow in Venturi using a high-speed camera and found that compared with the working pressure, the cavitating number had a more significant impact on the pulsating frequency, eigenfrequency and dimensionless parameter
st of the length of the cavitating cloud and believed that the return jet was the main factor for cavitating cloud shedding. Xu
[48][49] studied the influence of the area ratio of the diffuser inlet to the nozzle outlet, the volume of the displacement container and the configuration of the suction gap on the performance of the Venturi counter flow diverter pumping system. The RFD system with an area ratio greater than 1 showed a higher efficiency, and the reduction in the volume pressure around the jet core led to cavitation, which led to a reduction in the lifting efficiency.
 Encyclopedia
Encyclopedia
