Computational Simulation Methods in Ship Broken Ice

Computational Simulation Methods in Ship Broken Ice: Comparison

Please note this is a comparison between Version 2 by Lindsay Dong and Version 1 by Fang Li.

The majority of sea ice in polar regions can be generalized into two types, (a) level ice that exists as a continuous form, and (b) broken ice that consists of discontinuous ice blocks. Broken ice includes brash ice that normally accumulates in ice channels, sliding ice pieces that form from breaking continuous ice, unconsolidated ice ridges generated by compression between ice floes, and ice floe fields (the most common broken ice condition in the polar region) that appear and evolve with natural processes. In recent years, computational simulation models have increasingly been used for the evaluation of ship operability under broken ice conditions, presenting some challenging issues. Models for different types of broken ice, including ice floes, ice ridges, brash ice, and sliding ice pieces, are reviewed separately. A ship’s response in broken ice is divided into two categories: resistance, which relates to the overall ship performance, and local loads, which relates to structural safety.

broken ice
ship
ice floe
computational simulation
discrete element method

1. Introduction

1.1. Forms of Broken Ice

Broken ice is discontinuous, consisting of myriad ice pieces. The ice size, shape, and distribution vary between different types of broken ice, and examples are shown in

Figure 1

. Brash ice rubbles,

Figure 1

a, are small compared to other types of broken ice and usually cover ice channels as floating layers with full concentration. The ice rubbles are relatively spherical due to repeating contact with ships and other ice rubbles. Unconsolidated ice ridge rubbles,

Figure 1

b, share a similar piling feature to brash ice, but the size is larger and the shape is normally not spherical. The ridge keel cross-section can be approximated as a triangle or trapezoid. Sliding ice pieces,

Figure 1

c, form when ice sheets are broken up by a ship; these have a similar dimension magnitude to ice ridge rubbles. The movement of these ice pieces is restricted by the existence of the intact ice sheets, forming a layer of ice pieces that covers the underwater ship body. Ice floe fields,

Figure 1

d, are discrete and float on the sea surface. This ice condition is described by its ice concentration, ice diameter, ice thickness, and floe shape.

Figure 1.

Various forms of broken ice: (

) a brash ice channel, (

) an ice ridge, (

) sliding ice pieces under an advancing ship during a model-scale test, and (

) an ice floe field (Figure sources: (

) are from the first author, (

b) is from [1]).

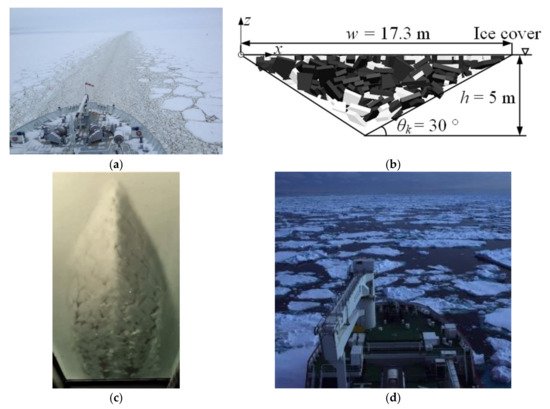
1.2. Interaction between a Ship and Broken Ice

The main desired outcomes of modelling a ship advancing in broken ice include an increased understanding of ice resistance and ice loads on local structures. Ice resistance is the average summation time of the force of the ice along the hull, which, in surge direction, is equivalent to the ice resistance, and in yaw direction, is the resisting turning moment of the ship. These factors relate to ship performance in areas such as attainable speed, fuel consumption, and maneuvrability in ice. Local ice load refers to the maximum force exerted on a certain part of the ship’s structure, usually impacting one or several frames. This relates to the structural safety of ships, especially for low ice-strengthened merchant ships sailing in floe fields. In this paper, the capabilities of existing simulation methods to estimate resistance and the local ice load are discussed, respectively.

1.3. Simulation of a Ship Advancing in Broken Ice

Computational simulation methods offer the opportunity to model the interaction with high fidelity and can potentially provide more accurate results. Due to the discrete nature of broken ice, the Discrete Element Method (DEM) plays a central role in the development of numerical simulation programmes for ships traveling through broken ice. The classical DEM adopts an explicit scheme using rigid blocks and is usually referred to as the Smooth Discrete Element Method (SDEM) [9], as being applied in [1,3,10]. The remaining work relevant to broken ice falls into the category of the non-smooth Discrete Element Method (NDEM), which adopts an implicit, non-smooth or event-driven scheme, e.g., [11,12,13].

Contact modelling, ice breaking, and the effect of fluid flow are the three main issues associated with the computational modelling of ships in broken ice. The contact is usually modelled as elastic [14] or viscoelastic [10], and in some models, there is a plastic limit to mimic ice crushing [12]. Most of the existing models assume broken ice as rigid [10], which is relevant for broken ice of small size such as brash ice, ice ridge rubbles, sliding ice pieces, and small-size ice floes, of which a deformable response to a ship is negligible. However, when the ice floe size is sufficiently large, the floes can be broken up by a ship, for which discrete elements need to be incorporated corresponding to possible failure modes [12]. The DEM and NDEM themselves do not include a fluid solution, i.e., the water surrounding the ship, and the ice requires an additional solution to account for this lack. The fluid effect has been considered using empirical formulae [11,12], including the Arbitrary Lagrangian-Eulerian framework (ALE) [14,15], the Lattice Boltzmann Method (LBM), and Computational Fluid Dynamics (CFD) [3,16].

) is from ^[1]).

1.2. Interaction between a Ship and Broken Ice

During the interaction between a ship and broken ice, kinetic energy is dissipated to push aside, accelerate, submerge, crush, or further break the ice pieces, as well as to compensate for friction. The differences in size and distribution among various types of broken ice result in differences in the energy dissipation process. Given the same thickness and floe shape, the failure of ice by bending and splitting is less dominant when the size of the broken ice is small, but becomes increasingly important for larger ice floes. This affects the dissipation of the ship’s kinetic energy and ultimately determines the interaction force ^[2]. In addition to ice failure, hydrodynamics also plays an important role in the interaction process, e.g., through added mass, drag force, and wake ^[3]. The fluid flow affects the motion of the ship and the ice before and after the contact and influences the force needed to break the ice ^[4]. The main desired outcomes of modelling a ship advancing in broken ice include an increased understanding of ice resistance and ice loads on local structures. Ice resistance is the average summation time of the force of the ice along the hull, which, in surge direction, is equivalent to the ice resistance, and in yaw direction, is the resisting turning moment of the ship. These factors relate to ship performance in areas such as attainable speed, fuel consumption, and maneuvrability in ice. Local ice load refers to the maximum force exerted on a certain part of the ship’s structure, usually impacting one or several frames. This relates to the structural safety of ships, especially for low ice-strengthened merchant ships sailing in floe fields. In this paper, the capabilities of existing simulation methods to estimate resistance and the local ice load are discussed, respectively.

1.3. Simulation of a Ship Advancing in Broken Ice

Unlike formulae used for estimating ship resistance in level ice, analytical formulae proposed for the estimation of broken ice resistance are rare, except those used for sliding ice pieces originating from a breaking ice sheet which have been integrated into the level ice resistance formulae (e.g., submersion resistance as a part of the Lindqvist formula ^[5]). Examples of existing formulae for broken ice includes ^[6] for a brash ice channel, ^[7] for an ice ridge and ^[8] for an ice floe field. While analytical formulae for level ice have been widely compared by research institutes and design companies using model and full-scale tests, the accuracy of these formulae for broken ice is not thoroughly confirmed by measurements. Computational simulation methods offer the opportunity to model the interaction with high fidelity and can potentially provide more accurate results. Due to the discrete nature of broken ice, the Discrete Element Method (DEM) plays a central role in the development of numerical simulation programmes for ships traveling through broken ice. The classical DEM adopts an explicit scheme using rigid blocks and is usually referred to as the Smooth Discrete Element Method (SDEM) ^[9], as being applied in ^[1][3][10]. The remaining work relevant to broken ice falls into the category of the non-smooth Discrete Element Method (NDEM), which adopts an implicit, non-smooth or event-driven scheme, e.g., ^[11][12][13]. Contact modelling, ice breaking, and the effect of fluid flow are the three main issues associated with the computational modelling of ships in broken ice. The contact is usually modelled as elastic ^[14] or viscoelastic ^[10], and in some models, there is a plastic limit to mimic ice crushing ^[12]. Most of the existing models assume broken ice as rigid ^[10], which is relevant for broken ice of small size such as brash ice, ice ridge rubbles, sliding ice pieces, and small-size ice floes, of which a deformable response to a ship is negligible. However, when the ice floe size is sufficiently large, the floes can be broken up by a ship, for which discrete elements need to be incorporated corresponding to possible failure modes ^[12]. The DEM and NDEM themselves do not include a fluid solution, i.e., the water surrounding the ship, and the ice requires an additional solution to account for this lack. The fluid effect has been considered using empirical formulae ^[11][12], including the Arbitrary Lagrangian-Eulerian framework (ALE) ^[14][15], the Lattice Boltzmann Method (LBM), and Computational Fluid Dynamics (CFD) ^[3][16].

2. Computational Simulation Methods

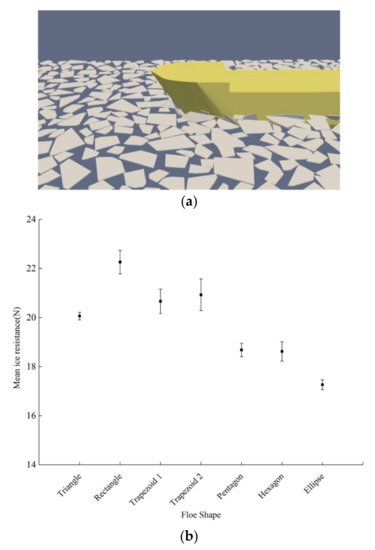
2.1. Ice Floe

The ice floe condition is the most common broken ice condition encountered in polar seas because it can form naturally from water as new-frozen ice, or when large ice pieces break up due to heat or ocean waves. Compared to other types of broken ice, the interaction between a ship and ice floes occupies the largest proportion modelled in existing computational work. The size of ice floe varies between different locations and seasons. The modelling of ship interactions with pancake ice and small ice cakes is relatively straightforward, given that the smaller floes, the less deformable ice behaviour is expected. Particularly, pancake ice has a flat, round appearance [31], which pairs well with the disk-shaped type of the DEM particles, which is compatible with computational speeds. Huang et al. [3,32] presented a coupled CFD+DEM simulation model specifically for pancake ice, as shown in

2.1. Ice Floe

The ice floe condition is the most common broken ice condition encountered in polar seas because it can form naturally from water as new-frozen ice, or when large ice pieces break up due to heat or ocean waves. Compared to other types of broken ice, the interaction between a ship and ice floes occupies the largest proportion modelled in existing computational work. The size of ice floe varies between different locations and seasons. The modelling of ship interactions with pancake ice and small ice cakes is relatively straightforward, given that the smaller floes, the less deformable ice behaviour is expected. Particularly, pancake ice has a flat, round appearance ^[17], which pairs well with the disk-shaped type of the DEM particles, which is compatible with computational speeds. Huang et al. ^[3][18] presented a coupled CFD+DEM simulation model specifically for pancake ice, as shown in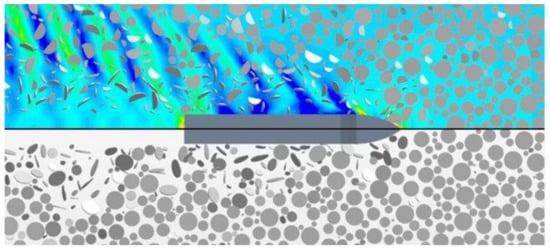

Figure 3, aiming to calculate ice resistance in such ice fields. In this work, each pancake ice floe is represented as one DEM particle, and the surrounding fluid is modelled by CFD to account for the ship’s wake. The work of Huang et al. has achieved good agreement in terms of resistance using model-scale experiments with synthetic ice, and in terms of fuel consumption, using a series of full-scale measurements on the Northern Sea Route [32,33,34]. Less than 10% of deviation was achieved in those comparisons.

2, aiming to calculate ice resistance in such ice fields. In this work, each pancake ice floe is represented as one DEM particle, and the surrounding fluid is modelled by CFD to account for the ship’s wake. The work of Huang et al. has achieved good agreement in terms of resistance using model-scale experiments with synthetic ice, and in terms of fuel consumption, using a series of full-scale measurements on the Northern Sea Route ^[18][19][20]. Less than 10% of deviation was achieved in those comparisons.

Figure 32. The CFD+DEM coupled simulation of a ship in a pancake ice field [3] showing a ship advancing in ice floes with and without the wake effect. It can be seen that the waves can push the floes away from the hull, but when the waves are eliminated, the floes slide closely along the hull and present more contacts.

Most of the other simulation models assume an unbreakable ice floe without specifying the range of the ice floes to which their models apply. Nonetheless, it can be inferred that these models target mainly small ice floes such as pancake ice and ice cakes. Ji et al. [10] presented another model using a 3D circular disk to model ice floe, aiming to estimate ice resistance and local ice loads. Hydrodynamic forces are simplified as drag and added mass. Their simulation shows that ice resistance varies little when floe size changes, which differs from the results of Huang et al. [3]. This might be due to the neglect of fluid flow modelling. No detailed validation was carried out for this model. Kim et al. [35] adopted a simulation setup similar to that of Ji et al., but the simulation was carried out in 2D. Their model was developed for the evaluation of ship maneuverability in floe ice. They also conducted model tests using synthetic ice with up to a 30% concentration to validate the numerical model. Reasonable agreement was shown in terms of speed and turning radius. It is found that the turning radii are smaller in ice than in open water. However, it is unknown whether this model also applies to floe ice of higher concentration.

The shape of the ice floe becomes more irregular in the size range of an ice cake, which brings up the need to model ice floes using shapes other than circular disks. Yang et al. [36] managed to combine multiple common floe shapes in one simulation for the estimation of ice resistance, as shown in

The CFD+DEM coupled simulation of a ship in a pancake ice field ^[3] showing a ship advancing in ice floes with and without the wake effect. It can be seen that the waves can push the floes away from the hull, but when the waves are eliminated, the floes slide closely along the hull and present more contacts.

Most of the other simulation models assume an unbreakable ice floe without specifying the range of the ice floes to which their models apply. Nonetheless, it can be inferred that these models target mainly small ice floes such as pancake ice and ice cakes. Ji et al. ^[10] presented another model using a 3D circular disk to model ice floe, aiming to estimate ice resistance and local ice loads. Hydrodynamic forces are simplified as drag and added mass. Their simulation shows that ice resistance varies little when floe size changes, which differs from the results of Huang et al. ^[3]. This might be due to the neglect of fluid flow modelling. No detailed validation was carried out for this model. Kim et al. ^[21] adopted a simulation setup similar to that of Ji et al., but the simulation was carried out in 2D. Their model was developed for the evaluation of ship maneuverability in floe ice. They also conducted model tests using synthetic ice with up to a 30% concentration to validate the numerical model. Reasonable agreement was shown in terms of speed and turning radius. It is found that the turning radii are smaller in ice than in open water. However, it is unknown whether this model also applies to floe ice of higher concentration. The shape of the ice floe becomes more irregular in the size range of an ice cake, which brings up the need to model ice floes using shapes other than circular disks. Yang et al. ^[22] managed to combine multiple common floe shapes in one simulation for the estimation of ice resistance, as shown in

Figure 4. Their model adopts a physical engine for the simulation of contacts and motions, modelling contact force as an impulse. Restitution coefficients are set separately for ship-ice and ice-ice contact to account for the energy loss during collisions. Their work suggests that one NDEM particle can represent these common shapes, and the shape of the ice floe has a clear influence on the computed resistance. Fluid flow is accounted for via added mass and drag force. The results were well compared in terms of mean resistance with model tests using synthetic ice with ice concentrations of up to 60%.

3. Their model adopts a physical engine for the simulation of contacts and motions, modelling contact force as an impulse. Restitution coefficients are set separately for ship-ice and ice-ice contact to account for the energy loss during collisions. Their work suggests that one NDEM particle can represent these common shapes, and the shape of the ice floe has a clear influence on the computed resistance. Fluid flow is accounted for via added mass and drag force. The results were well compared in terms of mean resistance with model tests using synthetic ice with ice concentrations of up to 60%.

Figure 43. The simulation of a ship in an ice floe field with different shapes and the effect of floe shape on mean ice resistance [36]: (

The simulation of a ship in an ice floe field with different shapes and the effect of floe shape on mean ice resistance ^[22]: (

) simulation screenshot; (

) resistance for different ice shapes.

Table 1 lists the representative models that have been reviewed for replicating a ship in floe ice, in terms of a contact force model, an ice floe shape model, a fluid flow model, and an ice cracking model, including a validation method. The models may contain a wider set of elements that are challenging to condense in one table. The reviewed work using DEM mainly adopted regular floe shapes, i.e., those that can be formulated using mathematical equations. For irregular floe shapes, if the interaction of an abnormal edge is encountered by a ship, it may lack a DEM algorithm to express the contact. It can be observed that only a few models account for local crushing via e.g., a plastic limit [12,42] or constant pressure [11], which is essential for the calculation of the local ice load. The viscoelastic contact model is adopted by most classical DEM models, while an impulse is used in models based on a physical engine. Common shapes to model ice floes include a circular disk, polygon, a square, or a rectangle. Only Huang et al. [3] model the fluid flow extensively using the CFD, while several other models adopt ALE [14,15,43,44] and LBM [47]. The other models simply use drag and added mass. The evaluation of bending and splitting failure is enabled using SAMS, as shown by Jou et al. and Sawamura. Compared to other models, SAMS covers the failure of ice, including crushing, bending and splitting under various scenarios, most comprehensively.

lists the representative models that have been reviewed for replicating a ship in floe ice, in terms of a contact force model, an ice floe shape model, a fluid flow model, and an ice cracking model, including a validation method. The models may contain a wider set of elements that are challenging to condense in one table. The reviewed work using DEM mainly adopted regular floe shapes, i.e., those that can be formulated using mathematical equations. For irregular floe shapes, if the interaction of an abnormal edge is encountered by a ship, it may lack a DEM algorithm to express the contact. It can be observed that only a few models account for local crushing via e.g., a plastic limit ^[12][23] or constant pressure ^[11], which is essential for the calculation of the local ice load. The viscoelastic contact model is adopted by most classical DEM models, while an impulse is used in models based on a physical engine. Common shapes to model ice floes include a circular disk, polygon, a square, or a rectangle. Only Huang et al. ^[3] model the fluid flow extensively using the CFD, while several other models adopt ALE ^{[14][15][24][25]} and LBM ^[26]. The other models simply use drag and added mass. The evaluation of bending and splitting failure is enabled using SAMS, as shown by Jou et al. and Sawamura. Compared to other models, SAMS covers the failure of ice, including crushing, bending and splitting under various scenarios, most comprehensively.

Table 1.

The summary of models of a ship advancing in floe ice fields.

	Contact Model	Floe Shape	Fluid Flow	Cracking	Validation
Huang et al. ^[3]	Viscoelastic	Circular disk	CFD	No	Model test with synthetic ice; full-scale measurement
Ji et al. ^[10]	Viscoelastic	Circular disk	Empirical	No	No
Kim et al. [35]	Kim et al. ^[21]	Viscoelastic	Circular disk	Empirical	No	Model test with synthetic ice
Yang et al. [36]	Yang et al. ^[22]	Impulse	Polygon	Empirical	No	Model test with synthetic ice
DECICE	Viscoelastic	Polygon	Empirical	No	Model test with refrigerated ice
Polojarvi et al. [42]	Polojarvi et al. ^[23]	Elastic-viscous-plastic	Square	Empirical	No	Full-scale measurement
Wang and Derradij-Aouat [43]	Wang and Derradij-Aouat ^[24]	Elastic	Square	ALE	No	Model test with refrigerated ice
Kim et al. [44]	Kim et al. ^[25]	Elastic	Triangle	ALE	No	Model test with refrigerated ice and synthetic ice
Guo et al. ^[14]	Elastic	Square	ALE	No	Model test with synthetic ice
Wang et al. ^[15]	Elastic	Square	ALE	No	Model test with synthetic ice
Kim et al. [45]	Kim et al. ^[27]	Elastic	Polygon	Empirical	No	Model test with refrigerated ice
Guo et al. [46]	Guo et al. ^[28]	Viscoelastic	Square	Empirical	No	Model test with synthetic ice
Janßen et al. [47]	Janßen et al. ^[26]	Impulse	Random	LBM	No	No
GEM	Plastic	Polygon	Empirical	Yes	¹	Full-scale measurement
SAMS	Elastic-viscous-plastic	Polygon	Empirical	Yes	Full-scale measurement
Jou et al. [50]	Jou et al. ^[29]	Viscoelastic	Rectangle	No	Yes	No
Sawamura ^[13]	Impulse	Rectangle	Empirical	Yes	No
Liu et al. [52]	Liu et al. ^[30]	Elastic	Polygon	No	Yes	No

¹ Not yet published.

3.2. Brash Ice

Konno [53] deployed physically based modelling using a physical engine to simulate a ship advancing in a brash ice channel. Ice rubbles are modelled as spherical and cubic particles packed tightly together. The fluid force is accounted for via drag. The study revealed the need to set the correct ice-ice frictional coefficient and distribution of ice piece size.

Mucha [54] presented CFD+DEM modelling of a ship going through a brash ice channel. Ice rubbles are modelled by polyhedral particles. The author tested the sensitivity of resistance on material properties, packing density, degree of coupling, as well as the choice of a reference frame. This work shows the feasibility of CFD+DEM to simulate ship interaction with brash ice, but the results are not compared to physical measurement.

A coupled CFD+DEM approach is adopted by Luo et al. [16]. Brash ice rubbles are modelled using tetrahedral and irregular polyhedral particles, which are composite particles composed of several basic spherical particles. Interaction between fluid and ice particles is modelled using drag and added mass. Good agreement is achieved between the simulation results and model test results in terms of mean resistance. As shown in

Not yet published.

2.2. Brash Ice

Brash ice normally exhibits smaller sizes than pancake ice, making allowing it to be modelled as rigid bodies. Nonetheless, pieces of brash ice rubble lie on top of each other, and therefore must be modelled in 3D. Motions of brash ice rubble are largely affected by wake and bow waves. Thus, it can be important to model hydrodynamics numerically unless ship speed is very low. In addition, cohesion may prevail between ice rubbles due to freezing. Konno ^[31] deployed physically based modelling using a physical engine to simulate a ship advancing in a brash ice channel. Ice rubbles are modelled as spherical and cubic particles packed tightly together. The fluid force is accounted for via drag. The study revealed the need to set the correct ice-ice frictional coefficient and distribution of ice piece size. Mucha ^[32] presented CFD+DEM modelling of a ship going through a brash ice channel. Ice rubbles are modelled by polyhedral particles. The author tested the sensitivity of resistance on material properties, packing density, degree of coupling, as well as the choice of a reference frame. This work shows the feasibility of CFD+DEM to simulate ship interaction with brash ice, but the results are not compared to physical measurement. A coupled CFD+DEM approach is adopted by Luo et al. ^[16]. Brash ice rubbles are modelled using tetrahedral and irregular polyhedral particles, which are composite particles composed of several basic spherical particles. Interaction between fluid and ice particles is modelled using drag and added mass. Good agreement is achieved between the simulation results and model test results in terms of mean resistance. As shown in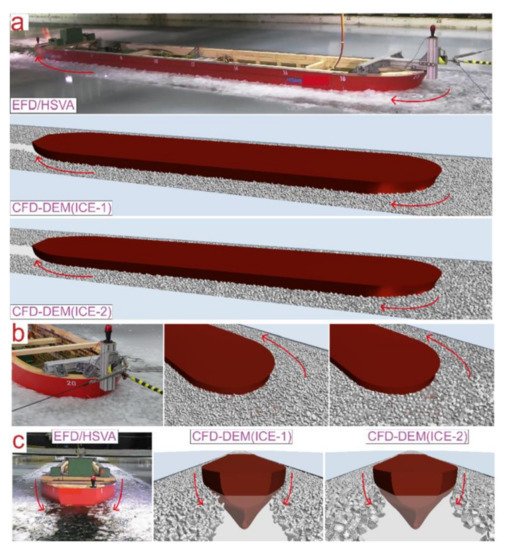

Figure 6, the simulated movement of ice resembles that in the model tests.

4, the simulated movement of ice resembles that in the model tests.

Figure 64. The comparison of brash ice movement results from a model test and from a CFD+DEM coupled simulation [16].

Overall, there are fewer investigations using numerical simulation of ships advancing in brash ice compared to those for ice floes, and the methods are less diverse, mainly being conducted using classical DEM and not accounting for crushing. Existing work has demonstrated the capability of numerical simulation for the qualitative assessment of ice clearing ability, but its validity for quantitative evaluation remains an uncertainty. Additional work is expected to add more insight into issues such as the ice-ice friction, influence of cohesion, effect of fluid flow, role of crushing, and influence of ship speed on ice resistance.

3.3. Ice Ridge

There have been several applications of DEM in ridge keel punch through tests [41,59], and in ridge interaction with offshore structures [60], but studies of a ship going through an ice ridge are rare. The simulation by Gong et al. [1] using the Aalto University in-house DEM code is among the few extensive investigations of ridge resistance via numerical simulation. The scope is limited to an unconsolidated ridge in which ice rubbles are not frozen together. Ridge keels are modelled as triangle and trapezoid shapes, depending on their width and height, as shown in

The comparison of brash ice movement results from a model test and from a CFD+DEM coupled simulation ^[16].

2.3. Ice Ridge

There have been several applications of DEM in ridge keel punch through tests ^[33][34], and in ridge interaction with offshore structures ^[35], but studies of a ship going through an ice ridge are rare. The simulation by Gong et al. ^[1] using the Aalto University in-house DEM code is among the few extensive investigations of ridge resistance via numerical simulation. The scope is limited to an unconsolidated ridge in which ice rubbles are not frozen together. Ridge keels are modelled as triangle and trapezoid shapes, depending on their width and height, as shown in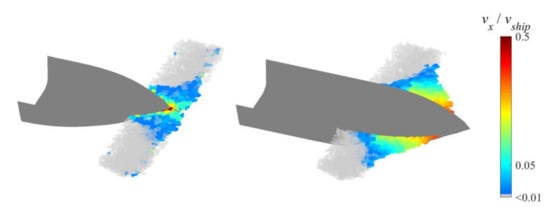

Figure 7. Their work reveals the influence of ridge width on two resistance components, namely friction force and deformation force. The resistance results obtained from the simulation is shown to have a similar magnitude as that predicted by the empirical formula of Malmberg [7], but no validation against actual measurement was performed. In the dissertation by Gong [61], the influence of bow angles on ridge resistance is also investigated. As a conclusion, Gong et al. reported that the unconsolidated ice ridge resistance is proportional to the total ice mass. Another investigation using DEM was conducted by Hisette et al. [62], where the authors compare simulation results with model test results and demonstrate good agreement on resistance. However, the ice-ice friction coefficient has to be selected and the results can be sensitive to this.

5. Their work reveals the influence of ridge width on two resistance components, namely friction force and deformation force. The resistance results obtained from the simulation is shown to have a similar magnitude as that predicted by the empirical formula of Malmberg ^[7], but no validation against actual measurement was performed. In the dissertation by Gong ^[36], the influence of bow angles on ridge resistance is also investigated. As a conclusion, Gong et al. reported that the unconsolidated ice ridge resistance is proportional to the total ice mass. Another investigation using DEM was conducted by Hisette et al. ^[37], where the authors compare simulation results with model test results and demonstrate good agreement on resistance. However, the ice-ice friction coefficient has to be selected and the results can be sensitive to this.

Figure 75. A DEM simulation of a ship penetrating a narrow ice ridge [61].

3.4. Sliding Ice Pieces from Icebreaking

Unlike those for other types of broken ice, the DEM-based simulation has not been applied to simulate a ship’s interaction with sliding ice pieces. Most existing models for a ship going through level ice invests the major effort into the modelling of icebreaking from the level ice sheet, while the subsequent process with broken ice pieces is treated in a simplified way, typically modelled using buoyancy, drag, and added mass, e.g., [49,63].

Towing in presawn ice (see

A DEM simulation of a ship penetrating a narrow ice ridge ^[36].

2.4. Sliding Ice Pieces from Icebreaking

After a ship breaks level ice, broken ice pieces move downwards and sideways, thereby forming a layer of ice pieces covering a certain extent of the hull. This differs from offshore structures, where ice typically accumulates against the structure. Lindqvist ^[5] assumes the covering extent to be 70% as a constant. However, it is reasonable to infer that the actual extent varies between different hull forms. Simulation methods have the potential to distinguish such differences and provide ship-specific solutions. Most of the previous simulation models for level ice breaking involve the simulation of sliding ice pieces, except for those using semi-empirical methods to calculate submersion resistance. Unlike those for other types of broken ice, the DEM-based simulation has not been applied to simulate a ship’s interaction with sliding ice pieces. Most existing models for a ship going through level ice invests the major effort into the modelling of icebreaking from the level ice sheet, while the subsequent process with broken ice pieces is treated in a simplified way, typically modelled using buoyancy, drag, and added mass, e.g., ^[38][39]. Towing in presawn ice (see

Figure 8) is a standard procedure recommended by ITTC [64] for the evaluation of resistance arising from ice pieces that have broken away from the level ice sheet. Konno and Mizuki [65] carried out a dedicated simulation on a ship going through presawn ice using their physically based simulation tool. The simulation reveals some problems with the model in detecting the contact with parallelepiped ice pieces. The comparison with model-scale tests remains qualitative and no validation on resistance has been provided. Another dedicated simulation was presented by Sawamura [66]. The contacts are modelled as an impulse and the fluid is modelled with drag force. Sawamura presents a 2D and a 3D version of a ship traveling in presawn ice and concludes that the model can describe the process qualitatively. Later, the 2D-version is validated using model tests employing synthetic ice [67], which shows an underestimation of resistance due to the neglection of hydrodynamics.

6) is a standard procedure recommended by ITTC ^[40] for the evaluation of resistance arising from ice pieces that have broken away from the level ice sheet. Konno and Mizuki ^[41] carried out a dedicated simulation on a ship going through presawn ice using their physically based simulation tool. The simulation reveals some problems with the model in detecting the contact with parallelepiped ice pieces. The comparison with model-scale tests remains qualitative and no validation on resistance has been provided. Another dedicated simulation was presented by Sawamura ^[42]. The contacts are modelled as an impulse and the fluid is modelled with drag force. Sawamura presents a 2D and a 3D version of a ship traveling in presawn ice and concludes that the model can describe the process qualitatively. Later, the 2D-version is validated using model tests employing synthetic ice ^[43], which shows an underestimation of resistance due to the neglection of hydrodynamics.

Figure 86. A model ship towed through presawn ice at the Aalto ice tank (photo taken by the first author).

5

A model ship towed through presawn ice at the Aalto ice tank (photo taken by the first author).

3. Conclusions

To date, the major computational models created to investigate a ship’s interactions with broken ice have focused on a ship’s interaction with ice floes. There are certain studies on the interaction with brash ice, but ridged ice and sliding ice pieces have received little attention despite their importance in a ships’ ice-going capability. More computational investigations on ridged ice and sliding ice pieces are required to fully understand these processes.

Most models of ship interactions with ice floes are created for resistance estimation, while only a few works have addressed local ice loads. More future research is suggested on the estimation of local loads, which serves as a structural safety evaluation, especially in the context of merchant ships traveling through the Arctic region.

Most models assume ice to be unbreakable, making them suitable for modelling broken ice only up to a certain size. Introducing a cracking mechanism can widen the range of applicability of the existing models. For example, this could be achieved by using clumped DEM particles.

The role of crushing during a ship’s interaction with small-sized broken ice is recommended for investigation in future work. Many models simplify this process by defining it as elastic contacts due to the complexity of modelling, but the effect of small ice-piece crushing on ice resistance estimations has yet to be thoroughly clarified.

The majority of existing models simplify the hydrodynamic force as drag and added mass, which deviates the estimation, especially when ship wakes play a big role in the movement of broken ice. Coupling between DEM and CFD offers good potential for dealing with the factor of broken ice interaction with ships. CFD gives good indications of the wake variation versus the ship speed, which is what the ship-associated flow mainly depends on. With a widespread reduction of the extent, thickness, and compactness of sea ice, hydrodynamics is expected to be increasingly important for studying ship-ice interactions. Therefore, further development and validation of CFD-based methods are particularly recommended.

References

Gong, H.; Polojärvi, A.; Tuhkuri, J. Discrete element simulation of the resistance of a ship in unconsolidated ridges. Cold Reg. Sci. Technol. 2019, 167, 102855.
Sazidy, M. Development of Velocity Dependent Ice Flexural Failure Model and Application to Safe Speed. Ph.D. Thesis, Memorial University of Newfoundland, St. John’s, NL, Canada, 2015.
Huang, L.; Tuhkuri, J.; Igrec, B.; Li, M.; Stagonas, D.; Toffoli, A.; Cardiff, P.; Thomas, G. Ship resistance when operating in floating ice floes: A combined CFD&DEM approach. Mar. Struct. 2020, 74, 102817.
Keijdener, C.; Hendrikse, H.; Metrikine, A. The effect of hydrodynamics on the bending failure of level ice. Cold Reg. Sci. Technol. 2018, 153, 106–119.
Lindqvist, G. A straightforward method for calculation of ice resistance of ships. In Proceedings of the 10th International Conference on Port and Ocean Engineering under Arctic Conditions, Luleå, Sweden, 12–16 June 1989; pp. 722–735.
Riska, K.; Wilhelmson, M.; Englund, K.; Leiviskä, T. Performance of Merchant Vessels in Ice in the Baltic; Research Report No. 52; Ship Laboratory, Helsinki University of Technology: Espoo, Finland, 1997.
Malmberg, S. Omfartygs Fastkling i is (of Ship’s Becoming Beset in Ice). Master’s Thesis, Helsinki University of Technology, Espoo, Finland, 1983.
Aboul-Azm, A. Ship Resistance in Ice Floe Covered Waters; Memorial University of Newfoundland: St. John’s, NL, Canada, 1989.
Tuhkuri, J.; Polojärvi, A. A review of discrete element simulation of ice-structure interaction. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2018, 376, 20170335.
Ji, S.; Li, Z.; Li, C.; Shang, J. Discrete element modeling of ice loads on ship and offshore structures. Acta Oceanol. Sin. 2013, 188, 45–54.
Daley, C.; Alawneh, S.; Peters, D.; Quinton, B.; Colbourne, B. GPU modeling of ship operations in pack ice. In Proceedings of the International Conference and Exhibition on Performance of Ships and Structures in Ice, Banff, AB, Canada, 17–20 September 2012; pp. 122–127.
Lubbad, R.; Løset, S.; Lu, W.; Tsarau, A.; van den Berg, M. Simulator for Arctic Marine Structures (SAMS). In Proceedings of the ASME 2018 37th International Conference on Ocean, Offshore and Arctic Engineering (OMAE2018), Madrid, Spain, 17–22 June 2018.
Sawamura, J. 2D numerical modeling of icebreaker advancing in ice-covered water. Int. J. Nav. Archit. Ocean Eng. 2018, 10, 385–392.
Guo, C.Y.; Zhang, Z.T.; Tian, T.P.; Li, X.Y.; Zhao, G.D. Numerical Simulation on the Resistance Performance of Ice-Going Container Ship Under Brash Ice Conditions. China Ocean Eng. 2018, 32, 546–556.
Wang, C.; Hu, X.; Tian, T.; Guo, C.; Wang, C. Numerical simulation of ice loads on a ship in broken ice fields using an elastic ice model. Int. J. Nav. Archit. Ocean Eng. 2020, 12, 414–427.
Luo, W.; Jiang, D.; Wu, T.; Guo, C.; Wang, C.; Deng, R.; Dai, S. Numerical simulation of an ice-strengthened bulk carrier in brash ice channel. Ocean Eng. 2020, 196, 106830.
Thomson, J.; Ackley, S.; Girard-Ardhuin, F.; Ardhuin, F.; Babanin, A.; Boutin, G.; Brozena, J.; Cheng, S.; Collins, C.; Doble, M.; et al. Overview of the Arctic Sea State and Boundary Layer Physics Program. J. Geophys. Res. Ocean. 2018, 123, 8674–8687.
Huang, L.; Li, Z.; Ryan, C.; Ringsberg, J.W.; Pena, B.; Li, M.; Ding, L.; Thomas, G. Ship resistance when operating in floating ice floes: Derivation, validation, and application of an empirical equation. Mar. Struct. 2021, 79, 103057.
Li, Z.; Ryan, C.; Huang, L.; Ding, L.; Ringsberg, J.W.; Thomas, G. A comparison of two ship performance models against full-scale measurements on a cargo ship on the Northern Sea Route. Ships Offshore Struct. 2021, 16, 237–244.
Ryan, C.; Huang, L.; Li, Z.; Ringsberg, J.W.; Thomas, G. An Arctic ship performance model for sea routes in ice-infested waters. Appl. Ocean Res. 2021, 117, 102950.
Kim, H.; Im, N.; Sawamura, J. Experimental and numerical investigation of ship-ice interactions in pack ice. In Proceedings of the International Conference on Port and Ocean Engineering under Arctic Conditions, Busan, Korea, 11–16 June 2017.
Yang, B.; Sun, Z.; Zhang, G.; Wang, Q.; Zong, Z.; Li, Z. Numerical estimation of ship resistance in broken ice and investigation on the effect of floe geometry. Mar. Struct. 2021, 75, 102867.
Polojärvi, A.; Gong, H.; Tuhkuri, J. Comparison of Full-scale and DEM simulation Data on Ice Loads Due to Floe Fields on a Ship Hull. In Proceedings of the 26th International Conference on Port and Ocean Engineering under Arctic Conditions, Moscow, Russia, 14–18 June 2021.
Wang, J.; Derradji-Aouat, A. Ship Performance in Broken Ice Floes—Preliminary Numerical Simulations; Institute for Ocean Technology, National Research Council: St. John’s, NL, Canada, 2010.
Kim, M.-C.; Lee, S.-K.; Lee, W.-J.; Wang, J. Numerical and experimental investigation of the resistance performance of an icebreaking cargo vessel in pack ice conditions. Int. J. Nav. Archit. Ocean Eng. 2013, 5, 116–131.
Janßen, C.F.; Mierke, D.; Rung, T. On the development of an efficient numerical ice tank for the simulation of fluid-ship-rigid-ice interactions on graphics processing units. Comput. Fluids 2017, 155, 22–32.
Kim, J.H.; Kim, Y.; Kim, H.S.; Jeong, S.Y. Numerical simulation of ice impacts on ship hulls in broken ice fields. Ocean Eng. 2019, 182, 211–221.
Guo, W.; Zhao, Q.S.; Tian, Y.K.; Zhang, C.W. Research on total resistance of ice-going ship for different floe ice distributions based on virtual mass method. Int. J. Nav. Archit. Ocean Eng. 2020, 12, 957–966.
Jou, O.; Celigueta, M.A.; Latorre, S.; Arrufat, F.; Oñate, E. A bonded discrete element method for modeling ship–ice interactions in broken and unbroken sea ice fields. Comput. Part. Mech. 2019, 6, 739–765.
Liu, R.W.; Xue, Y.Z.; Lu, X.K.; Cheng, W.X. Simulation of ship navigation in ice rubble based on peridynamics. Ocean Eng. 2018, 148, 286–298.
Konno, A. Resistance evaluation of ship navigation in brash ice channels with physically based modeling. In Proceedings of the International Conference on Port and Ocean Engineering under Arctic Conditions, Lulea, Sweden, 9–12 June 2009; Volume 2, pp. 855–863.
Mucha, P. Fully-coupled CFD-DEM for simulations of ships advancing through brash ice. In Proceedings of the SNAME Maritime Convention, Tacoma, DC, USA, 30 October–1 November 2019; Volume 1.
Polojärvi, A.; Tuhkuri, J. 3D discrete numerical modelling of ridge keel punch through tests. Cold Reg. Sci. Technol. 2009, 56, 18–29.
Karulin, B.E. Simulation of ridge keel behaviour in direct shear and punch tests by discrete element method. In Proceedings of the 16th IAHR International Symposium on Ice, Dunedin, New Zealand, 2–6 December 2002; Volume 2002, pp. 143–151.
Yulmetov, R.; Bailey, E.; Ralph, F. A discrete element model of ice ridge interaction with a conical structure. In Proceedings of the International Conference on Port and Ocean Engineering under Arctic Conditions (POAC 2017), Busan, Korea, 11–16 June 2017.
Gong, H. Discrete-Element Modelling of Ship Interaction with Unconsolidated Ice Ridges: Ridge Resistance and Failure Behaviour. Ph.D. Thesis, Aalto University, Espoo, Finland, 2021.
Hisette, Q.; Alekseev, A.; Seidel, J. Discrete element simulation of ship breaking through ice ridges. In Proceedings of the International Offshore and Polar Engineering Conference, San Francisco, CA, USA, 25–30 June 2017; pp. 1401–1409.
Lubbad, R.; Løset, S. A numerical model for real-time simulation of ship–ice interaction. Cold Reg. Sci. Technol. 2011, 65, 111–127.
Wang, F.; Zhou, L.; Zou, Z.J.; Song, M.; Wang, Y.; Liu, Y. Study of continuous icebreaking process with cohesive element method. Brodogradnja 2019, 70, 93–114.
Resistance tests in Ice. ITTC Recommended Procedures and Guidelines; The International Towing Tank Conference: Zürich, Switzerland, 2017; Available online: https://www.ittc.info/media/8053/75-02-04-021.pdf (accessed on 26 December 2021).
Konno, A.; Mizuki, T. Numerical Simulation of Pre-sawn Ice Test of Model Icebreaker Using Physically Based Modeling. In Proceedings of the 18th IAHR International Symposium on Ice, Sapporo, Japan, 28 August–1 September 2006; pp. 17–23.
Sawamura, J.; Tachibana, T. Development of a Numerical Simulation for Rotating and Sliding of the Ice Floes Along a Ship Hull. In Proceedings of the Port and Ocean Engineering under Arctic Conditions, Montreal, QC, Canada, 10–14 July 2011.
Sawamura, J.; Kioka, S.; Konno, A. Experimental and numerical investigation on ice submerging for icebreaker with 2D model test using synthetic ice. In Proceedings of the 23rd International Conference on Port and Ocean Engineering under Arctic Conditions (POAC ‘15), Trondheim, Norway, 14–18 June 2015.