Submitted Successfully!
To reward your contribution, here is a gift for you: A free trial for our video production service.
Thank you for your contribution! You can also upload a video entry or images related to this topic.
Version Summary Created by Modification Content Size Created at Operation
1
Szymon Łukaszyk
 -- 470 2024-05-19 18:40:44 |
2 format
Jason Zhu
+ 2 word(s) 472 2024-05-20 09:00:13 |

Video Upload Options

Do you have a full video?
Send video materials Upload full video

Confirm

Are you sure to Delete?
Yes No
Cite
If you have any further questions, please contact Encyclopedia Editorial Office.
Łukaszyk, S. Łukaszyk-Karmowski Metric. Encyclopedia. Available online: https://encyclopedia.pub/entry/56660 (accessed on 30 June 2024).
Łukaszyk S. Łukaszyk-Karmowski Metric. Encyclopedia. Available at: https://encyclopedia.pub/entry/56660. Accessed June 30, 2024.
Łukaszyk, Szymon. "Łukaszyk-Karmowski Metric" Encyclopedia, https://encyclopedia.pub/entry/56660 (accessed June 30, 2024).
Łukaszyk, S. (2024, May 19). Łukaszyk-Karmowski Metric. In Encyclopedia. https://encyclopedia.pub/entry/56660
Łukaszyk, Szymon. "Łukaszyk-Karmowski Metric." Encyclopedia. Web. 19 May, 2024.
Łukaszyk-Karmowski Metric
Edit

The Łukaszyk–Karmowski metric (LK-metric) defines a distance between two random variables or vectors. LK-metric is not a metric as it does not satisfy the identity of indiscernibles axiom of the metric; for the same random variables, its value is greater than zero, providing they are not both degenerated.

distance functions identity of indiscernibles ugly duckling theorem

1. Introduction

LK-metric[1] between two continuous random variables X and Y having a joint probability density function (PDF) F(x,y) is defined as

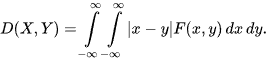
If X and Y are independent, then

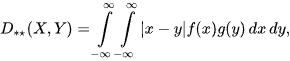
where f(x) and g(y) are PDFs of X and Y, and subscripts denote their types. For example, if X and Y have normal PDFs having the same standard deviation σ but different means μx, μy, then

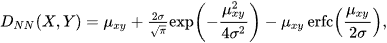
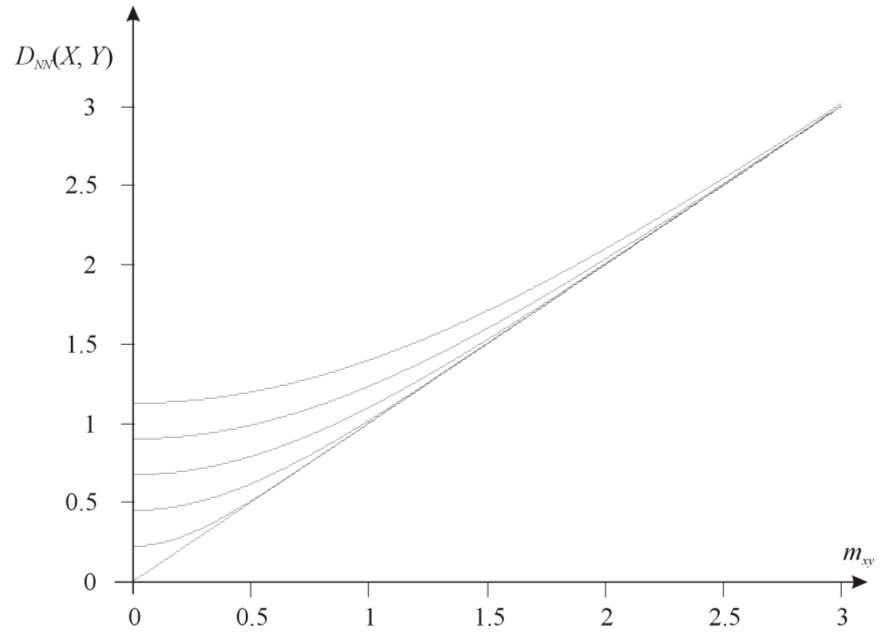
LK-metric between two random variables having normal PDFs and the same standard deviations σ = {0, 0.2, 0.4, 0.6, 0.8, 1}.

where μxy=|μx-μy|. For discrete X and Y, LK-metric has a form

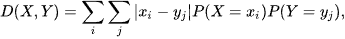
and for random vectors X and Y, LK-metric becomes

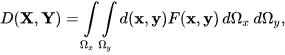
where d(x,y) is a metric function, such as the Euclidean metric. In case, X and Y are mutually and internally independent, a simplified form of LK-metric can also be defined as

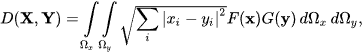
If X and Y are degenerated, almost sure variables having the Dirac delta (or one-point, in the discrete case) PDFs, then LK-metric becomes the metric between their mean values.

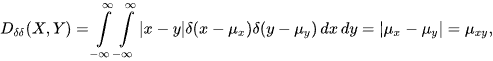
and obviously

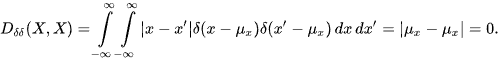
However, in any other case

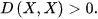
LK-metric satisfies all the remaining axioms of the metric. It is symmetric by definition, and it satisfies the triangle inequality

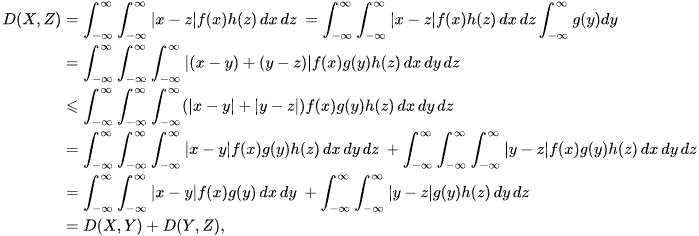
Thus

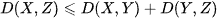
since

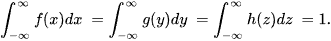
LK-metric is not the only distance function that does not satisfy the identity of indiscernibles axiom[2]. For example, the partial metric[3] also allows each object not necessarily to have zero distance from itself. However, the partial metric satisfies two additional axioms of small self-distances and modified triangle inequality, which are not satisfied by LK-metric[4]. Remarkably, the identity of indiscernibles ontological axiom, introduced to philosophy by Gottfried Wilhelm Leibniz around 1686, is also invalidated by the ugly duckling theorem[5] stated in 1969 and asserting that every two objects one perceives are equally similar (or equally dissimilar). Consequently, the identity of indiscernibles is neither a logical nor empirical principle.

This characteristic non-zero distance effect built in LK-metric allows to avoid ill-conditioning problems in radial basis function interpolation[6][7] and inverse distance weighting[8][9][10][11], where the interpolation accuracy can be improved by choosing the type of distance metric[12][11] and leads to a smooth interpolation function[13]. By preventing zero distances based on parameter uncertainty, LK-metric can, furthermore, be used in analysis of nondeterministic dynamical systems with competing attractors[14]. Since LK-metric represents the mean of distances between all the outcomes of the two uncertain objects, it can also be used in uncertain nearest neighbor classification[15]. The actual value of an uncertain object is modeled by a probability density function[16]. LK-metric has been successfully applied in various fields of science and technology[17][18][19][20][21][22][23][24][13][25][26][27][28][29][30][7][31][32][33][34][35][10][36][37][38][39][40][41][42][43][44][45][11][46][47][48][49][14][50][51].

References

  1. S. Lukaszyk; A new concept of probability metric and its applications in approximation of scattered data sets. Comput. Mech.. 2004, 33, 299-304.
  2. Andrzej Tomski; Szymon Łukaszyk; Reply to "Various issues around the L1-norm distance". Ipi Lett.. 2024, 2, 1-8.
  3. S. G. Matthews; Partial Metric Topology. Ann. New York Acad. Sci.. 1994, 728, 183-197.
  4. Castro, Pablo Samuel and Kastner, Tyler and Panangaden, Prakash and Rowland, Mark. A Kernel Perspective on Behavioural Metrics for Markov Decision Processes. Transactions on Machine Learning Research. 2022, https://openreview.net/forum?id=nHfPXl1ly7, 2835-8856.
  5. Satosi Watanabe; Epistemological Relativity. Ann. Jpn. Assoc. Philos. Sci.. 1986, 7, 1-14.
  6. You-Long Zou; Fa-Long Hu; Can-Can Zhou; Chao-Liu Li; Keh-Jim Dunn; Analysis of radial basis function interpolation approach. Appl. Geophys.. 2013, 10, 397-410.
  7. Abbas M. Abd; Suhad M. Abd; Modelling the strength of lightweight foamed concrete using support vector machine (SVM). Case Stud. Constr. Mater.. 2017, 6, 8-15.
  8. M. Gentile; F. Courbin; G. Meylan; Interpolating point spread function anisotropy. Astron. Astrophys.. 2012, 549, A1.
  9. İ. Bedii Özdemir; A modification to temperature-composition pdf method and its application to the simulation of a transitional bluff-body flame. Comput. Math. Appl.. 2018, 75, 2574-2592.
  10. Christos Anagnostopoulos; Edge-centric inferential modeling & analytics. J. Netw. Comput. Appl.. 2020, 164, 102696.
  11. Zhanglin Li; An enhanced dual IDW method for high-quality geospatial interpolation. Sci. Rep.. 2021, 11, 1-17.
  12. You, Hojun and Kim, Dongsu. Development of an anisotropic spatial interpolation method for velocity in meandering river channel. J. Korea Water Resour. Assoc.. 2017, 50, 1023.
  13. Petar Durdevic; Leif Hansen; Christian Mai; Simon Pedersen; Zhenyu Yang; Cost-Effective ERT Technique for Oil-in-Water Measurement for Offshore Hydrocyclone Installations. IFAC-PapersOnLine. 2015, 48, 147-153.
  14. Kaio C. B. Benedetti; Paulo B. B. Goncalves; Stefano Lenci; Giuseppe Rega; Global analysis of stochastic and parametric uncertainty in nonlinear dynamical systems: adaptative phase-space discretization strategy, with application to Helmholtz oscillator. Nonlinear Dyn.. 2023, 111, 15675-15703.
  15. Fabrizio Angiulli; Fabio Fassetti; Nearest Neighbor-Based Classification of Uncertain Data. ACM Trans. Knowl. Discov. Data. 2013, 7, 1-35.
  16. Angiulli, Fabrizio and Fassetti, Fabio. Indexing Uncertain Data in General Metric Spaces. IEEE Transactions on Knowledge and Data Engineering. 2012, 24, 1640-1657.
  17. James C. Davidson; Seth A. Hutchinson. A Sampling Hyperbelief Optimization Technique for Stochastic Systems; Springer Science and Business Media LLC: Dordrecht, GX, Netherlands, 2009; pp. 217-231.
  18. Gang Meng; Jane Law; Mary E Thompson; Small-scale health-related indicator acquisition using secondary data spatial interpolation. Int. J. Heal. Geogr.. 2010, 9, 50-50.
  19. Yingjie Xia; Xingmin Shi; Li Kuang; Junhua Xuan. Parallel geospatial analysis on windows HPC platform; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, United States, 2010; pp. 210-213.
  20. V. Roshan Joseph; Lulu Kang; Regression-Based Inverse Distance Weighting With Applications to Computer Experiments. Technometrics. 2011, 53, 254-265.
  21. Fengxiang Xu; Guangyong Sun; Guangyao Li; Qing Li; Crashworthiness design of multi-component tailor-welded blank (TWB) structures. Struct. Multidiscip. Optim.. 2013, 48, 653-667.
  22. Chanyoung Jun; Subhrajit Bhattacharya; Robert Ghrist. Pursuit-evasion game for normal distributions; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, United States, 2014; pp. 83-88.
  23. Simon Pedersen; Christian Mai; Leif Hansen; Petar Durdevic; Zhenyu Yang; Online Slug Detection in Multi-phase Transportation Pipelines Using Electrical Tomography∗∗Supported by the Danish National Advanced Technology Foundation through PDPWAC Project (J.nr. 95-2012-3).. IFAC-PapersOnLine. 2015, 48, 159-164.
  24. Branislav Brutovsky; Denis Horvath; Towards inverse modeling of intratumor heterogeneity. Open Phys.. 2015, 13, 103.
  25. S. Mohammed Hosseini; Samira Smaeili; Numerical integration of multi-dimensional highly oscillatory integrals, based on eRPIM. Numer. Algorithms. 2014, 68, 423-442.
  26. Luciana Balsamo; Suparno Mukhopadhyay; Raimondo Betti; A statistical framework with stiffness proportional damage sensitive features for structural health monitoring. Smart Struct. Syst.. 2015, 15, 699-715.
  27. Bing Han; Xinbo Gao; Hui Liu; Ping Wang. Auroral Oval Boundary Modeling Based on Deep Learning Method; Springer Science and Business Media LLC: Dordrecht, GX, Netherlands, 2015; pp. 96-106.
  28. J. Park; V. Sreeja; M. Aquino; C. Cesaroni; L. Spogli; A. Dodson; G. De Franceschi; Performance of ionospheric maps in support of long baseline GNSS kinematic positioning at low latitudes. Radio Sci.. 2016, 51, 429-442.
  29. Grzegorz Lenda; Marcin Ligas; Paulina Lewińska; Anna Szafarczyk; The use of surface interpolation methods for landslides monitoring. KSCE J. Civ. Eng.. 2015, 20, 188-196.
  30. Jan Koloda; Jurgen Seiler; Andre Kaup; Frequency-Selective Mesh-to-Grid Resampling for Image Communication. IEEE Trans. Multimedia. 2017, 19, 1689-1701.
  31. Pijus Kasparaitis; Kipras Kančys; Phoneme vs. Diphone in Unit Selection TTS of Lithuanian. Balt. J. Mod. Comput.. 2018, 6, 162-172.
  32. Jorge Vicent; Jochem Verrelst; Juan Pablo Rivera-Caicedo; Neus Sabater; Jordi Munoz-Mari; Gustau Camps-Valls; Jose Moreno; Emulation as an Accurate Alternative to Interpolation in Sampling Radiative Transfer Codes. IEEE J. Sel. Top. Appl. Earth Obs. Remote. Sens.. 2018, 11, 4918-4931.
  33. Matthew J. Lake; Marek Miller; Ray F. Ganardi; Zheng Liu; Shi-Dong Liang; Tomasz Paterek; Generalised uncertainty relations from superpositions of geometries. Class. Quantum Gravity. 2019, 36, 155012.
  34. Aimilios Sofianopoulos; Mozhgan Rahimi Boldaji; Benjamin Lawler; Sotirios Mamalis; Investigation of thermal stratification in premixed homogeneous charge compression ignition engines: A Large Eddy Simulation study. Int. J. Engine Res.. 2018, 20, 931-944.
  35. D. J. Tan; D. Honnery; A. Kalyan; V. Gryazev; S. A. Karabasov; D. Edgington-Mitchell; Equivalent Shock-Associated Noise Source Reconstruction of Screeching Underexpanded Unheated Round Jets. AIAA J.. 2019, 57, 1200-1214.
  36. Xiaohui Chen; Qing Zhao; Fei Huang; Rongzu Qiu; Yuhong Lin; Lanyi Zhang; Xisheng Hu; Understanding spatial variation in the driving pattern of carbon dioxide emissions from taxi sector in great Eastern China: evidence from an analysis of geographically weighted regression. Clean Technol. Environ. Policy. 2020, 22, 979-991.
  37. Helin Gong; Yingrui Yu; Qing Li; Chaoyu Quan; An inverse-distance-based fitting term for 3D-Var data assimilation in nuclear core simulation. Ann. Nucl. Energy. 2020, 141, 107346.
  38. Liming Xu; Xianhua Zeng; He Zhang; Weisheng Li; Jianbo Lei; Zhiwei Huang; BPGAN: Bidirectional CT-to-MRI prediction using multi-generative multi-adversarial nets with spectral normalization and localization. Neural Networks. 2020, 128, 82-96.
  39. Zhanglin Li; Xialin Zhang; Rui Zhu; Zhiting Zhang; Zhengping Weng; Integrating data-to-data correlation into inverse distance weighting. Comput. Geosci.. 2019, 24, 203-216.
  40. Wei Wei; Zecheng Guo; Liang Zhou; Binbin Xie; Junju Zhou; Assessing environmental interference in northern China using a spatial distance model: From the perspective of geographic detection. Sci. Total. Environ.. 2020, 709, 136170.
  41. Yiyuan Han; Bing Han; Zejun Hu; Xinbo Gao; Lixia Zhang; Huigen Yang; Bin Li; Prediction and variation of the auroral oval boundary based on a deep learning model and space physical parameters. Nonlinear Process. Geophys.. 2020, 27, 11-22.
  42. Yuying Lin; Xisheng Hu; Mingshui Lin; Rongzu Qiu; Jinguo Lin; Baoyin Li; Spatial Paradigms in Road Networks and Their Delimitation of Urban Boundaries Based on KDE. ISPRS Int. J. Geo-Information. 2020, 9, 204.
  43. Aimilios Sofianopoulos; Mozhgan Rahimi Boldaji; Benjamin Lawler; Sotirios Mamalis; John E Dec; Effect of engine size, speed, and dilution method on thermal stratification of premixed homogeneous charge compression–ignition engines: A large eddy simulation study. Int. J. Engine Res.. 2019, 21, 1612-1630.
  44. Panagiotis Pergantas; Nikos E. Papanikolaou; Chrisovalantis Malesios; Andreas Tsatsaris; Marios Kondakis; Iokasti Perganta; Yiannis Tselentis; Nikos Demiris; Towards a Semi-Automatic Early Warning System for Vector-Borne Diseases. Int. J. Environ. Res. Public Heal.. 2021, 18, 1823.
  45. Mobeen Akhtar; Yuanyuan Zhao; Guanglei Gao; An analytical approach for assessment of geographical variation in ecosystem service intensity in Punjab, Pakistan. Environ. Sci. Pollut. Res.. 2021, 28, 38145-38158.
  46. Bogdan-Mihai Negrea; Valeriu Stoilov-Linu; Cristian-Emilian Pop; György Deák; Nicolae Crăciun; Marius Mirodon Făgăraș; Expansion of the Invasive Plant Species Reynoutria japonica Houtt in the Upper Bistrița Mountain River Basin with a Calculus on the Productive Potential of a Mountain Meadow. Sustain.. 2022, 14, 5737.
  47. Fabio Saggese; Vincenzo Lottici; Filippo Giannetti; Rainfall Map from Attenuation Data Fusion of Satellite Broadcast and Commercial Microwave Links. Sensors. 2022, 22, 7019.
  48. Zhiguang Han; Jianzhang Xiao; Yingqi Wei; Spatial Distribution Characteristics of Microbial Mineralization in Saturated Sand Centrifuge Shaking Table Test. Mater.. 2022, 15, 6102.
  49. Oludayo Ayodeji Akintunde; Chidi Vitalis Ozebo; Kayode Festus Oyedele; Groundwater Quality around upstream and downstream area of the Lagos lagoon using GIS and Multispectral Analysis. Sci. Afr.. 2022, 16, e01126.
  50. A. A. El-Atik; Y. Tashkandy; S. Jafari; A. A. Nasef; W. Emam; M. Badr; Mutation of DNA and RNA sequences through the application of topological spaces. AIMS Math.. 2023, 8, 19275-19296.
  51. David Hernández-López; Esteban Ruíz de Oña; Miguel A. Moreno; Diego González-Aguilera; SunMap: Towards Unattended Maintenance of Photovoltaic Plants Using Drone Photogrammetry. Drones. 2023, 7, 129.
More
©Text is available under the terms and conditions of the Creative Commons-Attribution ShareAlike (CC BY-SA) license; additional terms may apply. By using this site, you agree to the Terms and Conditions and Privacy Policy.
Upload a video for this entry
Information
Subjects: Mathematics, Applied
Contributor MDPI registered users' name will be linked to their SciProfiles pages. To register with us, please refer to https://encyclopedia.pub/register :
Szymon Łukaszyk
View Times: 121
Revisions: 2 times (View History)
Update Date: 20 May 2024
Table of Contents
    1000/1000
    Hot Most Recent
    Video Production Service
    Feedback