1. Introduction

Studies of polarization switching in ultrathin (nanoscale) polymer ferroelectric films of polyvinylidene fluoride-trifluoroethylene (P(VDF-TrFE)), obtained experimentally by the Langmuir-Blodgett (LB) method ^{[1][2][3][4][5][6][7]}, have shown that for nanosized (within the film thickness, when their sizes are less than or equal to the critical sizes required for the formation of a domain core), homogeneous non-domain switching of polarization is observed ^[1][6]. This occurs in accordance with the Landau theory of phase transitions ^[8], developed for ferroelectrics by Ginzburg and Devonshire (Ginzburg-Landau-Devonshire (LGD) theory) ^[8][9][10]. In such homogeneous media, the kinetics of the process and the time of polarization switching are well described by the Landau-Khalatnikov equation ^[11][12][13], in the approximation of continuous homogeneous media.

This was shown experimentally ^{[13][14][15][16]} and theoretically (in first-principle calculations ^[17], including with molecular dynamics (MD) approaches ^[18] and using quantum–mechanical semi-empirical methods ^{[17][18][19][20]}). The main condition for the applicability of the LGD theory in such systems is its homogeneity along the polarization switching direction.

It is clear that it is necessary to apply the numerical estimates obtained by the LGD theory with respect to specific and real ferroelectric samples (of any composition and geometry) with extreme caution, and it is necessary to take into account possible limitations and the approximate nature of the results obtained. Nevertheless, under certain conditions and for some structures, the estimates give quite reasonable values consistent with the experimental values, as we will see from the analysis of the results obtained for a number of different systems carried out in this article.

In this paper we do not deal with domain switching and consider only homogeneous switching and its transition to the domain one. But domain switching works well for the sizes when domain nuclei can already form—all these domain switching processes are remarkably described in the Tagantsev et al. monograph ^[21].

Naturally, many issues of domain formation and switching in various real systems remain quite complex and require careful analysis in each specific case. Note, in this case, that the phenomenological LGD theory itself does not answer all the questions and does not consider the mechanisms of polarization switching themselves (especially at the microscopic level). This is a continual theory and it describes the thermodynamics of changes in polarization during a phase transition, which is determined by the potential barrier between the polar and nonpolar phases in the LGD theory. This theory also considers the kinetics of changes in polarization in a homogeneous continuous medium according to the nonlinear Ladnau-Khalatnikov equations—again in the approximation of a homogeneous infinite medium. All this must be taken into account in applications to various real structures.

It is known that the LGD theory does not describe the switching of conventional bulk ferroelectrics, since it predicts the magnitude of the coercive field, which is 2 to 3 times higher than the experimental one. The large coercive fields predicted by the LGD theory came to be called intrinsic (or proper), and their experimental values are extrinsic (or improper).

Domain discovery helped resolve this inconsistency. As it turned out, such bulk ferroelectrics inevitably split into domains ^[21]. This division of the polar crystal into domains reduces the free energy of the crystal. In this case, the minimum of the free energy or thermodynamic potential of the crystal below the Curie point in the polar ferroelectric phase is achieved if the crystal is divided into domains.

The appearance of nanoscale ferroelectrics, namely, polymer ferroelectric films, the thickness of which reached the minimum possible values of one mono-molecular layer (~0.5 nm) ^{[1][2][3][4][5][6][7]}, created a completely new and different situation.

The question of domain formation does not arise, since such layers are much smaller than the size of a possible domain (~10 nm ^[21]), but at the same time all these layers (as one layer and also all layers as a whole, if their formation is created from several layers) created significant spontaneous polarization in their polar phase. In this case, even the values of the coercive fields turned out to be much larger than those in bulk ferroelectrics and they were close to the values of the LGD theory. It became possible to talk about the applicability of the LGD theory here.

Thus, here and further in this article, we will not talk about the formation of domain nuclei and the growth of domains (and their role in polarization switching), but we are only talking about non-domain homogeneous polarization switching, under the conditions of a continuous homogeneous medium, when its size (thickness) does not exceed the dimensions of the formation of domains and one can speak of one continuous medium in the direction of polarization.

2.  Main theoretical approach

2.1. Main relations

Let's take a quick introduction to LGD theory. 

As it was written above, the values of the intrinsic coercive field E_C are determined by the potential barrier between the polar and nonpolar phases as described by the polarization expansion coefficients of thermodynamic potential (or free energy) known from the LGD theory ^[4][5]:

                                                                       (1)

where Ф is free energy, Р is spontaneous polarization, Е is an external field, and the coercive field E_C is expressed as:

                                        (2)

where P₀ = P(T = T₀) = √(-β/γ), χ₀ = χ(T = T₀) = γ/2β², f(t) is the function:

                                                                                       (3)

and the reduced temperature is:

                                                                                (4)

Here, χ₀ is the ferroelectric contribution to the dielectric susceptibility, and α, β, γ are coefficients of the expansion of free energy in even degrees of polarization, known from the LGD theory. For estimates, it can be assumed that approximately E_C ~ P/χ₀ ~ P/εε₀, where ε is the relative permittivity and ε₀ is the dielectric constant of the vacuum.

2.2. Kinetics of polarization switching

The polarization switching kinetics of two-dimensional polymer ferroelectrics was described by Landau – Khalatnikov equation ^[11] and its solution for first-order phase transitions in two-dimenstional ferroelectrics was considered in ^[12][13]:

                                                                                                              (5)

where is the damping coefficient. In the general case, the gradient term can be taken into account. An investigation of the solution of this equation showed that in the vicinity of the coercive field E_C, the switching time sharply increases and its reciprocal can be expressed as ^[12]:

                                                                                                                                               (6)

where Otherwise

                                                                                                                                                   (7)

In this case, E_C is the proper (or intrinsic) coercive field of the ferroelectrics. This relation (7) shows linear behaviour of along E in the vicinity of E_C (for E > E_C).

This relation is more suitable for comparison with experimentally measured data and used in ^[14][15][18]. This relationship turned out to be convenient for analyzing the results of theoretical calculations when modeling the polarization switching processes in polymer ferroelectrics by molecular dynamics (MD) methods ^[18][19].

2.3. Two-dimensional ferroelectrics

The discovery of two-dimensional ferroelectrics ^[1][2] led to a new stage of the polarization switching development using the study of switching of these ultrathin single-crystal films. A start was maid by the development of a new method for growing single-crystal ferroelectric polymer films of polyvinylidene fluoride-trifluoroethylene (P(VDF-TrFE)) ^{[1][2][3][4][5][6][7]}.

Figure 1. Ferroelectric polymer polyvinylidene fluoride (PVDF): (a) and (c) PVDF in polar Trans  conformation and total polarization P > 0; (b) and (d) PVDF in a nonpolar Gauche conformation with total polarization P = 0. Adapted with permission from ref. ^[22], with permission from AIP Publishing, 2012. (e) The formation of the PVDF Langmuir-Blodgett (LB) film on the surface of the water. Adapted with permission from ref. ^[4], with permission from Physics-Uspekhi copyright holder - Uspekhi Fizicheskikh Nauk , 2000. (d) Transferring several mono-layers (ML) of LB PVDF film onto a substrate with an electrode. Adapted with permission from ref. ^[23], with permission from IEEE, 2005. (e) Image of the 1 M LB film of polyvinylidene fluoride-trifluoroethylene P(VDF-TrFE) by scanning tunneling microscopy ^[1][2]. Adapted with permission from ref. ^[2], with permission from Springer Nature, 1998.

To grow the ferroelectric films of this polymer, the Langmuir – Blodgett (LB) method ^{[1][2][3][4][5][6][7][14][15][16][23]} was used, based on the transfer of polymer chains (or mono-layers - ML) from the surface of the water to a substrate that carries an electrode. Figure 1 (a) - (d) show polymer chains and cells in the polar (ferroelectric) and nonpolar (paraelectric) phases (these images were built using HyperChem tool ^[20]); Figure 1 (e) - (g) show a transport scheme of LB layers and 1 ML observed in a scanning tunneling microscope. The Langmuir ferroelectric films obtained by this method in 1998 were thinner than any films obtained before. The thickness of one monolayer (1 ML) was 0.5 nm, that is, significantly less than the size of the critical domain nucleus known from the literature ^[21]. The thickness of Langmuir polymer films (two-dimensional ferroelectrics) was controlled by ellipsometry and atomic force spectroscopy, and two-dimensional ferroelectrics 0.5-1.0 nm thick were first obtained.

Experimental study ^[14][15] and computational simulation ^[17][18][19] of the polarization switching, carried out on this ultra-thin polymer ferroelectrics have confirmed relationships (6), (7). The MD approach calculations ^[18][19] were carried out using HyperChem software ^[20], using various modes and semi-empirical methods (PM3, etc.) for correct quantum-chemical calculations at each step of the MD simulation run process. As an example of the MD run calculations, simple 2 PVDF chains affected by an external electric filed E are presented (Figure 2).

Figure 2. Scheme of the MD run process for 2 PVDF-6 chains model with PM3 (in restricted Hartree-Fock approximation - RHF) calculations at each MD run step: (a) initial state; (b) final state after MD run with dipole moment D orientation turned (switched) in the opposite direction in electric field E; (c) changes of MD averages energies trajectory over time during MD run (in ps) and time of the switching _s for this PVDF system (red – kinetic energy, blue – total energy, green – potential energy). Adapted with permission from ref. ^[18], with permission from Elsevier, 2014.

The final time, for example, for the case of two-chain model rotation (switching time τ_S) was estimated from these MD energy trajectories (see Figure 2 c) using criteria ^[18]: δ = E_K/E_Kmax <10^–3, where E_K is the kinetic energy at the final point and E_Kmax is the kinetic energy at the maximum E_KIN point of the chain rotation (as shown on Figure 2 c). As result, this corresponds to reaching the rest point of the rotating chain and its new position from another opposite orientation of the total dipole Dt vector and polarization vector. With a similar precise we were calculated the values of the coercive field, obtained from a hysteresis loop, corresponding the switching of PVDF chain polarization into the opposite direction (or the same rotation of the PVDF chain). These data were used further in calculations of the thickness dependence of the coercive field (see below on Figure 5).

3. Main results and discussion

3.1. Polymer Ferroelectrics

As a result, this MD approach allows us to determine switching (or rotation) time τ_S (Figure 2c) for any PVDF system as the applied electric field E varies. Using this approach, the switching time τ_S = τ (Figure 3b) was calculated for various polymer ferroelectric models ^[18][19] and the results are compared with experimental data ^[14][15] (Figure 3a).

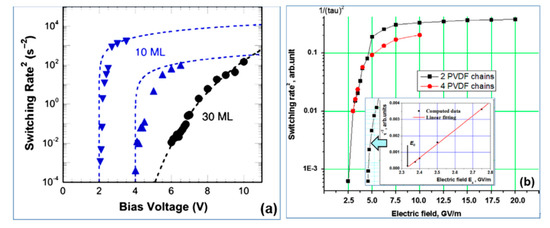
Figure 3. Switching time τs for polymer ferroelectrics presented as τ⁻² along E in a logarithmic scale: (a) experimental data ^[14][15] for thin LB of P(VDF-TrFE) film of 10 ML (5 nm) with critical behavior of τ⁻² for E → E_C in comparison with thick 30 ML (15 nm) films with exponential low (Reproduced with permission from ^[14]; AIP Publishing, 2011); (b) MD simulation run data ^[18][19] for PVDF models showing the critical behavior of τ⁻² with a change in E, which qualitatively coincides with the experimentally observed behavior; the inset shows the linear behavior of τ⁻² as E → E_C and determines the critical value of E_C ~ 2.3 GV/m (Reproduced with permission from ^[18]; Elsevier, 2014).

The critical behavior of τ⁻² for E → E_C for the P(VDF-TrFE) LB films was shown in ^[14][15] (see Figure 3a). Figure 3a presents the data measured for a film of 10 ML (5 nm). Dotted lines show theoretical points corresponding to Equation (7), and triangles show experimental data. For a thicker film of 30 ML (15 nm), the circles indicate the experimental values of τ⁻², and the dotted line indicates the exponential dependence. Figure 3b shows the calculated data using the MD run for τ⁻²(E) behavior in a similar logarithmic scale, which qualitatively coincides with the experimentally established character of the behavior ^[14][15] (Figure 3a). The inset in Figure 3b shows a linear dependence of Equation (7) τ⁻²(E) on E near E_C,, enabling interpolation to the intersection point with the horizontal axis, which determines the value of the coercive field E_C ~ 2.3–2.4 GV/m for 2–4 chain models (ML monolayers).

Taking into account that the field E is external and that for thin polymer layers representing monomolecular layers, the dielectric constant is ε ~ 2.4 ^{[17][24][25][26][27][28]} (while ε = 5 and greater is only for thick films), we obtain the limiting maximum value E_CMAX ~ E_C/ε ≅ 1 GV/m, which is a proper coercive field and corresponds to many known experimental data as well as the LGD theory ^{[1][2][3][4][5][6][7][16][28]}.

A similar study of the P(VDF-TrFE) LB films of different thicknesses was carried out in ^[16] (Figure 4). It was shown that for small thicknesses of 2–6 nm, the coercive field E_C is proper and practically unchanged, and in the region of thicknesses greater than 8 nm, a transition region arises. It was show that for thicknesses greater than 10–12 nm, the proper coercive field E_C becomes improper and is determined by the domain mechanism.

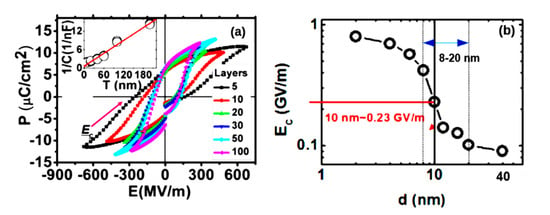
Figure 4. Hysteresis loops of the LB PVDF film ^[16]: (a) with different numbers of monolayers: 5, 10, 20, 30, 50 and 100 (the inset shows the linearity of the reciprocal capacity depending on the thickness); (b) E_C as a function of the LB thickness of the PVDF film (with the transition region at 8–20 nm). Reproduced with permission from ^[16]; AIP Publishing, 2014.

Another method for obtaining a coercive field is by calculation of hysteresis loops P(E) ^[26][27]. Calculations performed for different numbers of chains and film thicknesses using both these methods showed that the dependence of the obtained coercive field ^[18][19][27] is in good agreement with the experimentally established dependence of the coercive field E_C on the film thickness ^[16] (Figure 5).

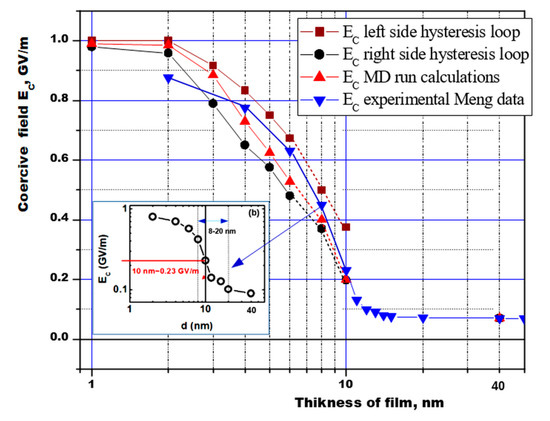
Figure 5. The dependence of the coercive field of the ferroelectric polymer PVDF on the film thickness, according to the results of calculations by different methods (from hysteresis loops and MD runs) in comparison with experimental data ^[16] (inset (b) is from Figure 4), taking into account the dielectric constant of an ultrathin molecular film ε ≈ 2.4. (It is our new original recalculated data, which continues from our preliminary calculations and estimations ^{[18][19][26][27]}).

This dependence can be conditionally divided into 3 regions: the region of purely homogeneous LGD switching (up to 8 nm); the transition region (8–12 nm), where a kind of domain precursor is noted; and the region above 12–16 nm and further, where the domain switching mechanism predominates (the coercive field remains almost unchanged and is kept at a low level of ~0.07–0.05 GV/m) ^{[16][18][19][26][27]}. Thus, these switching calculations of ultrathin polymer ferroelectrics confirm that two-dimensional ferroelectrics can consist, in principle, of several monolayers or several unit cells.

These above data are also in good agreement with the results for thin BaTiO₃ films (see in Figure 6 below), in which homogeneous switching was recently found at the scale level up 10 nm, and with a further increase in thickness they already correspond to thick films ^{[1][2][3][4][29]}.

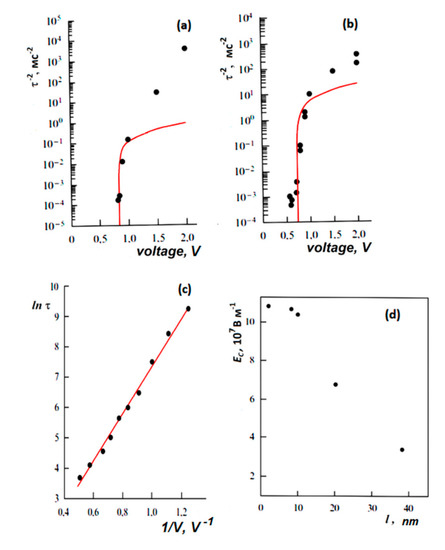
Figure 6. The dependence of the switching time τ on the applied voltage for 8-nm-thick BaTiO₃ film ^[1][3][29]: (a) in the capacitor and (b) in the probe mode in an atomic force microscope (lines τ⁻² (V) on (a) and (b) correspond to the calculations according to formula (7)); (c) for a BaTiO₃ crystal, the line corresponds to ln[τ(V⁻¹)] and is obtained relying on the piezo-response mode in an atomic force microscope; (d) the dependence of the coercive field on the thickness of the BaTiO₃ film. Reproduced with permission from ^[29]; Elsevier, 2013.The switching kinetics in these ultrathin single-crystal BaTiO₃ epitaxial laser films of 2–8 and 40 nm thickness synthesized on a SrRuO₃/SrTiO₃ substrate were studied in a SrRuO₃–ITO capacitor using an atomic force microscope. A detailed description of the technique is presented in ^[30]. The distinction between proper and improper behavior is shown in Figure 5, which shows the dependence of the switching time τ on the applied voltage V for a film of 8 nm thickness in a capacitor (Figure 6a), and under a tip of an atomic force microscope (Figure 6b). For comparison, the same dependence for a bulk BaTiO₃ single crystal of 1 mm thickness is shown in Figure 6c.

The dependence τ⁻²(V) for a barium titanate film in a capacitor and in an atomic force microscope is shown to be consistent with formula (2) (Figure 6a,b). These experimental results agree well with relation (7). On the contrary, the switching kinetics of the bulk crystal (Figure 6c) is nearly exponential; the switching has a domain nature. Figure 6d shows the dependence of the coercive field on the film thickness of barium titanate in the range from 2 to 40 nm. It is seen that in the range from 2 to 10 nm, the coercive field is intrinsic and proper (E_C ~ 0.12 GV/m) and weakly depends on the thickness, in accordance with the LGD theory. For thicknesses of 40 nm (or more), the coercive field sharply decreases, which corresponds to the transition to domain switching. Correspondingly, films with a thickness of 2 and 8 nm exhibit their intrinsic homogeneous LGD switching (5)–(7), and thicker films exhibit a domain exponential dependence. These data are also in good agreement with the above results for LB polymer films ^[16] (Figure 4 and Figure 5).

Thus, the obtained and experimentally observed dependencies of the switching time on the thickness of the barium titanate film (as well as the polymer films mentioned and described above) fully correspond to the Landau-Khalatnikov kinetics, and give the values of the coercive field E_C, which exactly follows from the phenomenological LGD theory.

At the same time, it should be noted that the surface field caused by the surface charge, in principle, affects the coercive field in thin two-dimensional and nanoscale ferroelectric fields ^[31]. However, as it was shown in paper ^[32], the switching kinetics of these two-dimensional and nanoscale films exactly follow the Landau-Khalatnikov kinetics. In addition, this has been verified in experiments ^[14][15][29] and demonstrated in this work for ferroelectric polymer films and perovskite ferroelectric films.

Moreover, it should be reiterated, that it is Landau-Khalatnikov kinetics that give the exact eigenvalue for the values of the coercive field E_C for nanosized ferroelectrics, which follows from the phenomenological LGD theory.

3.3. Nanosized materials based on the Hafnium Oxide

In the past few years, interest has also been wetnessed in nanoscale films based on hafnium oxide ^{[33][34][35][36]}, including those doped with silicon and several types of their solid solutions, such as for example, Hf_0.5Zr_0.5O₂ (HZO) ^[34][35]. Ferroelectricity was found in them and the corresponding hysteresis loops (Figure7) suggest the proper intrinsic nature of the coercive field (~ 0.1 - 0.2 GV/m) here and, apparently, the homogeneous nature of the polarization switching. Though these samples are fully blended HZO solid solutions ^[36], there are some doubts as to whether they are fully homogenous, fully monocrystallic since they can consist of several phases. Therefore, it is too early to draw conclusions about homogeneous switching and its kinetics. But these studies are ongoing.

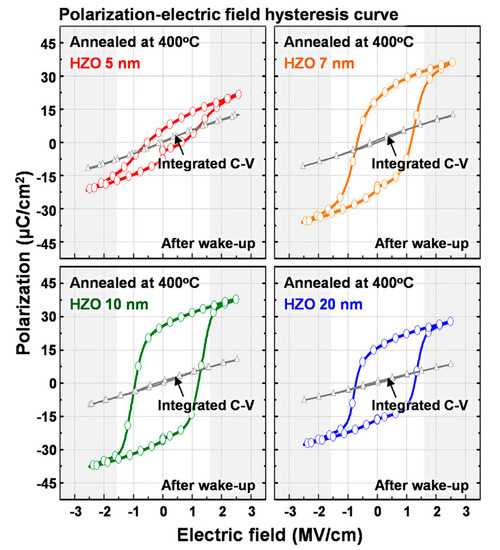
Figure 7. Polarization-electric field hysteresis and integrated capacitance voltage hysteresis curves of 5-, 7-, 10-, and 20-nm-thick HZO-based metal–insulator–metal (MIM) capacitors after wake-up field cycling (Reproduced with permission from ^[35]; AIP Publishing, 2018).