The latter, together with a deficiency in sand supply, may cause a deficit in the sediment budget balance over a period of time in the order of years: in a given area it may cause a long-term regression of the shoreline if the incoming sediment transport rate is lower than the leaving one. The shoreline dynamics must be considered also in view of the current beach erosion that could be exacerbated by future possible climate change scenarios (i.e., sea-level rise). Therefore, without interventions aimed to stabilize the shoreline position, an increase of coastal vulnerability (hence coastal risk) may happen
[2][3]. Even if it is sometimes defined as “acute erosion”
[4], the shoreline retreat is often temporary. It is related to cross-shore transport in the surf zone driven by waves and undertow currents, in a storm-related temporal scale (i.e., the same temporal scale as storm surge occurrence
[5][6]). To counteract coastal erosion, though, the strategies are not as conspicuous as one can think
[7]. One option is abandoning eroded coastal areas and moving landward, letting the natural equilibrium re-build itself. This solution in some cases could be the only option left, even though it is not easily achievable considering the social-economic stresses that affect directly the people
[8] and their risk perception
[9].
On the other hand, it is possible to gain shore protection by emulating the dune system as much as possible, and by nourishing the beach system, adding it back into the littoral system. In general, these methods are referred to as “soft-engineering”, “non-structural” or “quasi-natural”
[17] methods. Hard structures may promote migration of the eroding process to adjacent areas, whilst soft solutions often are long-term unsustainable
[18] due to maintenance issues: the choice and the design of one or another have to be adopted having a deep understanding of the causes of the erosion itself
[7]. Indeed, in the soft-methods group, it has to be distinguished also the “nature-based solutions” (NBS), properly defined as those that mimic the characteristics of the natural habitat. They are created by human design, engineering, and construction to provide specific services such as coastal risk reduction
[19]. NBS, which include for instance salt marsh, oyster reefs, mussel beds, vegetated foreshores, are increasingly being studied and implemented due to their capacity for coastal adaptation and protection and for their capacity to maintain the ecosystem healthy and resilient
[20][21][22]. Many NBS designs require a holistic consideration of different fields, ranging from biology, chemistry, and engineering then their implementation is still predominantly case-specific
[23][24]. On the other hand, beach nourishments as a well-established non-structural method are often viewed as a valid option. Indeed, they provide a series of benefits, ranging from the restoration buffer to extreme storms, to the increase of recreational attractiveness
[25], to the avoided lack of sand on the adjacent beaches. Their temporary nature (i.e., they do not solve the cause of erosion itself
[26], rather supply for a local sand deficiency) can be enhanced by the “hybrid” or “mixed” deployment of solutions
[2], whose main goal is to retain the added sand by increasing the lifetime of nourishment projects. The environmental sustainability of beach nourishment can be maintained by the adoption of low-impact solutions, both structural (i.e., low-crested submerged breakwaters) and non-structural. Among the “soft” ones, it is possible to name the artificial manipulation of beach groundwater
[27]. It has assumed several names, such as “beach dewatering”, “beach management system” (patented by
[28]), “Pressure Equalizing Modules” (PEM, patented by
[29]), or generally “beach dewatering system”. Hereinafter it is referred to as “Beach Drainage System” (BDS). It relies on the idea that groundwater level in the non-cohesive sandy porous medium, influenced by the seaward oscillations due to the waves (short or long), can, in turn, influence the sediment particle mobilization in the swash zone, according to the difference of the free surface elevation inside and outside the sand. Hence, the system consists of a series of drains placed in the beach, vertically (PEM) or horizontally (BDS) in the inter-tidal zone, that are expected to lower the groundwater table in order to achieve a “drier” beach, that in turn discourages the fluidization of the particles.
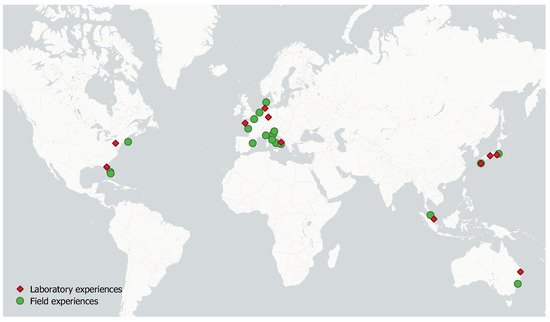
So far, the efficiency of BDS is not fully recognized, even though its history dates back for decades and it has been tested in several places in the world, both in the laboratory and in the field (Figure 1).
2. Laboratory Experience
The first study ever on the effects of a drained beach is represented by the laboratory campaign carried out in 1975 by Machemehl
[38]. He conducted a laboratory experiment on a draining system deployed perpendicularly to the shoreline and artificially pumped. A first good response of the dewatering for depositing sand at the beach face, with stabilizing behavior at the offshore zone, but with negligible effect in the breaking zone was obtained.
Kawata et al.
[39], in 1987, tested a system of horizontal pipes and pumps under controlled conditions in the laboratory (solitary and monochromatic incident waves). Different sand and foreshore initial profile have been adopted: 0.35 mm mean diameter sand and 1:10 slope for solitary tests; a coarser porous medium (0.94 mm), and milder slope (1:30) for regular waves. For solitary waves tests a steeper foreshore, a deeper breaker scour, a higher foreshore berm, and a higher step inshore were observed for the drained configuration if compared to the undrained one. For monochromatic waves tests, the drainage of the beach was observed to accelerate accretion of the foreshore through the development of a berm under mild wave conditions. Stormy conditions were also reproduced; the variation of the beach profile was less accentuated when the system was activated with respect to the case with no draining system, indicating the stabilizing action.
In 1991, the studies carried out by Ogden, Sidel and Weisman
[40][41] basically differed in considering the presence of tidal oscillations. The former
[40] focused on the influence of the position of the drain, the latter
[41] on the influence of tides. The experiments were carried out in a wave flume where irregular waves were generated. The gravity drainage consisted of a 2.54 cm diameter pipe, buried in the sand with a mean diameter of 0.35 mm, scaled according to the fall parameter
[7]. They did not find significant effects on the rate of erosion or accretion when the beach drain is used for the condition of negligible tides. On the contrary, they found some accretion on the upper part of the shoreface when tides were considered. In the end, they did not recommend the use of this tool where the tide is negligible, and at least to use a pumping system to enhance the infiltration rate.
In the meanwhile, Sato et al.
[42] in 1995 performed three-dimensional and two-dimensional laboratory tests on beach dewatering by pumps on fine sand (d
50 = 0.29 mm) to investigate the function, performance, and optimum location for installing a coastal drain system. They confirmed that a coastal drain system enhances the accretion of sediments on the beach face for accretive wave conditions and retards beach erosion for erosive wave conditions. Moreover, the greater the discharge drained by the system, the greater the efficiency. It is due to the larger amount of suspended sediments that migrate shoreward. Their new finding highlights the efficiency of the BDS also in stormy conditions. The breaking bar tends to migrate shoreward if the flow induced by the artificial drainage overcomes the wave-induced offshore component. Furthermore, they found that the best position for the drain is seaward the shoreline.
Other supportive feedback on the reliability of the drainage system was given by Oh and Dean
[43][44]. They performed laboratory experiments on two different scales. The influence of drainage was investigated for three different groundwater levels: the mean seawater level (m.s.l.), a level higher than the seawater level (i.e., saturated condition), and a level lower than the seawater level (i.e., drained condition). The tests showed the saturated groundwater condition has a stabilizing influence on the dynamics of sand along the cross-shore direction. This finding was not consistent with previous studies for which higher water table level was observed to enhance erosion. The sand bar swiped up the shore-face, with deposition of sand landward with respect to the equilibrium position. The lowered water table showed less effect on the mobilization of the particles. The small scale experiments have been performed to check the repeatability of the tests carried out at a larger scale, having scaled down the sand characteristics by the fall velocity parameter and the waves by the Froude criterion. Though, the small-scale experiments did not match with the larger scale ones, likely due to poorly-sorted sand. In the end, they stressed the need for additional controlled laboratory experiments.
The laboratory studies of
[45][46][47][48][49] at the end of 1990s’ followed indeed. In detail, Weisman et al.
[46] performed tests with and without both drainage and tidal cycle for accretive and mildly erosive conditions. They highlighted the scale effects that laboratory models dramatically suffered from, recognizing the greater efficiency of the system at prototype scale rather than laboratory tests one. Moreover, they found that the drain is effective for both the tested waves conditions. Hence, they suggested prototype installations working continuously during a year, at least except under highly erosive sea states and during flood tides when the water table is below the mean water level. At the International Conference of Coastal Engineering in 1996, some works on drainage systems were presented, hinting at the great interest that the community had been showing at that time about the relatively new perspectives that BDS would have opened up. Kanazawa et al.
[47], conversely to the classical configuration of the BDS, used a permeable layer buried in a fine sand (median diameter of 0.135 mm) in the same way as
[32]. In this study, the used sand was characterized in the laboratory and a permeability coefficient of 6.28 × 10 m/s was estimated. Both two-dimensional and three-dimensional experiments were conducted, with different aims. The 2D tests have been carried out to compare the performances on sand stability of three different types of drain layers. The three-dimensional investigation aimed to confirm the 2D results and to furnish qualitatively the extent of the stabilizing effect of the draining layers. In 2D tests, a gravel layer 10 cm wide, a gravel layer coupled with the presence of a draining pipe connecting the gravel layer to the seaward shoreline, and a spring coil mattress connected to the sea by a draining pipe were deployed as three different configurations of the drainage layer. The latter configuration was the one tested also in the wave basin (i.e., in the 3D investigation), but with more permeable sand. For this reason, it was not possible to evaluate the three-dimensional effects that a 2D experiment neglects, which are indeed fully tested in 3D case. From these studies, by three-dimensional modeling, it was possible to estimate, besides the lower set-up and groundwater table rising with respect to the 2D domain, that the shoreline changes experienced with the spring coil mattress permeable layer correspond to a reduction of wave height of about 40%.
Lemckert
[48] investigated the efficiency of the drain in a particular condition but still very common: swash zone, worth remind mainly influenced by infragravity waves, is interested in a three-dimensional wave field, resulting from the interaction of regular and edge waves. The porosity of the beach was observed to be more effective than the drainage on modifying the energy of edge waves, so it is realized that high porosity beaches are more effective in reducing the reflected wave field with respect to drained beaches.
Law et al.
[49] studied the evolution of transient beach profiles with and without artificial drainage. The results showed that the drain pipe placed beneath the center of the swash zone promotes initial accretion of the beach. The presence of the drain, as expected, induced a steeper upper beach slope. As the tests go on, the influence of the drain reduced. They even concluded that its effect can be in any case positive since it retards the erosion for the first hours. In prototype-scale terms, it may correspond, in some field conditions to one or two days, i.e., the period that the beach needs the most protection in storm conditions.
Gampathi and Chan
[50], adopting a similar asset as
[32] by using a draining layer, tested the influence of groundwater table on beach profile changes considering accretive and erosive wave climates, different levels of the water table, with and without different assets of the draining layer. It resulted that for increasing water table elevation the deposition of sand at the foreshore decreased when accretive waves were reproduced. On the other hand, it increased for erosive conditions. From the drained test, it has been deducted that the faster the drainage the better the performance. The drainage rate depends, among others, on the position of the drain.
Date back to 2011 the largest laboratory investigations ever carried out, undertaken at Gross Wellen Kannal (GWK) in Hannover (Germany) at prototype-scale. They investigated both the hydrodynamic
[51][52][53][54] and the morphodynamic aspects
[36][55].
The facilities size let investigate the BDS with no scale effects, that others have already stressed, but still under controlled conditions. The flume is about 300 m long, 5 m wide and 7 m deep. The sand consisted of a 0.33 mm mean diameter sand. The draining system was arranged as two corrugated pipes of 0.20 m diameter. Tests included static conditions (i.e., with no wave propagating in the channel) and dynamic ones with 3 different wave conditions. Static tests aimed to study the system efficiency in lowering the water table without the influence of the waves, in order to define the time in which the system reaches the steady-state. Dynamic tests were performed in order to evaluate the response of the system under different wave conditions. In particular, three different wave energy conditions (High, Medium, and Low energy) were tested out employing a JONSWAP spectrum
[56].
In terms of beach profile evolution, from
[36] they concluded that under high energy wave conditions the drainage system seemed to be inadequate in giving any stabilizing effect. For medium energy and low energy tests, the beach showed global stabilization and an increased accretion, respectively. The scarce performance of the system observed in the laboratory under different energy of incident waves proves to be dependent on the characteristics of the sand and the drains, as well as their position, and it marks a threshold for an asset of beach drainage that would be effective.
In the end, the authors suggested combining BDS with other coastal defenses, for instance, submerged breakwater to partially dissipate high waves. Indeed, Saponieri et al.
[18] tested three configurations: unprotected beach, only BDS protected, and BDS-submerged breakwater coupling protected, the latter with the aim to switch the sea state from high to medium and low energy states. It resulted that drains mainly worked under pressure conditions. The authors suggested enhancing the infiltration capability, for instance, promoting the drainage with a more permeable layer around the pipe. Besides BDS effects on both hydrodynamics and morphodynamics, the joint configuration of BDS and submerged sill highlighted interesting outcomes. The drainage seems to be capable to mitigate also the raising of the mean water level typical of the sheltered region shoreward the structure.
3. Field Campaigns
The pioneering work of Chappel et al.
[57] posed the base for groundwater manipulation in the field. In their two pilot studies along the Australian coast, they used a series of wells and pumps to regulate the water table, showing very positive results in beach aggradation after the survey campaign. Furthermore, for the first time, they suggested the installation of horizontal drains.
During the first 1980s, Danish Geotechnical Institute (DGI) installed a water filtration system at Hirtshals, pumping water from buried drain tubes along (and close to) the shoreline. Curtis and Davis
[58] reported that during the 8 months of the survey campaign the shoreline propagated seaward, even in the winter season. Though, in this period, the system did not manage to prevent erosion for severe storms. Nonetheless, the positive results boosted further studies and field campaigns. Later, since the positive results of the first experience, a seven years (1985–1991) campaign was led in Danmark by DGI at Thorsminde
[31][59][60] resulting in a total accretion of the beach of 30 m/m (i.e., per unit meter along the beach) over 7 years, while the neighborhood beaches experienced 25 m/m of erosion in the same period. The system was patented in 1987
[28] with the name of Beach Management System
® (BMS). Under that license, another installation was done at Sailfish Port (Florida) by CSI (Coastal Stabilization Inc.) with the American name of STABEACH
®. The system worked for at least 7 years, with some alternating performances. After about 2 years the installation, Dean
[61] independently reported that the beach resulted to be in local moderate accretion with a generalized more stable shoreline with respect to the control transects. Instead, different behaviors were exhibited by the northern and southern control sections during the following years. The system however was stopped during the years 1991–1992–1993 in the summer months due to the sea turtles’ nesting period, so it was not possible to study its capabilities on recovering, other than on preventing erosion. Moreover, an accretionary event was recorded even when the system was off. Turner and Leatherman
[27] concluded that it was difficult to discern any net positive effect, above all because the system was installed in the transition zone among two sections that experienced different trends of erosion and accretion. In the 1990s this method was investigated through both field and laboratory campaigns. During that period further investigations about the interaction between seepage and swash motion were performed (e.g.,
[62][63][64]), enhancing the knowledge about their correlation and their function on the sediment particles mobility within the swash zone. The commercial installation of STEABEACH
® was deployed at Nantucket beach, Florida. The project experienced a series of maintenance problems. The pumps did not work as expected, due to sand buildup within the pipes
[65]. Even after an increase in the pump size, the dewatering system achieved little success, and consequently, it has been removed by the local government, never reaching its potentiality due to maintenance issues. However, during the operative period of the system, Curtis et al.
[66] reported that it had no bad consequences on the local environment, vegetative communities, and public freshwater aquifer.
A field test was carried out by Davis et al.
[67] at Dee Why Beach, Australia. The drainage in this experiment was not pumped: they installed the type of drain commonly used for roads, laid vertically. The whole apparatus was surveyed for 18 months, stressing that it did not stop working, even though, due to some damages, the system reduced its efficiency. They stressed, in addition, that gravity drainage is a highly cost-effective means of beach stabilization, prone to extending the life of beach nourishment programs.
Katoh and Yanagishima
[32] used an alternative way to drain the sand, by means of a geotextile layer. The field survey was led, after laboratory tests
[47], at Kashima (Japan) in an already stable beach. It resulted that in the drained beach the speed of foreshore erosion in a storm slowed down and, in addition, the eroded foreshore is recovered quickly after a storm.
Another field campaign followed in the next years at Kashiwabaru Beach
[68], where a 2 years survey was carried out. The drains were laid parallel to the foreshore. From the comparison between the drained part and undrained part of the beach, it appeared that profile changes did not show any definite differences, although a small difference was found in the seaward area of the pipes. Analyzing that small difference, the amount of sand gained by the drain system was estimated as being roughly 5 m
3for 20 m of beach width.
The commercial installations of BMS or ECOPLAGE had been installed in the following years in different sites, i.e., France
[69][70][71], Italy
[35][72][73][74], Spain
[75]. French ECOPLAGE received good feedback from the site-installation local surveys. Unfortunately, international publications that confirmed the results did not follow, except for some documents (also in the French language) that are redacted by the constructors. The Spanish experience confirmed that beach drainage stops shoreline retreat and enhances quick recovery from storm erosion events. Regarding the systems installed in Italy, inconclusive results had been gained. In Alassio, both Ciavola et al.
[35] and Bowman et al.
[72] recognized the main role of the system in stabilizing the beach under normal to medium energy conditions, while under high energy conditions positive shoreline accretion trends faded. Again, Ciavola et al.
[35] reported the case of Procida (the beach did not benefit from the system and it was early abandoned) and of Bibione (where the survey campaign was interrupted). At Metaponto, as well, the monitoring did not continue as long as it should have to gain certain results. The same issue was for the Ostia site. Vicinanza et al.
[74], reporting in detail the case of Procida Island, expressed their not particularly positive opinion about such a system.
A commercial alternative version to the generic BDS is represented by PEM (Pressure Equalizing Modules), patented by
[29] and commercialized by EcoShore Inc. In this case, the essence of the tool does not change, except for the position whereby they are installed: they consist of 2 m long permeable tubes deployed vertically into the beach. They, as the name suggested, are placed in rows forming a matrix along the shoreline. They are vented at the top in order to make the pressure to be equalized. Jakobsen and Brøgger
[76][77] reported the three-years survey of the installation at Skodbjerge (Denmark), funded by the Danish Ministry of Transport. The functioning period ranged between January 2005 and 2008. At the end of that period, the analysis showed favorable performances. Actually, the report lacks deep analysis and information, above all about the average climate as well as recorded extreme events. The coast of Skodbjerge, historically in erosion, seemed to show a rising level of the beach in the area in front of the PEM, with an accumulation rate greater than 50% with respect to the reference areas (the neighbor check areas where the system was not installed), coming from long-shore sediment transport current. The same system has been deployed also in Malaysia
[78], and in Florida
[79]. In Malaysia, PEM combined with beach nourishment was installed in a pocket beach, most of it exposed to the main direction of the northeast monsoon. After three years it resulted to be stabilized, even with no natural sediment input: the losses are estimated as 25% of the total amount nourished at the beginning of the survey campaign. The critically eroding Hillsboro Beach (Florida), even with a delay with respect to the expectations, probably due to a reduced depth of the sand than usual, showed an undoubtedly accretive behavior (8.2 m in three years of the campaign; from 2008 to 2011). The survey dealt also with the influence of the system on the local fauna: no negative impacts were recorded.
The latest two field campaigns, independently documented, are
[80][81], with two opposite interpretations of the results. The first one describes 4 years of survey in the Netherlands of a passive vertical drainage system. It resulted that no measurable influence on the beach and dune volumes variations were recorded. In
[81], on the other end, after five years of “classical” BDS survey on a macro-tidal beach in France, it seemed that the beach drainage allows faster recovery of the upper part of the shoreface after a storm.
4. Mathematical modeling
The water table is defined as the surface where the pore pressure is atmospheric. Above the free surface, a layer of unsaturated medium is present: the pores between the solid grains are not full of water, but it results to be a three-phase area: liquid (water), solid (grains), and gas (air). The mathematical modeling of the groundwater motion in an unconfined aquifer (i.e., a flow whose upper boundary is represented by a water table) follows the classical approach of the continuum medium for irrotational flow. The simplest approach for the unconfined unsteady flow (since the presence of the time-dependent forcing) is the one-dimensional linearized Boussinesq equation [82], i.e. the combination of Darcy’s law and the mass conservation equation, considering the Dupuit’s assumption [83], which assumes equipotential lines as vertical and streamlines as horizontal and hence a hydrostatic pressure distribution.
Even if the first approach on modeling the groundwater dynamics at the coast is analytical
[84][85][86][87][88][89], only Fischione et al.
[90], according to the best authors knowledge, engaged to analytically model a beach drained by BDS. They solved the classical Boussinesq equation
[91] in an idealized finite-length rectangular domain with a simplified boundary condition to take into account the presence of the drain: a constant groundwater level has been imposed at the shoreward boundary, at a certain distance from the shoreline (i.e. the seaward boundary), where the effect of the waves has been modeled as a periodic function of the water table.
On the other hand, groundwater dynamics has been widely investigated by means of numerical modeling. Dominick et al.
[92] were among the first ones that tried to numerically predict water table fluctuation due to waves by an implicit finite-difference solution. The groundwater response to tide-induced oscillations has been taken into account in their studies by
[93][94][95]. The “BeachWin” model
[96] links beach groundwater and swash, simulating interacting wave motion, beach groundwater flow, and sediment transport in the swash zone. The model uses simplified descriptions of the various processes and does not consider either the effect of vertical flow in porous media on the sediment immersed weight or the increase and decrease of the boundary layer. The model has been validated by Ang et al.
[97] for controlled boundary conditions against the experimental results, with no satisfactory results. The numerical work of Li et al.
[98] is worth to be mentioned when the numerical modeling of dewatering systems is concerned. They included the drainage effects for the macro-tidal beach, modifying the model presented in
[99]. Two different types of dewatering systems were investigated: artificial and gravity drained beaches. In the first place, the original model by
[99] included a modified kinematic boundary condition for the water table, which takes into account the capillarity effects as well. The new kinematic boundary condition was incorporated into a boundary element method model. Karambas and Ioannidis
[100] modified the numerical model proposed by Karambas
[101] in order to take into account the effect of the draining system. They coupled the Boussinesq type model for the propagation of the waves in the near field with a porous flow model capable to account for sediment transport. Vesterby et al.
[69] coupled the commercial model powered by Danish Hydraulic Institute MIKE SHE (for groundwater dynamics) and MIKE 11 (for sea-level fluctuations and wave run-ups) and applied them to the real case of Les Sables d’Olonne
[71]. Saponieri and Damiani
[102][103] solved Richard’s equation by means of HYDRUS-2D code
[104] to simulate the GWK prototype-scale experiments
[52]. They tested the model for the static case (i.e., with no waves) in order to check the capability of the model to catch the draining capabilities of the BDS, and eventually use it to optimize the position of the drain according to its best performances. Against the groundwater-coastal hydrodynamic coupling, the approach used by
[105][106] resorts to the power of the Computational Fluid Dynamics to model a fine porous medium in presence of wave-forced groundwater table, in presence of a drained beach. The OpenFOAM
® library has been used to solve the Volume-Averaged Reynolds Averaged Navier–Stokes equation
[107]. In
[105], different drain diameters have been compared in terms of groundwater table and discharge, while
[106] modeled three-dimensional small-scale numerical tests for different draining patterns and sand permeability. The dynamic inside the pipe has been studied and analyzed, to gain insight into its hydraulic regime and eventually to define appropriate configurations to prevent the pipe from pressurizing.