Laser wakefield electron acceleration (LWFA) is an emerging technology for the next generation of electron accelerators. As intense laser technology has rapidly developed, LWFA has overcome its limitations and has proven its possibilities to facilitate compact high-energy electron beams.
1. Introduction
Laser wakefield acceleration (LWFA) has attracted much attention since it was proposed in 1979 by T. Tajima and J. Dawson
[1] due to its possibility to provide a huge acceleration field for electron acceleration. Thus, LWFA can realize table-top high-energy electron accelerators and be the next generation of electron accelerators with extremely high energy over 100 GeV. However, the proposal was pending for a long time because it required too high a performance for the high-power lasers at the time. As the chirped pulse amplification (CPA) technology
[2] initiated the rapid progress of high-power lasers in the 1990s, the short-pulse high-power lasers led to the realization of laser electron accelerations
[3][4][5], albeit the quality of the electron beam was not good enough for applications. In 2004, a milestone was laid in LWFA research: a mono-energetic collimated electron beam was achieved in the bubble regime by using an intense femtosecond laser
[6][7][8]. Since then, LWFA has been intensively investigated with high-power femtosecond lasers to provide high-quality electron beams and radiation sources
[9] for practical applications to non-destructive inspections, ultrafast x-ray spectroscopy, and x-ray microscopy.
Even though LWFA can provide a huge acceleration field, some scientific and technological problems need to be solved for practical applications. First of all, LWFA uses complex nonlinear dynamics of plasma media
[10], and its acceleration structure has the dimensions of tens of microns in space and hundreds of femtoseconds in time. Secondly, an intense laser pulse is modified significantly during the propagation through the plasma medium, and the modification of the laser pulse alters the plasma medium and acceleration process as a feedback loop. In addition, the electron injection into the plasma wave spontaneously happens in the plasma, which is called self-injection. Therefore, the whole acceleration process is highly nonlinear and unstable, limiting the electron energy
[11][12], beam quality and stability
[13][14]. Many studies are ongoing to solve these problems to improve the performance of LWFA.
The advancement of high-power laser technology is essential for the enhancement of LWFA performance. Enormous efforts have been exerted to increase the laser’s peak power and they consequently succeeded in building petawatt (PW) lasers
[15]. The development of PW lasers provided a chance to explore a new regime of laser particle accelerations and relativistic laser–plasma interactions. In the last decade, several types of PW lasers have been used in LWFA experiments. A PW laser was demonstrated in Texas university, Austin, by adapting the CPA to an Nd:Glass laser
[16]. This hybrid laser had a very low repetition rate, below one shot/hour. The most successful demonstration of PW lasers was based on Ti:Sapphire CPA lasers. These lasers can provide laser energy over 30 J, pulse duration of about 30 fs, and a repetition rate of over 0.1 shot/second
[17]. Ti:Sapphire lasers with peak power over PW have been commercialized and installed in several research institutes for relativistic laser–plasma science. As the laser power increases, the achievable electron energy by LWFA has increased by more than an order of magnitude, compared to the first demonstration of the bubble-regime LWFA in 2004. Recently, multi-GeV electron beams were obtained with a centimeter-long medium
[11][18][19]; the conventional radio-frequency (RF) acceleration technology requires a few hundred meters for such beams. In addition, many applications of LWFA or radiation sources from LWFA have been demonstrated in the last decade. Therefore, LWFA has the potential for compact linear accelerators and x-ray sources as the next generation of electron accelerators.
2. LWFA with Texas PW Laser
The PW laser at the University of Texas at Austin was developed by implementing the hybrid OPCPA scheme with Nd:glass laser amplifiers and had a pulse duration of 140 fs and pulse energy of 140 J
[16]. The laser has been used for various laser–plasma experiments such as electron acceleration, ion acceleration, and neutron generation. Especially, the electron energy of 2 GeV was successfully demonstrated
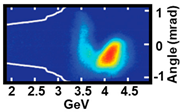
[11]. The experiment was performed by focusing the PW laser pulses with a spherical mirror having an f-number of 47 onto a 7-cm-long helium gas cell. The accelerated electron beam was dispersed by a 6.7-cm-long dipole magnet having a field strength of 1.1 T. Fiducial arrays made of tungsten wires were inserted between the magnet and the detection screens to measure the electron energy correctly. The beam cross-section at the focus was not optimal: the intensity profile was asymmetric and had several spots.
The experimental results showed that the PW laser pulses produced electron beams with energy over 2 GeV, as shown in Table 1. According to the energy formula (1), the electron energy of 2 GeV is expected for the PW laser’s power and a plasma density of 5 × 1017 electrons/cm−3. The electron energy was lower at lower plasma densities, which is opposite to the prediction from (1). This behavior can be attributed to the poor focal spot. A low-quality focal spot with internal structures may be beneficial to induce self-injection in such a low-density plasma but deteriorates the laser propagation and electron acceleration. It was pointed out that the spatial shaping of the PW laser pulse is necessary to enhance the electron energy.
Table 1. Experimental results for three different laser shots from the Texas PW laser
[11]. Reproduced with permission from [Xiaoming Wang], [
Nat. Commun]; published by [macmillan Publishers Limited], [2013].
3. Dual-Stage LWFA with PW Laser at UQBF
In this section, we review the dual-stage LWFA with the PW lasers at the Ultrashort Quantum Beam Facility (UQBF), Advanced Photonics Research Institute (APRI), GIST. The APRI group successfully constructed two PW beamlines in 2012 by using the CPA scheme and Ti:Sapphire amplification media. The first beamline produced energy of 30 J and a pulse duration of 30 fs
[17], and the second beamline did 45 J
[20] and the same pulse duration. The PW laser at UQBF was used for LWFA experiments to produce multi-GeV electron beams. The achievable electron energy can be increased by increasing the laser power and lowering plasma density. However, the self-injection of electron bunches into plasma waves can be prohibited by reducing the plasma density. Thus, the plasma medium density and profile should be carefully designed to maximize the electron energy: the acceleration length should be maximized while keeping self-injection occurring. One solution can be to combine gas media of different lengths and densities called dual-stage or cascaded acceleration.
The dual-stage LWFA experiments were performed by focusing the PW laser onto a dual gas jet medium consisting of 4-mm and 10-mm helium gas jets
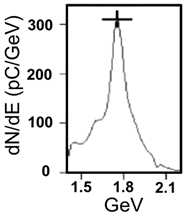
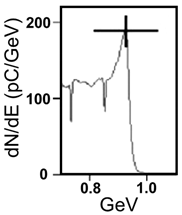
[18]. The first 4-mm helium jet acted as an injector stage, and the second 10-mm jet boosted electron energy. Laser pulses with an energy of 25 J were focused with a 4-m long concave spherical mirror, as shown in Figure 1a. The wavefront aberration of the laser pulse was corrected using a deformable mirror installed before the compressor. The laser pulse was stretched to 60 fs with a positive chirp by detuning the compressor grating.
Figure 1. (
a) Experimental layout for dual-stage LWFA with a PW laser pulse, (
b) schematic drawing of the dual-stage target, and (
c) the experimental result with the dual-stage target
[18]. In figure (
c), the red line shows the electron spectrum from the first target, and the black line shows the spectrum after the second target.
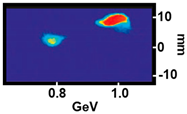
In the dual-stage LWFA, several issues should be properly treated to obtain a high-energy electron beam. Firstly, a self-injection should happen in the first medium, and the resulting electron bunch should have sufficient energy when entering the plasma wave in the second medium. Secondly, the electron beam and driving laser pulses should be properly coupled into the second medium. Thirdly, the electron beam should be accelerated in the second medium without an additional electron injection. The first 4-mm helium gas jet was optimized to obtain a 400 MeV electron beam by tuning the gas pressure to have an electron density of 2 × 1018 electrons/cm3. No electron beam signal was observed when the second jet was used alone with densities below 1 × 1018 electrons/cm3. A significant enhancement of electron energy was observed with the dual gas jet target having electron densities of 2 × 1018 electrons/cm3 for the 4-mm jet and 0.8 × 1018 electrons/cm3 for the 10-mm jet. The gap between the two jets was about 2 mm, and the laser focus was at the middle of the gap. At this condition, the electron bunch from the first jet could be successfully coupled to the second target because the low plasma density in the second jet enlarged the plasma wavelength to have a higher chance to catch the electron bunch from the first target. In addition, the second target having a lower density than the self-injection threshold can prohibit continuous self-injection that can reduce the acceleration field strength. When the plasma densities of the two jets were independently controlled, the electron energy was over 3 GeV. At this condition, the electron beam has a charge of about 10 pC over 2 GeV energy, energy spread about 50%, and beam divergence of about 4 mrad.
The dual-stage acceleration can be a simple solution to obtain a high-energy electron beam. Recently, a dual-stage acceleration with two driving laser pulses was demonstrated by using capillary discharge media
[21]. Even though we can expect an energy gain at each stage in a staged acceleration, precise control of each stage for stability is challenging. In the dual gas jet target, the turbulence between the targets can also make the electron beam unstable. Thus, the method to handle the qualities of the accelerated electron beam should be investigated by manipulating the driving laser pulse and plasma medium. In addition, the electron energy with dual-stage LWFA was still much less than the 10 GeV that is expected for PW lasers because the laser propagation is limited to be an order of 1 cm. For increasing the electron energy further, an external guiding structure for PW laser pulses should be applied to keep the laser intensity over 10 cm.
In the dual-stage acceleration, the PW laser pulse was stretched to be positively chirped with a duration of 60 fs. It was stretched to control the acceleration gradient of LWFA by manipulating the pulse’s spectral phase
[22]; such a control method was demonstrated experimentally at UQBF
[13][23]. In particular, positive group-delay dispersion (GDD) enhanced the energy and charge of the electron beam, and third-order dispersion (TOD) improved the energy further and the stability of the beams. The combination of a dual-stage target and careful control of laser pulse properties can be an effective way to shape the electron beams and control the acceleration process.
4. LWFA with Capillary Discharge Plasmas at LBNL
Laser propagation through a plasma medium is a highly complicated process. To increase the electron energy for a given laser power, the elongation of laser propagation is a critical issue in LWFA research. Most experiments were performed with self-guiding schemes that provide a much longer propagation length than the Rayleigh range by balancing relativistic self-focusing and diffraction. For increasing the electron energy, the plasma density should be lower, and the medium length should be longer. However, the elongation of laser-propagation length by relativistic self-guiding is getting more difficult as plasma density is lowered because the critical power for self-guiding increases as the plasma density decreases. Thus, the elongation of the laser propagation through the plasma medium is an essential technique to increase electron energy with PW laser pulses. One of the solutions is guiding the laser pulse with a plasma channel. The plasma channel guiding utilizes a refractive index gradient in the transverse direction like optical fibers. It can contain high-intensity laser pulses in the relativistic regime due to the extremely high-intensity limit of the plasma medium. Several groups developed plasma channel technology
[24][25][26], and the research group at Lawrence Berkley National Laboratory successfully applied the plasma channel to enhance the electron energy
[16][22][23].
The first successful application of a plasma channel to LWFA demonstrated 1 GeV electron acceleration by focusing a 40 TW laser pulse to a 3.3 cm plasma channel
[27]. The plasma channel was formed in a pre-ionized hydrogen plasma confined in a capillary tube. The capillary tube was fabricated on a sapphire block by laser machining: two gas inlets and a central tube with a few-hundred-microns diameter. The hydrogen gas was fully ionized by a high-voltage electric pulse applied to the electrodes at both ends of the capillary tube. The pre-formed plasma had a hyperbolic electron density profile in the transverse direction that can guide an intense laser pulse. The capillary discharge medium has an obvious advantage of elongated laser propagation through the plasma medium.
Table 2 summarizes the successful demonstrations of electron energy enhancement with capillary discharge plasma channels and sub-PW
[19] and PW lasers
[12].
Table 2. Characteristics of electron beams from capillary discharge plasma channel and experimental condition for three different experiments with laser power of 40 TW
[27], 300 TW
[19], and 850 TW
[12]. Reproduced with permission from [Leemans, W.P], [
Nat. Phys]; published by [Nature Publishing Group], [2006].
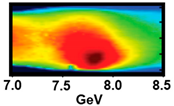
The enhancement of electron energy with the laser power is not straightforward, even though the plasma channel can guide the driving laser pulse to a long distance. Since 1-GeV electron beam was produced with a laser power below 100-TW, as seen in experiment 1 in Table 2, a 1-PW laser pulse should have the capability to generate a 10-GeV electron beam. Despite the use of sub-PW laser pulses, a 4.2-GeV electron beam was produced with a 9 cm capillary discharge plasma channel because of the nonlinear evolution of laser pulses with a top-hat profile. The nonlinear propagation in the plasma can assist the self-injection process but disturb smooth laser propagation in the plasma channel. For increasing electron energy in LWFA further, a longer laser propagation through a plasma with a lower plasma density is essential. However, the nonlinear laser propagation can prohibit the increase of electron energy by limiting long and smooth laser propagation through a plasma medium with an extremely low electron density below 5 × 1017 electrons/cm3. Although experiment 2 in Table 2 was performed with an almost perfectly focused laser beam of Strehl ratio of about 0.8, the top-hat laser profile in the near field, ordinarily formed by the laser amplification, induced a nonlinear laser propagation and hindered the additional increase of electron energy.
The nonlinear laser propagation problem has been mitigated by steepening the transversal electron density gradient of the plasma channel. A nanosecond laser, focused on the axis of the discharge capillary plasma channel, heated through inverse bremsstrahlung the core of the capillary discharge plasma channel and created a deeper electron density valley that could guide a PW laser pulse efficiently. Thus, the effect of the nonlinear laser propagation could be mitigated, and the laser pulse could propagate tens of centimeters in a plasma channel with an electron density of 3 × 1017 electrons/cm3. This improvement of laser propagation by a steepened plasma channel made it possible to generate the most energetic electron beam of about 8 GeV from LWFA (experiment 3 in Table 2). This result implies that engineering the plasma medium is a key to realizing the maximum electron energy expected by the power-scaling of the electron energy. Consequently, more and more efforts should be exerted to control the plasma medium, not only to increase laser power but also to find suitable electron acceleration conditions for high-energy electron beams by LWFA.