In mathematics, Weierstrass's elliptic functions are elliptic functions that take a particularly simple form. They are named for Karl Weierstrass. This class of functions are also referred to as p-functions and they are usually denoted by the symbol ℘. They play an important role in theory of elliptic functions. A ℘-function together with its derivative can be used to parameterize elliptic curves and they generate the field of elliptic functions with respect to a given period lattice.
- elliptic functions
- elliptic curves
- ℘-function
1. Definition
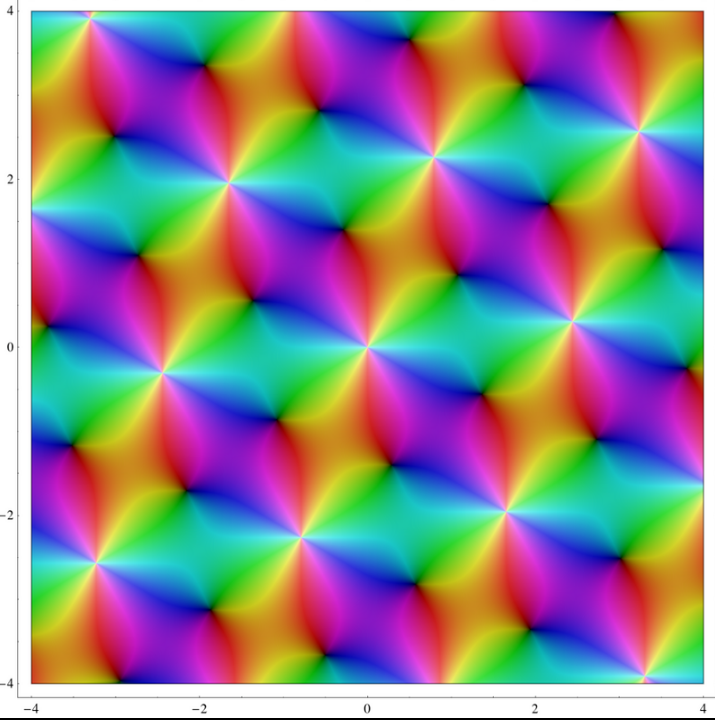
Let
This series converges locally uniformly absolutely in
The Weierstrass
Because the sum
It is common to use
2. Motivation
A cubic of the form
For the quadric
Because of the periodicity of the sine and cosine
In a similar way one can get a parameterization of
There is another analogy to the trigonometric functions. Consider the integral function
It can be simplified by substituting
That means
Elliptic functions are also inverse functions of integral functions, namely of elliptic integrals. In particular the
Let
Then
3. Properties
- ℘ is an even function. That means
The second last equality holds because
- ℘ is meromorphic and its derivative is[6]
It follows that
4. Laurent Expansion
Let
where
5. Differential Equation
Set
This relation can be verified by forming a linear combination of powers of
6. Invariants
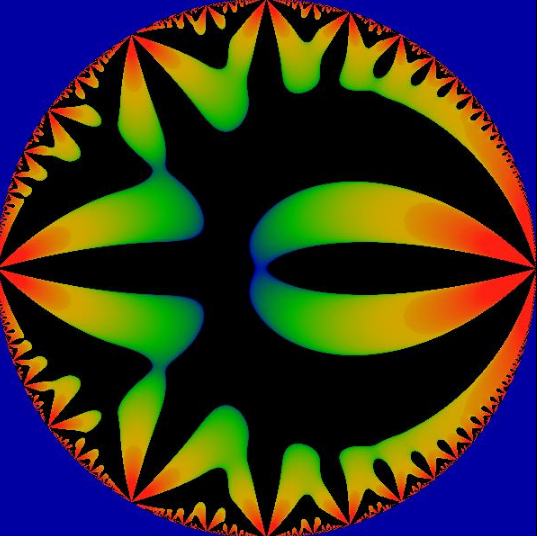
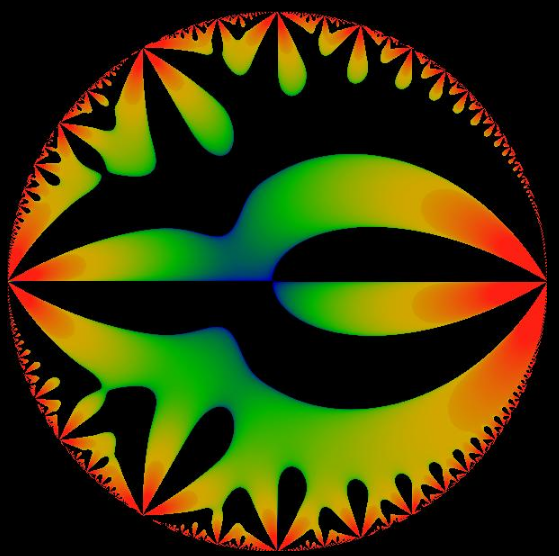
The coefficients of the above differential equation g2 and g3 are known as the invariants. Because they depent on the lattice
The series expansion suggests that g2 and g3 are homogeneous functions of degree −4 and −6. That is[7]
If
Let
That means g2 and g3 are only scaled by doing this. Set
As functions of
The Fourier series for
where
7. Modular Discriminant
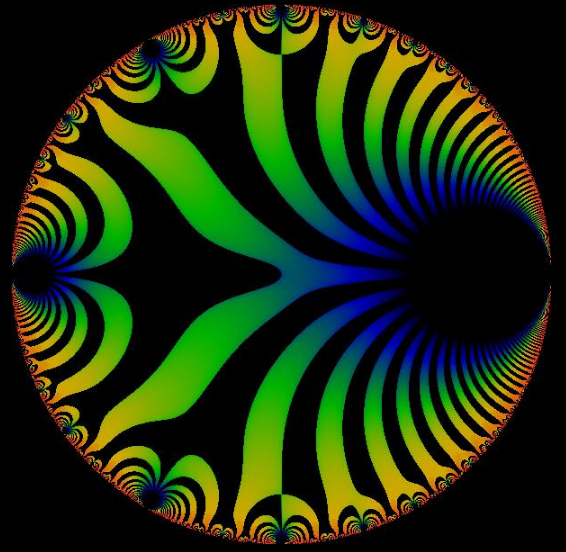
The modular discriminant Δ is defined as the discriminant of the polynomial at right-hand side of the above differential equation:
The discriminant is a modular form of weight 12. That is, under the action of the modular group, it transforms as
where
Note that
For the Fourier coefficients of
8. The Constants e1, e2 and e3
They are pairwise distinct and only depend on the lattice
Because those roots are distinct the discriminant
That means the half-periods are zeros of
The invariants
9. Relation to Elliptic Curves
Consider the projective cubic curve
For this cubic, also called Weierstrass cubic, there exists no rational parameterization, if
Now the map
It can be shown that every Weierstrass cubic is given in such a way. That is to say that for every pair
The statement that elliptic curves over
10. Addition Theorems
Let
As well as the duplication formula:[17]
-
These formulas also have a geometric interpretation, if one looks at the elliptic curve
The group structure of
The sum of three pairwise different points
This is equivalent to:
where
11. Relation to Jacobi's Elliptic Functions
For numerical work, it is often convenient to calculate the Weierstrass elliptic function in terms of Jacobi's elliptic functions.
The basic relations are:[20]
where
and their argument w equals
12. Typography
The Weierstrass's elliptic function is usually written with a rather special, lower case script letter ℘.[21]
In computing, the letter ℘ is available as \wp in TeX. In Unicode the code point is U+2118 ℘ SCRIPT CAPITAL P (HTML ℘ · ℘), with the more correct alias weierstrass elliptic function.[22] In HTML, it can be escaped as ℘.
| Preview | Template:Charmap/showcharTemplate:Charmap/showcharTemplate:Charmap/showcharTemplate:Charmap/showchar | |
|---|---|---|
| Unicode name | SCRIPT CAPITAL P / WEIERSTRASS ELLIPTIC FUNCTION | |
| Encodings | decimal | hex |
| Unicode | 8472 0 0 0 | U+2118 |
| UTF-8 | 226 132 152 0 0 0 | E2 84 98 00 00 00 |
| Numeric character reference | ℘ |
℘ |
| Named character reference | ℘ | |
The content is sourced from: https://handwiki.org/wiki/Weierstrass%27s_elliptic_functions
References
- Apostol, Tom M. (1976). Modular functions and Dirichlet series in number theory. New York: Springer-Verlag. pp. 9. ISBN 0-387-90185-X. OCLC 2121639. https://www.worldcat.org/oclc/2121639.
- Hulek, Klaus. (2012) (in German), Elementare Algebraische Geometrie : Grundlegende Begriffe und Techniken mit zahlreichen Beispielen und Anwendungen (2., überarb. u. erw. Aufl. 2012 ed.), Wiesbaden: Vieweg+Teubner Verlag, p. 8, ISBN 978-3-8348-2348-9
- Rolf Busam (2006) (in German), Funktionentheorie 1 (4., korr. und erw. Aufl ed.), Berlin: Springer, p. 259, ISBN 978-3-540-32058-6
- Jeremy Gray (2015) (in German), Real and the complex : a history of analysis in the 19th century, Cham, p. 71, ISBN 978-3-319-23715-2
- Rolf Busam (2006) (in German), Funktionentheorie 1 (4., korr. und erw. Aufl ed.), Berlin: Springer, p. 294, ISBN 978-3-540-32058-6
- Apostol, Tom M. (1976) (in German), Modular functions and Dirichlet series in number theory, New York: Springer-Verlag, p. 11, ISBN 0-387-90185-X
- Apostol, Tom M. (1976). Modular functions and Dirichlet series in number theory. New York: Springer-Verlag. pp. 14. ISBN 0-387-90185-X. OCLC 2121639. https://www.worldcat.org/oclc/2121639.
- Apostol, Tom M. (1976) (in German), Modular functions and Dirichlet series in number theory, New York: Springer-Verlag, p. 14, ISBN 0-387-90185-X
- Apostol, Tom M. (1990). Modular functions and Dirichlet series in number theory (2nd ed.). New York: Springer-Verlag. pp. 20. ISBN 0-387-97127-0. OCLC 20262861. https://www.worldcat.org/oclc/20262861.
- Apostol, Tom M. (1976). Modular functions and Dirichlet series in number theory. New York: Springer-Verlag. pp. 50. ISBN 0-387-90185-X. OCLC 2121639. https://www.worldcat.org/oclc/2121639.
- Chandrasekharan, K. (Komaravolu), 1920- (1985). Elliptic functions. Berlin: Springer-Verlag. pp. 122. ISBN 0-387-15295-4. OCLC 12053023. https://www.worldcat.org/oclc/12053023.
- Rolf Busam (2006) (in German), Funktionentheorie 1 (4., korr. und erw. Aufl ed.), Berlin: Springer, p. 270, ISBN 978-3-540-32058-6
- Tom M. Apostol (1976) (in German), Modular functions and Dirichlet series in number theory, New York: Springer-Verlag, p. 13, ISBN 0-387-90185-X
- K. Chandrasekharan (1985) (in German), Elliptic functions, Berlin: Springer-Verlag, p. 33, ISBN 0-387-15295-4
- Hulek, Klaus. (2012) (in German), Elementare Algebraische Geometrie : Grundlegende Begriffe und Techniken mit zahlreichen Beispielen und Anwendungen (2., überarb. u. erw. Aufl. 2012 ed.), Wiesbaden: Vieweg+Teubner Verlag, p. 12, ISBN 978-3-8348-2348-9
- Hulek, Klaus. (2012) (in German), Elementare Algebraische Geometrie : Grundlegende Begriffe und Techniken mit zahlreichen Beispielen und Anwendungen (2., überarb. u. erw. Aufl. 2012 ed.), Wiesbaden: Vieweg+Teubner Verlag, p. 111, ISBN 978-3-8348-2348-9
- Rolf Busam (2006) (in German), Funktionentheorie 1 (4., korr. und erw. Aufl ed.), Berlin: Springer, p. 286, ISBN 978-3-540-32058-6
- Rolf Busam (2006) (in German), Funktionentheorie 1 (4., korr. und erw. Aufl ed.), Berlin: Springer, p. 287, ISBN 978-3-540-32058-6
- Rolf Busam (2006) (in German), Funktionentheorie 1 (4., korr. und erw. Aufl ed.), Berlin: Springer, p. 288, ISBN 978-3-540-32058-6
- Korn GA, Korn TM (1961). Mathematical Handbook for Scientists and Engineers. New York: McGraw–Hill. pp. 721.
- This symbol was used already at least in 1890. The first edition of A Course of Modern Analysis by E. T. Whittaker in 1902 also used it.[21]
- The Unicode Consortium has acknowledged two problems with the letter's name: the letter is in fact lowercase, and it is not a "script" class letter, like U+1D4C5 𝓅 MATHEMATICAL SCRIPT SMALL P, but the letter for Weierstrass's elliptic function. Unicode added the alias as a correction.[22][23]
