The Isolated Micro-Grid Composition

The Isolated Micro-Grid Composition: History

Please note this is an old version of this entry, which may differ significantly from the current revision.

Subjects: Engineering, Electrical & Electronic

Contributor:

The isolated micro-grid system can be composed of a variety of energy sources such as the Photovoltaic generator, the wind system, a methanation system, possibly even a hydraulic generator in such farm places. All these energy sources must be connected to serve the load part and when there is excessive production of electrical energy, an electrical storage system must appear.

energy management
micro-grid

1. Photovoltaic Generator

A photovoltaic generator is a set of solar panels that convert light energy (sunlight) into electrical energy that varies depending on the influence of light and temperature, using solar cells that are connected in series and/or parallel to each other to obtain desired characteristics such as power, voltage, and current. As shown in the diagram, the equivalent circuit of a solar photovoltaic (PV) has numerous types [18,19,20]. It is determined by the number of diodes connected in series. One parallel diode, shunt resistance, series resistance, a current source, and a diode are all included in the basic version. For a single diode model, the modeling step begins with expressing the output current as shown in Equation (1).as it is in [21]:

I_{P V} = I_{P h} - I_{D} - I_{P}

(1)

The current of a single cell is calculated by integrating the temperature and irradiation factors:

I_{P h} = [I_{s c} + K_{i} . (T - T_{n})] . \frac{G}{G_{n}}

(2)

Equation (3) presents the current in the path of the diode:

I_{D} = I_{0} (e^{\frac{(V_{o c})}{a . V_{t}}} - 1)

(3)

The thermal voltage of a diode is given as in Equation (4).

V_{t} = \frac{k . T . n_{s}}{q}

(4)

The diode saturation current

I_{0}

depends on the temperature of the panel.

I_{P}

is the current passing through the parallel resistance of the circuit. All of these parameters may be found in Equations (5) and (6), respectively.

I_{0} = I_{0, n} . {(\frac{T}{T_{n}})}^{3} . \exp [\frac{q . E_{g}}{a . k} . (\frac{1}{T_{n}} - \frac{1}{T})]

(5)

I_{P} = \frac{V + I_{P V} . R_{s}}{R_{p}}

(6)

Then, the expression (1), becomes as it is in Equation (7).

I_{P V} = [I_{s c} + K_{i} . (T - T_{n})] . \frac{G}{G_{n}} - I_{0} [e^{\frac{(V + I_{P V} . Rs)}{a . V_{t}}} - 1] - \frac{V + I_{P V} . R_{s}}{R_{p}}

(7)

As a result, once the number of panels in serial or parallel is determined, the whole PV system voltage and current can be stated as in Equations (8) and (9), respectively.

V P V = N s . (a . K . T q) ln {N p . I p h - I p v + N p . I s N p . I s} - N s N p . I p v . R s

(8)

I P V = I P h . N p - I 0 . N P - ⎡⎣⎢⎢⎢ e ( V + I P V . Rs . N s N p ) a . V t N s - 1 ⎤⎦⎥⎥⎥ - V + I P V . R s N s N p R p . ( N s N p )

(9)

The maximum power equals 1.5 kW that can be given by the built PV model. The model was built using the datasheet’s accessible information. Table 1 illustrates the PV module’s essential specifications. To develop a model of a 250 W solar photovoltaic module, a real PV module SUN EARTH SOLAR POWER TPB156x156-72-P 250 W was considered as a standard module.

Table 1. PV system parameters per PV panel.

Parameters	Values	Parameters	Values
$I_{P h}$	7.9163 A	$K_{i}$	0.07 %/deg.C
$I_{0}$	1.3196 × 10⁻¹⁰ A	a	0.9467
$I_{s c}$	7.89 A	T	273 + (10, 15, 25, 30) K
$V_{o c}$	43.4 V	$T_{n}$	298 K
$R_{s}$	0.53082 Ω	G	(500, 750, 1000) W/m²
$R_{p}$	159.1067 Ω	$G_{n}$	1000 W/m²
$V_{m p}$	34.4 V	$I_{m p}$	7.27 A
K	1.38 × 10⁻²³ J/K	$E_{g}$	1.2 eV
q	1.6 × 10⁻¹⁹ C	$n_{s}$	72
$P_{P V}$	5 kW	$V_{P V}$	172 V
$I_{P V}$	29.069 A	$N_{s} * N_{p}$	5*4

2. Wind Turbine

As it is known, the wind turbine system is made up of two main components. The mechanical subsystem, which describes the propeller part and the gearbox tool, which adapts the torque and speed from the two sides and converts the wind power to a mechanical power adapted to the electrical subsystem. This second subsystem is built on an electrical machine, which can be any of several different types of electrical machines [22]. At the end of this loop, a variety of converters and inverters are used to adapt the outputted voltage to the main load or charge. Figure 1 shows this loop. In this research work, the permanent magnet machine is used to build the global wind system. In the next section, the corresponding mathematical model is given and described [23].

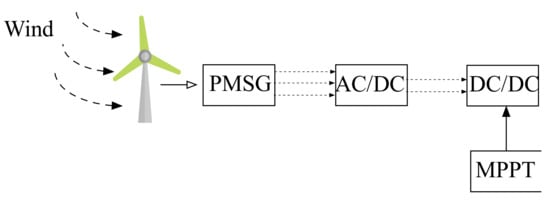
Figure 1. Wind system control loop.

2.1. Mechanical Subsystem

Equation (10) gives the mechanical power output of the wind turbine [24].

P m = P w . C p = 1 2 . ρ . S . V 3 w . C p (λ, β)

(10)

(C_{p})

represents the power coefficient of the wind turbine. It is expressed in Equation (11).

C p (λ, β) = C 1 . (C 2 λ i - C 3 . β - C 4) . e - C 5 λ i + C 6 . λ

(11)

λ_{i}

is given by Equation (12) as:

λ i = 1 1 λ + 0.08 . β - 0.035 β 3 + 1

(12)

Equation (13) presents The Tip speed ratio.

λ = R . Ω V v

(13)

Generally, the wind turbine specification can be analyzed by the curve, which draws the evolution of the power coefficient

C_{p}

in concordance to the angle of orientation

β

of propellers and the specific speed of the main shaft of the propellers noted

λ

. Figure 2 gives this relationship. According to the law of Betz, this coefficient can reach a maximum value of 59% in theory but practically it can reach 40% for the most efficient wind turbines [25].

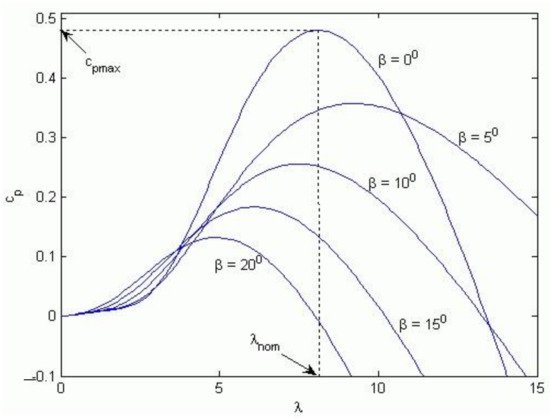
Figure 2. Wind system parameters according to the propeller orientation factor.

The mechanical angular speed of the turbine is calculated using the relationship between the electrical torque and the mechanical torque, which includes the mass of the generator and the turbine as in Equation (14).

J d ω m d t = T e - T m - F . ω m

(14)

2.2. Electrical Subsystem

For the final output desired physical parameter, which is the electrical current, an electrical machine is used to generate the electrical power. Modeling this block is necessary for completing all the generator block equations [26].

The use of the permanent magnet synchronous generator (PMSG) is preferable for WT technology. Due to its permanent magnets, the dynamic model of the PMSG does not depend on equations related to the rotor winding. For this, we can develop the dynamic model of the PMSG by using the equations of Voltage and flux. The voltage system of the equation noted

(V_{s}^{a b c})

and magnetic flux system of the equation noted

(Ø_{s}^{a b c})

can be visualized in Equations (15) and (16). All of these systems are created in a three-phase process (ABC) [27,28].

V a b c s = r s . i a b c s . d Ø a b c s d t

(15)

J d ω m d t = T e - T m - F . ω m

(16)

The rotor reference frame can be used to characterize the PMSG’s dynamic model. As a result, the voltage system of equations in the d and q axes is:

V q s = r s . i q s . L q . d i q s d t + ω r . L d . i d s . Ø m

(17)

V d s = r s . i d s + d i d s d t - ω r . L q . i q s

(18)

The electromagnetic torque is given by:

T e = (3 2) . (P 2) . (Ø m . i qs + (L d - L q) . i qs . i ds)

(19)

The parameters of the wind system can be visualized in Table 2.

Table 2. Wind system parameters.

Mechanical Parameters				Electrical Parameters
Parameter	Value	Parameter	Value	Parameter	Value
$P_{m e}$	13,500 W	$C_{4}$	5	$T_{e}$	50 N.m
$C_{p}$	0.48	$C_{5}$	21	$P$	4
$V_{w}$	-- m/s	$C_{6}$	0.0068	$Ø_{m}$	0.1688 web
$λ$	1.2	$S$	33.36 m²	$r_{s}$	0.0918 Ω
$Ω$	300 tr/min	$ρ$	1.292 Kg/m	$ω_{r}$	314.159 tr/min
$R$	3 m	$J$	148.5 Kg.m²
$β$	0 deg	$F$	1.4 N.m.s/rad
$C_{1}$	0.5176	$ω_{m}$	31.41 rad/s
$C_{2}$	116	$T_{m}$	45 N.m
$C_{3}$	0.4

3. Lithium Battery Pack

The main component inside this energy loop is based on the electric energy accumulator, which is based on the lithium battery version. For assuring the needed simulation and studying correctly the comportment of this system, a mathematical battery model is required to characterize the electrical characteristics. The given mathematical model is based on a Second-Order RC Equivalent Circuit Model (ECM) to describe the external electrical performance of the battery, as shown in Figure 3. The ECM consists of an open circuit voltage source (OCV), an internal ohmic resistance

R_{0}

, and two parallel RC arrays. Resistance

R_{1}

and capacity

C_{1}

are used to simulate the reactivity distribution to capture the local characteristics of the electrolyte. The charge transfer resistor

R_{2}

and the double-layer electrical capacity

C_{2}

, are used to represent the interface impedance of the battery [29,30,31,32].

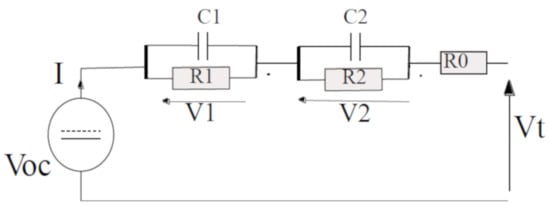
Figure 3. Battery pack: equivalent electrical diagram.

The differential equations of the ECM are exposed in Equations (20)–(22).

d V 1 d t = - V 1 R 1 . C 1 + I C 1

(20)

d V 2 d t = - V 2 R 2 . C 2 + I C 2

(21)

V t = V o c [S O C (t)] - V 1 - V 2 - I . R 0

(22)

I

is the current,

R_{0}

represents the Ohmic resistance of the storage device,

V_{1}

and

V_{2}

denote the polarization voltage over

R_{1} . C_{1}

and

R_{2} . C_{2}

, respectively

V_{t}

is the terminal voltage,

V_{o c}

represents the open-circuit voltage (OCV), which is a function of

S O C

The

S O C

value can be expressed as in Equation (23), where

Q_{n}

is the battery rated capacity [33].

S O C (t 1) = S O C (t 0) - \int t 1 0 I ( t ) d t Q n

(23)

The battery rated capacity depends on the effect of the capacity fading. The remaining battery capacity available is expressed in Equation (24) [34].

Q n = 3600 . Q i n i t . C C F

(24)

where

C C F

represents the Capacity correction factor and

Q_{i n i t}

is the Initial battery capacity [Ah]. From the other side, the functioning of the battery system can be summarized by the following Equations (25) and (26).

E c h (t) = E b a t (t - 1) + (E H E S (t) - E L (t)) . η b a t

(25)

E d i s (t) = E b a t (t - 1) - (E L (t) - E H E S (t))

(26)

where

E_{c h}

is the battery power in the charging,

E_{d i s}

is the battery power in the discharging,

η_{b a t}

is the battery efficiency (%),

E_{L}

is the load demand, and

E_{H E S}

is the power delivered from the energy system (W). Figure 4 shows the discharge characteristic example of the used battery. Table 3 shows the key specification of the considered battery.

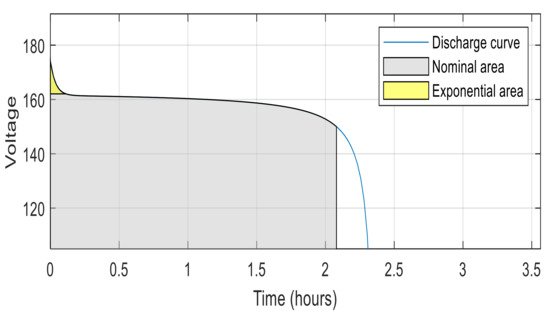
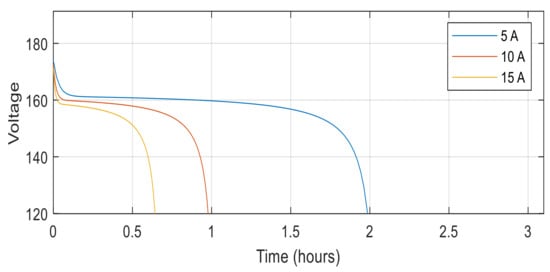
Figure 4. Discharge specification of the used battery.

Table 3. Battery system parameters.

Parameter	Value
$P_{n}$	1500 Wh
$V_{n}$	150 V
$Q_{n}$	10 Ah
$R_{0}$	0.15 Ω

4. Converters

Basically, numerous inverters must be used for having a DC flow from the major energy sources. A three-phase static converter based on a diode bridge rectifier (not controlled) is used to convert the alternating type (AC) electrical energy produced by the wind turbine into continuous type electrical energy (DC), connected directly by a boost converter (DC/DC) to reach the MPPT [35,36]. This last converter has the same objective for the photovoltaic system. On the other hand, a buck-boost converter (DC/DC) is installed to control the charge and discharge of the battery pack.

Based on these specific works, cited as [37,38], related to the electronic converters, it is easy to express the input and output voltage of the boost converter as in Equation (27).

V_{i n t}

is the input voltage,

V_{o u t}

is the output voltage,

D

is duty cycling.

V i n t = V o u t 1 - D

(27)

The buck-boost converter has two operation modes. It depends on the position of the Isolate Gate Bipolar Transistor (IGBT), which can be found inside. Supposing that these two switches have the nomination of

K_{10}

and

K_{11}

. The relationship between these two parameters can be expressed in Equation (28).

K 11 = 1 - K 10

(28)

As this chopper will be connected to a battery pack from one side and a DC bus line from the other side,

V_{b a t}

denotes the battery voltage side and

V_{d c}

denotes the DC bus voltage side. So, the relationship between the two voltages can be expressed as in Equation (29) and the output current can be formulated as in Equation (30).

L b a t . d i b a t d t + r b a t . i b a t = V b a t - V d c . (1 - K 10)

(29)

I o u t = I b a t . (1 - K 10)

(30)

This entry is adapted from the peer-reviewed paper 10.3390/su14052870

© Text is available under the terms and conditions of the Creative Commons Attribution (CC BY) license; additional terms may apply. By using this site, you agree to the Terms and Conditions and Privacy Policy.