The functioning of our universe and atomic is based on the oscillation of the particle itself and asymmetrically between matter and antimatter. This mechanism is a classical an⸱harmonic oscillator and uses a linear oscillation of the particle, where the energy can be represented by the graph of a potential well followed by the principle of energy conservation between kinetic energy and potential energy. This an⸱harmonic oscillation therefore occurs with an gravitational oscillator (see "hole through the earth simple harmonic motion"), followed by an singularity avoidance. Indeed the important kinetics of the particle leads to an singularity avoidance over the supermassive black hole to plot the Higgs field.
- universe
- anharmonic oscillator
- singularity avoidance
- gravitational oscillator
- kartazion
- cyclic model
- oscillating Universe
- oscillating model
- Higgs
- theory
1. Introduction
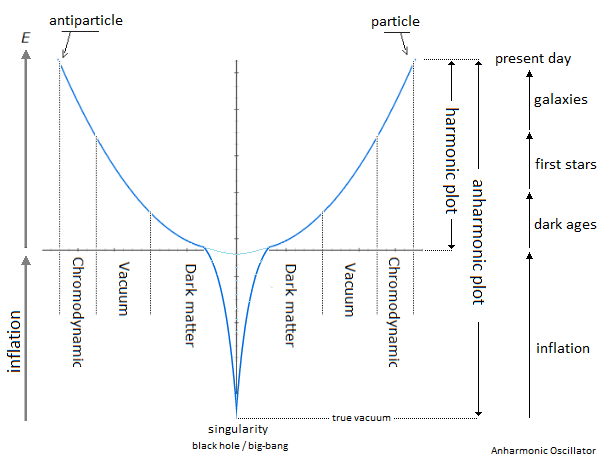
The functioning of our universe and atomic is based on the oscillation of the particle itself. This mechanism is a classical an⸱harmonic oscillator and uses a linear oscillation of the particle, where the energy can be represented by the graph of a potential well. This an⸱harmonic oscillation therefore occurs between matter and antimatter, followed by an avoidance of the gravitational singularity. This singularity avoidance [1][2][3] is of the supermassive black hole and / or big-bang type. On the other hand, and at total rest at x=0, this particle is at the bottom of the potential well, at the lowest level, at the singularity, namely the total collapse of the universe which represents a state of true vacuum. But again and by a more sustained oscillation a singularity avoidance which is opposite of rest creates the levitation of the particle into higher energy levels in its potential well. This singularity avoidance allows access to the current false vacuum without falling at the bottom of the well, thanks to the Kinetic Energy or the inertia of the particle. The role of the singularity in our model is fundamental and implies that it is the source and driver of the actual known result of quantum and cosmological fluctuations in relation to the motion of the particle.
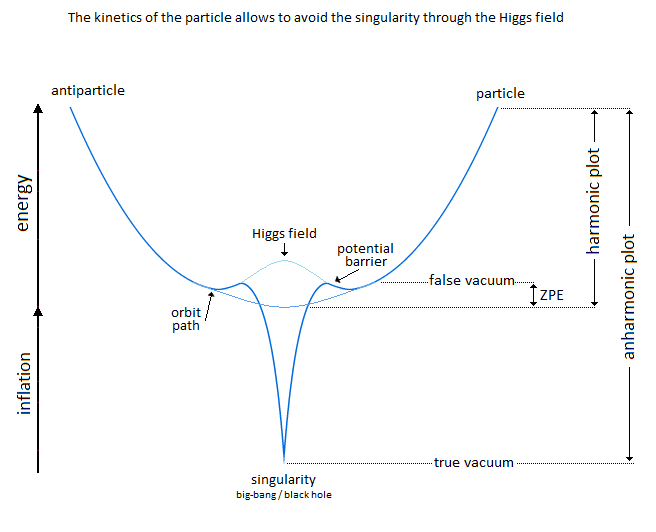
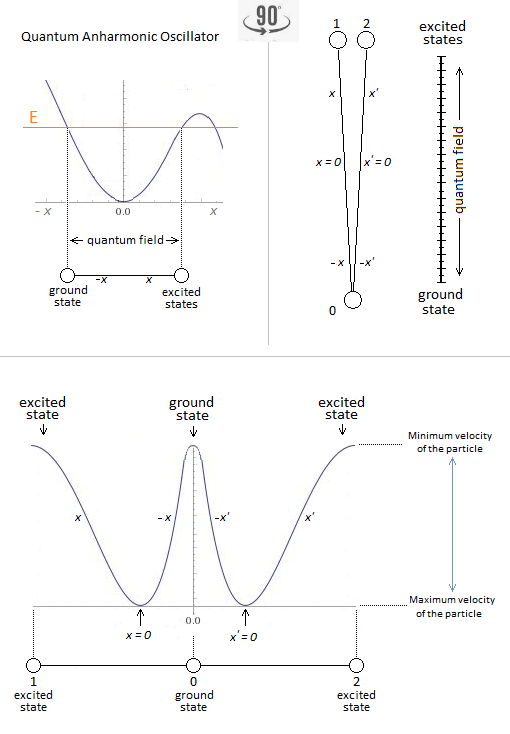
Harmonic Oscillator vs Anharmonic Oscillator. Indeed the difference is made here and is between the ideal harmonic layout and the absolut anharmonic layout of the energy potential well. In the case of singularity avoidance due to the high Kinetic Energy of the particle, the plot of the curve of the potential well tends towards a harmonic shape rather than an anharmonic one. Moreover the anharmonic implication can be double: the variation of speed of the particle followed by the shape of the potential well due to the singularity can involve this anharmonicity. More exhaustively we can still imply the internuclear distance and the morse potentials. In the following illustration the integration of the singularity at x=0 is not yet represented.
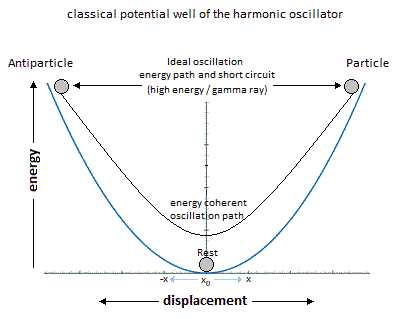
If the energy potential well has a curved shape, it represents the form of the amount of energy delivered to the particle according to x. Which does not mean that the particle moves in a half-moon. The particle moves nice and well ina straight line in a radiative or linear way. In the previous graph we can distinguish three types of possible interpretations of the states and energy paths of the particle. At 1 and at the bottom of the harmonic energy potential well at x=0, we have the rest of the motionless particle (or ZPE). In 2 and with Kinetic Energy applied to the particle is at x=0 the maximum speed of the particle and drawing a harmonic curve. In 3 it should be noted that the ideal trajectory of the particle is of the short circuit type between matter and antimatter and would trace a straight line, i.e. the shortest energy path in the potential well. This utopian energy path is actually impossible for the particle to take. However, the annihilation of the electron / positron pair into a gamma photon should indicate a short-circuit type trajectory, and ultimately define the characteristic of a boson. In the following illustration an integration of the gravitational singularity disturbs the harmonic shape of the potential well. The greater the kinetics of the particle in its oscillation, the more the shape of the potential well tends towards harmony by avoidance of gravitational singularity. But if the kinetics of the particle is weak, then the particle falls at the bottom of this gravitational singularity and traces the anharmonicity of the potential well.
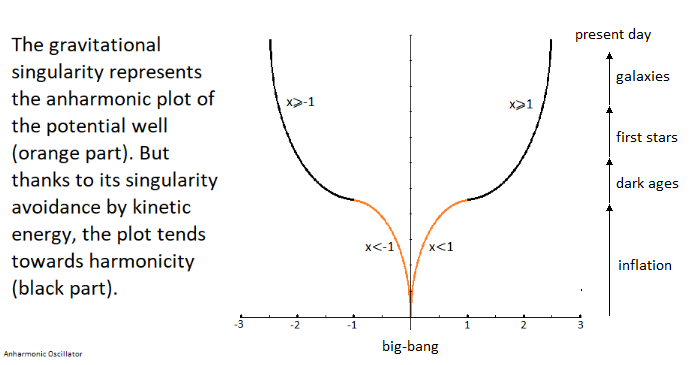
In a second part of this introduction, it is a question of the singularity avoidance where the particle through its path, traces the Higgs field. The Potential Energy is in relation to the depth of the singularity, because to pass over a black hole would have for value an enormous Potential Energy, and would trace the curve of the potential of Higgs of it.
2. Perpetual oscillation & ideal distribution of energies
Perpetual motion is utopian. But in our case it represents an oscillation without mechanical constraint followed by the principle of energy conservation. In an interpretation of classical mechanics, the an⸱harmonic oscillation of the particle can be represented through a gravitational oscillator. It is initially used gravity to subject by attraction a movement to the particle. Here is the example chosen of an oscillation of the particle which uses the same direction as the vector of gravity to be able to move. Its description is based on the "hole through the earth" [34][45] which does not really have an example in French but which is nevertheless well studied forits principle. Here is his summary explanation: "If you drilled a hole in the axis of the Earth from pole to pole, and inserted a long, thin vacuum chamber into it, and then dropped an object into one end of that chamber, it would fall into the hole, picking up speed and it would move very fast when it reached the center of the Earth so it would continue until it reached the other pole where it would stop, then fall back "bounce" to come back and start again perpetually." (Search engine and keywords with 'hole through the earth simple harmonic motion').
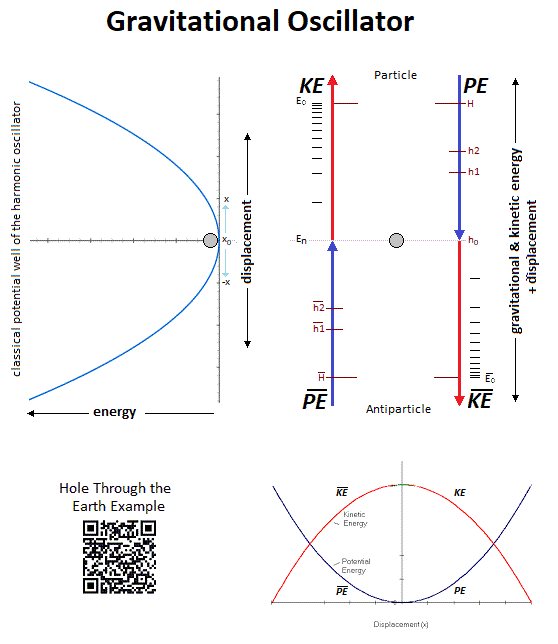
This linear gravitational oscillator uses a particle of mass m oscillating vertically along the gravity vector G. The oscillation has two phases. The first is the falling phase of the particle with its Potential Energy PE and the second is the reverse phase which corresponds to the Kinetic Energy KE. Example: It is from the center of the Earth that which is in Potential Energyis transformed into Kinetic Energy and is reversed at the level of the point 0 or x=0 and vice-versa.
3. Kinetic Energy & Potential Energy
In conclusion, for the gravitational oscillator presented above, the distribution of the two energies follows the following diagram and operates according to the linear displacement of the particle, i.e. the alternation of the Potential Energy PE with the Kinetic Energy KE.
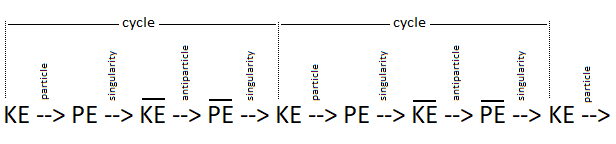
Potential Energy - Kinetic Energy = 0
4. Source of quantum fluctuations & gravitational singularity
In our model the source and the material existence as we know it is based on the implication of the singularity of the big-bang type for the universe and of the supermassive black hole type for the functioning of the galaxies, and other theoretical singularity as for that of the center of an atom [56]. In other words, the divergent evolution of the volume of the singularity is responsible for the matter that remains in its space-time. As gravity (Potential Energy) pulls the particle towards the core of the singularity, and who approaches it to fall inside, is then suddenly expelled by pure energy emanating from that same singularity. Indeed the singularity generates in 1 the gravitational attraction noted Potential Energy PE and in 2 the prediction that the source of energy is by scratching and / or astrophysical relativistic jet noted Kinetic Energy KE emanates from this same singularity [7][8]. Moreover in 3 it should be noted that the singularity generates eletric charges on the particle.
In a more speculative and exotic definition and given our knowledge of the singularity in terms of gravitation followed by its time dilation, we could associate the center of the earth as such. Indeed for the particle which oscillates in the radiatis direction ive oscillation from the center to the surface and vice versa, it becomes easy to imagine an singularity avoidance at the level of the Earth's core in the same way as the supermassive black hole in relation to its galaxy [9][10].
5. Singularity avoidance and Higgs
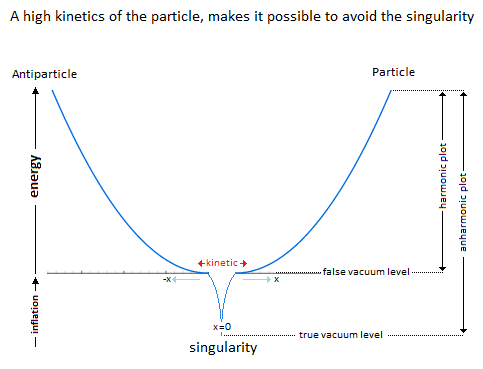
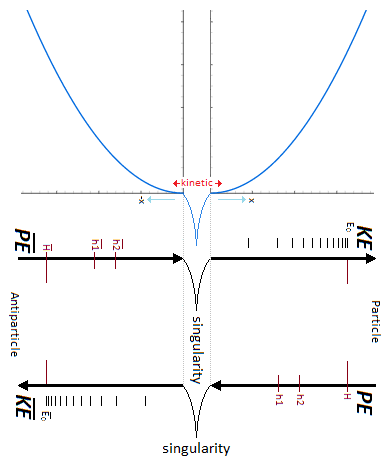
Singularity avoidance [1][2][3] is due to the high Kinetic Energy of the particle. At x=0 when the particle is going as fast as possible, its Kinetic Energy allows it not to fall at the bottom of the well at the level of the gravitational singularity. Gravity (Potential Energy) corresponds to the matter attracted towards this singularity, while energy pushes it back. In our case it is simply a particle rather than a cluster of matter.
A significant kinetics of the particle makes it possible to avoid the singularity, and the path takes above this singularity by the particle determines the plot of the Higgs field (vector). Singularity avoidance and Higgs (scalar field) [611].
In a second time the theoretical junction between the singularity avoidance and the Higgs field can be done by the enormous Potential Energy. Indeed, the Potential Energy being that in relation to the depth of the gravitational singularity gives the particle a much greater mass, or even a maximum value.
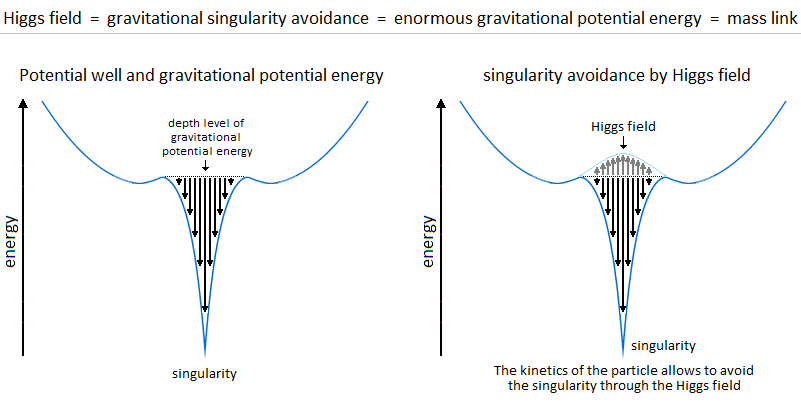
The Higgs field and potential are also well used to be able to represent the metastability [712][813][914] of the universe, as well as its interpretation as a quantum particle for its origin of the mass.
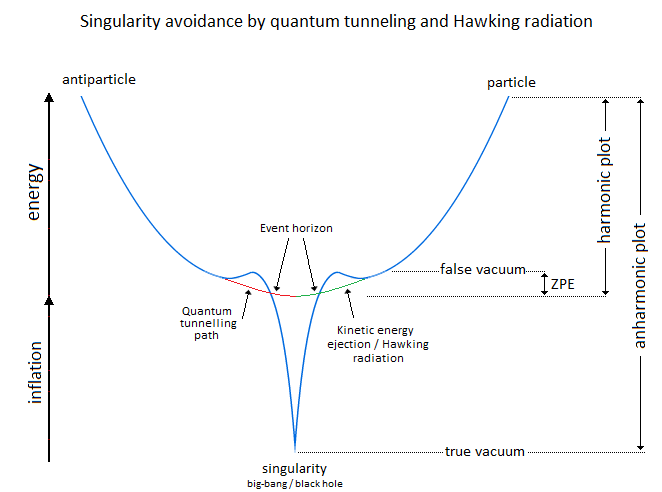
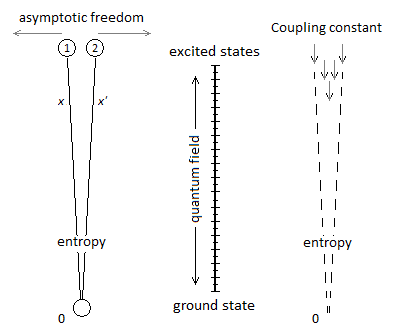
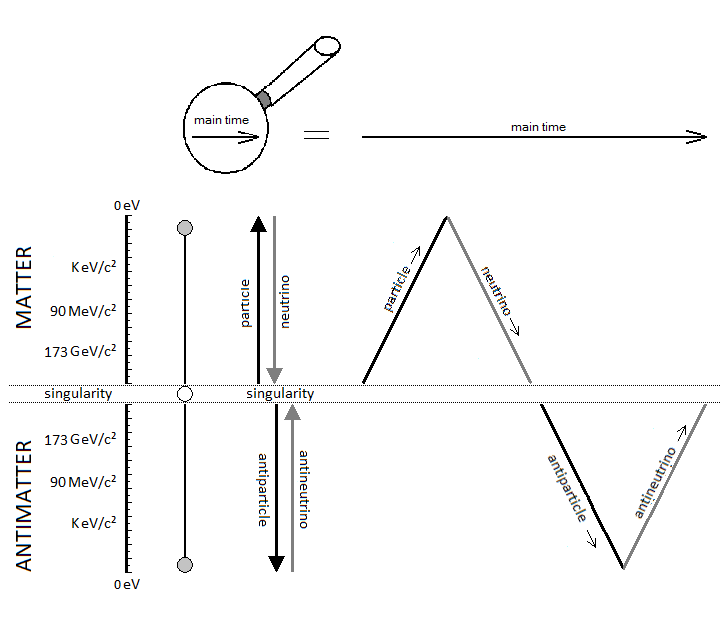
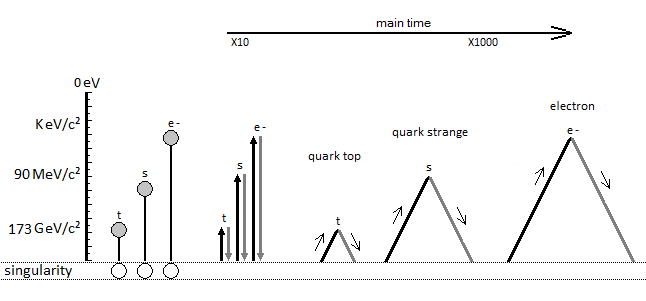
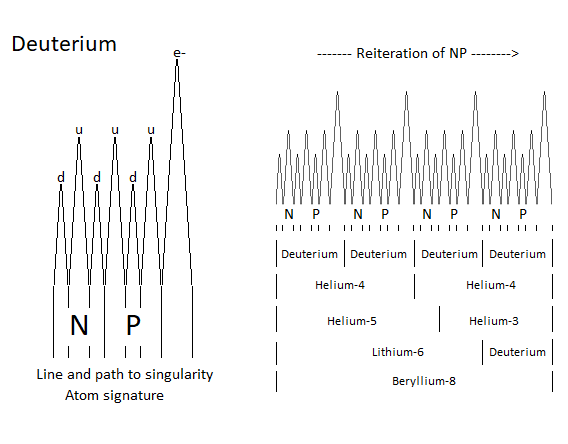
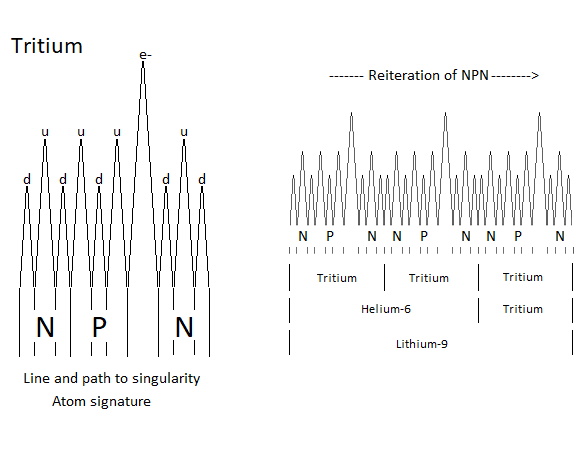
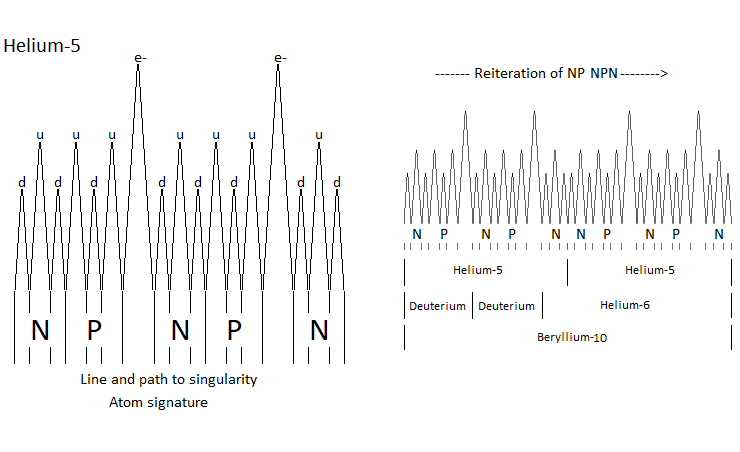
Singularity avoidance = enormous Potential Energy = Higgs mechanism = mass link It should be understood that in the case of slowed kinetics, the particle will then tend to pass through the virtual slit of the tunnel effect instead of the singularity avoidance by the Higgs field and finds itself at the level of the black hole horizon event. This being said, this does not mean that the particle will finally reach true vacuum because kinetic energy is then sent or subjected to the particle in order to be able to eject [107][118] it again into the upper harmonic well of our universe / galaxies. This is to be considered as a singularity avoidance at the horizon event of a black hole. We indicate a Potential Energy barrier around gravitational singularities [1215]. This barrier is due to the deformation of the space-time curvature. At least a difference in level where the Potential Energy is felt around the mass object. Without Kinetic Energy, the inertia of the object (or particle) allows it to slide along the potential barrier that defines the path of the orbit around an even more massive object. In other words, the deformation of the space-time curvature due to an average object is felt at the level of the heights of the energies, and creates a barrier. Vacuum metastability is determined by the amount of Kinetic Energy applied to the particle to trace its potential field. If the Kinetic Energy of the particle is sufficient and therefore if the range of the energy condition allows the energy potential barrier to be passed, then singularity avoidance occurs; But during an attenuation of the kinetics of the particle or even a total stop of the inertia, that will cause by its amount of lower energy its fall towards the singularity and will reach the true vacuum. In other words, the inertial disturbance of the ZPE of the particle makes it possible to remain in the false vacuum by inertia, until a fictitious drop in this energy slows it down and then causes it to fall through a virtual slit to reach the true vacuum. According to the units of Planck, the number and the quantity of gravitons put end to end (point to point) along the vector of gravity G, then gives the total of the energy of gravitational potential. Each increment / decrement of the graviton, is obtained by adding or subtracting a linear total of energy quanta, which can be represented by the size of the particle. It becomes easy to take into account the number of gravitons accumulated over the length of a ray noted in nm where this ray is parallel to the length of the vector G inorder to be able to have a relationship between the number of gravitons in relation to the Potential Energy. Most important is the role and the why of antimatter. As we can see the gravitational oscillator looks like a balanced perpetual motion without mechanical constraints. It is precisely on this side of the balance without mechanical constraint of the oscillation, that it makes that between Kinetic Energy and gravitation (Potential Energy) the role of the antimatter becomes important. This allows the particle to simply bounce (due to deceleration from depletion of the particle's Kinetic Energy) to turn around using Potential Energy. There is therefore no impact of the particle that occurs in its cycle of oscillation. The Dirac Sea is a perfect representation of what the electron becomes in the depths of energy. Dirac predicts antimatter and the positron [1417]. We must therefore imply an anti-inflation followed by its anti-universe. This therefore explains why we do not find the expected antimatter in the matter side of the universe, because antimatter is indeed found on the anti-universe side. In conclusion during a high energy collision, the annihilation of the pairs of particles make detect thanks to the gamma photon the presence of antimatter through the space-time. We understand that the symmetry breaking is located at x=0 at the level of the gravitational singularity. There is therefore a link between the Higgs potential and the symmetry breaking around x=0. If the charge and the parity CP are inter-changed following a linear movement of the particle either from bottom to top, then the arrow of time is perpendicular and flows for example from left to right. Still based on the oscillation of the particle itself, its presence distribution through its momentum and its position according to x (displacement) is asymmetrically arranged between matter and antimatter [1518]. Indeed the particle cannot be on matter and antimatter at the same time. Based on the functioning of the mechanism of the anharmonic oscillator, involves during the movement of the particle, to make it go through different physical stages due to its high speed of displacement between matter and antimatter. We can also talk about the particle's energy flow to express the different cosmological or quantum states observed (Kasimir effect, etc.) [1619]. The path of the particle is therefore linear and forms round trips. The path of the particle is therefore radiative. When the particle reaches a sufficient speed, a transformation into mass energy by the equivalence principle occurs. A small trace of this energy is found in vacuum and represents quantum vacuum energy. The successive addition of vacuum energy gives dark matter. In other words, the convergence of the energy flow (quantum fluctuation) of the quantum vacuum, in a more restricted space, close to the singularity, then in turn becomes dark matter [1720][1821][1922]. At its opposite and the opposite of the singularity, there is matter expressed by quantum chromodynamics. Dark matter and quantum vacuum energy / quantum fluctuation is produced with the condition of the particle moving at very high speeds. Here is an example of the absolute path taken by the particle. Here is a simple energy potential well to explain dark matter and quantum vacuum and quantum chromodynamics in relation to the oscillator energy potential well: Dark energy corresponds to the increase in the size of the potential well. In the gravitational oscillator, the incrementation of the particle, that is to say a height of radiation greater than the previous height in the potential well, is produced by additional energy called dark energy; And which makes the particle advance further and further from its gravitational source. The example of a galaxy, where inside does not know an expansion of size in relation to its super massive black hole, uses a constant amount of Kinetic Energy in relation to gravity (Potential Energy). The increase in this Kinetic Energy that we have seen so far, then becomes dark energy, while it is only a amount of energy greater than the previous energy impulse. In other words, to move the particle further and further away from the singularity (i.e. big-bang) with the gravitational oscillator, more energy is needed. This extra amount of energy is dark energy [2023][2124][2225][2326]. Schrödinger's Cat is a thought experiment that illustrates the result of the quantum superposition paradox. If the particle is on A, then it cannot be on B. But at very high frequencies the particle has almost a 50% chance of being on A and B at the same time, hence the superposition state. If you were to randomly choose a position between A and B, then you would either have the particle in the hand (alive) or no particle in the hand, hence the cat experiment. In Prediction; The superposition of quantum states is at least straddling matter and antimatter before being detected on the matter side [2427][2528][2629]. Quantum ChromoDynamics (QCD) would only be a duplication of the particle itself. It describes the mechanics of the quark superposition transition. In other words, quantum chromodynamics is the alternation of the particle itself to form the different combinations of quarks [6]. QCD is the oscillation of the field which interferes with the particle in its convergence of the position towards its point of oringne 0 and relating to the coupling constant (gauge coupling parameter). On the other hand during the material divergence due to the corelation of the particle on the surface of the energy sea, separating the quarks (which is only e.g. the alternating oscillation of the particle between 0 and 1 and 2) acts on the contrary of the coupling constant on asymptotic freedom [30][31][32][33][34][35]. It becomes obvious to make the link between entropy and plsma oscillation [36][37]. The implication of radiation is simply due to the fact that the particle in its oscillation is linear. The direction of the work of the particle is along the vector of gravity, namely parallel to it. The gravitational oscillator has two types of radiation. First there is the vertical radiation, called fermionic, i.e. the normal oscillation of the particle from bottom to top and from top to bottom; And there is the horizontal radiation, or bosonic radiation which is not the oscillator. For the fermionic radiation and with the example of the lepton we have a movement of the particle from bottom to top in the oscillator which makes it possible to transport an electric charge to the surface of the sea of energy. In the opposite direction, either from top to bottom the particle goes down again in neutrino, or with a neutral charge. IOW this model, there is the link between Kinetic Energy and electric charge, and the neutrino follows the Potential Energy, (gravity). Bosonic radiation, in its analogous interpretation, emits a kind of electric arc that occurs horizontally. The quantum atom is basically composed of quantum leap of the particle between matter and anti-matter. These jumps correspond to the Bottom-up oscillation and have an almost instantaneous value. They can for example be of the order of a few million or a few billion jumps in a nanosecond. The Pauli exclusion is always respected because there is only one particle present per atom created by a reiteration of its position in different and unique states. The neutrino represents the particle without electric charge and is electrically neutral. The neutrino has a direct relationship with that of Potential Energy. On the contrary, when the particle is emitted by Kinetic Energy, the latter carries an electric charge. The dosage of the Kinetic Energy makes it possible to deliver the amount of energy necessary in term of electron-volt. Thanks to the principle of reiteration, and if you had the choice between neutron and proton, the probability of finding an N neutron followed by a Pproton like NP or NPN in the atomic nuclei is substantial. Which brings us, and in relation to the atomic signature, to the conclusion of a composition rich in Deuterium, Tritium and Helium 4-5-6. The line spacing corresponds to the atomic signature as a function of the energy delivered by the particle. The smaller the energy in electron volts, the larger the line spacing. The absence of lines indicates that there are no particles in the field to be studied. Each line represents the path to the singularity by neutrinos as it descends, and responsible for the electrical charges generated as it ascends. The constitution of the physical laws as well as the result of this chaos of the universe until us suggests that it was premeditated. The name of the main theory described in this paper is called Kartazion. Kartazion model of quantum physics and cosmological according to Arnaud Andrieu.6. Second interpretation of singularity avoidance
7. Orbit, inertia, ZPE & potential barrier
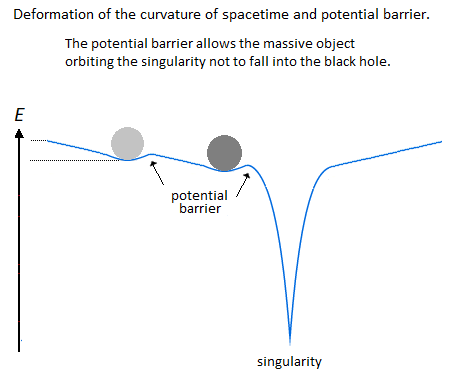
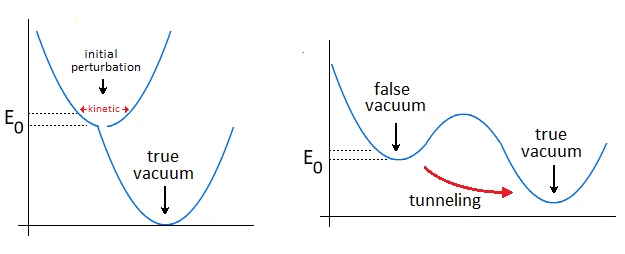
The ZPE (Zero Point Energy) represents the initial perturbation of the particle. At the quantum level this means that when the particle is at rest at the bottom of the harmonic potential well, the particle undergoes an oscillating disturbance. In cosmology this disturbance represents the inertial movement as an object orbiting around the gravitational singularity / massive object. In other words, the initial quantum disturbance of the Zero Point Energy (ZPE) corresponds to the cosmological movement of the object / particle located in the false vacuum in orbit around the gravitational singularity [1316]. Deformation also occurs for massive objects like stars.8. Vacuum metastability
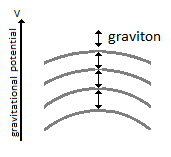
9. Graviton & Potential Energy
10 - Asymmetry CP matter antimatter
11. Symmetry breaking CP & arrow of time T
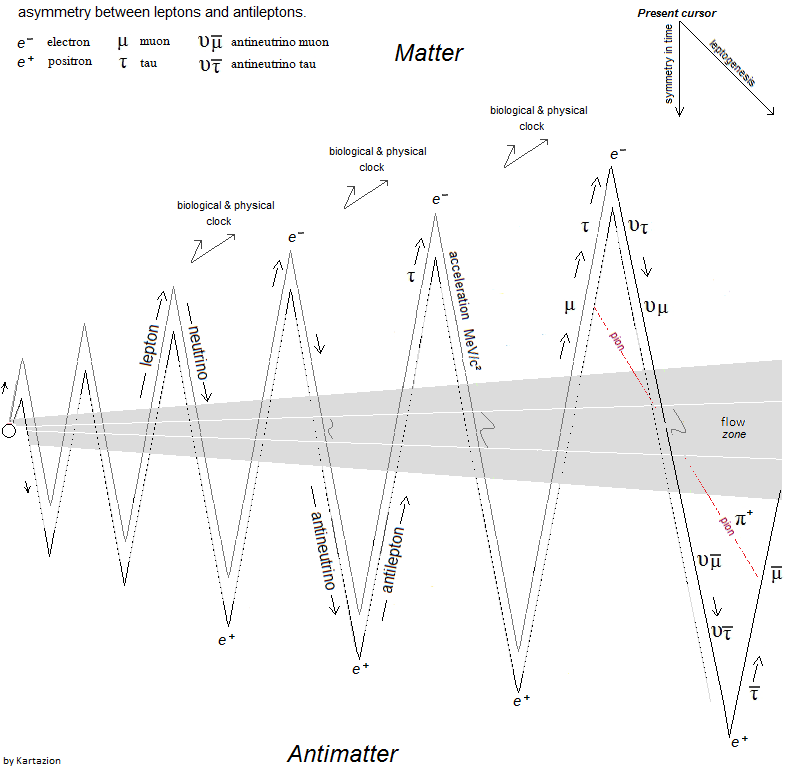
12. Path of the particle, quantum fluctuation & dark matter
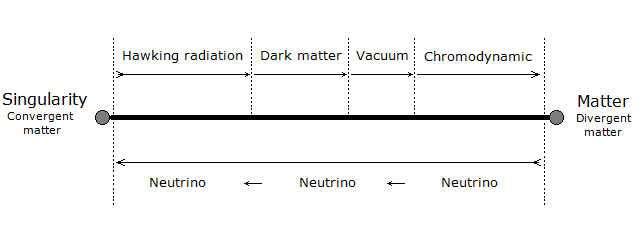
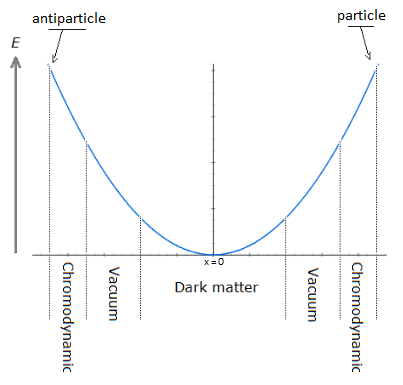
13. Dark energy
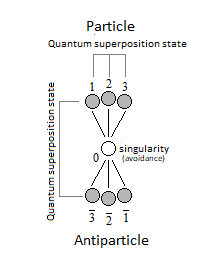
14. Quantum superposition
15. Quantum ChromoDynamics
Here we understand through quantum chromodynamics, which represents finite matter, that the general interaction field of quarks in its form of confinement, shapes objects as we perceive them. In other words, the general field of the universe guides the quarks by forming the different atoms through the harmonic oscillator. This field is responsible for where the particle is located in the universe.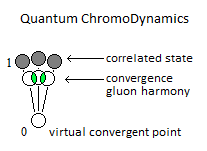
16. Particle radiation & boson
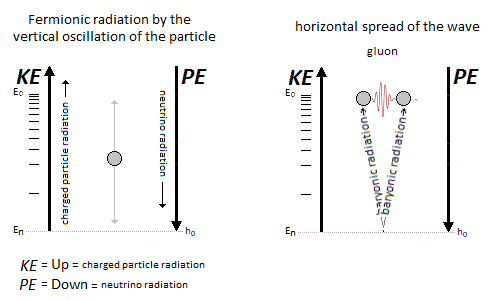
17. Atom & quantum atom
Pure quantum atom. The pure quantum atom is non-isotropic. It corresponds to the two choices which is that of the neutron N or the proton P. The reiteration in a series of Neutrons N followed by Protons P (same number of N as of P) is a pure atom. The atomic model described here represents the synopsis of the logical sequence of the oscillation mechanism of a single moving particle. Here is the diagram of the anharmonic characteristic of the particle. Its oscillation is located between matter and antimatter, where between two its acceleration would then be almost instantaneous by singularity avoidance. We can see by the anharmonic oscillator the classic version of the internuclear distance followed by the morse potentials to be able to give the energy wanted to the particle.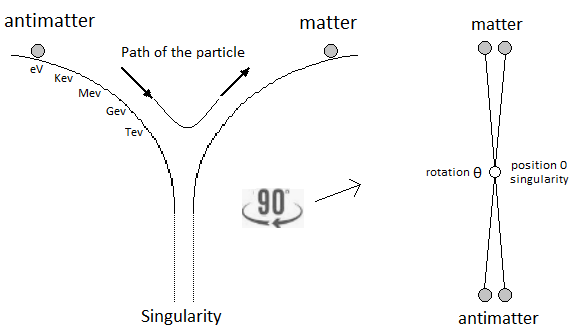
18 - Example structure & conclusion
References
- Iberê Kuntz; Roberto Casadio; Singularity avoidance in quantum gravity. Physics Letters B 2020, 802, 135219, 10.1016/j.physletb.2020.135219.
- V Husain; Singularity avoidance, lattices, and quantum gravity. Canadian Journal of Physics 2008, 86, 583-586, 10.1139/p07-201.
- Andrew J. Simoson; Falling down a Hole through the Earth. MJ Brunnemann; T Thiemann; On (cosmological) singularity avoidance in loop quantum gravity. Clathematssics Magazine al and Quantum Gravity 2004, 77, 171, 10.2307/3219113.6, 23, 1395-1427, 10.1088/0264-9381/23/5/001.
- Journey through the center of the Earth . Hyperphysics. Retrieved 2022-7-28Andrew J. Simoson; Falling down a Hole through the Earth. Mathematics Magazine 2004, 77, 171, 10.2307/3219113.
- The hydrogen atom with an origin centred singularity . ResearchGate. Retrieved 2022-7-28Journey through the center of the Earth . Hyperphysics. Retrieved 2022-7-28
- Luca Fabbri; Black Hole singularity avoidance by the Higgs scalar field. The European Physical Journal C 2018, 78, 1028, 10.1140/epjc/s10052-018-6505-6.The hydrogen atom with an origin centred singularity . ResearchGate. Retrieved 2022-7-28
- Marcelo Gleiser; Metastability in the early Universe. Physical Review D 1990, 42, 3350-3361, 10.1103/physrevd.42.3350.How is Matter Ejected from an Event Horizon Around a Black Hole? . The National Radio Astronomy Observatory. Retrieved 2022-7-28
- Katherine J. Mack; Robert McNees; Bounds on extra dimensions from micro black holes in the context of the metastable Higgs vacuum. Don N Page; Hawking radiation and black hole thermodynamics. New Journal of Physical Review D s 20019, 99, 063001, 10.1103/physrevd.99.063001.5, 7, 203-203, 10.1088/1367-2630/7/1/203.
- Itzhak Bars; Paul J. Steinhardt; Neil Turok; Cyclic cosmology, conformal symmetry and the metastability of the Higgs. PA Marasco; G Cresci; L Posti; F Fraternali; F Mannucci; A Marconi; F Belfiore; S M Fall; A universal relation between the properties of supermassive black holes, galaxies, and dark matter haloes. Monthlysics Letters B Notices of the Royal Astronomical Society 20213, , 50726, 50-55, 10.1016/j.physletb.2013.08.071., 4274-4293, 10.1093/mnras/stab2317.
- How is Matter Ejected from an Event Horizon Around a Black Hole? . The National Radio Astronomy Observatory. Retrieved 2022-7-28Andrew King; The Supermassive Black Hole—Galaxy Connection. null 2013, 49, 427-451, 10.1007/978-1-4939-2227-7_21.
- Don N Page; Hawking radiation and black hole thermodynamics. NLuca Fabbri; Black Hole singularity avoidance by the Higgs scalar field. Thew Journal of European Physics al Journal C 2005, 18, 7, 203-203, 10.1088/1367-2630/7/1/203.8, 1028, 10.1140/epjc/s10052-018-6505-6.
- Nikolaos Tetradis; Black holes and Higgs stability. Journal of CosmologMarcelo Gleiser; Metastability in the early Universe. Phy and Astroparticle Physics 2ical Review D 199016, , 42016, 036-036, 10.1088/1475-7516/2016/09/036., 3350-3361, 10.1103/physrevd.42.3350.
- Bernhard Haisch; Alfonson Rueda; H.E. Puthoff; Physics of the zero-point field: implications for inertia, gravitation and mass. SpeKatherine J. Mack; Robert McNees; Bounds on extra dimensions from micro black holes in the context of the metastable Higgs vacuum. Physicualations in Science and Technology Review D 201997, 20, 99-114, 10.1023/a:1018516704228., 99, 063001, 10.1103/physrevd.99.063001.
- Mark Kowitt; Gravitational repulsion and Dirac antimatter. International Journal of Theoretical Itzhak Bars; Paul J. Steinhardt; Neil Turok; Cyclic cosmology, conformal symmetry and the metastability of the Higgs. Physics Letters B 201996, 35, 605-631, 10.1007/bf02082828.3, 726, 50-55, 10.1016/j.physletb.2013.08.071.
- Tong Bor Tang; Li Zhi Fang; The cosmic asymmetry in matter-antimatter. VistNikolaos Tetradis; Black holes and Higgs stability. Journal of Cos inmology and Astronomparticle Phy sics 201984, 6, 27, 1-23, 10.1016/0083-6656(84)90010-2.016, 036-036, 10.1088/1475-7516/2016/09/036.
- Lawrence Slifkin; Entropy and the Frequency of a Harmonic Oscillator. AmBernhard Haisch; Alfonson Rueda; H.E. Puthoff; Physics of the zero-point field: implications for inertia, gravitation and mass. Sperculations in Scican Journal of Phence and Technologysics 1965, 33, 408-408, 10.1119/1.1971569.97, 20, 99-114, 10.1023/a:1018516704228.
- Four reasons why the quantum vacuum may explain dark matter . PhysOrg.com. Retrieved 2022-7-28Mark Kowitt; Gravitational repulsion and Dirac antimatter. International Journal of Theoretical Physics 1996, 35, 605-631, 10.1007/bf02082828.
- Antonio Capolupo; Quantum Vacuum, Dark Matter, Dark Energy, and Spontaneous Supersymmetry Breaking. AdvTong Bor Tang; Li Zhi Fang; The cosmic asymmetry in matter-antimatter. Vistances in High EnergAstronomy Physics 20 198, 4, 2018, 1-7, 10.1155/2018/9840351.7, 1-23, 10.1016/0083-6656(84)90010-2.
- Dragan Slavkov Hajdukovic; Quantum vacuum and dark matter. Lawrence Slifkin; Entropy and the Frequency of a Harmonic Oscillator. Astmerophysics and Space Science 20ican Journal of Physics 11, 965, 337, 9-14, 10.1007/s10509-011-0938-9., 408-408, 10.1119/1.1971569.
- Davide Castelvecchi; New type of dark energy could solve Universe expansion mystery. Nature 2021, 1, 1, 10.1038/d41586-021-02531-5.Four reasons why the quantum vacuum may explain dark matter . PhysOrg.com. Retrieved 2022-7-28
- Eric V. Linder; Dark Energy, Expansion History of the Universe, and SNAP. PROCEEDINGSAntonio Capolupo; Quantum Vacuum, Dark Matter, Dark Energy, and Spontaneous Supersymmetry Breaking. Advances OFin THE INTERNATIONAL CONFERENCE “PHYSICAL MESOMECHANICS. MATERIALS WITH MULTILEVEL HIERARCHICAL STRUCTURE AND INTELLIGENT MANUFACTURING TECHNOLOGY” High Energy Physics 2003, 655, 193-207, 10.1063/1.1543500.18, 2018, 1-7, 10.1155/2018/9840351.
- Jacob Schaf; dark energy expansion. UniverDragan Slavkov Hajdukovic; Quantum vacuum and dark matter. Asal Joutrnal of Pophysics and ApplicatioSpace Scien ce 2015, 9, 182-187, 10.13189/ujpa.2015.090403.1, 337, 9-14, 10.1007/s10509-011-0938-9.
- Dragan Huterer; David Kirkby; Rachel Bean; Andrew Connolly; Kyle Dawson; Scott Dodelson; August Evrard; Bhuvnesh Jain; Michael Jarvis; Eric Linder; et al.Rachel MandelbaumMorgan MayAlvise RaccanelliBeth ReidEduardo RozoFabian SchmidtNeelima SehgalAnže SlosarAlex van EngelenHao-Yi WuGongbo Zhao Growth of cosmic structure: Probing dark energy beyond expansion. AsDavide Castelvecchi; New type of dark energy could solve Universe expansion mystery. Naturoparticle Physics 20214, 63, 23-41, 10.1016/j.astropartphys.2014.07.004., 1, 1, 10.1038/d41586-021-02531-5.
- Gang Xin; Peng Wang; Exploring superposition state in multi-scale quantum harmonic oscillator algorithm. Eric V. Linder; Dark Energy, Expansion History of the Universe, and SNAP. PROCEEDINGS OF THE INTERNATIONAL CONFERENCE “PHYSICAL MESOMECHANICS. MATERIALS WITH MULTILEVEL HIERARCHICAppliedL Soft Computing TRUCTURE AND INTELLIGENT MANUFACTURING TECHNOLOGY” 20021, 107, 107398, 10.1016/j.asoc.2021.107398.3, 655, 193-207, 10.1063/1.1543500.
- Eva Zakka-Bajjani; François Nguyen; Minhyea Lee; Leila R. Vale; Raymond W. Simmonds; Jose Aumentado; Quantum superposition of a single microwave photon in two different ’colour’ states. NJacob Schaf; dark energy expansion. Universatl Jourenal of Physics and Application 2011, 7, 599-603, 10.1038/nphys2035.5, 9, 182-187, 10.13189/ujpa.2015.090403.
- Martin J. Renner; Časlav Brukner; Computational Advantage from a Quantum Superposition of Qubit Gate Orders. Dragan Huterer; David Kirkby; Rachel Bean; Andrew Connolly; Kyle Dawson; Scott Dodelson; August Evrard; Bhuvnesh Jain; Michael Jarvis; Eric Linder; et al.Rachel MandelbaumMorgan MayAlvise RaccanelliBeth ReidEduardo RozoFabian SchmidtNeelima SehgalAnže SlosarAlex van EngelenHao-Yi WuGongbo Zhao Growth of cosmic structure: Probing dark energy beyond expansion. Astroparticle Physical Review Letters 2022, 128, 230503, 10.1103/physrevlett.128.230503.14, 63, 23-41, 10.1016/j.astropartphys.2014.07.004.
- Gang Xin; Peng Wang; Exploring superposition state in multi-scale quantum harmonic oscillator algorithm. Applied Soft Computing 2021, 107, 107398, 10.1016/j.asoc.2021.107398.
- Eva Zakka-Bajjani; François Nguyen; Minhyea Lee; Leila R. Vale; Raymond W. Simmonds; Jose Aumentado; Quantum superposition of a single microwave photon in two different ’colour’ states. Nature Physics 2011, 7, 599-603, 10.1038/nphys2035.
- Martin J. Renner; Časlav Brukner; Computational Advantage from a Quantum Superposition of Qubit Gate Orders. Physical Review Letters 2022, 128, 230503, 10.1103/physrevlett.128.230503.
- Kanako Yamazaki; T. Matsui; Gordon Baym; Entropy in the quark–hadron transition. Nuclear Physics A 2015, 933, 245-255, 10.1016/j.nuclphysa.2014.10.046.
- David Dudal; Subhash Mahapatra; Thermal entropy of a quark-antiquark pair above and below deconfinement from a dynamical holographic QCD model. Physical Review D 2017, 96, 126010, 10.1103/physrevd.96.126010.
- Tri Quoc Truong; Tadashi Tsubone; Munehisa Sekikawa; Naohiko Inaba; Border-collision bifurcations and Arnol’d tongues in two coupled piecewise-constant oscillators. Physica D: Nonlinear Phenomena 2019, 401, 132148, 10.1016/j.physd.2019.132148.
- P. A. Cook; Meson coupling constants in a quark model. Il Nuovo Cimento A Series 10 1967, 48, 570-572, 10.1007/bf02818032.
- R. Brout; From asymptotic freedom to quark confinement. Nuclear Physics B 1988, 310, 127-140, 10.1016/0550-3213(88)90057-0.
- D Gabor; Plasma oscillations. British Journal of Applied Physics 1951, 2, 209-218, 10.1088/0508-3443/2/8/301.
- Toshio Nakayama; Irreversibility in Plasmas: Entropy Production. Progress of Theoretical Physics 1974, 51, 77-81, 10.1143/ptp.51.77.
- Ettore Minardi; Minardi, E. Thermodynamics of High Temperature Plasmas. Entropy, 2009, 11, 124-221. Entropy 2009, 11, 457-462, 10.3390/e11030457.
 Encyclopedia
Encyclopedia
