You're using an outdated browser. Please upgrade to a modern browser for the best experience.
Please note this is a comparison between Version 2 by Conner Chen and Version 3 by Conner Chen.
ComparThed with an styrene butadiene rubber (SBR) sample without influence of the polarity of three plasticizer, the conductivity of s on the mechanically unloaded oil-extended SBR samples decreases by an order of magnitude. The polarity of the plasticizer shows hardly any influence because the plasticizers only affect the distribution of the filler clusters. Under static load, the stability of the filler network of four filled styrene butadiene rubber (SBR) compounds is investigated by the simultaneous mechanical and dielectric properties seem to be oil-dependentanalysis (DMA & DEA).
- plasticizer
- mechanical stability
1. Introduction
Plasticizers are a widely used additive in rubber compounds [1][2][3][4][1,2,3,4]. They are particularly important and, as the third-highest ingredient in terms of content level, come in right after rubber and fillers. As processing aids, the plasticizers are added in different concentrations in order to impart rubber products with the desired elastic properties in the operating temperature range [5][6][7][8][9][10][5,6,7,8,9,10].
As a fluid component, the plasticizer migrates in the rubber matrix and its macromolecules are integrated into the polymer chains through intermolecular interactions. Consequently, the intermolecular forces of the polymer chains and the number of free valences in the three-dimensional structure are reduced. The internal space between the polymer chains is thus larger, and the free volume that allows the polymer chains to flow above their glass transition temperature increases [11][12][13][14][15][11,12,13,14,15]. This new conformation of the polymer chains, in turn, increases their mobility and enhances the filler distribution in the rubber mixture [16][17][18][19][20][21][16,17,18,19,20,21]. Above a certain percolation threshold, a filler network is formed that reinforces the rubber compounds and provides the necessary mechanical stability [16][17][16,17]. This applies to both the carbon-based fillers such as carbon black and silica [18][19][20][21][18,19,20,21]. Indeed, the plasticizer type strongly affects the mechanical properties of rubber products due to a shift in the glass transition temperature. Consequently, the strain, the mechanical stress, the modulus of elasticity and the damping behavior change [22][23][24][22,23,24].
Furthermore, the dielectric properties of rubber samples filled with electrically conductive filler depend on the structure of its filler network [25][26][27][28][29][30][31][32][25,26,27,28,29,30,31,32]. This applies to filler networks made of electrically conductive fillers such as carbon-based carbon black or hybrid filler networks, provided that at least one electrically conductive filler is present [25][26][27][28][25,26,27,28]. The non-conductive component is mainly used because of its excellent mechanical reinforcement, as is the case with silica used in dynamic systems such as car tires [29][30][31][32][29,30,31,32]. Aloui et al. have shown that mechanically induced changes in the structure of the electrically conductive filler network have a direct impact on dielectric mechanisms such as charge transport and polarization [33][34][33,34]. These, in turn, have consequences for the dielectric constant and the dielectric conductivity of rubber samples [35][36][37][38][39][35,36,37,38,39].
The direct relationship between mechanical and dielectric properties makes simultaneous mechanical and dielectric analysis of rubber samples filled with electrically conductive filler an outstanding technique for opening up new horizons in evaluating the microstructure dynamics of rubber materials under mechanical load and hence reproducing authentic situations from operation modes [40][41][42][43][40,41,42,43]. In addition to quality measurements on test samples, examinations on installed end products can also be guaranteed if sensors are installed to record the current material properties during use and to monitor them in the subsequent step. Mainly the dielectric properties are used as a response to the mechanical load [44].
2. Excursus: Dielectric Relaxation in Elastomers
ε∗(ω)=εinf+Δε1+(iωτ)α with 0<α≤1
ε′(ω)=εinf+Δε⋅1+(ωτ)αcos[απ2]1+2(ωτ)αcos[απ2]+(ωτ)2α
ε′′(ω)=σdcωε0+Δε⋅(ωτ)αsin[απ2]1+2(ωτ)αcos[απ2]+(ωτ)2α
3. Compound Preparation
Figure 1.
Structural formula of plasticizers SN400, MES and DAE.
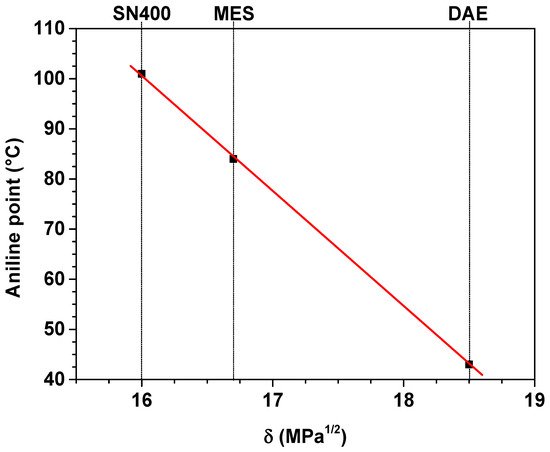
Figure 2.
Aniline point and solubility parameter of the plasticizers.
Table 1.
Compound formulation in phr.
| No Oil | SN400 | MES | DAE | |
|---|---|---|---|---|
| SBR 1502 | 100 | 100 | 100 | 100 |
| N 330 | 60 | 60 | 60 | 60 |
| SN400 | - | 20 | - | - |
| MES | - | - | 20 | - |
| DAE | - | - | - | 20 |
| ZnO | 2.5 | 2.5 | 2.5 | 2.5 |
| Stearic acid | 1 | 1 | 1 | 1 |
| TMQ | 1 | 1 | 1 | 1 |
| 6PPD | 1 | 1 | 1 | 1 |
| CBS | 1.8 | 1.8 | 1.8 | 1.8 |
| DDTD | 0.2 | 0.2 | 0.2 | 0.2 |
| Sulphur | 1.5 | 1.5 | 1.5 | 1.5 |
The antioxidants 2,2,4-Trimethyl-1,2-dihydrochinolin (TMQ) and N-(1.3-Dimethylbutyl)-N′-phenyl-p-phenylenediamine (6PPD) were added at a concentration of 1 phr. The samples were sulfur-vulcanized. In addition to sulfur, the vulcanization accelerators N-cyclohexyl-2-benzothiazolesulfenamide (CBS) and Dimethyldiphenylthiuram disulfide (DDTD) were used.
