Encyclopedia
Please note this is a comparison between Version 2 by Amina Yu and Version 1 by Andreas Stylianou.
Collagen fibrils are dissected from collagen rich tissues, such as rat tail tendons. Tissue samples such as tendons are sectioned with scalpels and washed with deionized water or phosphate-buffered saline. Subsequently, bundles of collagen fibers are collected with tweezers and then deposited on clean substrates such as microscope glass slides.
- Atomic Force Microscopy (AFM)
- nanomechanical properties
- mechanical heterogeneous samples
- biological samples
- nanoscale
1. Introduction
Collagens are the major proteins in mammals (almost 30% of the total mammalian protein) [1]. The collagen superfamily consists of more than 50 collagens and collagen-like proteins, with the fibrous collagens, including collagen I, tending to be of most interest [2,3][2][3]. Collagen molecules are composed of three polypeptide chains that contain the repeating amino acid motif (Gly-X-Y), where X and Y can be any amino acid. The molecules of collagen type I, which is the major protein in the extracellular matrix [4] form rod-shaped triple helices assembled to form fibrils [4], which are then properly aligned in order to form bundles and fibers [4]. A unique and interesting characteristic of collagen fibers is the fact that collagen molecules are packed in a quarter-staggered fashion so as to form the D-band periodicity, which is a repeating banding pattern of about 67 nm, depending on the tissue (Figure 1) [1]. Collagen fibrils are the elementary building blocks in many tissues and organs, and play a crucial role in a number of pathological conditions [1]. Furthermore, collagen is considered one of the most widely used biomaterials, mainly due to its unique properties such as the ability of self-assembly, bio-compatibility, bio-degradability, and non-toxicity [5].
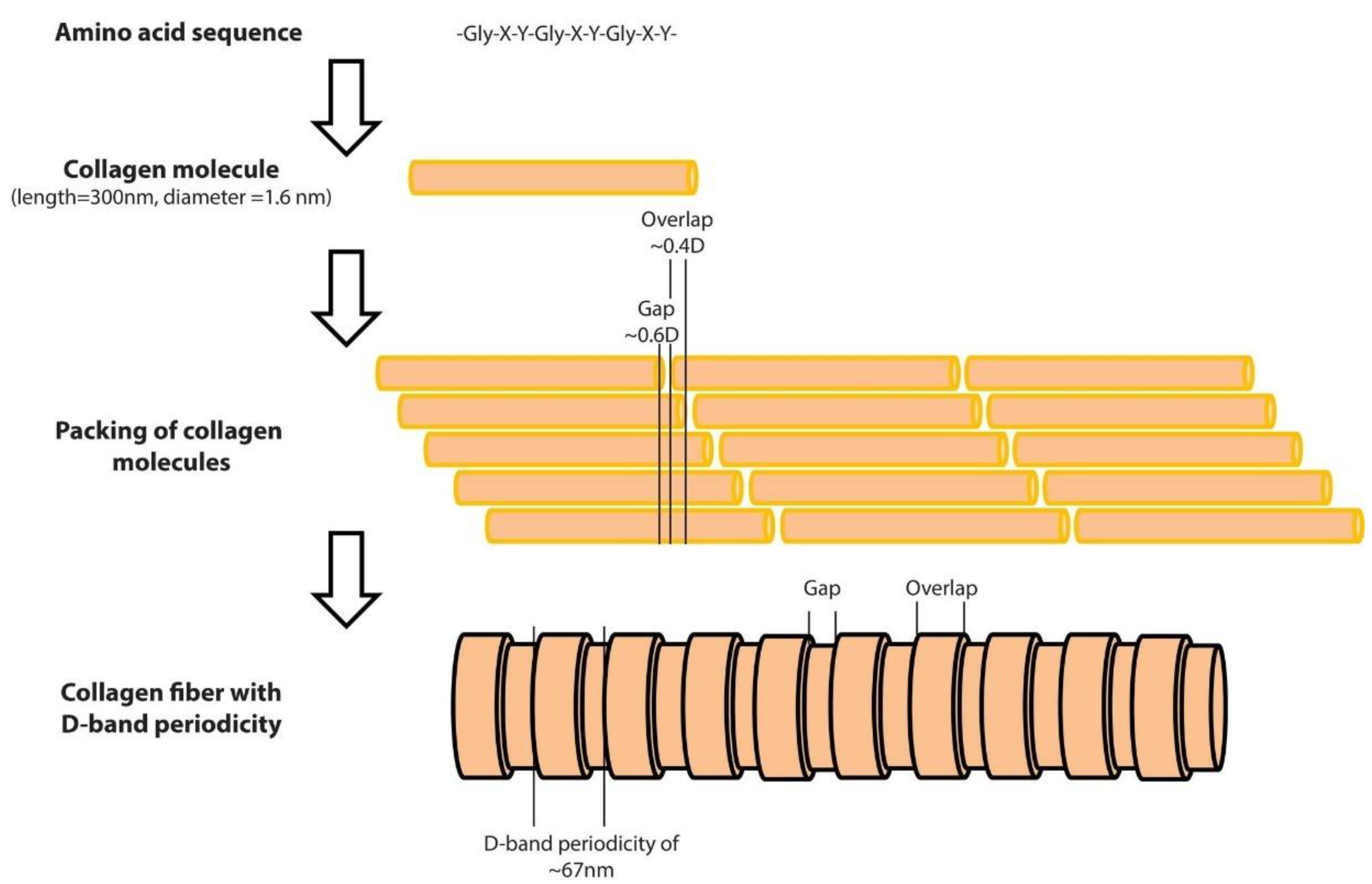
Figure 1. The structure of a collagen fiber type I, from the amino acid sequence to the D-band periodicity. As presented at the top of the figure, the collagen molecules consist of three amino acid chains. The length of the collagen molecule is 300 nm, while its diameter is 1.6 nm. The amino acid chains form rod-shaped triple helices which are assembled to form collagen molecules. Molecules are packed in a quarter-staggered fashion in order to form fibrils with a pattern that is known as the D-band periodicity. This pattern consists of overlapping and gap regions; it is a repeating banding pattern of about 67 nm as shown at the bottom of the figure.
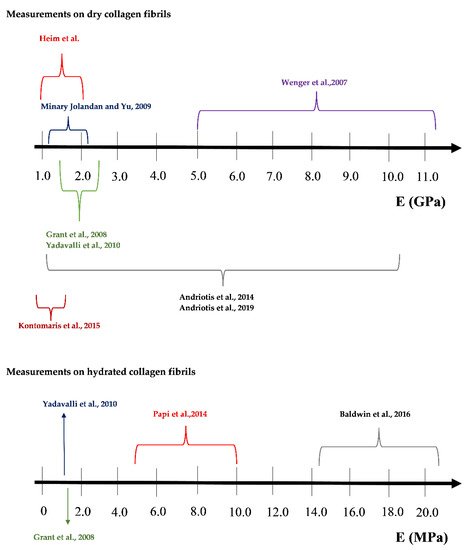
The mechanical properties of individual collagen fibrils have been extensively explored during the last decades using various methods such as Brillouin spectroscopy [6[6][7],7], force spectroscopy [8], X-ray data [9], microelectromechanical systems (MEMS) [10[10][11][12],11,12], steered molecular dynamics simulation of tropocollagen-like molecules [13], and Atomic Force Microscopy (AFM) nanoindentation [14,15,16][14][15][16]. The AFM nanoindentation became the leading technique regarding the characterization of biological samples at the nanoscale due to its great potential to be employed in real clinical activities. In particular, the nanomechanical characterization of individual collagen fibrils at the nanoscale has been related to new methods for the accurate and early diagnosis of cancer [17] and osteoarthritis [18]. Thus, significant scientific interest towards the accurate determination of collagen fibrils Young’s modulus using AFM nanoindentation has arisen during the last two decades. However, the reported values of Young’s modulus in the literature vary significantly. In particular, Grant et al. used Hertzian mechanics (sphere–sphere contact) to calculate the Young’s modulus of type I collagen fibrils obtained by bovine Achilles tendon [19]. The Young’s modulus resulted in 1.9 ± 0.5 ±
0.5 GPa for dry fibrils and in 1.25 ±
GPa for dry fibrils and in 1.25 ± 0.1 MPa for fibrils in buffer solution [19]. Wenger et al. calculated the Young’s modulus of dry collagen type I fibrils from rat-tail tendon using the Oliver and Pharr analysis (sphere–elastic half space interaction) [20]. Their results were in the range of 5–11.5 GPa [20]. Heim et al. performed AFM nanoindentation experiments on collagen type I fibrils in air environment obtained from Cucumaria frondosa [21]. The Young’s modulus resulted in 1–2 GPa. The contact mechanics model in their analysis was the Hertz model (sphere–cylinder contact) [21]. Minary-Jolandan and Yu determined the Young’s modulus on overlapping (~2.2 GPa) and gap regions (~1.2 GPa) on dry collagen fibrils (type I) obtained by bovine Achilles tendon using Hertzian analysis (sphere–cylinder contact) [22]. Yadavally et al. used collagen type I from calf skin in their experiments. Using Hertzian analysis (sphere-sphere contact), they calculated the Young’s modulus equal to 1.9 ± 0.5 GPa for dry and 1.2 ± 0.1 MPa for hydrated collagen fibrils [23]. Andriotis et al. performed experiments on dry collagen type I fibrils from wild type mouse tail tendon (7.0 ± 1.5 GPa using Hertzian analysis, sphere-sphere contact and 9.4 ± 1.7 GPa using the Oliver and Pharr method), from rat tail tendon (3.2 ± 1.1 GPa, Oliver and Pharr analysis) and from human bronchial biopsies (6.6 ± 0.7 GPa, Oliver and Pharr analysis) [24]. Kontomaris et al. tested collagen type I fibrils from bovine Achilles tendon in air environment and the results were 0.9–1.5 GPa [25]. For their analysis the Oliver and Pharr model regarding the interaction of a sphere with an elastic half space was employed. Andriotis et al. used collagen from tail tendon of a two-month old mouse, and the results were 1–10 GPa [26] using the Oliver and Pharr method.±0.1
Papi et al. used the PeakForce Quantitative Nanomechanical Property Mapping (PFQNM) to characterize the nanomechanical properties of collagen fibrils in sclera (type I collagen is the predominant form of collagen in sclera) [27]. The model that was used for processing the force curves was the Derjaguin–Muller–Toporov (DMT) model [27] and the results were in the range 5–10 MPa. Baldwin et al. presented Young’s modulus maps of normal and kinked hydrated collagen fibrils [28]. In case of normal fibrils the Young’s modulus resulted in 17.3 ± 3.9 MPa [28]. TheIt analysis was performed using Sneddon’s model [28]. Interesting research has been also applied to collagen rich tissues. Kazaili et al. measured Young’s modulus of 5 μm thick corneal sections and the Young’s modulus resulted in the range 2 to 2.45 GPa [29]. For their analysis, the PFQNM mode was used in air environment [29].
The range of Young’s modulus values in the literature is also presented in Figure 2.
As already mentioned, the accurate nanomechanical characterization of individual collagen fibrils is crucial in order to use AFM as a diagnostic tool for various diseases [16,17,18][16][17][18]. Thus, in this paper, the reasons for such an extended range of Young’s modulus values in the literature are are presented and discussed. The variability of the Young’s modulus values of single collagen fibrils is related to the dehydration state of the fibril [24], to errors in data processing [30], and to uncertainties regarding the AFM probe calibration procedures (the determination of the spring constant of the AFM cantilever and the exact shape and size of the tip) [20]. Apart from the water concentration within the fibril, it is significant to note that even for dry collagen fibrils the range of the resulting Young’s modulus values is extremely broad (even when using the exact same experimental conditions). In particular, the range of Young’s modulus values regarding individual dry collagen fibrils is 0.9–11.5 GPa [20,24,25,26][20][24][25][26]. ThisIt reviewwas focuses on the methods that are being used for the processing of the force–indentation data in indentation experiments in air environment, and analyzes the factors that lead to such an extended variation in the Young’s modulus values. It is known that environmental conditions affect the results of AFM data on biological samples (e.g.for example, humidity affects the tip–surface interaction and also alters the sample’s mechanical properties) [31]. In addition, according to Quigley et al., a dehydration–rehydration cycle leads to a decrease in radial modulus [32]. Thus, in order to reduce the factors that affect the results, the discussion in this paper concerns only measurements performed in air. Finally, new research approaches are proposed towards an accurate gold standard nanomechanical characterization method in the future.
2. The Mechanical Heterogeneity of Collagen Fibrils
Firstly, it must be clarified that collagen fibrils are not linear elastic solids. In fact, the diameter of each fibril changes along the axial direction (the collagen fibril consists of an alternating gap and overlapping regions), with a highly reproducible D-band periodicity of approximately 67 nm [22,25][22][25]. The aforementioned heterogeneity results also in a mechanical heterogeneity. In particular, Minary-Jolandan and Yu performed experiments on type I collagen fibrils prepared from bovine Achilles tendon and calculated the Young’s modulus on overlapping regions ~2.2 GPa, while on gap regions ~1.2 GPa [22]. TheIt analysis was performed using the theory regarding the sphere–cylinder purely elastic interaction [22]. Kontomaris et al. reported similar results regarding the aforementioned mechanical heterogeneity. In their experiments (which w were also conducted on type I collagen from bovine Achilles tendon) theywas found a Young’s modulus of ~1.1 GPa for overlapping regions and ~0.9 Gpa for gap regions [25]. In tTheir analysis, the Oliver–Pharr method for elastic–plastic contact using a spherical indenter was used [25]. It is also significant to note that mechanical properties maps of collagen fibrils in terms of Young’s modulus have been previously presented [66][33]. The mechanical heterogeneity of collagen due to overlapping and gap regions is a significant factor related to the broad distribution of Young’s modulus values in the literature and is highly related to the dehydration state. In particular, Spitzner et al. investigated how the water influences the mechanical properties of individual type I collagen fibrils on the nanometer scale. In particular, the mechanical contrast between overalapping and gap regions is small in dry state, while in hydrated state the differences are significant [68][34].
