概率时间地理建模 | Encyclopedia MDPI

概率时间地理建模: Comparison

Please note this is a comparison between Version 1 by wang ai sheng and Version 2 by wang ai sheng.

The possibility of moving objects accessing different types of points of interest (运动物体在特定时间访问不同类型兴趣点（POI) at specific time is not always the same, so quantitative time geography research needs to consider the actual POI semantic information, including POI attributes and time information.）的可能性并不总是相同的，因此定量时间地理学研究需要考虑实际的POI语义信息，包括POI属性和时间信息。

time geography
space–time trajectory
POI
semantic information
position probability

Introduction

1. 引言

Probabilistic time geography holds that the possibility of moving objects accessing reachable position points is not always the same [1], and is related to the space-time distance between space-time anchor points. The first law of geography states that the closer the space distance between geographic entities, the stronger the correlation. Similarly, in time geography, the lower the time cost to the space-time anchor, the more likely moving objects are to appear. This means that in quantitative in time geography analysis, the closer the time and space distance from the determined space-time anchor point, the greater the probability of moving objects appearing. Therefore, we expect a quantitative measure model of position probability in time geography.

概率时间地理学认为，移动物体进入可到达位置点的可能性并不总是相同的[1]，并且与时空锚点之间的时空距离有关。地理第一定律指出，地理实体之间的空间距离越近，相关性越强。同样，在时间地理学中，时空锚点的时间成本越低，移动物体出现的可能性就越大。这意味着，在定量时间地理分析中，时空距离确定的时空锚点越近，运动物体出现的概率就越大。因此，研究人员期待在时间地理学中建立位置概率的定量测量模型。

Downs introduced a position probability measurement model that considers space-time distances, known as Time-Geographic Density Estimation (TGDE) [2]. From the perspective of historical experience data, TGDE measures the access probability of the reachable point to be measured by calculating the time cost between two adjacent anchor points in time distance from the reachable point. This method combines kernel density estimation and time geography, and follows the first law of geography to construct a time geographic density estimation model based on geographic ellipse. Consequently, the space-time uncertainty of moving objects between any two time adjacent space-time anchor points can be expressed as a probability density cloud. Such probability density cloud can be used in two aspects: First, kernel density estimation [3], which is the superposition of all density clouds on the space-time trajectory, is used to express the home range estimation of the moving object in the space-time trajectory region; The other is interaction analysis [4], that is, the intersection analysis of density clouds in the same time period, which is used to measure the interaction probability of two moving objects in the space-time trajectory period. Such a probability density cloud modeling method is effective in homogeneous space [5].

Downs引入了一种考虑时空距离的位置概率测量模型，称为时间地理密度估计（TGDE）[2]。TGDE从历史经验数据的角度出发，通过计算两个相邻锚点与可到达点的时间距离来衡量待测点的访问概率。该方法将核密度估计与时间地理学相结合，遵循地理第一定律，构建了基于地理椭圆的时间地理密度估计模型。因此，任意两个相邻时空锚点之间移动物体的时空不确定性可以表示为概率密度云。这种概率密度云有两种使用方式：一是核密度估计[3]，即时空轨迹上所有密度云的叠加，用于表示运动物体在时空轨迹区域的原点距离估计;另一种是相互作用分析[4]，即同一时间段内密度云的交集分析，用于测量两个运动物体在时空轨迹周期内的相互作用概率。这种概率密度云建模方法在均匀空间中是有效的[5]。

However, up to this point, the position probabilistic modeling method of probabilistic time geography still stays in the homogeneous space, only considering the time and space information of the position point, but ignoring the semantic attribute information. This article will make two important extensions. Firstly, the distribution of position probability is extended from feedback to mutual feedback. The classical method of position probability allocation is the calculation of the time cost from the space-time anchor point to the measured point, which is a one-way feedback, only considering the ability constraint of whether the moving object has enough ability to reach the measured point, which refers to the joint constraint of the moving speed and time of the moving object. However, the semantic information of the point to be measured also has an impact on the access of the moving object, such as the moving object will not access the supermarket closed after 22:00 or the access probability is 0, which belongs to the ability constraint. On the basis of considering the time cost of the point to be measured, we will consider the mechanism of the semantics of the point to be measured on the access probability, and form the “Mutual feedback model” of the anchor point and the point to be measured. Then, we also extend the position probability model of homogeneous space to heterogeneous space, especially urban space. In urban space, activity places are often marked with Point of Interest (POI) on the map, so POI can be used to express the space-time position points accessed by moving objects, and in different periods, different types of POI have different attraction to moving objects. POI position points that contain semantic attribute information can have an impact on access to moving objects, such as on weekdays when a child accesses school in the morning instead of going to the park. Therefore, we will have a method for calculating the probability of position in heterogeneous space.

然而，到目前为止，概率时间地理学的位置概率建模方法仍然停留在齐次空间中，只考虑了位置点的时间和空间信息，而忽略了语义属性信息。本文将进行两个重要的扩展。首先，将持仓概率的分布从反馈扩展到相互反馈;位置概率分配的经典方法是计算从时空锚点到被测点的时间代价，这是单向反馈，只考虑运动物体是否有足够的能力到达被测点的能力约束，是指运动物体的运动速度和时间的联合约束。但是，被测点的语义信息也会对移动物体的出入产生影响，例如在22：00后移动物体关闭超市或出入概率为0的情况下，属于能力约束。在考虑待测点的时间成本的基础上，考虑待测点的语义对访问概率的机理，形成锚点与待测点的“相互反馈模型”。然后，将同质空间的位置概率模型推广到异质空间，特别是城市空间。在城市空间中，活动场所常在地图上标注兴趣点（POI），因此POI可以用来表示运动物体所访问的时空位置点，在不同时期，不同类型的POI对运动物体具有不同的吸引力。包含语义属性信息的 POI 位置点可能会影响对移动对象的访问，例如在工作日，当孩子早上上学而不是去公园时。因此，我们将有一种计算异构空间中位置概率的方法。

The rest of this article is organized as follows. Section 2 explains the relevant basis and background of this study, including the related concepts of time geography, the introduction of POI and POI in time geography. Section 3 describes in detail the probabilistic time geographic modeling method considering POI semantics, including POI position probability modeling, POI time weight modeling, POI type weight modeling and POI access probability modeling. Section 4 describes the research process of this paper, that is, using the model proposed in Section 3 to calculate the POI access probability considering POI semantics. Section 5 summarizes the research in this paper and discusses potential extensions.

2. 时间地理

Related work

时间地理学研究时空约束下运动物体的时空不确定性[9,10,11]。时空约束在时间地理学中可分为能力约束、权限约束和组合约束[8]。能力约束是指运动物体进行各种活动的能力受到限制，如运动物体具有自己的最大运动速度;权限约束是指移动物体在开展活动时受到法律、习俗和社会规范的限制，例如活动场所仅在营业时间开放时;组合约束是指在进行活动时，运动物体与其他运动物体或活动场所的相互作用，例如运动物体前往餐厅型POI位置点用餐[8]。时间地理学的研究分为定性研究和定量研究两大类。

This section provides the basic theory of time geography and probabilistic time geography, and discusses the mechanism of interaction between POI and moving objects. Time geography provides an important theoretical framework for measuring the space-time uncertainty of potential access to space-time position points for moving objects [6,7]. Probabilistic time geography is an extension of (classical) time geography [8], paying more attention to the possibility of space-time position points being accessed. These theories will be closely integrated in the work of this paper. In this paper, the space-time semantic information of POI is introduced to reflect the possibility of moving objects accessing POI, that is, the POI position probability measure considering the semantic information.

在定性研究中，时间地理学主要采用三个关键概念：时空轨迹、时空棱镜和潜在路径区域（PPAs）来描述时空不确定性[12,13,14,15]。时空轨迹可以看作是地理位置点的时间序列，可以通过STP在时间地理上表示：

S T P = l 0 , l 1 , … , l i , … , l n

其中

S T P

表示时空路径（图 1）;

n

为轨迹点总数;

l_{i}

i 为运动物体的

i t h

轨迹点，可描述为

(x_{i}, y_{i}, t_{i})

、

(x_{i}, y_{i}),

和

t_{i},

分别表示轨迹点的二维空间位置和时间戳。轨迹点有时称为锚点或控制点。STP表示运动物体的方向运动趋势，而时空棱镜可以表示运动不确定性的时空边界。时空棱镜表示在起点和终点、能力约束（如最大移动速度）和权限约束（如地理环境）的共同约束下，运动物体可以到达的所有可能的时空点的集合（图1a）。时空棱镜在平面空间中的足迹或投影称为PPA，在均匀空间中，它可以描述为以两个锚点

{(l}_{i - 1}, l_{i})

为焦点的椭圆，并且

v_{m a x} \cdot (t_{i} - t_{i - 1})

作为主轴：

P P A i = x | | x − l i − 1 | | + | | l i − x | | ≤ t i − t i − 1 · v m a x

其中 P P A i 表示

i th

轨迹段上的 PPA，x 表示

x

{P P A}_{i}

{P P A}_{i}

，

| | - | |

表示二维空间中的欧几里得距离，t i−

t_{i} - t_{i - 1}

是每对轨迹锚点的预算时间

(l_{i - 1}, l_{i})

，

v_{m a x}

是运动物体的最大移动速度。

2.1. Time geography

Time geography studies the space-time uncertainty of moving objects under space-time constraints [9-11]. Space-time constraints can be divided into ability constraints, authority constraints and combination constraints in time geography [8]. Ability constraint means that the ability of the moving object to carry out various activities is limited, such as the moving object has its own maximum moving speed; Authority constraint means that the moving object is restricted by laws, customs and social norms when carrying out activities, such as the activity place is only open during business hours; The combination constraint refers to the interaction between the moving object and other moving objects or activity places when carrying out activities, such as a moving object going to a restaurant-type POI position points for dining [8]. The research of time geography is divided into two categories: qualitative research and quantitative research.

In qualitative research, time geography primarily employs three key concepts: space-time trajectories, space-time prisms, and potential path areas (PPA) to describe space-time uncertainty [12-15]. Space-time trajectories can be viewed as time series of geographic position points, which can be represented in time geography through STP:

where, represents the space-time path (Figure 1), is the total number of trajectory points, and is the trajectory point of the moving object, which can be described as , and respectively represent the two-dimensional space position and time stamp of trajectory points. Trajectory points are sometimes called anchor points or control points. The STP expresses the directional movement trend of the moving object, while the space-time prism can express the space-time boundary of the moving uncertainty. The space-time prism represents the set of all possible space-time points that a moving object can reach under the common constraints of the starting and ending points, the ability constraints (such as the maximum moving speed) and the authority constraints (such as the geographical environment) (Figure 1a). The footprint or projection of the space-time prism in the plane space is called PPA, and in the homogeneous space can be described as an ellipse with the two anchor points as the focal point and as the major axis:

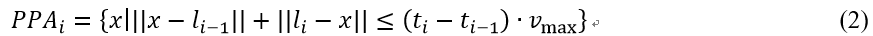
Where, represents PPA on the trajectory segment, represents any point in , represents the Euclidean distance in two-dimensional space, is the budget time of each pair of trajectory anchor points , and is the maximum moving speed of the moving object.

Figure

图 1.

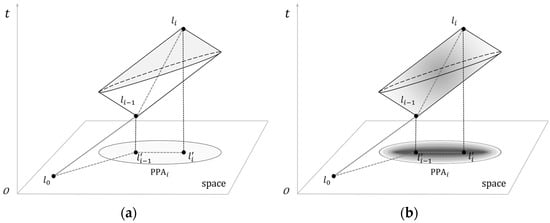
Basic tools of time geography: (a) space-time prism and PPA; (b) probabilistic space-time prism and probabilistic PPA.

时间地理学的基本工具：（a）时空棱镜和PPA;（b）概率时空棱镜和概率PPA。

In quantitative research, time geography assigns a position probability to each position point within a PPA or space-time prism to represent how likely it is that a moving object will access a certain reachable position at a given time. Therefore, the quantitative research of time geography is also called probabilistic time geography, which is a fusion of time geography and probability theory [8]. The basic idea is: in the PPA or space-time prism during a pair of trajectory anchor points, the position probabilities of moving objects accessing different position points at any time are not always equal, and the size of the probability value changes with the change of state information [16-18]. For any reachable position point, different position probabilities are typically assigned to the position points based on the time cost of reaching that point from the starting and ending points, often using distance decay kernel function models such as Brownian bridge and TGDE [7, 16, 19-21].

在定量研究中，时间地理学为 PPA 或时空棱镜中的每个位置点分配位置概率，以表示移动物体在给定时间访问某个可到达位置的可能性。因此，时间地理学的定量研究又称概率时间地理学，是时间地理学与概率论的融合[8]。其基本思想是，在PPA或时空棱镜中，在一对轨迹锚点出现时，运动物体在任何时候访问不同位置点的位置概率并不总是相等的，并且概率值的大小随着状态信息的变化而变化[16,17,18].对于任何可到达的位置点，通常根据从起点和终点到达该点的时间成本为位置点分配不同的位置概率，通常使用距离衰减核函数模型，如布朗桥和TGDE[7,16,19,20,21]。

The qualitative and quantitative research methods of time geography analyze the space-time uncertainty of moving objects and consider the three space-time constraints of time geography. These methods are valid in homogeneous space, especially when not distinguishing the semantic attributes of position points, such as the type of POI and time semantic information. However, differences in the attachment characteristics of space position points will produce different attractions for moving objects and change over time.

时间地理学的定性和定量研究方法分析了运动物体的时空不确定性，并考虑了时间地理学的三个时空约束。这些方法在齐次空间中是有效的，特别是在不区分位置点的语义属性时，例如POI的类型和时间语义信息。然而，空间位置点的附着特性的差异会对运动物体产生不同的吸引力，并随时间而变化。

2.2. POI

3. 兴趣点

POI refers to geographic position points on a map that are of interest to moving objects, with information including position, attributes (types), and time [22,23]. People's travel always has certain preference and purpose [24], which is why navigation and planning are essential in people's journeys. POI has gradually become the key search information for people's travel navigation [25], which is related to POI representing certain functions and needs, and therefore has become an important information for human activity analysis [26].

POI是指地图上移动物体感兴趣的地理位置点，其信息包括位置、属性（类型）和时间[22\u23]。人们的旅行总是有一定的偏好和目的[24]，这就是为什么导航和计划在人们的旅程中是必不可少的。POI逐渐成为人们出行导航的关键搜索信息[25]，它与代表某些功能和需求的POI有关，因此成为人类活动分析的重要信息[26]。

There are significant differences in the attractiveness of different types of POI to individuals. For example, restaurant POI may attract people to eat, tourist attractions POI may attract people to access, transportation facilities POI may affect people's living habits and travel choices, and shopping consumption POI may affect people's consumption behaviors and so on. The space distribution of POI positions usually has a certain aggregation [27]. For example, a large number of various service types of POI are gathered in urban business districts, so as to attract nearby consumers to travel.

不同类型的POI对个人的吸引力存在显着差异。例如，餐厅POI可能吸引人们就餐，旅游景点POI可能吸引人们进入，交通设施POI可能影响人们的生活习惯和出行选择，购物消费POI可能影响人们的消费行为，等等。POI位置的空间分布通常具有一定的聚集性[27]。例如，在城市商圈聚集了大量各种服务类型的POI，从而吸引附近的消费者出行。

The same type of POI will have different attraction to individuals at different time periods [28-30]. For example, the attraction of restaurant POI to individuals at meal time is much higher than that at other time; the attraction of corporate POI to individuals is greater on working days; and the attraction of tourist attraction POI is generally greater on holidays. POI can better reflect the space-time activity behavior of urban residents, and has a strong explanatory power for the behavior of moving objects accessing the space-time position points. Therefore, it is necessary to consider the actual POI semantic information in the quantitative calculation of position probability.

相同类型的POI在不同时间段对个体的吸引力不同[28,29,30]。例如，餐厅POI在用餐时间对个人的吸引力远高于其他时间;在工作日，企业利益向标对个人的吸引力更大;旅游景点POI的吸引力在节假日普遍更大。POI能够较好地反映城市居民的时空活动行为，对运动物体进入时空位置点的行为具有较强的解释力。因此，在位置概率的定量计算中，有必要考虑实际的POI语义信息。

2.3. POI in time geography

4. 时间地理学中的 POI

Based on the analysis above, it can be concluded that the attractiveness of POI has an impact on the access of moving objects. The quantitative description of this influence will affect the calculation of position probability. The activity of an individual moving object usually includes various POI position points, and there is space-time proximity between the space-time trajectories of moving objects and the accessed POI positions. Existing studies have analyzed the attractiveness of POI from two aspects.

基于上述分析，可以得出结论，POI的吸引力对运动物体的进入有影响。对这种影响的定量描述将影响仓位概率的计算。单个运动物体的活动通常包括各种POI位置点，并且运动物体的时空轨迹与访问的POI位置之间存在时空接近。现有研究从两个方面分析了POI的吸引力。

Zeng proposed a method to analyze the change of attraction of urban POI based on taxi track [31]. He calculated the attractiveness and distribution of different categories of POI in different time periods according to the proximity of taxis staying near different categories of POI in different time periods, and then statistically analyzed the law of citizen activities. He believes that the attractiveness of POI is related to its attributes, position, and time period, and that existing methods ignore the influence of time and category on the attractiveness of POI. This method evaluates the attractiveness of POI through statistical analysis, and presents the distribution of POI in a large range of global attractiveness, but ignores the semantic information of POI itself and the personalized attractiveness of POI to a single space-time trajectory. Moreover, this method requires a large number of independent trajectory data samples, and lacks a priori in calculating the probability distribution. Downs assigns access probabilities to space positions including POI positions through TGDE [2], and the access probability of POI positions reflects the attraction of the POI to moving objects. This method only considers the start and end points of space-time trajectories, without considering the time budget of corresponding activities at POI positions. This method also ignores the semantic information of POI, and lacks verification of the posterior probabilities of POI accesses based on empirical data.

曾先生提出了一种基于出租车轨道的城市POI吸引力变化分析方法[31]。他根据出租车在不同时间段停留在不同类别POI附近的距离，计算了不同时间段不同类别POI的吸引力和分布，然后统计分析了公民活动的规律。他认为，POI的吸引力与其属性、位置和时间段有关，现有方法忽略了时间和类别对POI吸引力的影响。该方法通过统计分析来评价POI的吸引力，呈现POI在大范围全局吸引力中的分布，但忽略了POI本身的语义信息以及POI对单一时空轨迹的个性化吸引力。此外，该方法需要大量独立的轨迹数据样本，在概率分布计算上缺乏先验性。Downs使用TGDE[2]为包括POI位置在内的空间位置分配访问概率，POI位置的访问概率反映了POI对移动物体的吸引力。该方法仅考虑时空轨迹的起点和终点，而不考虑POI位置相应活动的时间预算。该方法也忽略了POI的语义信息，缺乏基于经验数据的POI访问后验概率验证。

The above research indicates that the influence of POI semantic information on the trajectory of moving objects is significantly different, and correspondingly, the possibility of moving objects on the same trajectory accessing POI with different attractiveness is also different. In time geography, on the one hand, the attraction of POI is an uncertainty measure of space-time position points, which is the probability of space-time position statistics through a large number of space-time trajectories. On the other hand, relative to the space-time trajectory, POI is the position point that the moving object can reach in space-time, and the access activity at the POI position point is also a part of the time budget between the anchor points of the space-time trajectory.

上述研究表明，POI语义信息对运动物体轨迹的影响存在显著差异，相应地，同一轨迹上的运动物体访问具有不同吸引力的POI的可能性也不同。在时间地理学中，一方面，POI的吸引力是时空位置点的不确定性度量，即通过大量时空轨迹进行时空位置统计的概率。另一方面，相对于时空轨迹，POI是运动物体在时空中可以到达的位置点，POI位置点处的访问活动也是时空轨迹锚点之间时间预算的一部分。

Therefore, the time geography based on space-time trajectory to predict space-time uncertainty needs to incorporate the semantic information of POI into the position probability distribution, including POI type and time factor, etc. At present, TGDE only considers the space position and the time cost from the position point to the two anchor points, without considering the difference of POIs in space positions. In this paper, a POI probability model considering the proximity of moving trajectories is proposed based on the known trajectory information and the space-time distance between the trajectory point and the POI. The model expresses the space-time uncertainty between known precise space-time position points based on the space-time constraints of moving speed and time limitations, and provides all the information, including time, space and semantic information, for this space-time uncertainty measurement, which avoids the error caused by single information. This model not only provides a theoretical basis for the quantification of position probability, but also provides a time geography support for the statistics of POI attraction. For example, in the space-time prism of a moving object, the trajectory that does not contain POI can be excluded when calculating the space-time trajectory of a moving object passing through POI.

因此，基于时空轨迹预测时空不确定性的时间地理学需要将POI的语义信息纳入位置概率分布中，包括POI类型、时间因子等。目前，TGDE只考虑了空间位置和从位置点到两个锚点的时间成本，没有考虑POI在空间位置上的差异。该文基于已知的轨迹信息以及轨迹点与POI之间的时空距离，提出了一种考虑运动轨迹邻近性的POI概率模型。该模型基于移动速度和时间限制的时空约束，表达了已知精确时空位置点之间的时空不确定性，为这种时空不确定性测量提供了包括时间、空间和语义信息在内的所有信息，避免了单一信息带来的误差。该模型不仅为位置概率的量化提供了理论依据，而且为POI吸引力的统计提供了时间地理支持。例如，在运动物体的时空棱镜中，在计算通过POI的运动物体的时空轨迹时，可以排除不包含POI的轨迹。