1. Blocks
Blocks are fundamental components of a blockchain database and serve as data structures that permanently store transaction data in a blockchain
[1]. A block stores a subset of all the latest transactions the network still needs to validate. After validating the data, the block is stored in a ledger. Subsequently, a new block is generated to accommodate the inclusion and verification of recent transactions. A block is a repository of data that, once written, is immutable and cannot be modified or deleted.
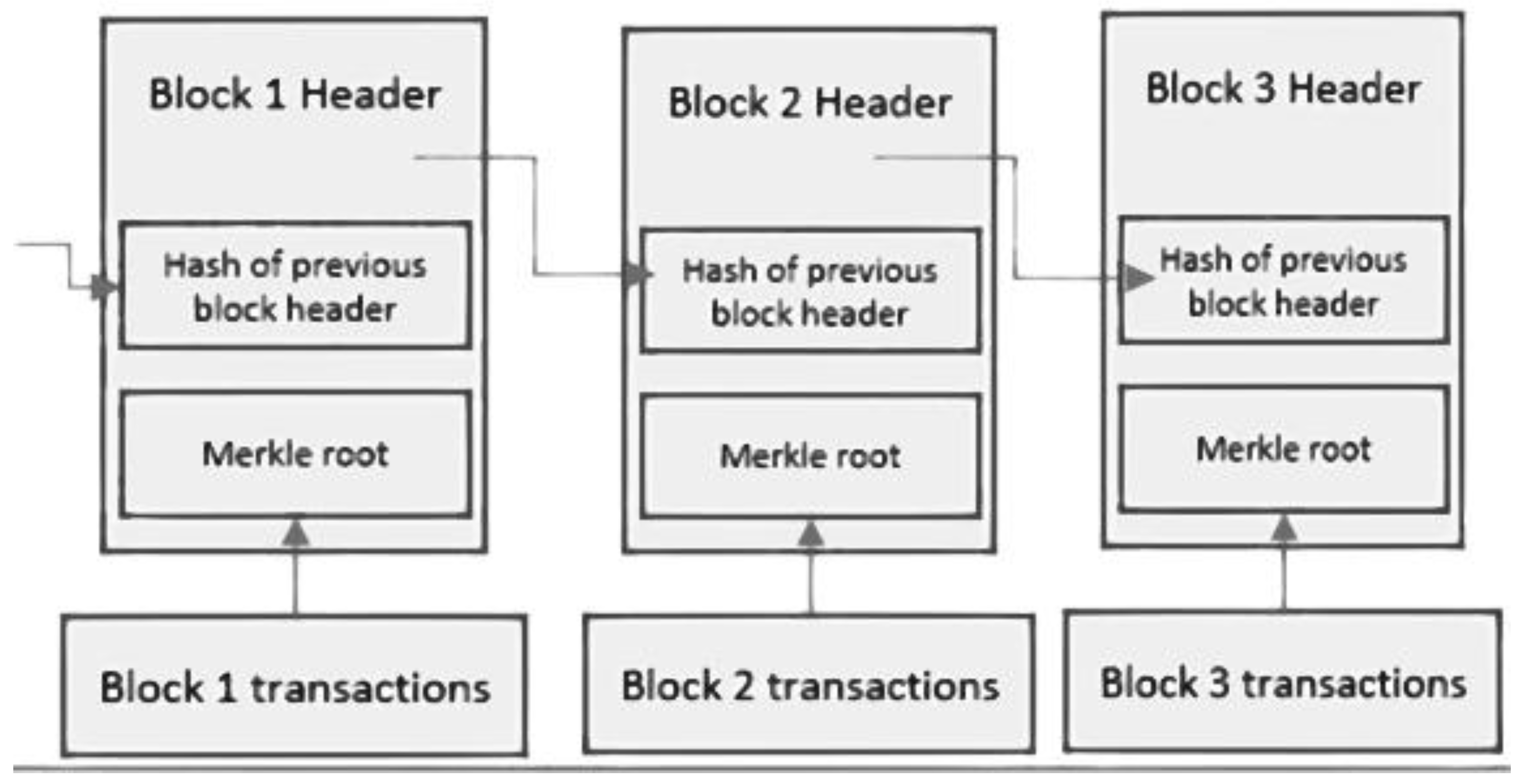
Figure 1 illustrates the general format of chained blocks, highlighting the following elements of information within a block.
Figure 1. General format of chained blocks.
- −
-
The term “magic number” refers to a numerical figure encompassing distinct characteristics and serving as an identifier for a single block inside the network.
- −
-
The block size parameter establishes a predetermined restriction on the size of a block, which restricts the amount of data that may be inputted.
- −
-
The block header is a component that contains pertinent information on the block.
- −
-
The transaction counter is a numerical value that indicates the total number of transactions in a given block.
- −
-
Transactions refer to a comprehensive batch of all recorded transactions in a particular block
[1].
2. Transactions
Blockchain transactions refer to transmitting data via a network of computers within a blockchain system. In a blockchain, interconnected nodes form a network that collectively maintains transactional data as replicated copies, often known as a digital ledger
[2].
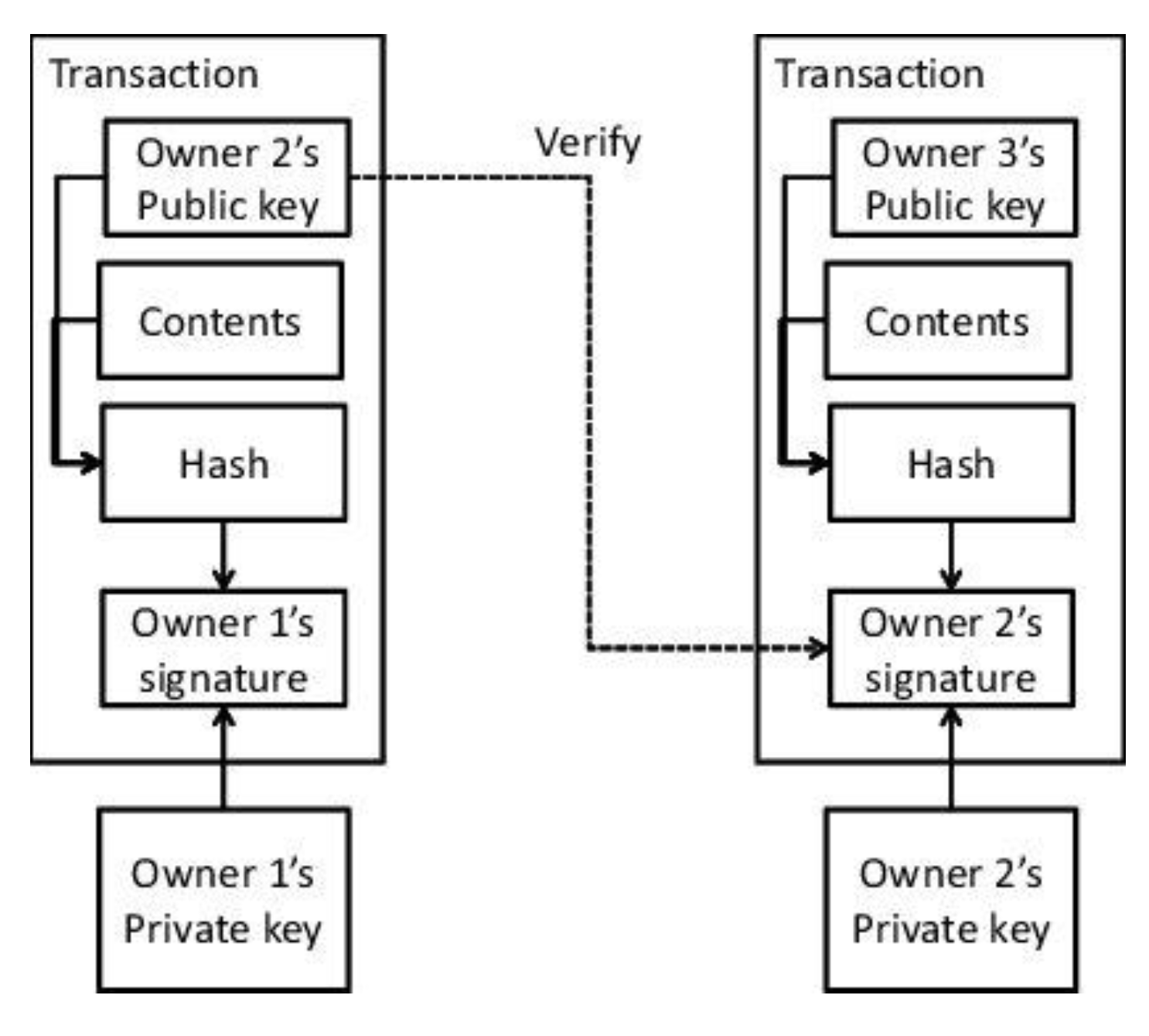
Figure 2 presents an example of a Bitcoin transaction, illustrating how data transmission is facilitated within such a network.
Figure 2. Example of a Bitcoin transaction.
3. Nodes
Blockchain nodes are integral components within a network, authorized to maintain a distributed ledger and act as communication hubs for various network responsibilities. While sharing foundational purposes, these nodes can be categorized into specific roles based on their functionalities.
One of the critical categories is the transaction-executing nodes. These nodes are primarily tasked with the validation and verification of the transactions. The core role of a blockchain node within this category involves ensuring the legitimacy of each sequential set of transactions, known as blocks. Among these, full nodes represent a significant subset. They retain and record all transactions within the blockchain in their storage and perform the critical function of validating blocks and transactions. In contrast, lightweight nodes, another form of transaction-executing node, have reduced storage requirements. They primarily focus on retrieving block headers for transaction verification and minimizing their data storage requirements.
Another pivotal category is the mining nodes. These nodes engage in the generation and integration of new blocks into the blockchain. The process begins when a miner attempts to add a new block of transactions to the blockchain and broadcasts this information across the network. It is important to note that the allocation of a block reward varies and is not a consistent feature of any blockchain network. Table 1 presents a comparison between transaction-executing nodes and mining nodes, highlighting the distinct roles and functionalities of these two key categories within a blockchain network.
Table 1. Comparison: transaction-executing nodes vs. mining nodes.
| Aspect |
Transaction-Executing Nodes |
Mining Nodes |
| Purpose |
Validate and relay transactions across the network. |
Add new blocks to the blockchain. |
| Primary Responsibility |
Ensure transactions comply with network rules. |
Collect, verify, and process transactions into blocks. |
| Reward System |
Do not typically receive cryptocurrency rewards. |
Receive rewards in the form of minted cryptocurrency. |
| Hardware Requirements |
Do not require significant computational power. |
Requires high computational power. |
| Storage |
It may or may not store the entire blockchain history. |
Store blockchain as the whole history and validation transactions. |
| Incentive |
Maintain network integrity for their applications. |
Monetary rewards for adding successful blocks. |
Nodes have the discretion to accept or reject a block based on their authenticity, which is determined by the validity of their signature and transaction
[3]. When a node receives a new block of transactions, it saves and stores its highest position within the current chain of blocks. In summary, nodes perform the following functions.
-
Nodes play a crucial role in the validation process of determining the legitimacy of a block of transactions because they have the authority to accept or reject it.
-
The nodes are responsible for preserving and retaining transaction blocks, including the entire transaction record in the blockchain.
-
The transaction history is distributed and propagated by nodes to other nodes, which may require synchronization with the blockchain
[1].
4. Game Theory
Game theory, a pivotal theoretical framework in disciplines such as economics and computer science, rigorously examines interactions characterized by formalized incentives and outcomes
[4]. This mathematical framework facilitates the analytical exploration of strategic interactions among rational entities, particularly in scenarios in which an agent’s payoff depends on the actions undertaken by other agents
[5].
Strategic decision making is especially salient within the blockchain domain. Blockchain networks can be conceptualized as intricate ecosystems of nodes (or agents) continuously engaging in decisions, such as validating a transaction or selecting transactions for block inclusion. These decisions are not autonomous but interdependent and influenced by other network participants’ potential actions and determinations. In this context, game theory provides the tools for modeling these multifaceted decisions, encompassing all network entities’ potential strategies and actions
[6]. This is crucial for anticipating node behavior when confronted with choices that can invariably impact rewards or overarching network security.
In a blockchain ecosystem, the application of game theory is invaluable for modeling and analyzing the strategic interactions of nodes. This includes decision making, strategies, and payoffs, particularly in an environment with adversarial nodes. The incorporation of game theory into blockchain networks requires a thorough assessment of node strategies, the extent of information accessible, and the ensuing payoffs. It is noteworthy that peers’ strategies and actions often modulate these payoffs. The game-theoretic approach provides a mathematical framework for modeling and analyzing strategic interactions among rational entities.
In the context of the proposed framework, nodes aim to optimize their rewards by adjusting the values within their trust matrices. However, these adjustments are not arbitrary. They are guided by rules and strategies that consider the actions of the other nodes. The application of game theory ensures that no single method dominates, preventing any node from accruing excessive rewards unfairly. Furthermore, nodes that consistently exhibit cooperative behavior or uphold the integrity of the network are more likely to be rewarded, thereby ensuring fairness in the reward distribution.
A theoretical game model is constructed, wherein the executors within a blockchain network are conceptualized as players. This model inherently aligns with the characteristics of cooperative games. Such an alignment is predicated on the rationale that nodes that function as collaborative agents are incentivized to maximize their reward outcomes collectively. Furthermore, this game is classified as a non-zero-sum game. This classification stems from the principle that rewards accrued by any individual node do not preclude the distribution of the gains among the other participating nodes. The non-zero-sum nature of the game intrinsically fosters a cooperative dynamic among the nodes as they endeavor to optimize their collective earnings while facilitating transaction execution and broadcast.
Moreover, the game is delineated as perfect information. This delineation is based on the premise that each node possesses comprehensive knowledge of the actions undertaken by its counterparts. Such transparency is achieved via a truth matrix serving as a repository of all player actions, enabling informed decision-making processes. Given that this decision-making process and strategic evolution occur over multiple rounds, the game can be conceptualized as infinite.
5. Nash Equilibrium
The Nash Equilibrium, a foundational concept in game theory introduced by John Nash, represents a state in a strategic interaction where no player can unilaterally change their strategy to achieve a better outcome, given the other players’ strategies. In more formal terms, a strategy set constitutes a Nash Equilibrium
[7] if no player can obtain a higher payoff by deviating from their strategy, whereas other players keep their strategies unchanged. In blockchain networks, Nash Equilibrium can provide insightful perspectives regarding the stability of the strategy adopted by nodes. For instance, in a scenario where nodes decide whether to validate and broadcast transactions or act maliciously or honestly, a Nash Equilibrium would occur if no single node could unilaterally change its strategy (e.g., from honest to malicious or vice versa) to achieve a higher payoff, given the strategy adopted by the other nodes.
Thus, analyzing and ensuring scenarios in which honest behavior and cooperation among nodes form a Nash Equilibrium becomes pivotal, ensuring the robustness and security of the blockchain network among rational self-interested nodes. The proposed framework’s primary objective is to engineer systemic convergence where all participating nodes within the blockchain network attain a Nash Equilibrium state. This desired equilibrium state underscores a strategic orientation wherein the actions and behaviors of the nodes collectively gravitate towards optimizing the gains for the entire network. Underpinning this strategic alignment is the principle that engaging in cooperative behavior is each node’s most advantageous course of action. This cooperative dynamic was facilitated and reinforced by a meticulously designed system of rewards and penalties.
The game’s rules are structured to guide the nodes towards this equilibrium. The system is designed to incentivize collaborative efforts and discourage actions detrimental to the network’s collective interests.
6. Graph Modeling of Blockchain Nodes and Researchers' Game Framework
Blockchain networks consist of several nodes engaging in interactions and reaching choices guided by self-interests. In the initial phase of developing researchers' framework, it is essential to construct a mathematical model to facilitate a deeper understanding of the interactions among nodes within the blockchain network. Researchers chose to represent the network using a graph-based model owing to graphs’ versatile properties and structural intricacies. Specifically, researchers opted for an undirected graph to illustrate node communication dynamics effectively. Furthermore, the representation of the graph in a matrix form simplifies its depiction and enhances its integration into researchers' algorithmic constructs. Subsequent stages involve incorporating this matrix into the strategic game, meticulously formalized in the forthcoming chapter. This integration enables nodes to make informed decisions in each round and adjust their trust matrices accordingly. The final step involves the implementation of an incentive mechanism designed to reward nodes proportionally based on their honest contributions and overall participation in the network. Figure 3 provides a high-level overview of this entire process, illustrating the key stages and how they interconnect within researchers' framework.
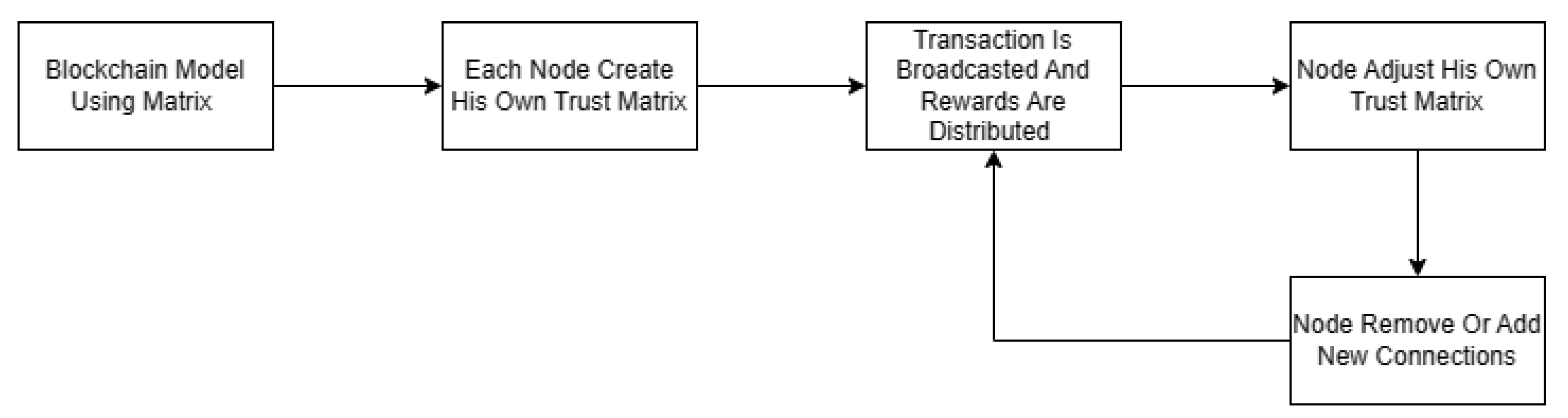
Figure 3. High-level overview of researchers' process.
7. Game Framework and Rules
In the initial phase of researchers' research, researchers focused on conceptualizing interactions within a blockchain network using graph theory and matrix representations. This part aims to construct a strategic game, facilitating nodes to adjust their trust matrix and make strategic decisions over successive rounds. These decisions include creating or removing connections with other nodes based on evolving strategies. Researchers begin by defining the critical elements of the framework as follows.
▪ Set of Players: This is represented by a finite set of nodes within the graph, denoted as 𝑁={1,2,…,n}, where each node is a player in the game. These players can exhibit malicious or honest behaviors depending on their chosen strategies. To capture the dynamic nature of the network and align with real-world scenarios, a node is permitted to switch strategies across rounds, thus enabling both cooperative and non-cooperative behaviors.
▪ Actions/Strategies: Nodes have two fundamental types of actions. The first relates to handling transactions or messages, where a node decides to either forward it to the next node or refrain from doing so, essentially choosing to cooperate. This can be represented by a binary sequence, such as {00011…00}. For simplicity, researchers initially assume that a node applies the same action uniformly across all nodes, although the model retains the flexibility to account for varying actions in future iterations. The second action concerns modifying relationships with other nodes, influenced by their strategy and the trust matrix. This could entail removing connections with nodes that fall below a specific reputation score, as determined by the trust matrix.
▪ Payoffs: Each node’s actions result in a reward or a penalty. Actively participating in transaction broadcasting yields rewards proportionate to the node’s contribution to the network’s connectivity and transaction throughput. In contrast, non-cooperative behavior reduces the node’s reputation, potentially leading to isolation within the network. For this process to be fair, a node will be rewarded based on his weight and contribution to the network, meaning that a node that broadcasted to three nodes will not be rewarded the same as one that broadcasted to ten nodes; having this logic will incentivize nodes to prefer to be hubs in the network. The Reward System chapter provides a detailed exposition of this mechanism.
▪ Order of Play: The first player in each round is determined by the initiator of the transaction, essentially chosen using a pseudo-random process based on transaction initiation. The selected node then propagates the transaction to other nodes, akin to a token being passed in a ring topology.
The game possesses several distinct characteristics:
▪ Cooperative Nature: The framework views nodes as components of potential coalitions, with an ideal scenario being a single unified coalition. Represented as (𝑁,𝑣), the game promotes common interests by focusing on a network’s well-being and security. The power set 2𝑛 encapsulates all possible coalitions, for example, for a graph of three nodes. At first sight, it appears that the most optimal strategy for a node is to be alone in its coalition. However, because the game is repetitive, acting with this strategy may be better because it does not encourage nodes to participate in the group’s gain. The design incentivizes stable and fair coalitions, where no subset of players is incentivized to form a smaller coalition, and each player’s payoff is proportional to their contribution.
▪ Repetitive Game Structure: Denoted as 𝐺𝑇 The game is repetitive, with players selecting strategies at each stage of the game iteration. The cumulative payoff is the sum of the rewards obtained across all stages, and strategies are adapted based on the history of the play, allowing dynamic responses to the outcomes of previous rounds.
▪ Non-Zero-Sum Nature: The game is designed as a non-zero-sum game. If 𝑢𝑖(𝑠𝑖) denotes the payoff for choosing a strategy, then no combination of strategies results in a total payoff sum of zero.