As a powerful tool in scientific research and industrial technologies, the cold atom absolute gravity sensor (CAGS) based on cold atom interferometry has been proven to be the most promising new generation high-precision absolute gravity sensor. However, large size, heavy weight, and high–power consumption are still the main restriction factors of CAGS being applied for practical applications on mobile platforms. Combined with cold atom chips, it is possible to drastically reduce the complexity, weight, and size of CAGS.
1. Introduction
The absolute gravity sensor has been proven to be a powerful tool in scientific research and industrial technologies. In scientific research areas, it is considered to be a powerful tool to detect weak equivalence principle (WEP) violations
[1][2], which can directly imply the equivalence between inertial and gravitational mass. Moreover, as a powerful tool, the absolute gravity sensor also has been widely applied to searching for dark energy
[3][4], gravitational waves
[5][6], determining the Newtonian gravitational constant
G [7][8], short–range forces
[9][10] and so on. On the other hand, this sensor has been widely applied in industrial areas including inertial navigation
[11][12], archeology
[13][14], monitoring earthquakes
[15][16], groundwater
[17][18], resource exploration
[19][20], and many other applications.
Cold atom absolute gravity sensors (CAGSs) based on cold atom interferometry have been proven to be the most promising new generation absolute gravity sensor
[21][22]. The prototype of the cold atom gravimeter was demonstrated by Steven Chu’s group at Stanford University, in 1991
[23]. The research of CAGSs has made great progress over the last 30 years. Different from the classical optical absolute gravity sensor using mechanical mass as a free-falling object, CAGSs use the cold atom cluster as the free–falling object to sense the gravity with the advantages of better stability, a long service life
[24][25] and a high sampling rate
[25]. The sensitivity and accuracy of CAGSs have exceeded the performance of the traditional optical absolute gravimeter of FG-5
[24][25]. As reported, the uncertainty of a CAGS was demonstrated to be high, up to 1.3
μGal Gal (1 μGal = 10
−8 m/s
2)
[26], while the sensitivity of a CAGS can also be high, up to
4.2 μGal/Hz−−−√4.2 Gal/Hz [27]. There are many research groups involved in this research area and some reported representative works of CAGSs are summarized in
Table 1. The early research of CAGSs mainly focused on improving sensitivity and stability. Over the past decade, researchers gradually shifted their attention to miniaturization and applications of CAGSs. Portable CAGSs have been demonstrated to be applicable on various mobile platforms, including vehicles
[28][29], shipboard
[30][31], airborne
[32][33], and even in aerospace
[18][34][35]. However, weight, power consumption, and noise in a dynamic environment are still the main restriction factors of CAGSs being applied for practical applications, especially on mobile platforms
[16][19][34][36][37]. Many different strategies have been proposed to solve these problems, and using atom chips is one of the most promising methods, since they can significantly reduce the complexity, weight, and size of CAGSs.
Table 1. State–of–the–art of gravity sensors from different research groups as reported.
| Research Group |
Sensitivity (μGalHz√)Sensitivity |
Uncertainty (μGal) |
Ref. |
| Stanford Uni. |
8 |
3.0 |
[38][39][40] |
| Humboldt Uni. |
9.6 |
3.2 |
[19] |
| SYRTE |
5.6 |
1.3 |
[25][26][41] |
| HUST |
4.2 |
3.0 |
[27][42] |
An atom chip was originally proposed to miniaturize setups of cold atom systems using some mature technologies in integrated circuit areas
[43][44]. The first atom chip was reported in 1999
[45], and only a few years later the Bose–Einstein condensation (BEC) was realized with an atom chip
[46][47]. Subsequently, the development of atom chips has brought many significant changes and surprises to cold atom sensors including CAGSs, as in
Figure 1.
An atom chip was originally proposed to miniaturize setups of cold atom systems using some mature technologies in integrated circuit areas
[43][44]. The first atom chip was reported in 1999
[45], and only a few years later the Bose–Einstein condensation (BEC) was realized with an atom chip
[46][47]. Subsequently, the development of atom chips has brought many significant changes and surprises to cold atom sensors including CAGSs, as in
Figure 1.
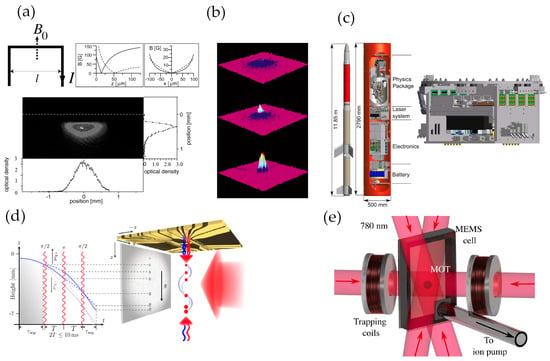
Figure 1. (
a) Wire layout of an atom chip for quadrupole potential with generated magnetic potentials and an image of a cold atom cloud (photo reproduced with permission from American Physical Society)
[45]; (
b) Time–of–flight absorption images of BEC generated with an atom chip (photo reproduced with permission from Springer Nature)
[47]; (
c) the Matter–wave Interferometry in Microgravity (MAIUS) rocket (photo reproduced with permission from Springer Nature)
[48]; (
d) Atom–chip based gravimeter and space-time trajectories of a BEC (photo reproduced with permission from American Physical Society)
[49]. (
e) Image of a chip–scale MOT (photo reproduced with permission from AIP publishing)
[50].
2. Basic Theory of CAGS and Magneto–Optical Trap (MOT)
The working principle of a CAGS is based on the cold atom, and the preparation of a cold atom cloud (with a temperature below 10 μK) is an essential process for a CAGS. Following the atomic cooling techniques proposed by several Nobel laureates based on the Doppler effect
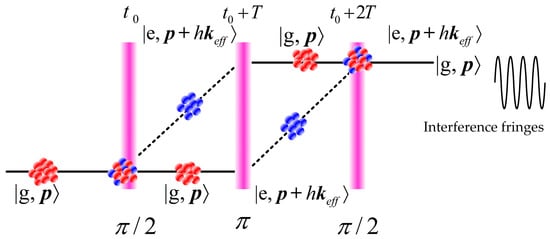
[51][52], neutral atoms can be trapped by a magneto–optical trap (MOT) first, and then the trap uses polarization gradient cooling to further reduce the temperature of the atom cloud, so that the coherence of atoms can be further improved. With a well–prepared cold atom cloud, a three–pulse sequence (π/2–π–π/2) will be applied to split, redirect and recombine the matter–wave of cold atom clouds as a typical Mach-Zehnder interferometer as in
Figure 2. The accumulated phase difference includes the free evolution phase determined by Feynman’s path integral approach, and the laser–atom interaction with gravity information contained. Through careful calculation, the gravity acceleration can be extracted from the interference fringes, obtained by scanning one of these variables, such as the chirp rate of Raman optical frequency
[51].
Figure 2. Pulse sequence process of an atom interferometer.
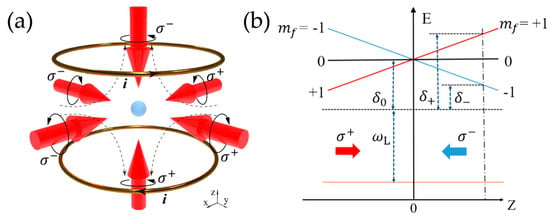
As an important component of CAGSs, the magneto–optical trap (MOT) was originally proposed and realized by Raab et al., as the most widely used method for cold atom cloud preparation
[53][54][55]. The fundamental work principle of the three–dimensional MOT was realized by three pairs of circularly polarized red–detuned lasers with a static gradient magnetic field, as in
Figure 3a. The static gradient magnetic field is usually around 10 G/cm, traditionally provided by a pair of anti–Helmholtz coils, and the magnetic field strength is zero at the intersection of three pairs of lasers
[56]. The energy level of these atoms in the MOT will split due to the Zeeman effect, and one possible configuration of the energy level structure is as shown in
Figure 3b. Researchers can set the total angular momentum of the ground state as
J=0J=0, and
J=1J=1 for the excited state, which contains three magneton energy levels
mf=0,±1m generated by Zeeman splitting in the magnetic field. These magneton energy levels degenerate at the center of the magnetic field. Taking
Z direction as an example, as in
Figure 3b, two lasers collide these atoms from opposite directions. The frequency of these lasers is
wLaser, the detuning frequency of which is relative to atomic resonance frequency at zero magnetic fields, or
δ0. In addition, the polarization directions of these two lasers are σ+ and σ−. Due to the transition selection rules, the detuning of σ+ and σ− lasers are
δ+ and
δ−, respectively. Assuming the initial position of an atom is
Z0 (
Z0 > 0), then
δ+>δ− and atoms will absorb more photons from σ− lasers, resulting in a negative centripetal force to atoms. Similarly, the atoms will gain a positive centripetal force if
Z0 < 0, and all atoms will feel the radiation force from lasers pointing to the center, becoming trapped in the center as in
Figure 3b.
Figure 3. (a) Schematic diagram of a three–dimensional magneto–optical trap. (b) Atomic energy level splitting and trapping principle.
The traditional MOT is usually constructed by the combination of the magnetic coil and laser system, making the experimental system complicated and very heavy. Recently, with the development of integrated circuits and micro/nanofabrication processing, various designs have been proposed to simplify this system with different types of atom chips. The atom chips are usually fabricated with surface micro/nanostructures to accurately control the magnetic, electric, or light field. Using these atom chips, the confinement and coherent manipulation of cold atoms can be realized on a small scale under low power consumption conditions.