Supersonic Flow Control: History
View Latest Version
Please note this is an old version of this entry, which may differ significantly from the current revision.
Subjects: Physics, Fluids & Plasmas
Contributor:
Sher Afghan Khan

In high-speed fluid dynamics, base pressure controls find many engineering applications, such as in the automobile and defense industries. Several studies have been reported on flow control with sudden expansion duct. 

  • flow control
  • de Laval nozzle
  • CD nozzle
  • microjet
  • supersonic flow
  • CFD
  • DOE

1. Introduction

In supersonic vehicles, the flow of exit from the rockets and missile engines has become a significant issue. It has been found that the loss of air inside the high-speed vehicle engines turns to increases the base drag. For example, a nozzle with sudden expansion ducts will form a recirculation zone, increasing base drag. When the base drag increases, the total amount of exit pressure will decrease, and this decrement will result in the loss of the forwarding force or thrust. Hence, many studies have reported controlling the high-speed flows as a passive and active control method. In a passive control method, the duct shape is modified with additional devices/shapes, such as ribs, cavities, cylinders, aerospikes, splitter plate, etc. In addition, researchers used different devices of flow formation, such as a nozzle as internal flow control and bluff body, non-circular cylinders, airfoil, and wings as external aerodynamics flow control. On the other hand, the active control of high-speed flow has been studied extensively over the last two decades. Researchers have used a high-speed nozzle with a sudden expansion duct and a microjet controller; a tiny hole in the base area is drilled to control the flow, which was found to be an excellent technique in a supersonic flow problem. Hence, this review is more focused on the active control approach, using a microjet in a CD nozzle.

The abrupt expansion of the external compressible flow over the back of the projectiles and its association to the base pressure has long been the focus of researchers’ interest. The base drag, which accounts for a significant portion of the overall drag, is determined by the base pressure. Generally, the base pressure for a high-speed projectile is lower than the ambient pressure. The vast majority of the ballistics test data were supplied, leading us to presume that the base pressure ratio depends entirely on the flight Mach number. Compared to traditional ballistics testing processes, the experimental investigation of the internal flow apparatus provides several distinguishing benefits. A significant amount of air supply is lowered, which would generally be necessary for the wind tunnel test section to be large enough so that wall interference and other factors do not disrupt the model flow. Internal flows are free of stings and other support devices that are necessary for external flow investigations. The most significant benefit of the internal flow device is that static pressure and surface temperature measurements can be recorded as well as the entry to the expansion and the wake zone (Figure 1). These observations are crucial if the theoretical predictions are to be extensively investigated.

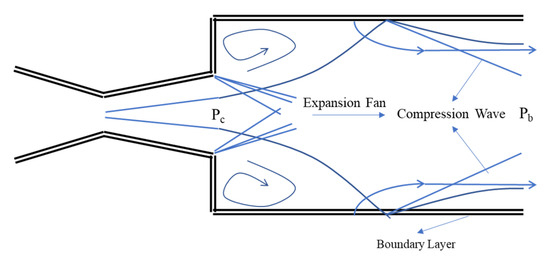
Applsci 11 06899 g001 550
Figure 1. Sudden expansion flow field. Reprinted with permission from ref. [1]. Copyright 2021 Springer.

The sudden expansion problems in the subsonic and supersonic flow regimes are found in many applications. We discovered that previous researchers used a system to replicate high altitude conditions in jet and rocket engine test cells, jet discharge results in insufficient, sub-atmospheric discharge pressure. This was found by Khan et al. [2], who used microjets to control the sudden expansion flow (base pressure) from the CD nozzle as an active control method. 

2. High-Speed Flow Development in Nozzle

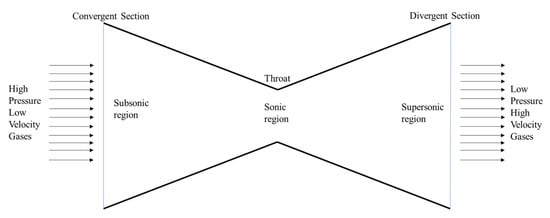
For supersonic flow development and investigations, a CD nozzle is utilized in most studies. A nozzle with no expanding part cannot produce supersonic air [3]; the flow is sonic at the throat; therefore, an asterisk denotes conditions at a sonic level. At the throat, the Mach number = 1, (V = a) and the throat area (a). Figure 2 illustrates a basic CD nozzle model [4] and the parameters with an asterisk are defined as critical values. If a high-pressure tank is connected to a pipe, then the velocity at the pipe exit changes depending on the backpressure. At any other region of the nozzle, the Mach number velocity and the local area can be derived by the continuity equation [5][6][7]. Even though it is possible to study one-dimensional flow behavior directly, it is only a particular case of two-dimensional flow. One of the known one-dimensional flow phenomena is the normal shock and formation of oblique shock waves. The occurrence of oblique shock waves in different flow fields occurs, such as flight at high Mach numbers, aircraft design, diffusers, and supersonic nozzles.

Applsci 11 06899 g002 550
Figure 2. Convergent–divergent (CD) nozzle.

3. Passive Control Methods

It was observed that the high-speed flow controlled by the excitation of free shear-layer instabilities [8] uses localized arc filament plasma actuators in jets for different Mach numbers at a supersonic range. Focusing the aspect ratio of the nozzle and nozzle pressure ratio (NPR) with the Mach number variation, the elliptic jet control with limiting tab [9] was investigated and showed that each parameter is important to control the jet flows, similar to the overexpanded plug nozzle jet [10] controlled by the passive method. Additionally, some researchers used ventilated triangular tabs to control the jet [11], control the supersonic elliptic jet with ventilated tabs [12], and to measure the impact of the tab location relative to the nozzle exit on the shock structure of a supersonic jet [13].

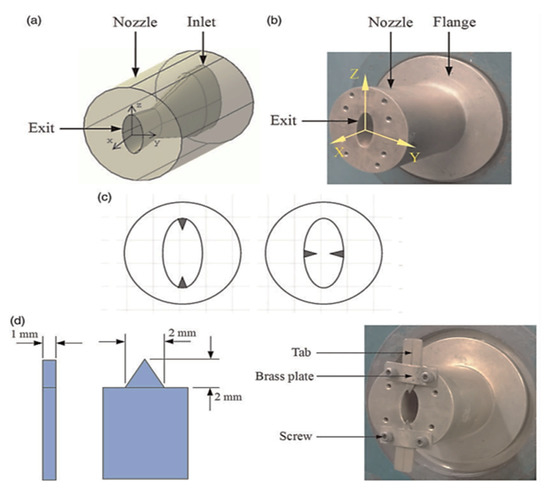
Khan et al. [14] experimentally investigated the effect of the extended cowl on the flow field of planar plug nozzles for two different Mach number ranges (1.8 and 2.2) to observe the influence of the cowl length for the pressure distribution. Manigandan and Vijayaraja [15] experimentally investigated the flow-field and acoustic characteristics of the elliptical throat in the CD nozzle. According to the findings, switching from an elliptical to a circular throat alters the shock cell architecture, resulting in a substantial shift in the scream amplitude, owing to wave weakening. The jet controlled for mixing the flow was experimentally studied by Khan et al. [16] for the enhancement of the supersonic twin-jet mixing by vortex generator to observe the effects and the behavior of the daughter streams. Similarly, an impinging plug nozzle jet using a vortex generator [17] was studied experimentally. Figure 3 shows the nozzle and tab details, CAD drawing (Figure 3 a), photographic view without tabs (Figure 3 b), schematic sketch of nozzle exits with triangular tabs along the major and minor axes (Figure 3 c), and triangular tab dimensions and the photograph of the nozzle with triangular tabs along the major axis (Figure 3 d).

Applsci 11 06899 g004 550
Figure 3. Jet control device, (a) Nozzle drawing, (b) Nozzle image without tabs, (c) Both minor and major tabs on the nozzle drawing (d) Triangular tabs dimension and nozzle image with major tabs [18].

The bulk of these noise reduction systems are referred to be passive since they cannot be switched off or changed while in flight and might result in performance losses. Penn State [19] is developing a fluid insert technique for supersonic jet noise reduction. The fluid insert method aims to reduce noise in low bypass ratio turbofans while having minimal impact on engine performance. The fluid inserts blast air into the diverging portion of the nozzle on demand, which may be turned off or adjusted depending on the flight regime. Although significant research has been conducted in the form of noise measurements and Reynolds-averaged Navier–Stokes (RANS) calculations to enhance the fluid insert technology [20][21], the reason why these inserts work is still not understood completely. The correlation of changes in the flow field with corresponding changes in the noise is inadequate, using only existing RANS data [22][23]. It was suggested that unstable scale-resolving simulations be used to obtain more insight into the flow field and to better understand noise reduction techniques [19]. Additionally, when properly structurally supported, acoustic reflectors of an adequate scale are a suitable noise reduction solution for the high-pressure venting typical of blowdown operations [24]. Due to disadvantages, such as delayed convergence and the complexity of the phase shift mechanism, a unique technique was used that does not use secondary path modeling [25] and the sensitivity of noise to system uncertainties [26]. Fluid inserts reduce the convection speed of wave packets in the jet shear layer, resulting in a reduced Mach wave radiation angle [27].

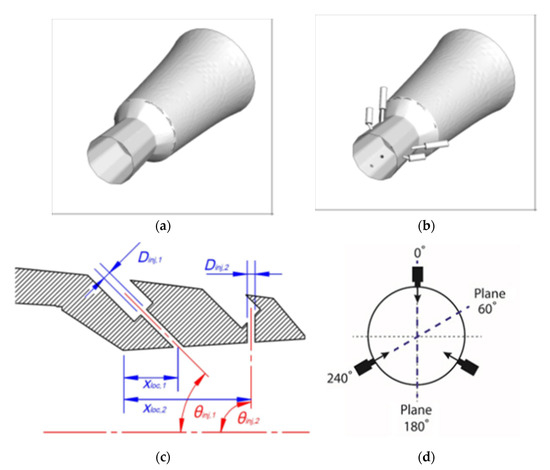
This interaction is discussed here with a focus on noise creation and reduction when a jet is parallel to or impinging on a solid wall. Various researchers have presented computational methods [28][29] with large-eddy simulations [30][31][32][33][34], high fidelity simulations [35] and 3D simulation [36]. The noise reduction of supersonic jets by nozzle trailing-edge changes was investigated experimentally [37], and hemispherical noise reduction reflectors on transonic jet flows [38] were conducted. Different injection sites, angles, and circumstances were also investigated, resulting in distinct acoustic behavior and flow-field changes [39]. Using steady fluidic injection, researchers conducted an empirical scaling analysis of supersonic jet control [40]. Aft and lateral wall inclinations for a cavity [41] supersonic cavity flow utilizing high-speed upstream injection [42] and cavity dynamics to the introduction of various storage configurations installed at different positions inside the cavity [43] were all numerically modeled for noise reduction. The employment of a single injector as a fluid insert helps break up the large-scale structures of the flow, according to direct cross-correlations of near-field data with far-field microphone signals [44]. Pipe-jet noise is reduced via geometric changes in the form of trailing edge castellations. The interaction between the streamwise vortices is determined by the number of castellations, which changes the sound generated [45]. Figure 4 shows the noise reduction configurations, which are adopted in the nozzle exit region, and represents the baseline nozzle (Figure 4 a), nozzle (Figure 4 b), schematic of a single fluid insert in the nozzle (Figure 4 c), and designation of different azimuthal planes for the 3FC-2FI nozzle (Figure 4 d).

Applsci 11 06899 g005 550
Figure 4. Details of nozzle configurations. Reprinted with permission from ref. [27]. Copyright 2020 Springer.

4. Active Control Methods

The major consideration of this review work is to explore the active control methods of supersonic flows. Therefore, this section is split into the methodologies employed by the researchers: experimental, CFD, and soft computing approaches.

In summary, Khan et al., investigated the active control of the base pressure [2][46][45][47][48][49][50] in which they considered the differed Mach number at various supersonic flow ranges, such as Mach numbers 1.25, 1.30, 1.48, 1.6, 1.8 and 2.0 in [48][49]; 1.87, 2.2 and 2.58 in [46]; and 2.0, 2.5 and 3.0 in [45], respectively. The test area ratio was from 2.56, 3.24, 4.84, and 6.25; the NPR was from 3 to 9, and the duct length for L = 10D until 1D. More attention was given in the recent study to wall pressure distribution for different inertia levels, relief to the flow, NPR, and the L/D ratio [51][52][53][54][55][56][57][58][59]. After monitoring the flow from the active microjet control, it is also necessary to know whether the flow changes in the duct or not. Hence, recent findings have also shown that several studies are conducted with equivalent or variable Mach number and area ratios with the same NPR and L/D ratios. The majority of wall pressure flows were considered with varying the area ratio, such as 2.56 [51][52], 3.24 [53][54][60][61], 4.84 [55][56][57][62], and varying the Mach number for the same area ratio 2.56 with Mach 2 and 3 [58], 1.3. 1.9, and 2.4 [63] and for the area ratio 6.25 with Mach 1.1 and 1.5 [64] and 2.1 and 2.8 [65].

Researchers used the DOE principle based on the current problem and found that this could be an efficient way to achieve the base pressure control with an appropriate parameter. Therefore, to refine the base pressure control, the DOE technique was used. The impact of microjets on control was achieved to obtain base pressure differences of various parameters. The general DOE approach method can be seen in Figure 5 .

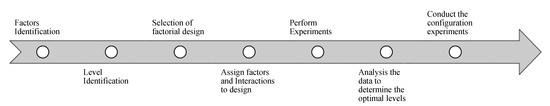
Applsci 11 06899 g011 550
Figure 5. Steps in implementation of DOE approach. Reprinted with permission from ref. [1]. Copyright 2021 Springer.

The experimental study was used for various parametric combinations using microjets to control the base pressure, using a CD nozzle. The data were used to refine the optimal mix of parameters employed to provide precise control of base pressure for improved performance with DOE [66]. A Taguchi design L 9-OA and variance analysis (ANOVA) was used to analyze the influence of nozzle parameters affecting the base pressure. Multiple linear regression models, confirmation checks, and linear regression equations were performed for accuracy in an optimization. The ANOVA method was also used to obtain the individual parameter's statistical significance on the total base pressure variability [67]. The observation on the control becomes effective for lower area ratio, compared to the higher area ratio with the aid of 15 arbitrary test cases; two linear regression model presentations were tested for their estimated accuracy [68]. To optimize the response surface methodology (RSM) of experimental data, non-linear regression models based on the central composite design (CCD) and box-Behnken design (BBD) were developed to simplify the input-output relationships [69]. The DOE with L 27-OA and ANOVA was used to determine the feedback (in percentage terms) of various process parameters and their correlations with and without control on the base pressure [70][71]. The optimum nozzle parameters were targeted, such as convergent angle, divergent angle, and the throat radius of the nozzle; the best values were assessed based on the flow parameters [72]. Jaimon et al. [73] used the DOE method to predict the suddenly expanded flow with and without microjets as an active control. To develop the linear model, they used a complete factorial design of the L 16 orthogonal array (OA). Using Taguchi’s L 27 orthogonal array, a regression analysis was made [74], and optimized results investigated the suitable parameters for base pressure control.

This entry is adapted from the peer-reviewed paper 10.3390/app11156899

References

  1. AAabid, A.; Khan, S.A. Investigation of High-Speed Flow Control from CD Nozzle Using Design of Experiments and CFD Methods. Arab. J. Sci. Eng. 2021, 46, 2201–2230.
  2. Khan, S.A.; Rathakrishnan, E. Control of Suddenly Expanded Flows with Micro-Jets. Int. J. Turbo Jet Engines 2003, 20, 63–82.
  3. Ramanjaneyulu, S. Design and flow analysis of Convergent Divergent nozzle using CFD. Int. J. Res. Appl. Sci. Eng. Technol. 2019, 7, 4020–4029.
  4. Singh, J.; Zerpa, L.E.; Partington, B.; Gamboa, J. Effect of nozzle geometry on critical-subcritical flow transitions. Heliyon 2019, 5, 19.
  5. Rathakrishnan, E. Applied Gas Dynamics; Wiley: Hoboken, NJ, USA, 2019.
  6. Greyvenstein, G.P. An implicit method for the analysis of transient flows in pipe networks. Int. J. Numer. Methods Eng. 2001, 53, 1127–1143.
  7. Keir, A.S.; Ives, R.; Hamad, F. CFD analysis of C-D nozzle compared with theoretical & experimental data. INCAS Bull. 2018, 10, 53–64.
  8. Samimy, M.; Webb, N.; Crawley, M. Excitation of Free Shear-Layer Instabilities for High-Speed Flow Control. AIAA J. 2018, 56, 1770–1791.
  9. Rathakrishnan, E.; Ethirajan, R. AR 4 elliptic jet control with limiting tab. Fluid Dyn. Res. 2017, 50, 025505.
  10. Khan, A.; Kumar, R. Experimental Study and Passive Control of Overexpanded Plug Nozzle Jet. J. Spacecr. Rocket. 2018, 55, 778–782.
  11. Jacksi, K.; Ibrahim, F.; Ali, S. Scholars Journal of Engineering and Technology (SJET). Sch. J. Eng. Technol. 2018, 9523, 49–53.
  12. Akram, S.; Rathakrishnan, E. Control of Supersonic Elliptic Jet with Ventilated Tabs. Int. J. Turbo Jet Engines 2017, 37, 267–283.
  13. Kumar, P.A.; Aileni, M.; Rathakrishnan, E. Impact of tab location relative to the nozzle exit on the shock structure of a supersonic jet. Phys. Fluids 2019, 31, 076104.
  14. Khan, A.; Panthi, R.; Kumar, R.; Ibrahim, S.M. Experimental investigation of the effect of extended cowl on the flow field of planar plug nozzles. Aerosp. Sci. Technol. 2019, 88, 208–221.
  15. Manigandan, S.; Vijayaraja, K. Flow field and acoustic characteristics of elliptical throat CD nozzle. Int. J. Ambient. Energy 2017, 40, 57–62.
  16. Khan, A.; Akram, S.; Kumar, R. Experimental study on enhancement of supersonic twin-jet mixing by vortex generators. Aerosp. Sci. Technol. 2020, 96, 105521.
  17. Panthi, R.; Krishna, T.V.; Nanda, S.R.; Khan, A.; Kumar, R.; Sugarno, M.I. Experimental Study of Impinging Plug Nozzle Jet Using a Vortex Generator. J. Spacecr. Rocket. 2020, 57, 1414–1418.
  18. Kumar, S.A.; Rathakrishnan, E. Elliptic jet control with triangular tab. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2017, 231, 1460–1477.
  19. Prasad, C.; Morris, P.J. Unsteady Simulations of Fluid Inserts for Supersonic Jet Noise Reduction. AIAA Scitech 2019 Forum 2019, 1–22.
  20. Gao, J.; Xu, X.; Li, X. Numerical simulation of supersonic twin-jet noise with high-order finite difference scheme. AIAA J. 2018, 56, 290–300.
  21. Chen, B.; Wang, Y. Active aerodynamic noise control research for supersonic aircraft cavity by nonlinear numerical simulation. Int. J. Electr. Eng. Educ. 2021.
  22. Zhu, W.; Xiao, Z.; Fu, S. Numerical modeling screen for flow and noise control around tandem cylinders. AIAA J. 2020, 58, 2504–2516.
  23. Patel, T.K. Analysis of Supersonic Jet Noise in the Sideline and Upstream Directions Using the Navier-Stokes Equations. Ph.D. Thesis, University of Florida, Gainesville, FL, USA, 2020.
  24. Coombs, J.; Schembri, T.; Zander, A. The effect of hemispherical surface on noise suppression of a supersonic jet. In Proceedings of the Acoustics 2019—Sound Decisions: Moving forward with Acoustics, Mornington Peninsula, Australia, 13–14 May 2019; pp. 1–10.
  25. Mondal, K.; Das, S.; Hamada, N.; Abu, A.; Das, S.; Faris, W.; Thiam, H.; Toh, T.; Ahmas, A. An improved narrowband active noise control system without secondary path modelling based on the time domain. Int. J. Veh. Noise Vib. 2019, 15, 110–132.
  26. Berton, J.J.; Huff, D.L.; Geiselhart, K.; Seidel, J. Supersonic Technology Concept Aeroplanes for Environmental Studies. AIAA Scitech 2020 Forum 2020.
  27. Prasad, C.; Morris, P.J. A study of noise reduction mechanisms of jets with fluid inserts. J. Sound Vib. 2020, 476, 115331.
  28. Salehian, S.; Mankbadi, R.R. A review of aeroacoustics of supersonic jets interacting with solid surfaces. AIAA Scitech 2020 Forum 2020, 1–31.
  29. Rahmani, S.K.; Alhawwary, M.A.; Wang, Z.J.; Phommachanh, J.; Hill, C.; Hartwell, B.; Collicott, B.; Swim, J.; Farokhi, S.; Taghavi, R.; et al. Noise Mitigation of a Supersonic Jet Using Shear Layer Swirl. AIAA Scitech 2020 Forum 2020, 1–30.
  30. Horner, C.; Sescu, A.; Afsar, M.; Collins, E.; Azarpeyvand, M. Passive Noise Control Strategies for Jets Exhausting over Flat Surfaces: An LES Study. AIAA Aviation 2020 Forum 2020, 1–17.
  31. Prasad, C.; Morris, P.J. Steady active control of noise radiation from highly heated supersonic jets. J. Acoust. Soc. Am. 2021, 149, 1306–1317.
  32. Pourhashem, H.; Kumar, S.; Kalkhoran, I.M. Flow field characteristics of a supersonic jet influenced by downstream microjet fluidic injection. Aerosp. Sci. Technol. 2019, 93, 105281.
  33. Li, B.; Ye, C.-C.; Wan, Z.-H.; Liu, N.-S.; Sun, D.-J.; Lu, X.-Y. Noise control of subsonic flow past open cavities based on porous floors. Phys. Fluids 2020, 32, 125101.
  34. Martin, R.; Soria, M.; Rodriguez, I.; Lehmkuhl, O. On the Flow and Passive Noise Control of an Open Cavity at Re = 5000. Flow Turbul. Combust. 2021, 1–26.
  35. Ye, C.C.; Zhang, P.J.Y.; Wan, Z.H.; Sun, D.J.; Lu, X.Y. Numerical investigation of the bevelled effects on shock structure and screech noise in planar supersonic jets. Phys. Fluids 2020, 32.
  36. Su, Z.; Liu, E.; Xu, Y.; Xie, P.; Shang, C.; Zhu, Q. Flow field and noise characteristics of manifold in natural gas transportation station. Oil Gas Sci. Technol. Rev. l’IFP 2019, 74, 70.
  37. Wei, X.; Mariani, R.; Chua, L.; Lim, H.D.; Lu, Z.; Cui, Y.; New, T.H. Mitigation of under-expanded supersonic jet noise through stepped nozzles. J. Sound Vib. 2019, 459, 1–18.
  38. Coombs, J.; Zander, A.; Schembri, T. Influence of a Hemispherical Noise Reduction Reflector on Transonic Jet Flows. In Proceedings of the 22nd Australasian Fluid Mechanics Conference AFMC2020, Brisbane, Australia, 7–10 December 2020.
  39. Cuppoletti, D.; Gutmark, E.J.; Hafsteinsson, H.E.; Eriksson, L.-E. Elimination of Shock Associated Noise in Supersonic Jets by Destructive Wave Interference. AIAA J. 2018, 57, 720–734.
  40. Kumar, P.A.; Kumar, S.M.A.; Mitra, A.S.; Rathakrishnan, E. Empirical scaling analysis of supersonic jet control using steady fluidic injection. Phys. Fluids 2019, 31, 056107.
  41. Mancini, S.; Kolb, A.; Gonzalez-Martino, I.; Casalino, D. Effects of wall modifications on pressure oscillations in high-subsonic and supersonic flows over rectangular cavities. In Proceedings of the 25th AIAA/CEAS Aeroacoustics Conference, Delft, The Netherlands, 20–23 May 2019; pp. 1–20.
  42. Xiansheng, W.; Dangguo, Y.; Jun, L.; Fangqi, Z. Control of Pressure Oscillations Induced by Supersonic Cavity Flow. AIAA J. 2020, 58, 2070–2077.
  43. Robertson, G.; Kumar, R. Effects of a Generic Store on Cavity Resonance at Supersonic Speeds. AIAA J. 2020, 58, 4426–4437.
  44. Prasad, C.; Morris, P. Effect of fluid injection on turbulence and noise reduction of a supersonic jet. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2019, 377, 20190082.
  45. Anureka, R.; Srinivasan, K. Passive control of pipe-jet noise using trailing-edge castellations. Appl. Acoust. 2020, 170, 107516.
  46. Khan, S.A.; Rathakrishnan, E. Active Control of Suddenly Expanded Flows from Overexpanded Nozzles. Int. J. Turbo Jet Engines 2002, 19, 119–126.
  47. Khan, S.A.; Rathakrishnan, E. Active Control of Suddenly Expanded Flows from Underexpanded Nozzles. Int. J. Turbo Jet Engines 2004, 21, 233–254.
  48. Khan, S.A.; Rathakrishnan, E. Control of Suddenly Expanded Flows from Correctly Expanded Nozzles. Int. J. Turbo Jet Engines 2004, 21, 255–278.
  49. Khan, S.A.; Rathakrishnan, E. Active Control of Suddenly Expanded Flows from Underexpanded Nozzles—Part II. Int. J. Turbo Jet Engines 2005, 22, 163–183.
  50. Khan, S.A.; Rathakrishnan, E. Active Control of Base Pressure in Supersonic Regime. J. Inst. Eng. Aerosp. Eng. J. 2006, 87, 1–10.
  51. Khan, S.A.; Aabid, A.; Chaudhary, Z.I. Influence of control mechanism on the flow field of duct at mach 1.2 for area ratio 2.56. Int. J. Innov. Technol. Explor. Eng. 2019, 8, 1135–1138.
  52. AAabid Fharukh, A.; Sher Afghan, K. Experimental Investigation of Wall Pressure Distribution in a Suddenly Expanded Duct from a Convergent-Divergent Nozzle. In Proceedings of the 2019 6th IEEE International Conference on Engineering Technologies and Applied Sciences (ICETAS), Kuala Lumpur, Malaysia, 20–21 December 2019; p. 6.
  53. Khan, S.A.; Aabid, A.; Mokashi, I.; Ahmed, Z. Effect of Micro Jet Control on the Flow Filed of the Duct at Mach 1.5. Int. J. Recent Technol. Eng. 2019, 8, 1758–1762.
  54. Khan, S.A.; Mokashi, I.; Aabid, A.; Faheem, M. Experimental Research on Wall Pressure Distribution in C-D Nozzle at Mach number 1.1 for Area Ratio 3.24. Int. J. Recent Technol. Eng. 2019, 8, 971–975.
  55. Faheem, M.; Kareemullah, M.; Aabid, A.; Mokashi, I.; Khan, S.A. Experiment on of nozzle flow with sudden expansion at mach 1.1. Int. J. Recent Technol. Eng. 2019, 8, 1769–1775.
  56. Akhtar, M.N.; Bakar, E.A.; Aabid, A.; Khan, S.A. Effects of micro jets on the flow field of the duct with sudden expansion. Int. J. Innov. Technol. Explor. Eng. 2019, 8, 636–640.
  57. Azami, M.H.; Faheem, M.; Aabid, A.; Mokashi, I.; Khan, S.A. Experimental Research of Wall Pressure Distribution and Effect of Micro Jet at Mach 1.5. Int. J. Recent Technol. Eng. 2019, 8, 1000–1003.
  58. Aabid, A.; Khan, S.A. Determination of wall pressure flows at supersonic Mach numbers. Mater. Today Proc. 2021, 38, 2347–2352.
  59. Saleel, A.; Baig, M.A.A.; Khan, S.A. Experimental Investigation of the Base Flow and Base Pressure of Sudden Expansion Nozzle. IOP Conf. Ser. Mater. Sci. Eng. 2018, 370, 012052.
  60. Khan, S.A.; Ahmed, Z.; Aabid, A.; Mokashi, I. Experimental research on flow development and control effectiveness in the duct at high speed. Int. J. Recent Technol. Eng. 2019, 8, 1763–1768.
  61. Azami, M.H.; Faheem, M.; Aabid, A.; Mokashi, I.; Khan, S.A. Inspection of Supersonic Flows in a CD Nozzle using Experimental Method. Int. J. Recent Technol. Eng. 2019, 8, 996–999.
  62. Akhtar, M.N.; Bakar, E.A.; Aabid, A.; Khan, S.A. Control of CD nozzle flow using microjets at mach 2.1. Int. J. Innov. Technol. Explor. Eng. 2019, 8, 631–635.
  63. Aabid, A.; Khan, S.A. Studies on Flows Development in a Suddenly Expanded Circular Duct at Supersonic Mach Numbers. Int. J. Heat Technol. 2021, 38, 185–194.
  64. Aabid, A.; Khan, S.A.; Baig, M.A.A.; Reddy, A.R. Investigation of Flow Growth in aDuct Flowsfor Higher Area Ratio. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1057, 10.
  65. Aabid, A.; Khan, S.A.; Baig, M.A.A.; Rao, K.S. Effect of Control on the Duct Flow at High Mach Numbers. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1057, 9.
  66. Quadros, J.D.; Khan, S.A.; Antony, A.J. Investigation of effect of process parameters on suddenly Expanded flows through an axi-symmetric nozzle for different Mach Numbers using Design of Experiments. IOP Conf. Ser. Mater. Sci. Eng. 2017, 184, 012005.
  67. Quadros, J.D.; Khan, S.A.; Antony, A.J. Base Pressure Behaviour in a Suddenly Expanded Duct at Supersonic Mach Number Regimes using Taguchi Design of Experiments. Mech. Mech. Eng. 2018, 22, 1077–1098.
  68. Quadros, J.; Khan, S.A.; Antony, A.J. Study of Effect of Flow Parameters on Base Pressure in a Suddenly Expanded Duct at Supersonic Mach Number Regimes using CFD and Design of Experiments. J. Appl. Fluid Mech. 2018, 11, 483–496.
  69. Quadros, J.D.; Khan, S.A.; Anthony, J.A. Modelling of Suddenly Expanded Flow Process in Supersonic Mach Regime using Design of Experiments and Response Surface Methodology. J. Comput. Appl. Mech. 2018, 49, 149–160.
  70. Quadros, J.D.; Khan, S.; Antony, A. Study of base pressure behavior in a suddenly expanded duct at supersonic mach number regimes using statistical analysis. J. Appl. Math. Comput. Mech. 2018, 17, 59–72.
  71. Al-khalifah, T.; Aabid, A.; Khan, S.A.; Azami, M.H.B.; Baig, M. Response surface analysis of the nozzle flow parameters at supersonic flow through microjets. Aust. J. Mech. Eng. 2021, 13, 1–15.
  72. Majil, D.D.; Poojitha, A.; Devi, G. Computational Study on Optimization of Rocket Nozzle. Master’s Thesis, University of Southampton, Southampton, UK, 2016; pp. 8–13.
  73. Quadros, J.; Khan, S.; Anthony, J.A. Predictive modeling of suddenly expanded flow process in the Supersonic Mach number regime using response surface methodology. Int. J. Recent Res. Asp. 2018, 49, 149–160.
  74. Al-khalifah, T.; Aabid, A.; Khan, S.A. Regression Analysis of Flow Parameters at High Mach Numbers. Solid State Technol. 2020, 63, 5473–5488.
More
© Text is available under the terms and conditions of the Creative Commons Attribution (CC BY) license; additional terms may apply. By using this site, you agree to the Terms and Conditions and Privacy Policy.
This entry is offline, you can click here to edit this entry!
Video Production Service
Feedback