Encyclopedia
Please note this is an old version of this entry, which may differ significantly from the current revision.
Subjects:
Engineering, Civil
Despite the recent initiatives and developments in building design provisions using performance-based design, practicing engineers frequently adopt force-based design approaches, irrespective of the structural system or building irregularity. Modern seismic building codes adopt the concept of simplifying the complex nonlinear response of a structure under seismic loading to an equivalent linear response through elastic analytical procedures using seismic design response factors. Nevertheless, code-recommended seismic design response factors may not result in a cost-effective design with a uniform margin of safety for different structural systems.
- multi-story buildings
- structural systems
- seismic response factors
- design codes
1. Introduction
Rapid urbanization in major metropolitan areas around the globe has witnessed scarcity and a high cost of land, giving rise to a remarkable increase in the number of multi-story building constructions. These multi-story buildings, if not acceptably designed, can be under significant threat from natural hazards that can cause potential damage to the structures, resulting in substantial economic losses. Lateral loads, particularly in wind- or earthquake-prone regions, usually govern the design of a multi-story building. Inelastic analysis is required to capture the seismic behavior realistically since buildings are expected to experience large deformations under the design of an earthquake. Practicing engineers adopt elastic analysis methods in the design of structures instead of nonlinear analysis, either due to economic reasons or a lack of required knowledge to utilize nonlinear analysis procedures. The inelastic response of a structure is accounted for in the elastic analysis methods by reducing seismic forces and amplifying deformations to arrive at safe designs with optimized costs. Thus, seismic design response factors play an essential part in the safety and economy of structures.
Seismic response factors prescribed in various design codes and guidelines covering different regions, structural systems, and constructional practices may not provide cost-effective designs for different structures and seismic zones with a uniform margin of safety [1,2,3,4,5,6]. Accurately calibrating these factors optimizes the seismic design forces, reducing costs for the overall structural system without compromising structural safety. This highlights the need for verifying the code-provided seismic design response factors of multi-story buildings with various structural systems using well-founded assessment methodologies.
2. Seismic Design Response Factors
The seismic design forces of structures are derived in design codes by reducing the anticipated elastic seismic forces with a force reduction factor. The factors used to reduce seismic forces and amplify deformations to arrive at a safe design with optimized cost are termed seismic design response factors. Seismic design response factors may be based on engineering judgment and have a limited analytical basis [1]. The values of these seismic design factors adopted in seismic design codes do not provide uniform safety margins covering various structural systems, although they dictate the performance of buildings and the seismic design process. This presses the need for the proper selection of appropriate values of the seismic design factors for building structures, which has been a debatable issue in the development of seismic design provisions and highlighted in several previous studies. The shortcomings in seismic design factors are particularly evident at various performance levels and under bi-directional input ground motions [7,8,9,10]. Hence, the accurate evaluation of seismic design factors and the interrelationships between the different design parameters are essential components in the seismic design of multi-story buildings.
The reserve strength and the ductility levels in a structure are utilized to reduce the seismic forces through the response modification factor [1,2,3,4,5,6]. Lateral load-resisting systems are designed to be deflection-controlled and possess adequate inelastic deformation capacity. The ductile detailing is essential to ensure that the components of these systems achieve a desirable behavior. Some previous studies highlighted the significance of redundancy in the structure to the seismic design response factors [11,12,13,14]. 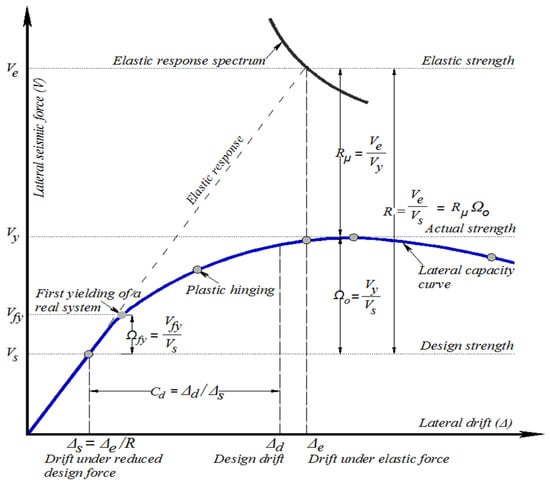Figure 2 illustrates a typical lateral force–deformation relationship defining the components of seismic response factors, including the response modification factor (R), ductility reduction factor (Rμ), deflection amplification factor (Cd), and structural overstrength factor (Ωo), as recommended in various building codes [1,2]. The values of the R, Cd, and Ωo factors depend on the structural system and material.
Figure 2. Seismic design coefficients and their inter-relationship.
2.1. Historical Perspective of Seismic Response Factors
The evolution of seismic-resistant designed buildings can be traced several decades after the San Francisco earthquake of 1906 and classified into three phases [15,16]. The first phase adopted the application of the prescribed percentage of building weight as an applied load to the structure [17]. This was published under the first seismic code provisions of the Uniform Building Code (UBC) presented by SEAOC in 1927. The second phase used the concept of the seismic base shear (V) related to zone factor (Z), building system type (K), building period (C), and building weight (W) [18]. The response modification factor was introduced under this phase for the first time in the late 1970s to calculate the design base shear (Vs) of the structure by reducing the elastic base shear (Ve) with the reduction factor (R) using 5% damped acceleration for different systems. The present phase, also defined as the third phase, is based on applying the equivalent lateral force on the structure and employing spectral acceleration maps representing the site seismicity, importance factors, the natural building period, factors affecting the site, and the response modification factors [19].
2.2. Seismic Response Factors in Various Codes
Seismic design factors serve a similar function in all seismic design codes. These factors introduced in the seismic codes are denoted in different terms and assigned different numerical values. Brief comparisons of these factors practiced in various seismic codes are summarized in Table 1.
Table 1. Comparison of seismic design coefficients.
| Seismic Provisions | Applicable Region/ Country |
Response Modification Factor |
Deflection Amplification Factor |
Deflection Amplification Factor/ Response Modification Factor |
|---|---|---|---|---|
| ASCE 7-22 (2022) [20] | U.S. and other countries | R | Cd | 0.50–1.00 |
| Eurocode 8 (2004) [4] | Europe | q a | q | 1.00 c |
| NZS 1170.4 (2016) [21] | New Zealand | µ b | µ | 1.00 c |
| NBCC (2020) [22] | Canada | Rd/Ro | Rd/Ro | |
| MCBC (2015) [6] | Mexico | Q a | Q | 1.00 c |
| UBC | ||||
| UBC (1994) [23] | U.S. and other countries | Rw | (0.375)Rw | 0.375 |
| UBC (1997) [24] | R | 0.7R | 0.70 |
a reduces to 1.0 at T = 0 s and is period-dependent in the short period range. b does not reduce to 1.0 at T = 0 s and is period-dependent in the 0.45–0.7 s range. c greater than 1.0 in the short period range.
3. Methods of Assessing Seismic Response Factors
3.1. Single Degree of Freedom (SDOF) Systems: Assessing Demands
Newmark and Hall [29,30] parameterized the reduction factor as a function of ductility. The concept proposed by Newmark focused on SDOF structures covering long period and short period ranges. The strength reduction factor was considered equal to the displacement ductility factor for elastic long period and ductile systems with similar initial stiffness and equal displacement. An equal energy approach was proposed by computing the reduction factor for the short period elastic and inelastic structures. The ductility reduction factor (Rμ) was considered equal to unity for short-period structures. Three period zones covering the short period (<0.2 s), intermediate period (0.2 to 0.5 s), and long period (>1 s) were considered in the study. Newmark and Hall established a relationship with the Rμ factor and verified it as a function of ductility (µ) that was period dependent (T) and proposed equations to evaluate the behavior factor (Rμ).
Krawinkler and Nassar [31] established a relationship to evaluate the force reduction factor using the nonlinear response of SDOF systems. Fifteen ground motions were selected for statistical analysis, representing alluvium soil and rock sites with an earthquake magnitude of 5.7 to 7.7 in the Western USA region. The structural system parameters were examined using the fundamental period (T), strain hardening coefficient (α), the level of yield, and type of inelastic behavior of material (bilinear versus stiffness degrading) apart from the epicentral distance in estimating the sensitivity of strength reduction factors.
Miranda and Bertero [32] evaluated the strength reduction factors using the elastic strength demands under 124 recorded ground motions over different conditions of soil, representing low shear wave velocity. The study was based on different soil conditions representing rock, very soft, and alluvium sites. The average strength reduction factors were evaluated for SDOF systems on each soil group, assuming 5% critical damping under the influence of magnitude and epicentral distance. Soil conditions significantly influenced the strength reduction factors, whereas the earthquake magnitude and its epicentral distance had a negligible effect.
3.2. Multi-Degree of Freedom (MDOF) Systems: Assessing the Capacities
3.2.1. Steel-Braced Structures
Calado et al. [43] evaluated the strength reduction factor (q) of low-rise steel buildings by analyzing three structural systems using a simple steel frame, cantilever, and diagonally braced cantilever buildings. The analysis used a single-story frame to study the cantilever buildings with and without braces, whereas two and three stories were used for frame buildings. Natural and artificial accelerograms represented various seismic loading conditions with 15 to 25 s. durations. The study characterized the failure of the structures using a numerical index based on the damage accumulation. The study concluded that the q factors were very conservative based on their parametric results influenced by different natural frequencies. The study was limited to regular 2D ideal structures with limited accelerograms, and other structural systems were not investigated.
Asgarian and Shokrgozar [45] investigated ductility, overstrength, and response modification factors for the Buckling Restrained Braced Frames (BRBFs) in four to fourteen-story buildings with split X, diagonal, chevron (V and inverted V) bracing configurations. Employing the Iranian National Building Code (2006) and seismic parameters from Iranian Earthquake Resistant Design Codes (2005), the study used two-dimensional IPA, linear dynamic, and nonlinear inelastic dynamic analysis (IDA) with three natural ground motion records. Seismic parameters for BRBF in various configurations were evaluated using the ultimate limit state and the allowable stress methods. The study found conservative seismic response values compared to design codes, indicating decreased overstrength and ductility factors with increased story height. However, the study’s reliance on a limited number of seismic records may not fully capture the variability in input ground motions.
3.2.2. Steel-Framed Structures
Mohammadi [53] considered elastoplastic MDOF models with various dynamic characteristics to evaluate the effect of the deflection amplification factor of steel frame buildings. Buildings with five to fifteen stories were selected and designed, as per AISC design procedures. A two-dimensional nonlinear dynamic analysis assessed the seismic performance under twenty-one accelerograms from ten different earthquakes. The study proposed an approximate approach to evaluate maximum inelastic deformation in a structure using given strengths and deflection amplification factors. The study concluded that the values of the deflection amplification factor for the MDOF systems obtained were more significant than the theoretical values. The study was limited to regular structures with a specific structural system, while other structural systems were not investigated.
Foutch and Wilcoski [54] proposed an approach for determining R factors of steel MRFs using both analytical and previous experimental work. Steel regular buildings were designed and detailed per the International Building Code (IBC). The IDA was performed on each building under 20 near-fault input ground motions to suit the Los Angeles site. The R factors evaluated were conservative, indicating that the values can be reduced considerably. The demand and capacity for the proposed study were based on story drift, which may not be effective on stiff systems, like steel-braced frames and shear walls. The study was limited to the moment-resisting frames, while other structural systems were not investigated.
Izadinia et al. [59] derived and compared the seismic response factors using capacity curves from different pushover analysis methods. Three regular steel frames from the SAC project of three to twenty stories were considered in the study to evaluate the Rμ, Ωo, and R factors. Conventional pushover analysis (CPA) and adaptive pushover analysis (APA) were conducted on each frame, and the results were compared. The study adopted force- and displacement-based adaptive pushover analyses (FAPA and DAPA) using various constants and load patterns. The study identified a relative difference of 16% and 17% of R factor and ductility ratios (µ) between CPA and APA (FAPA or DAPA) due to larger results in APA using different seismic records. The study was limited to regular 2D MRFs with static pushover analysis, while dynamic analysis was not considered.
3.2.3. Steel Walls and Other Structures
Elnashai and Broderick [65] evaluated the strength reduction factors (q) of moment-resisting composite frames. Twenty frames from two, three, six, and ten stories grouped under two sets of ten frames each were analyzed and designed to the member capacity, as per the Eurocodes. The first set represented frames with bare steel columns, while the second set represented partially encased composite columns. Six sets of ground motions with various intensities were employed by performing dynamic analysis to determine the seismic response of the selected frames. The strength reduction factor evaluated for steel moment-resisting and composite frames was exceptionally higher than the code recommendation. The study was conducted on the selected class of structures and recommended further studies on various structures, including braced frames.
Moroni et al. [66] evaluated the seismic response factors for confined masonry buildings by comparing the linear and nonlinear analyses of eight Chilean buildings with three and four stories. Three-dimensional THA was conducted under severe earthquakes representing Chilean and Mexican records to assess the seismic behavior and evaluate the force reduction factor, ductility reduction factors, displacement amplification factors, and ductility ratios. The wall density of the buildings reflecting the building period and the nature of earthquake intensity influenced the strength reduction factor. Lower damage levels were witnessed in the buildings with higher wall density, resulting in lower values of the strength reduction factor.
Kurban and Topkaya [68] assessed the seismic design factors of shear plate shear wall (SPSW) systems with different geometrical characteristics designed as per AISC seismic provisions. They analyzed forty-four SPSW systems from two, four, six, eight, and ten-story buildings, considering the story mass, plate thickness, and plate aspect ratio as the prime variables. Twenty near-field and far-field earthquake records were employed in the 3D finite element analysis to compute the overstrength (Ωo), ductility reduction factor (Rµ), displacement amplification (Cd), and response modification factors (R). Equations were developed for mean values and lower and upper bound ranges, proposing a relationship between the Cd and R factors. Cd increased with an increase in R, which increased with the ISDR values. The study was limited to regular ideal SPSW frames, and further research was recommended on realistic SPSW systems that represent a part of moment-resisting systems.
3.2.4. RC Frame Structures
The strength reduction factors (q) of low-rise- to medium-rise reinforced concrete (RC) buildings were evaluated based on the ductility and overstrength by Kappos [71] for earthquake motions in Southern Europe. Five RC buildings with one to five stories were analyzed using six sets of ground motions representing rock and alluvium sites by scaling the design spectrum to 35%. Two-dimensional IPAs and THAs were employed to evaluate the strength reduction factor dependent on overstrength. They observed that the calculated ductility-dependent part of the strength reduction factor exceeded the code values. The combined reduction factor was reasonably conservative compared to the recommended EC8 values for short and intermediate periods. The study only focused on regular RC buildings, 2D nonlinear analysis, and seismic records representing southern Europe.
Chryssanthopoulos et al. [72] proposed a probabilistic assessment methodology of the strength reduction factors (q) of RC frames designed as per Eurocode 8. The study analyzed a three-bay, ten-story regular RC frame for the medium ductility class, considering various spatial distribution scenarios, failure criteria, random member capacity, and inter-story drift. Adequate safety margins were estimated for the ultimate limit state compared to the service limit state, which depended mainly on the adopted structural criterion. The strength reduction factors were calibrated based on actual behavior factors, considering hazard and ultimate limit state vulnerability curves. The study focused on a 2D regular frame with limited input ground motions.
Maheri and Akbari [74] investigated the seismic behavior factor (R) on a dual system with RC frames and steel bracings, braced with steel X and knee-braced systems. Three regular RC buildings with four, eight, and twelve stories were considered to assess the effect of story height, load sharing of the bracing system, and the type of bracing on the R factor. The design base shear was obtained using a PGA of 0.3 g for the dual system. The elements of the R factor, including the ductility reduction factor and overstrength factor, were evaluated using the 2D IPA based on a study by Mwafy and Elnashai [75]. The results generated from the numerical IPAs were verified with three similar model results obtained from the experimental pushover results [76]. The results were found to be conservative with the code-recommended values. The study was limited to regular RC-braced buildings investigated with 2D pushover analysis without inelastic dynamic analysis.
3.2.5. RC Shear Wall Structures
Challal and Gauthier [87] evaluated the seismic response of RC-coupled shear walls (CSWs) through nonlinear deformation and ductility response, designed as per the NBCC [3] and Canadian Concrete Standards [88]. Five buildings with six, ten, fifteen, twenty, and thirty stories were considered in the design using three Canadian seismic zones. Nonlinear dynamic analysis under five seismic records verified inter-story drift and assessed plastic hinges, displacement, and rotational ductility in walls and coupling beams. The code-specified drift limit was conservative, with lower drifts for taller CSWs. Maximum displacement and ductility demand factors were conservative in comparison with the NBCC limit, which decreased with an increase in the story height. The study was limited to regular structures using 2D analysis with few seismic records and recommended further investigation with different irregularities under a more extensive range of ground motions.
Elnashai and Mwafy [73,75] evaluated Ωo and R on RC wall buildings designed with modern seismic codes. Regular frame-wall buildings with eight stories were designed according to EC8. The seismic design factors were evaluated using IPAs and IDAs with eight natural and artificial records. The calculated R factors were over-conservative compared with the design code, prompting a recommendation to increase R values, especially for structures with high ductility levels at lower PGA values. The study focused on medium-rise buildings designed to Eurocode standards.
Maysam Samadi and Norouz Jahan [64] examined the impact on seismic design parameters such as (a) the response modification factor (R), (b) the deflection amplification factor (Cd), (c) the overstrength factor (Ω), and (d) the damping ratios for tall steel buildings. The study examined regular steel buildings with 28 and 56 stories, featuring steel-braced and RC shear wall cores with outriggers placed at every quarter of the building height, resulting in forty-four building models. Seismic parameters were assessed using the modal response spectrum (MRS), pushover, and nonlinear time history (NLTH) 3D analyses. Including the outriggers increased the response modification factor, overstrength, stiffness, and damping ratios, particularly in the buildings with RC core walls, while reducing ductility in both systems. Their study also identified inadequacy in the code-recommended Cd values.
The previous studies conducted since 2001 on assessing the seismic response factors of MDOF systems for RC shear wall structures were based on 2D analytical works using IPAs and IDAs. Earlier studies were based on regular shear wall buildings, and the evaluations of seismic response factors were based on unidirectional seismic loading. In earlier studies, irregular shear wall buildings under the effect of bi-directional loading employing 3D inelastic analysis were not considered.
This entry is adapted from the peer-reviewed paper 10.3390/buildings14010247
This entry is offline, you can click here to edit this entry!
