Radiated Emissions from Printed Circuit Boards with MR: Comparison
View Latest Version
Please note this is a comparison between Version 3 by Dean Liu and Version 4 by Dean Liu.

With the growth of the radioelectronic complexity and the demand for cutting edge devices, the need to protect them and increase their reliability is also rising. There are many methods to provide this. Modal reservation is one of the most effective, reliable, and least complicated methods used nowadays. Using this method in tracing and mounting of printed circuit boards can guarantee both electromagnetic compatibility (by using modal filtering) and reliability (by using the cold redundancy) of the final electronic device.

  • electromagnetic compatibility
  • redundancy
  • modal reservation

1. Introduction

A highly reliable system requires that engineers should increase component reliability (for example, changing the component material) or apply redundancy methods using the existing resources without violating the system’s cost, weight, and volume constraints [1][2]. The second choice is preferable because of its simplicity [3] and its first implementation is referred in the literature to the work conducted in 1956 [4]. Redundancy is likely to be applied in critical systems where uninterrupted operation is required, and the maintenance is difficult and expensive, for example in the case of onboard radioelectronic systems. In this case, the mission time of communication satellites may last for more than 10 years [5]. Systems or subsystems may be reserved in active or inactive mode with lower failure rates, since the reserved components will be in standby mode until failure [6]. In addition, those reservation types can be combined and divided into sub-types: parallel and k-out-of-n active reservation types, and the cold, hot, and warm standby reservation types [7][8]. Reservation can be employed not only to improve a system’s reliability, but also its efficiency [9]. Moreover, reservation is considered to be a quick solution to achieve any desirable level of reliability in the early design stage [10]. Since reservation can be performed in different forms, the optimized design of the system can be obtained by reaching the balance between the system’s cost and reliability. Several works have been devoted to developing mathematical models to determine system reliability [11][12][13], even in real-time applications [14]. Reservation in all its types has been used in different fields. It has been employed in information protection [15], memristive devices [16], and in neural networks [17]. It has even been employed in nanotechnology which has a high defect rate [18][19]. Moreover, this technique is also popular in space [20][21] and aviation [22] applications, and in modern systems related to unmanned aerial vehicles, for example to protect their datalink [23]. Reservation is also used in communication applications [24]. The printed circuit board (PCB) failures can lead to critical problems and have various causes such as aging, heating, and insulting [25]. To manage these failures, reservation should be commonly used in PCB design. Several works have described the use of this technique for this purpose [26][27]. However, reservation is not always useful, for example, if the system is not designed to deal with artificial or natural electromagnetic interference (EMI), that can be caused by reservation itself [28]. Thus, ensuring electromagnetic compatibility (EMC) in PCB design, even when the design includes reservation, is unavoidable when dealing with electromagnetic disturbances [29][30]. Some researchers have tried to increase the reliability of PCBs with reservation taking EMI into account [31], but there was no comprehensive way to do this [32][33]. In general, one can say that all traditional ways to deal with EMI consequences are not highly effective especially against ultrashort pulses (USP) [34].
Modal reservation (MR) is a method that can both improve the reliability of radioelectronic devices using cold standby reservation and ensures their EMC by using the so-called technique of modal filtering [35]. Modal filtering is used to protect radioelectronic devices from USPs; it is also used as a method to detect and diagnose contactless electrical connections in hidden walls [36]. MR can also be used in different applications. However, the most important application of this method is in critical and onboard radioelectronic devices. For example, MR is used to increase noise immunity of digital signal processing units [37] and power systems [38] of spacecraft autonomous navigation systems. This method has several types: based on the multiplicity of redundancy (single, dual, triple, … n-tuple) [39], based on the structure symmetry (symmetrical and asymmetrical) [40], and based on the target item (PCB trace or components, cable, dielectric) [41]. Most of these types have been extensively investigated in terms of conducted emissions. To fully explain the mechanism of this method and interpret its consequences, it is also necessary to study its characteristics in terms of radiated emissions (RE). This has been reflected in only a few works [42][43][44][45], where such investigations were performed using only the analysis of simulation results and without taking into account climate impacts.

2. Recent Research on RE from PCBs with MR

The following is a review of the recent studies and their results related to the investigation of REs from structures with MR. Researchers carried out the research to estimate and evaluate the efficiency of MR in terms of RE. All the results have been presented at different conferences and will soon be published. In addition, they form a necessary base to investigate the effect of climate conditions on REs from PCBs. As mentioned above, the RE from PCBs with MR has not been studied in detail except for a few works. Recently in [42], the authors proposed an algorithm based on the combined use of quasi-static (to calculate the current along the conductors) and electrodynamic (to calculate the field components) approaches to obtain the far field electric intensity from a test structure with MR. This algorithm provided sufficiently accurate results with less computational costs compared to the separate use of each approach. The current distribution and the radiation pattern obtained using the electrodynamic approach [46] and those obtained using the proposed algorithm were compared and showed good consistency. The algorithm was also tested on wires with dielectric insulation [43]. The study demonstrated that increasing the dielectric permittivity, the wave impedance of a single wire decreased, and the current amplitudes increased compared to their values from a wire without insulation. An asymmetrical radiation pattern and a change in the main maximum direction were also revealed and explained by the current amplitude growth in the first segment of the wire and the current phase shift along the wire. The same observations were noticed in the case of coupled wires, but increasing the insulation dielectric permittivity, the asymmetry of the radiation pattern decreased. The first analysis of the MR implementation effect on PCB RE was presented in [45] using the above proposed algorithm and comparing the results [44] obtained using the finite difference time domain method in another software. These preliminary simulation-based studies on a test structure proved that the use of a single MR reduced the RE by up to 10 GHz because of the electromagnetic coupling between the reserved and reserving conductors in an inhomogeneous dielectric medium. This is explained by the appearance of even and odd mode currents in opposite phases, which decreased the currents in the conductors and electric field strength from the contribution of these currents in the far field at certain frequencies. Thus, at these frequencies, a decrease in RE, as well as a decrease in susceptibility to radiated electromagnetic fields, can be expected. On the other hand, there are frequencies or directions where the opposite is noted, which might be associated with resonance shifts and field redistribution. To prove the above conclusions, an experimental study in the far or near field is required. However, these types of experiments are expensive, not informative (for example, in the far zone for such structures), and not feasible for academic purposes. Therefore, researchers recently started the preliminary studies using another approach to estimate the RE from PCBs with MR. Since there are various types of MR tracing and layouts, researchers started with the simplest MR type from (No. 1). To imitate the layout of a PCB without MR, researchers used the structure of a single microstrip line, and to imitate the layout of a PCB with MR, the structure of coupled microstrip lines was used. Figure 1 shows the cross-sectional parameters of the structures under study. The notation (l) refers to the length of each conductor, (w) to the width, (t) to the thickness of each conductor, and (s) refers to the distance between the conductors. The substrate thickness is referred to as (h). The material used was foiled heat-resistant fiberglass [47] known in Russia as (STF), with a relative dielectric permittivity (εr) of 4.7–5.5 and dielectric loss tangent (tgδ) of 0.017–0.035 at the frequency of 1 MHz. The notation (d) refers to the distance from the edge of the dielectric to the active conductor. The equivalent schematic diagrams of these PCBs are presented in Figure 2 and their manufactured prototypes in Figure 3. The PCBs had dimensions of 98 × 98 mm. The distance of the margins from the tap to the subminiature version A of connectors (SMA) was 10 mm at each side.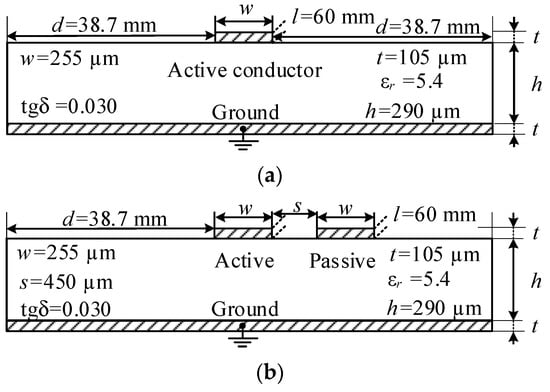
Symmetry 14 02466 g020 550
Figure 1. The cross-sectional parameters of the structures without (a) and with (b) MR.
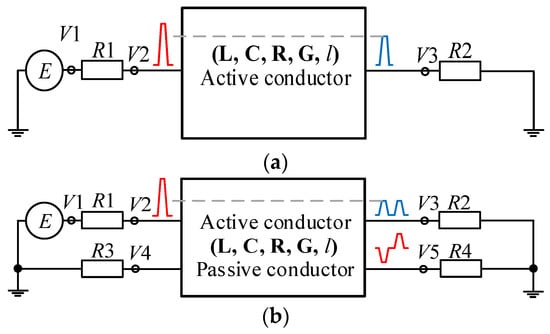
Symmetry 14 02466 g021 550
Figure 2. The structures without (a) and with (b) MR: equivalent schematic diagrams where R = 50 Ω and E is a harmonic electromotive source with an amplitude of 1 V.
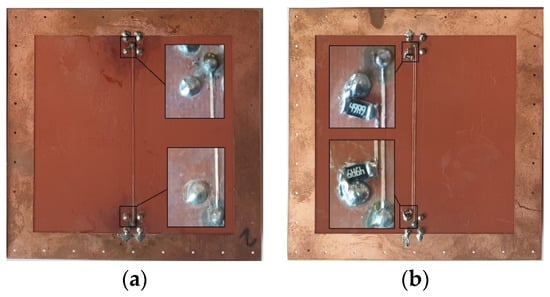
Symmetry 14 02466 g022 550
Figure 3. The PCB prototypes without (a) and with single (b) MR.
Firstly, researchers modeled these structures in the TALGAT software [48] and obtained the frequency dependences of their S-parameters [49]. The simulation was carried out at different values of εr, without taking the losses in conductors and dielectric into account. researchers measured the frequency dependences of the S-parameters using the vector circuit analyzer R&S ZVA 40 (two ports) which was connected to the PCB SMA connectors via the R&S ZVZ195 and Semflex 60637 measuring cables passing in the climate heat–cold chamber SPEC SU-262 to fix the temperature at 23 °C (Figure 4). Then, researchers compared the calculated and measured results. They showed good agreement, with an average difference between them being up to 5 dB. Researchers found that the transmission ratio for the PCB with MR was lower than without it because of the modal decomposition effect. However, this reduction will not affect the useful signal as the values of the transmission ratio remained higher than −3 dB (for the pass band) except for some frequencies of the frequency range under study, due to the frequency shift. After this, researchers decided that it was also necessary to have information about the MR effect in the case after short (SC) and open (OC) circuit failures. Therefore, researchers considered two other structures with the same parameters as in Figure 1. Their equivalent schematic diagrams and manufactured prototypes are presented in Figure 5 and Figure 6, respectively. In the same manner, the frequency dependences of the S-parameters for all the boards measured and calculated at different εr and tgδ values were obtained and compared and demonstrated consistency [50]. Researchers estimated the changes in |S21| and |S11| after SC and OC failures compared to those obtained for the PCB with MR (0.09, 3.9 dB and 0.16, 5.31 dB) and without it (0.35, 5 dB and 0.53, 2.86 dB).
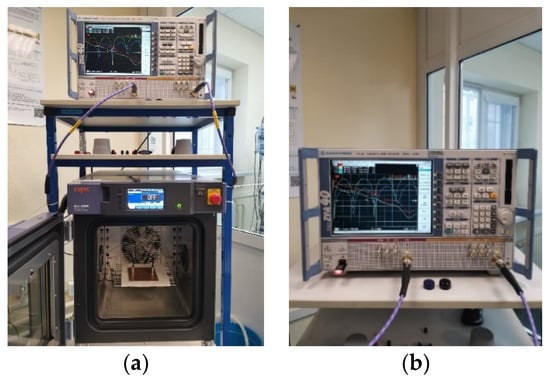
Symmetry 14 02466 g023 550
Figure 4. The climate heat-cold chamber (a) and the vector network analyzer (b).
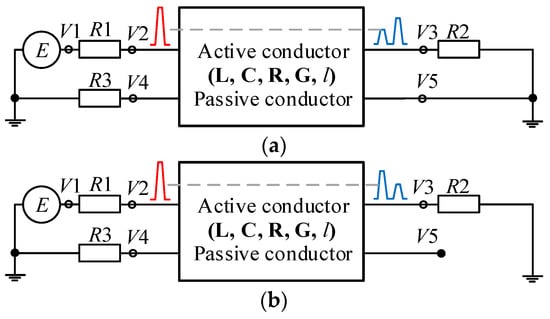
Symmetry 14 02466 g024 550
Figure 5. The structures with single MR after SC (a) and OC (b) failures: equivalent schematic diagrams where R = 50 Ω and E is a harmonic source with an amplitude of 1 V.
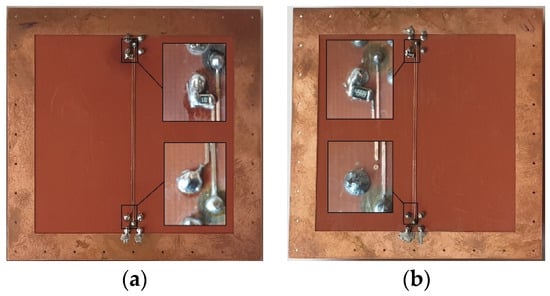
Symmetry 14 02466 g025 550
Figure 6. The PCB prototypes with MR after SC (a) and OC (b) failures.
After this, the RE levels from PCBs with MR were estimated before and after experimental failure. To do this, researchers used classical [51] and miniature [52] TEM-cells (Figure 7). Such TEM-cells are based on the coaxial transmission line of a rectangular cross-section and are widely used for testing interfering emissions and immunity of integrated circuits. When a generator supplies a signal with pre-defined characteristics to the input of the tested device placed in the regular part of the cell, a transverse electromagnetic wave propagates in the inner space of the cell forming a homogeneous electromagnetic field. Then, the signal can be absorbed by a matched load located on the opposite side of the cell next to the microwave connectors. The first resonance frequency of the TEM-cell is determined by its geometric parameters, which in turn determines the upper boundary of the operating TEM-cell frequency range.
Symmetry 14 02466 g026 550
Figure 7. The classical (a) and miniature (b) TEM-cells.
Using both TEM-cells, the classical (up to 2 GHz) [53], and the miniature (up to 5 GHz) [54], the frequency dependences of the voltage amplitude levels at the central conductor (CC) for all boards were obtained at room temperature using the VNA R&S ZVA 40 (four ports), Cascade microtech measurement cables (124-084-B), and RF Cable Assemblies 40 GHz 25”Lng VNA Conn Ends 2.92 mm (FD0BS0HR025.0) (Figure 8). The measured results obtained after failures were compared to those obtained before them. The comparison results demonstrated that the voltage amplitudes at the TEM-cell CC input and output for the PCB with MR were lower than for the PCB without MR by about 10–20%, except at some frequencies of the studied domain, because of the frequency shift. This means that the level of RE from the devices was also lower because it is proportional to the voltage amplitudes, which gives the PCB with MR an advantage over the PCB without MR since it can lessen RE, especially at high frequencies. After failure, it was found that, at low frequencies, failures had almost no effect on the level of RE. However, at high frequencies, after OC, it became even higher for the PCB without MR, and after SC failure, it was lower, even before failure.
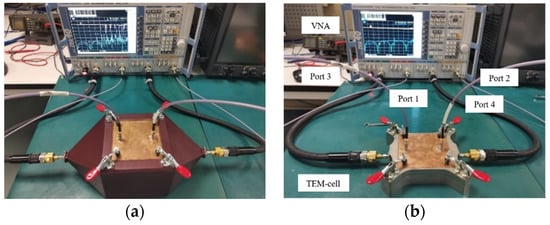
Symmetry 14 02466 g027 550
Figure 8. The connection of VNA ports to the input and output of the classical (a) and miniature (b) TEM-cell CC (Ports 3 and 4), and to the PCBs (Ports 1 and 2).
Next, researchers obtained the frequency dependences of the S-parameters for all PCBs at different temperatures in the climate chamber [55][56][57]. Estimating these characteristics can provide a prediction about the operability of these structures under any climate conditions. researchers found that, in general, the S-parameters of the prototypes decreased with increasing temperature and increased with decreasing temperature, compared to the results obtained at room temperature over the entire frequency range except for the resonance frequencies. researchers observed that at low temperatures, there was a noticeable shift in the resonances towards higher frequencies with a decrease in the |S21| values and an increase in the |S11| values. At high temperatures, the shift in resonances was observed towards the low frequencies with a decrease in the |S21| values and increase in the |S11| values. This is explained by the change in characteristics of the transmission line with changing temperatures, because of the change in the dielectric permittivity and the geometric parameters of the transmission line. In general, the transmission coefficients for the PCBs with MR before and after failure were smaller than those for the PCBs without MR. After failure they were less than before failure, in almost all of the investigated frequency range. The reflection coefficients after the SC failure were, in general, lower than before failure and without MR, and after OC failure, they were higher than before failure and for PCBs without MR. Moreover, the effect of MR remained after failure without affecting the useful signal except at high temperatures where the operating frequency range of the useful signal decreased considerably. Furthermore, there was a shift in resonances by up to 0.4 GHz towards the low frequencies after SC and towards higher frequencies after OC failure. This happens because of the reflection at the line ends, which increases the difference between them near the resonance frequencies.

References

  1. Trivedi, K.; Bobbio, A. Reliability and Availability Engineering: Modeling, Analysis, and Applications; Cambridge University Press: Cambridge, UK, 2017.
  2. Amari, S.V.; Dill, G. Redundancy optimization problem with warm-standby redundancy. In Proceedings of the Annual Reliability and Maintainability Symposium (RAMS), San Jose, CA, USA, 25–28 January 2010; pp. 1–6.
  3. Kuo, S.V.W.; Prasad, V.R.; Tillman, F.A.; Hwang, C.L. Optimal Reliability Design: Fundamentals and Applications; Cambridge University Press: Cambridge, UK, 2001.
  4. Von Neumann, J. Probabilistic logics and the synthesis of reliable organisms from unreliable components. In Automata Studies; Shannon, C.E., McCarthy, J., Eds.; Princeton Univ. Press: Princeton, NJ, USA, 1956; pp. 43–98.
  5. Chen, D.M. Satellite Engineering Series: Communications Satellite Payload Technology; China Astronautic Publishing House: Beijing, China, 2001.
  6. Coit, D.W. Maximization of System Reliability with a Choice of Redundancy Strategies. IIE Trans. 2003, 35, 535–543.
  7. Grida, M.; Zaid, A.; Kholief, G. Repairable 3-out-of-4: Cold standby system availability. In Proceedings of the Annual Reliability and Maintainability Symposium (RAMS), Orlando, FL, USA, 23–26 January 2017; pp. 1–6.
  8. Lobur, M.; Stefanovych, T.; Shcherbovskykh, S. Modelling of type I and II errors of switching device for systems with hot and cold redundancy based on two-terminal dynamic fault tree. In Proceedings of the 14th International Conference of the Experience of Designing and Application of CAD Systems in Microelectronics (CADSM), Lviv, Ukraine, 21–25 February 2017; pp. 19–21.
  9. Li, Y.; Zhang, Y.; Cao, R.; Liu, X.; Lv, C.; Liu, J. Redundancy design of modular DC solid-state transformer based on reliability and efficiency evaluation. CPSS Trans. Power Electron. Appl. 2021, 6, 115–126.
  10. Sankaraiah, G.; Raghunatha Reddy, Y.; Umasankar, C.; Sarma, B.D. Design and optimization of an Integrated Reliability redundancy system with multiple constraints. In Proceedings of the 2nd International Conference on Reliability, Safety and Hazard—Risk-Based Technologies and Physics-of-Failure Methods (ICRESH), Mumbai, India, 14–16 December 2010; pp. 118–122.
  11. Pan, D. Study on Optimization of System Reliability Redundancy Based on Hybrid Intelligent Algorithm. In Proceedings of the International Conference on Environmental Science and Information Application Technology, Wuhan, China, 4–5 July 2009; pp. 560–563.
  12. Boland, P.J.; El-Neweihi, E. Component redundancy vs system redundancy in the hazard rate ordering. IEEE Trans. Reliab. 1995, 44, 614–619.
  13. Shcherbovskykh, S.; Stefanovych, T. Modelling features of type I and II errors of switching device for system with double hot and double cold redundancy based on two-terminal dynamic fault tree. In Proceedings of the 14th International Conference on Advanced Trends in Radioelecrtronics, Telecommunications and Computer Engineering (TCSET), Lviv, Ukraine, 20–24 February 2018; pp. 753–756.
  14. Neves, F.G.R.; Saotome, O. Comparison between Redundancy Techniques for Real Time Applications. In Proceedings of the Fifth International Conference on Information Technology: New Generations (ITNG), Las Vegas, NV, USA, 7–9 April 2008; pp. 1299–1300.
  15. Peterson, W.; Weldon, E. Error-Correcting Codes, 2nd ed.; MIT Press: Cambridge, MA, USA, 1972.
  16. Zhu, X.; Xu, H.; Long, H.; Li, Q.; Li, Z.; Liu, H.; Wang, Y. Memristive Stateful Logic with N-Modular Redundancy Error Correction Design towards High Reliability. In Proceedings of the 5th IEEE Electron Devices Technology & Manufacturing Conference (EDTM), Chengdu, China, 8–11 April 2021; pp. 1–3.
  17. Sun, Z.; Ambrosi, E.; Bricalli, A.; Ielmini, D. Logic Computing with Stateful Neural Networks of Resistive Switches. Adv. Mater. 2018, 30, 1802554.
  18. Hansen, P.; Linton, M.; Mayo, R.; Murphy, M.; Patterson, D. Introduction to the iAPX 482 architecture. ACM SIGARCH Comput. Archit. News 1982, 10, 17–26.
  19. Namazi, A.; Nourani, M. Gate-Level Redundancy: A New Design-for-Reliability Paradigm for Nanotechnologies. IEEE Trans. Very Large-Scale Integr. (VLSI) Syst. 2010, 18, 775–786.
  20. Xiong, X.; Zhao, H.T.; Hu, T.B. Research on Redundancy Solution of Satellite Transponders Based on Reliability Analysis. In Proceedings of the International Conference on Quality, Reliability, Risk, Maintenance, and Safety Engineering (QR2MSE), Zhangjiajie, China, 6–9 August 2019; pp. 689–694.
  21. Sklaroff, J.R. Redundancy Management Technique for Space Shuttle Computers. IBM J. Res. Dev. 1976, 20, 20–28.
  22. Xiao, C.; Deng, L. Reliability Research on Airborne Dual Redundancy of Electrical Wiring Interconnection System. In Proceedings of the 11th International Symposium on Computational Intelligence and Design (ISCID), Hangzhou, China, 8–9 December 2018; pp. 137–140.
  23. Zhang, D.; Zhao, M.; Cheng, E.; Chen, Y. GPR-Based EMI Prediction for UAV’s Dynamic Datalink. IEEE Trans. Electromagn. Compat. 2021, 63, 19–29.
  24. Rentschler, M.; Laukemann, P. Performance analysis of parallel redundant WLAN. In Proceedings of the IEEE 17th International Conference on Emerging Technologies & Factory Automation (ETFA), Krakow, Poland, 17–21 September 2012; pp. 1–8.
  25. Slee, D.; Stepan, J.; Wei, W.; Swart, J. Introduction to printed circuit board failures. In Proceedings of the IEEE Symposium on Product Compliance Engineering, Toronto, ON, Canada, 26–28 October 2009; pp. 1–8.
  26. Labib, M.G.; Mahmoud, D.G.; Alkady, G.I.; Adly, I.; Amer, H.H.; Daoud, R.M.; ElSayed, H.M. Heterogeneous Redundancy for PCB Track Failures: An Automotive Example. In Proceedings of the 14th International Conference on Computer Engineering and Systems (ICCES), Cairo, Egypt, 17 December 2019; pp. 189–194.
  27. Chen, H.C.; Bai, Y.W. Improvement of High-Current Density PCB Design With PSU Load Balance and Redundancy on a High End Server System. Can. J. Electr. Comput. Eng. 2014, 37, 203–211.
  28. Van Waes, J.; Vankeirsbilck, J.; Pissoort, D.; Boydens, J. Functional safety standard’s techniques and measures in light of electromagnetic interference. In Proceedings of the XXVI International Scientific Conference Electronics (ET), Sozopol, Bulgaria, 13–15 September 2017; pp. 1–4.
  29. Pissoort, D.; Armstrong, K. Why is the IEEE developing a standard on managing risks due to EM disturbances? In Proceedings of the IEEE International Symposium on Electromagnetic Compatibility (EMC), Ottawa, ON, Canada, 25–29 July 2016; pp. 78–83.
  30. Van Waes, J.; Vanoost, D.; Vankeirsbilck, J.; Lannoo, J.; Pissoort, D.; Boydens, J. Resilience of Error Correction Codes Against Harsh Electromagnetic Disturbances: Fault Mechanisms. IEEE Trans. Electromagn. Compat. 2020, 62, 1017–1027.
  31. Luo, S.; Batarseh, I. A review of distributed power systems. Part II. High frequency AC distributed power systems. IEEE Aerosp. Electron. Syst. Mag. 2006, 21, 5–14.
  32. Pissoort, D.; Lannoo, J.; van Waes, J.; Degraeve, A.; Boydens, J. Techniques and measures to achieve EMI resilience in mission- or safety-critical systems. IEEE Electromagn. Compat. Mag. 2017, 6, 107–114.
  33. Lannoo, J.; Degraeve, A.; Vanoost, D.; Boydens, J.; Pissoort, D. Study on the use of different transmission line termination strategies to obtain EMI-diverse redundant systems. In Proceedings of the IEEE International Symposium on Electromagnetic Compatibility and 2018 IEEE Asia-Pacific Symposium on Electromagnetic Compatibility (EMC/APEMC), Suntec City, Singapore, 14–18 May 2018; pp. 210–215.
  34. Mora, N.; Vega, F.; Lugrin, G.; Rachidi, F.; Rubinstein, M. Study and Classification of Potential IEMI Sources. Syst. Des. Assess. Notes 2014, 41, 1–92.
  35. Gazizov, T.R.; Zabolotsky, A.M. New approach to EMC protection. In Proceedings of the 18th International Zurich Symposium on Electromagnetic Compatibility, Munich, Germany, 24–28 September 2007; pp. 273–276.
  36. Orlov, P.; Gazizov, T. Contactless Modal Phenomena Based Approach to Detecting, Identifying, and Diagnosing of Electrical Connections. Complexity 2018, 2018, 5081684.
  37. Orlov, P.E.; Medvedev, A.V.; Sharafutdinov, V.R.; Kalimulin, I.F. Quasistatic simulation of ultrashort pulse propagation in the spacecraft autonomous navigation system circuit with modal reservation. In Proceedings of the International Multi-Conference on Engineering, Computer and Information Sciences (SIBIRCON), Novosibirsk, Russia, 18–22 September 2017; pp. 495–500.
  38. Orlov, P.E.; Medvedev, A.V.; Sharafutdinov, V.R. Quasistatic Simulation of Ultrashort Pulse Propagation in the Spacecraft Autonomous Navigation System Power Circuit with Modal Reservation. In Proceedings of the 19th International Conference of Young Specialists on Micro/Nanotechnologies and Electron Devices (EDM), Erlagol, Russia, 29 June–3 July 2018; pp. 1–6.
  39. Orlov, P.E.; Buichkin, E.N.; Belousov, A.O.; Gazizov, T.R. Method of lay-out of a multilayer PCB for circuits with triple reservation. In Proceedings of the International Siberian Conference on Control and Communications (SIBCON), Astana, Kazakhstan, 29–30 June 2017; pp. 1–4.
  40. Belousov, A.O.; Chernikova, E.B.; Samoylichenko, M.A.; Medvedev, A.V.; Nosov, A.V.; Gazizov, T.R.; Zabolotsky, A.M. From Symmetry to Asymmetry: The Use of Additional Pulses to Improve Protection against Ultrashort Pulses Based on Modal Filtration. Symmetry 2020, 12, 1117.
  41. Orlov, P.E.; Buichkin, E.N. Quasistatic and electromagnetic simulation of interconnects of printed circuit boards with modal reservation. In Proceedings of the 18th International Conference of Young Specialists on Micro/Nanotechnologies and Electron Devices (EDM), Erlagol, Russia, 29 June–July 2017; pp. 54–58.
  42. Hasan, A.A.; Kvasnikov, A.A.; Gazizov, T.R. Approach to Estimation of Radiated Emission from Circuits with Modal Reservation. In Proceedings of the 21st International Conference of Young Specialists on Micro/Nanotechnologies and Electron Devices (EDM), Chemal, Russia, 29 June–July 2020; pp. 169–173.
  43. Hasan, A.A.; Gazizov, T.R. Estimation of the Radiated Emission from a Single and Coupled Wires with Insulation above the Ground Plane. In Proceedings of the IEEE 22nd International Conference of Young Professionals in Electron Devices and Materials (EDM), Souzga, the Altai Republic, Russia, 30 June–4 July 2021; pp. 149–152.
  44. Alhaj Hasan, A.; Zhechev, Y.S.; Gazizov, T.R. Estimation of radiated emissions from a structure with a single modal reservation. J. Phys. Conf. Ser. 2021, 1862, 012003.
  45. Hasan, A.A.; Gazizov, T.R. Comparing the Estimates of the Radiated Emission from a Structure with Modal Reservation by Two Approaches. In Proceedings of the IEEE 22nd International Conference of Young Professionals in Electron Devices and Materials (EDM), Souzga, the Altai Republic, Russia, 30 June–4 July 2021; pp. 145–148.
  46. Harrington, R.F. Matrix methods for field problems. Proc. IEEE 1967, 55, 136–149.
  47. Official Website of Elmika Company LLC. STF Technical Characteristics. Available online: http://elmica.ru/assets/files/listovki/folgirovannyj-steklotekstolit-2016.pdf (accessed on 30 September 2022).
  48. Kuksenko, S.P. Preliminary results of TUSUR University project for design of spacecraft power distribution network: EMC simulation. IOP Conf. Ser. Mater. Sci. Eng. 2019, 560, 012110.
  49. Alhaj Hasan, A.; Gazizov, T.R. Studying the Features of a PCB with Modal Reservation in the Frequency Domain Using TALGAT. In Proceedings of the IEEE Ural-Siberian Conference on Biomedical Engineering, Radioelectronics and Information Technology (USBEREIT), Yekaterinburg, Russia, 19–21 September 2022; pp. 102–107.
  50. Alhaj Hasan, A.; Gazizov, T.R. Frequency Characteristics of PCB with Modal Reservation before and after Failure Using TALGAT. In Proceedings of the IEEE 23rd International Conference of Young Professionals in Electron Devices and Materials (EDM), Altai, Russia, 30 June–4 July 2022; pp. 140–146.
  51. Komnatnov, M.E.; Gazizov, T.R. TEM Chamber. Patent No. 2,606,173, 28 December 2015.
  52. Demakov, V.; Komnatnov, M.E. Improved TEM-cell for EMC tests of integrated circuits. In Proceedings of the IEEE International Multi-Conference on Engineering, Computer and Information Sciences (SIBIRCON), Novosibirsk, Russia, 18–22 September 2017; pp. 399–402.
  53. Alhaj Hasan, A.; Gazizov, T.R. Measuring the Level of Radiated Emissions from PCBs with Modal Reservation before and after Failure. In Proceedings of the IEEE 23rd International Conference on Actual Problems of Electron Devices Engineering (APEDE), Saratov, Russia, 22–23 September 2022; pp. 13–16.
  54. Alhaj Hasan, A.; Gazizov, T.R. TEM-cell Measurements of the Radiated Emissions from PCBs with Modal Reservation before and after Failure. In Proceedings of the IEEE The International Ural Conference on Electrical Power Engineering (UralCon), Magnitogorsk, Russia, 23–25 September 2022; pp. 196–200.
  55. Alhaj Hasan, A.; Gazizov, T.R. Measuring Frequency Characteristic of PCBs with Modal Reservation under Climatic Impact. In Proceedings of the IEEE 2nd International Conference Problems of Informatics, Electronics and Radio Engineering (PIERE), Novosibirsk, Russia, 11–13 November 2022.
  56. Alhaj Hasan, A.; Gazizov, T.R. Measuring Frequency Characteristic of PCBs with Modal Reservation under Climatic Impact before and after Short Circuit Failure. In Proceedings of the IEEE 23rd International Conference on Dynamics of systems, mechanisms and machines, Omsk, Russia, 15–17 November 2022.
  57. Alhaj Hasan, A.; Gazizov, T.R. Measuring Frequency Characteristics of PCBs with Modal Reservation before and after Open Circuit Failure under Climatic Impact. In Proceedings of the IEEE International Conference of Siberian Conference on Control and Communications (Sibcon), Tomsk, Russia, 17–19 November 2022.
More
© Text is available under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/)
ScholarVision Creations
Feedback