The complete mining cycle of a mine can be divided into two categories: the development period and production period. During development, a series of tunnels from the surface to the ore body is excavated to establish a passage between the surface and ore body for transporting personnel, equipment, materials, ore and waste rock, as well as creating appropriate conditions for mining and forming an independent system for hoisting, transportation, ventilation, drainage, power supply, and water supply, within these tunnels. The main material to be transported is generally rock, which has a low economic value. Investments during this period are primarily used to purchase equipment and hire manpower; therefore, the mine operates at a negative profit during the development period. After mine development and any necessary planning are completed, the mine enters the production period. The haulage is primarily composed of ore with high economic value. The production period is divided into three periods: ramp-up, steady state and tailing-off periods. Early revenue is to offset the costs from the development period, and mines begin to make profit during the middle-term; however, in the last term, a portion of the profit must be used to maintain the stability of the ore body. In order to maximize profit during the production period, Topal ^[1] and Sandanayake et al. ^[2] considered mining process allocation, comprehensive scheduling, stope design, and construction of system planning.

Different mining cycles correspond to different mining plans. Wu and Li ^[3] pointed out that mine production planning can be divided into long-term, medium-term, and short-term planning according to the length of the planning time. The length of the time period for each planning type is different, leading to different optimization goals. Long-term planning defines the company’s long-term goals, emphasizing timeliness and strategy, and fully considers market changes; medium-term planning is guided by the long-term goals to complete the annual plan; and short-term planning can be divided into four plan types according to the length of time: seasonal planning, monthly planning, weekly planning, and daily planning, including product output, operation arrangement, labor allocation, grade control, product sales, and haulage cost. Planning the mine location and scheduling the mining operations is conducted during the production period. In short-term planning, resource planning is aligned to the mining cycle, and daily planning is generally aimed at equipment dispatch planning.

Previous scholars’ research on short-term production in underground mines only divided the mining areas of the mine at each period, and did not involve the actual mining process. The production dispatching planning of underground mining vehicles is a short-term production planning process that is different from short-term resource planning. Only after short-term resource planning is completed, can production dispatching planning begin. However, most short-term production planning does not involve precise equipment dispatch path optimization research. 

2. Optimization Results

First, the actual production data of the mine are brought into the bee colony algorithm to solve the short-term resource plan. Then, according to the spatial location of the stopes and ore passes to be mined in the short-term resource plan, the time parameters in the haulage equipment dispatch plan model are obtained, when are subsequently brought into non-dominated sorting genetic algorithm to calculate the haulage equipment scheduling scheme.

2.1. Resource Plan

The calculation results of the resource planning model are shown in Table 1. The number ‘1′ indicates that the site can be mined during the plan period; on the contrary, the number ‘0’ indicates that the site cannot be mined during the plan period. The number of the ore blocks in Table 1 are shown in Figure 51. For example, (1, 1, 4, 3) means the ore block numbered (4, 3) in the plan view in (1, 1) sublevel.

Figure 51.

 Simple spatial model of ore blocks.

Table 1. Calculation results.

Number	Result	Total Storage/t	Remaining Storage/t	Grade/%
(1, 1, 4, 3)	1	2883.92	2883.92	24.03
(1, 1, 8, 3)	1	2951.56	2951.56	61.74
(1, 1, 11, 3)	1	2631.26	2631.26	44.26
(1, 1, 1, 2)	1	2951.37	2951.37	36.46
(1, 1, 6, 2)	1	2601.16	2601.16	49.15
(1, 1, 9, 1)	1	2667.56	2667.56	49.28
(1, 1, 12, 1)	1	2916.49	2916.49	56.74
(2, 1, 1, 3)	1	2643.29	2643.29	35.77
(2, 1, 4, 3)	1	2708.67	2708.67	42.90
(2, 1, 10, 3)	1	2947.29	2947.29	31.48
(2, 1, 2, 1)	1	2938.19	2938.19	52.25
(2, 1, 5, 1)	1	2646.24	2646.24	54.64
(2, 1, 8, 1)	1	2951.56	2951.56	61.74
(2, 1, 11, 1)	1	2969.06	2969.06	44.26
(2, 1, 7, 3)	1	2671.67	2671.67	48.00
Others	0	/	/	/

2.2. Haulage Equipment Dispatch Plan

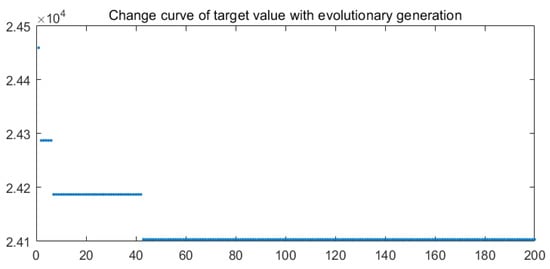
In order to prove the superiority of the non-dominated sorting genetic algorithm, the calculation results of the non-dominated sorting genetic algorithm are compared with the results of the traditional genetic algorithm. Solving a multi-objective problem using the traditional genetic algorithm method involves transforming the multi-objective problem into a single objective by weighting to select the superior individual and generating the superior scheme. Since the single running time of the electric locomotive is longer than that of the scraper, the proportion of F2 is relatively low, at 10%, while the proportion of F1 is 90%. The change curve of the target value in the calculation process with the evolutionary generation is shown in Figure 72. 

Figure 72.

 Change curve of the target value with evolutionary generation.

3. Conclusions

Based on 0-1 integer programming, combined with the production needs of underground mines under study, a short-term resource plan optimization model for underground mines is constructed with the maximum profit as the primary objective. The constraints of this model conform to the actual conditions of the mine. The artificial bee colony optimization algorithm is used to solve the model, and the requirements of the artificial bee colony for the nectar source are restricted by the constraints. The neighborhood search method can be used to find the optimal nectar source over a wide range. The addition of the following bee and scout bee can improve the optimization results. Then, based on the short-term resource plan, considering the constraints of each link in the underground mine ore haulage process, the shortest total equipment wait time is used as the objective function to construct an optimization model for haulage equipment between stopes, which can more completely describe the process of dynamic allocation of the scraper and electric locomotives. Using a non-dominated sorting genetic algorithm, a more accurate Pareto optimal solution set can be obtained by introducing elite selection strategy and selection methods based on reference points.