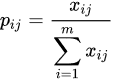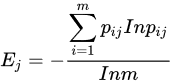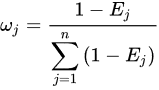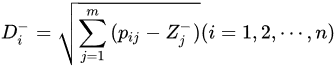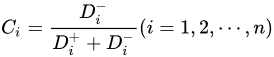The mine ventilation system is an indispensable component to improve coal mining efficiency and ensure the safety production. Only by clearly grasping the comprehensive evaluation quality of ventilation system can effective countermeasures be formulated. This paper establishes an evaluation index system for mine ventilation system by combining qualitative survey with quantitative research. Specifically, the primary indicators are screened through R-type clustering and coefficient of variation method. The weight of each index is determined by the entropy weight method. Moreover, the TOPSIS method are used to evaluate the quality of the mine ventilation system. Finally, this evaluation system is used to evaluate the ventilation renovation project in the production mining area of the Sihe mine. The evaluation results verify the effectiveness of the establishment of mine ventilation evaluation index system and evaluation methods.
1. Introduction
Coal has expanded globally as one of the foundational energy sources in the last few decades
[1]. With the intention of sustainably using the limited coal resources, improving coal mining efficiency and mining safety are key influencing factors
[2]. The premise of improving coal mining efficiency is to ensure the efficient operation of underground ventilation system. Moreover, most safety accidents in coal mines are related to underground ventilation systems
[3]. A mine ventilation system is a complex dynamic regulation system which is accountable for transporting fresh air flow to underground roadways, meeting the respiratory requirements of staff, diluting harmful gases in roadways and eliminating toxic gases unrelated to production. Therefore, mine ventilation plays an essential role in safe production at the mine. The safety and stability of the mine ventilation system can be affected by many factors, such as design defects in the ventilation system, inadequate daily maintenance, and failure of the ventilation system to match the changes of excavation production in a timely fashion. Dramatic changes in any one of the internal or external factors can generate an enormous challenge to the safety and stability of the ventilation system and expand the risk of potential accidents. Hence, a universal evaluation system is needed in order to evaluate the quality of mine ventilation systems, which can propound scientific evaluation according to changes in the mine ventilation system
[4].
The evaluation of mine ventilation systems primarily evaluates the quality of the ventilation system in three aspects: safety and reliability, economic rationality, and technical feasibility. The ventilation system corresponds to the actual demand by reasonably arranging air flow routes and the air use position of the ventilation network. For mine ventilation systems, effective evaluation methods have been proposed to improve the accuracy and effectiveness of evaluation, which can be primarily summarized in three categories: the traditional evaluation method, combining a geographic information system (GIS) with other evaluation methods; the empirical evaluation method, based on several important indicators; and the comprehensive evaluation method, based on multiple index items
[5].
Thanks to the efforts of researchers, more and more methods based on the comprehensive evaluation of multiple indicators have been proposed and extensively applied, including: fuzzy mathematics
[6], technique for order preference by similarity to ideal solution (TOPSIS)
[7], gray theory
[8], discriminant analysis method
[9], support vector machine method
[10], neural network method
[11], unascertained measure method
[12] and multi-level fuzzy comprehensive evaluation method
[13]. The comprehensive evaluation process based on multiple indicators is mainly composed of three parts: the establishment of an evaluation index system, the determination of index weight, and the selection of evaluation methods. Among these, determination of the weight of evaluation indicators is a significant part of the whole evaluation process. According to their different sources of original data and calculation processes when calculating the weight coefficient, weight determination methods are generally divided into three categories: the subjective weighting method, objective weighting method and combination weighting method
[14]. The most reasonable weighting method should consider not only the objective law of indicator data, but also the central role of expert experience
[15]. Zhang et al. used an improved analytic hierarchy process (AHP) and the entropy weight method to calculate the subjective and objective weight of the indexes for urban distribution networks
[16]. Guo et al. proposed the AHP-ENTROPY weighting method combining subjective and objective weighting methods to obtain better index weights
[17]. They achieved dynamic weighting of the indicators considering the dynamic changes of the index weights in different system scenarios.
After determining the reasonable index weight, the next crucial step is to select an evaluation method conforming to the actual situation. Zhao et al. proposed a performance evaluation method for smart meters based on grey correlation analysis
[18]. Zhang et al. used a comprehensive evaluation method to realize the introduction of dimensional and multi-index quantitative analysis
[19]. They used the comprehensive evaluation method to simplify the multi-level evaluation index, reduce the index hierarchy, and calculate the ideal solution. Shi presents the adoption of a fuzzy comprehensive evaluation model and group-decision AHP in evaluating the quality of construction projects
[20]. This method could improve the validity and accuracy of the assessment, and it did not rely on the experience of experts. Jia et al. established an index system using the indicator importance sort algorithm
[21]. This method was actually a combination method including expert experience and theoretical calculation.
Unlike other evaluation occasions, an evaluation system for mine ventilation has such unique characteristics as multiple indicators, large amounts of statistical data, and many influencing factors. Jiang et al. used the unascertained measure theory and the uncertain information method to determine an evaluation system for mine ventilation. They applied this method to a lead–zinc mine ventilation system and achieved general results
[22]. Cheng et al. proposed an integrated comprehensive method based on grey cluster analysis–fuzzy theory for selecting and evaluating the most suitable mine ventilation system
[23]. Zhou et al. presented an approach to rank the alternatives by G1-coefficient of variation method. The result showed that this method could rank the alternative development face ventilation mode reasonably
[24]. Yan et al. proposed a novel evaluation method based on cloud model clustering for the ventilation system of an underground metal mine
[25]. The ventilation effectiveness could be properly classified as demonstrated by one case. Gao used the factor analysis method to screen the ventilation indicators and created the AHP-WRSR evaluation model by combining the analytic hierarchy process (AHP) and weight rank–sum ratio (WRSR) to optimize the scheme of a mine ventilation system
[26]. However, qualitative methods such as expert consultation or literature summaries
[27] are mostly used for the above index screening methods, and a quantitative screening process for indicators is lacking. Furthermore, some methods for quantitative screening of indicators have repeated indicators. These issues make it difficult for indicators to reflect satisfactory ventilation system information. Some of the above methods require the experience of experts, and if the experience of experts is unreliable, the obtained results will also be affected. Moreover, the above evaluation model is only focused on the evaluation of existing schemes, and no new schemes are considered. Facing complex systems such as multiple samples and multiple indicators, the above methods have the shortcoming of computational complexity and insufficient flexibility.
2. Construction of Evaluation Index System for Mine Ventilation System
2.1. Weight Calculation Based on the Entropy Method
The entropy method is an objective method for ascertaining the index weight based on the information entropy of the original index data. In information theory, the entropy is a measure of information irregularity. If a definite indicator contains more information, it indicates that the uncertainty situation is miniature and the corresponding entropy value is smaller. If a definite indicator contains less information, it indicates that the uncertainty situation is considerable and the corresponding entropy value is also large. Entropy values can not only characterize the degree of disorder of data information, but also indicate the degree of dispersion of the data. The main steps of the entropy method are as follows.
Firstly, the evaluation matrix is normalized. The raw data are normalized to map the data to the [0, 1] interval, eliminating the impact of dimensional unit restriction for comparison between different metrics. Generally speaking, there are three categories in an index set: interval index, negative effect index, and positive effect index. These indicators have different tendencies and need to be consistent when there are many categories of indicators for an evaluation system. The normalized formula for the positive effect index is:

The normalized formula for the negative effect index is:

where
Xij represents the value after normalization of the
i sample of the
j index;
vij represents the original value of the
i sample of the
j index; max(
vij) represents the largest sample value among all samples for the
j indicator; and min(
vij) represents the smallest sample value among all samples for the
j indicator.
Secondly, the proportion of the
i sample value under the
j indicator is shown in the following equation, where P
ij represents the proportion of the
i sample value under the
j index:
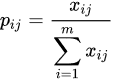
Thirdly, the entropy of each indicator Ej is shown in the following equation:
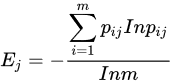
Fourthly, the indicator weight
Wj of the
j indicator is shown in the following equation:
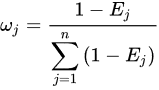
The value of
Wj is between 0 and 1. The larger the value, the more significant the value of the
j indicator.
3. Evaluation Model of TOPSIS
3.2. Evaluation Model of TOPSIS
The correlation between the evaluation scheme and each indicator is difficult to indicate accurately in a definite mathematical expression. Therefore, the evaluation results obtained by linearly adding the index value and the weight are not scientific. In this paper, TOPSIS is utilized to evaluate multiple indexes. TOPSIS method is a systematic evaluation method fitting for multi-index and multi-scheme decision analysis. By calculating the weighted Euclidean distance between a scheme and the positive ideal solution, the closeness of the scheme to the positive ideal solution is obtained. The evaluation model is as follows.
Firstly, a weight normalization matrix is constructed. Assuming
n evaluation schemes and
m evaluation indicators for each scheme, the following characteristic matrix is obtained.

where
Xi represents the value of the
j evaluation indicator in the
i evaluation scheme. Then, the characteristic matrix is normalized to obtain the normalized matrix.

Assuming that the weight of the indicator is
w, a weight normalization matrix in which the weight and normalization matrix are multiplied is determined.
Secondly, positive and negative ideal solutions are calculated.
Thirdly, the closeness of each evaluation scheme to the optimal and worst solution is calculated.
Fourthly, the closeness of the evaluation object to the positive ideal solution is calculated.
The value of Ci is between 0 and 1. The larger the Ci value, the more acceptable the i evaluation scheme.