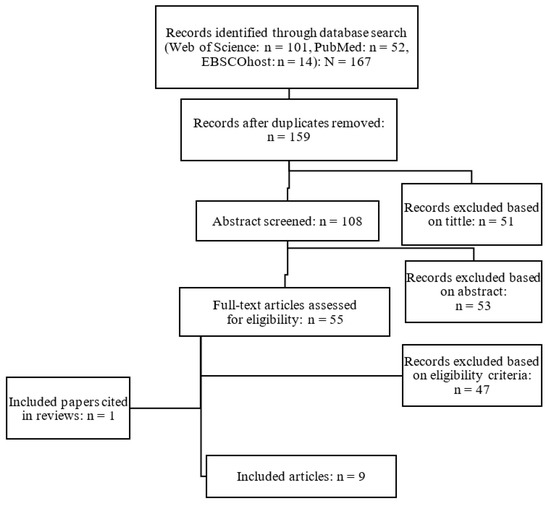
2. Qualitative and Qualitative Analysis
2.1. Synthesis of Findings (Qualitative Analysis)
Scientific evidence on the sample characteristics (B, C, D), variables (E), exercise and variation (F, G) device used (H) volume and intensity training (I, J) and results for strength, power and speed is shown in
,
and
. Format and design, including the author and the year of publication, the sample characteristics (overall number, gender, age, height and weight), the variable measured (strength, power and/or speed), type and number of variations, the device used (exercise ball, semi-sphere ball or suspension device), training volume (number of sets/repeats/rest per exercise), intensity training (percentage of one maximum repetition (1RM)), strength results (maximum strength, mean strength), power results (maximum power, mean power and concentric phase power) and speed results (maximum and mean speed) are included.
Table 1.
Scientific evidence on the sample characteristics (B, C, D) and variables (E).
Table 3.
Scientific evidence on strength results (K), power results (L) and speed results (M).
|
| Sample | Variables (E) | Experience (B) | Size and Sex (C) | Characteristics (D) | |
|---|
|
| Experience (B) | Size and Sex (C) | Characteristics (D) | |
|---|
|
| Anderson et al. (2004)[6] | Trained in strength, Instability 1 year earlier | 10 (M) | a: 26.2 ± 6.0 years | Strength |
| h: 177.3 ± 6.0 cm |
| w: 87.3 ± 12.2 kg |
| Behm et al. (2002)[7] | Trained | 8 (M) | a: 24.3 ± 6.7 years | Strength |
| h:178.1 ± 6.1 cm |
| w: 82.3 ± 8.9 kg |
| Chulvi-Medrano (2010)[ |
| Anderson et al. (2004)[6] | Trained in strength, Instability 1 year earlier |
2.2. Sample Characteristics
shows scientific evidence on the sample characteristics (B, C, D) and variables (E). Format and design, including the author and the year of publication, the sample characteristics (overall number, gender, age, height, and weight), the variable measured (strength, power and/or speed).
Evaluation of the characteristics of the sample: (B) Experience. The experience of the sample was quite heterogeneous, with the participants standing out trained (
n
= 3; 33.34%); trained in strength without experience in instability (
n
= 2; 22.22%), trained in strength and instability 1 year earlier (
n
= 2; 22.22%); recreational (
n
= 1; 11.11%); no previous experience in strength or instability indicated (
n
= 1; 11.11%); (C) Sex. The distribution of the sample was very unbalanced with more male participants (
n
= 158; 98.14%) than female participants (
n
= 3; 1.86%); (D) Characteristics of the sample. The whole sample was identified as being between 18 (lower limit) and 25 (upper limit) years of age. The height range was identified as 167 cm to 185 cm. The weight range was identified as 79 kg to 88 kg.
2.3. Tasks, Devices, and Training Parameters
Shows scientific evidence on exercise and variation (F, G) device used (H) volume and intensity training (I, J). Format and design, including type and number of variations, the device used (exercise ball, semi-sphere ball or suspension device), training volume (number of sets/repeats/rest per exercise), intensity training (percentage of one maximum repetition (1RM)).
According to exercise (F): The most evaluated sports task was the “bench press” in five studies (41.67%) and “squat” in four (33.33%). “Deadweight”, “plantar flexions” and “leg extension” were also evaluated (8.33% each of the exercises). (G) The number of situations. The number of comparisons between stable and unstable exercises was 100% of the situations that only compared the stable situation with an unstable one. (H) Type of device. The use of Swiss ball material was 54.55% (
n
= 6); the use of the semi-sphere ball was 36.36% of the studies analysed (
n
= 4). In only one study was a suspension device (TRX) used (
n
= 1; 9.09%). (I) Training volume. The number of series, repetitions and rest was quite heterogeneous. In the case of the series, only two studies are evident, comprising between three and six series. In the case of the repetitions, they varied from isometric execution to 25 repetitions, with the execution of 3–6 repetitions being the most used (60%). In terms of rest, they vary between 3 and 5 min. (J) Training intensity. In the case of the intensity of training, the percentage of load most used was 75% of 1RM (
n
= 3; 30%). 20% of the investigations did not use external load (
n
= 2). The rest of the investigations ranged from maximum repetition to 40% of 1RM.
shows scientific evidence on strength results (K), power results (L) and speed results (M). Format and design, including strength results (maximum strength, mean strength), power results (maximum power, mean power and concentric phase power) and speed results (maximum and mean speed).
In performance measures, it can be seen how the use of instability decreases in some cases substantially in relation to the stable condition. Although with loads close to the RM no differences are appreciated. In terms of power, the difference seems to be slighter in stable and unstable condition and even at a higher number of repetitions the instability seems to improve power production. The execution speed also shows a lower production when instability is added.
2.4. Strength Results
Concerning the strength parameter with a Swiss ball, the bench press exercise showed 59.4% less isometric strength in instability [6], 5.9%[10], but no differences were found in 1RM [9]. For the lower limb exercises, 70.5% less was evidenced in the unstable condition in leg extension exercises than in the stable condition while the unstable force in plantar flexors was 20.2% less than the stable condition[7]. Also with the same exercise, a decrease with the execution with the semi-sphere ball concerning the stable condition of 19% was evidenced [11]. In the case of the deadweight exercise, the decrease in the maximum isometric contraction between the stable condition and the execution with semi-sphere ball was 10.2%[8]. Regarding suspension training squat exercise with bipodal execution, in the eccentric phase, peak and average force showed a decrease of 46.8% and 13.8% respectively for the lower left limb. In the concentric phase, the use of the suspension training tool caused a decrease of 12.6% in peak force and 12.8% in mean force. For the right lower limb, in the eccentric phase, during execution with the suspension training tool, the force decreased by 42.9% and the mean force by 11.7%. In the concentric phase, during execution with the suspension training tool, the peak and average force decreased respectively by 11.9% and 13.2%. During monopodal execution, the eccentric phase in the left limb, the peak force suffered a decrease of 41.8% and the average force a decrease of 18.1%. In the concentric phase, on the other hand, the use of the suspension training tool caused a decrease of 13.5% in peak force and 15.8% in average force. For the right limb during monopodal execution, in the eccentric phase, the force has decreased by 45.1% and the average force by 17.4%. In the concentric phase, the use of the suspension training tool caused a decrease of 12.4% in the force and 14.3% in the mean force [12].
Concerning the strength parameter with a Swiss ball, the bench press exercise showed 59.4% less isometric strength in instability [13], 5.9% [82], but no differences were found in 1RM [81]. For the lower limb exercises, 70.5% less was evidenced in the unstable condition in leg extension exercises than in the stable condition while the unstable force in plantar flexors was 20.2% less than the stable condition [79]. Also with the same exercise, a decrease with the execution with the semi-sphere ball concerning the stable condition of 19% was evidenced [83]. In the case of the deadweight exercise, the decrease in the maximum isometric contraction between the stable condition and the execution with semi-sphere ball was 10.2% [80]. Regarding suspension training squat exercise with bipodal execution, in the eccentric phase, peak and average force showed a decrease of 46.8% and 13.8% respectively for the lower left limb. In the concentric phase, the use of the suspension training tool caused a decrease of 12.6% in peak force and 12.8% in mean force. For the right lower limb, in the eccentric phase, during execution with the suspension training tool, the force decreased by 42.9% and the mean force by 11.7%. In the concentric phase, during execution with the suspension training tool, the peak and average force decreased respectively by 11.9% and 13.2%. During monopodal execution, the eccentric phase in the left limb, the peak force suffered a decrease of 41.8% and the average force a decrease of 18.1%. In the concentric phase, on the other hand, the use of the suspension training tool caused a decrease of 13.5% in peak force and 15.8% in average force. For the right limb during monopodal execution, in the eccentric phase, the force has decreased by 45.1% and the average force by 17.4%. In the concentric phase, the use of the suspension training tool caused a decrease of 12.4% in the force and 14.3% in the mean force [84].
2.5. Power Results
For the variable of power with a Swiss ball, a decrease in the unstable situation of 9.9% concerning the stable situation has been evidenced with the chest press exercise [
82], and of 10.3% in the average power, 7.3% in the maximum power and 11.5% in the power exercised in the concentric phase[13]. For the average power, with the execution of 25 repetitions, a decrease of 6.9% was found in the unstable bench press, although in the last three repetitions the average power exercised in the unstable condition was 5.6% higher than the stable condition. On the contrary, among the first three repetitions, the unstable data was 12.9% lower than the stable condition. The power exercised in the concentric phase of the bench press was reported to be 4.6% lower in the unstable bench press, although in the last three repetitions the power exercised in the concentric phase of the unstable condition was 13.2% higher than the stable condition. On the contrary, among the first three repetitions, the unstable data was 13.8% lower than the stable condition[14]. To average power, 25 repetitions showed a decrease of 19.3% in the unstable squat, although in the last three repetitions the average power exercised in the unstable condition was 21.4% higher than the stable condition. On the contrary, among the first three repetitions, the unstable condition was 17.1% lower than the stable condition. The power exercised in the concentric phase of the squat was reported to be 18% lower in the unstable squat, although in the last three repetitions the power exercised in the concentric phase of the unstable condition was 20.6% lower than the stable condition. On the contrary, among the first 3 repetitions, the unstable condition was 16.2% lower than the stable condition [14].
], and of 10.3% in the average power, 7.3% in the maximum power and 11.5% in the power exercised in the concentric phase [85]. For the average power, with the execution of 25 repetitions, a decrease of 6.9% was found in the unstable bench press, although in the last three repetitions the average power exercised in the unstable condition was 5.6% higher than the stable condition. On the contrary, among the first three repetitions, the unstable data was 12.9% lower than the stable condition. The power exercised in the concentric phase of the bench press was reported to be 4.6% lower in the unstable bench press, although in the last three repetitions the power exercised in the concentric phase of the unstable condition was 13.2% higher than the stable condition. On the contrary, among the first three repetitions, the unstable data was 13.8% lower than the stable condition [86]. To average power, 25 repetitions showed a decrease of 19.3% in the unstable squat, although in the last three repetitions the average power exercised in the unstable condition was 21.4% higher than the stable condition. On the contrary, among the first three repetitions, the unstable condition was 17.1% lower than the stable condition. The power exercised in the concentric phase of the squat was reported to be 18% lower in the unstable squat, although in the last three repetitions the power exercised in the concentric phase of the unstable condition was 20.6% lower than the stable condition. On the contrary, among the first 3 repetitions, the unstable condition was 16.2% lower than the stable condition [86].
2.6. Speed Results
Only one research study has been shown to consider the execution speed of strength exercises measured in instability. In the case of the speed variable with a Swiss ball, there has been a 9.1% decrease in the unstable condition concerning the stable banking press [
82].
2.7. Study Selection and Assessment (Qualitative Analysis)
The quality analysis (STROBE’ checklist) yielded the following results (
): (a) The quality scores ranged from 13–16; (b) The average score was 14.3 points; (c) Of the 9 included studies, 5 (55.55%) were considered to ‘fair quality’ (13–14 points); and 4 (44.44%) were categorized as ‘good quality’ (15–16 points).
Table 4.
The study quality analysis (STROBE’ checklist).
| Reference | Title and Abstract | Introduction | Methods | Results | Other Analysis | Discussion | Other Information | Strobe Points | Study Quality |
|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | | |
|---|
| Anderson et al. (2004) [13] |
| 10 (M) | a: 26.2 ± 6.0 years | Strength |
| + | + | + | - | - | + | + | + | - | - | + | + | - | - | + | + | - | + | - | + | + | - | 13 | FAIR |
| h: 177.3 ± 6.0 cm |
| Behm et al. (2002) [79] | + | + | + | - | - | + | + | + | - | - | + | + | - | - | + | + | + | + | - | + | + | - | 14 | FAIR |
| Chulvi-Medrano (2010) [80] | + | + | + | + | + | + | + | + | - | - | + | + | - | - | + | + | - | + | + | + | + | - | 16 | GOOD |
| Goodman et al. (2008) [81] | + | + | + | + | + | + | + | + | - | - | + | + | - | - | + | + | + | + | - | + | + | - | 16 | GOOD |
| Koshida et al. (2008) [82] | + | + | + | + | + | + | + | - | 15 | GOOD |
| Saeterbakken & Fimland (2013) [83] | + | + | + | + | - | + | + | + | - |
| 8] | Trained in strength Experience with instability | 31 (M) | a: 24.29 ± 0.48 years | Strength |
| h: 167.98 ± 8,11 cm |
| w: 79.08 ± 2,37 kg |
| - | + | + | - |
| Goodman et al. (2008)[9] | Recreational | 13 (10 M, 3 W) | a: 24.1 ± 1.6 years | Strength |
| h: 176.7 ± 3.0 cm |
| w: 76.0 ± 3.9 kg |
| Koshida et al. (2008)[10] | Trained | 20 (M) | a: 21.3 ± 1.5 years | Strength |
| h: 167.7 ± 7.7 cm |
| w: 87.3 ± 12.2 kg |
| Behm et al. (2002)[4] | Trained | 8 (M) | a: 24.3 ± 6.7 years | Strength |
| h:178.1 ± 6.1 cm |
| w: 82.3 ± 8.9 kg |
| + | - | + | + | + | - | - | + | + | - | - | + | - | + | + | - | + | - |
| Chulvi-Medrano (2010)[8] | Trained in strength Experience with instability | 31 (M) | a: 24.29 ± 0.48 years | Strength |
| + |
| h: 167.98 ± 8,11 cm |
| + | + | - | 13 | FAIR |
| Sannicandro et al. (2015) [84] | + | + | + | - | - | + | + | + | - | - | + | + | - | - | + | + | + | + | - | + | + | + | 15 | GOOD |
| Zemkova (2012) [85] | + | + | + | - | - | + | + | + | - | - | + | + | - | - | + | + | - | + | + | + | + | + | 14 | FAIR |
| w: 79.08 ± 2,37 kg |
| Zemkova et al. (2017) [86] | + | + | + | - | - | + | + | + | - | - | + | + | - | - | + | + | - | + | - | + | + | - | 13 |
| Goodman et al. (2008)[9] | Recreational | 13 (10 M, 3 W) | a: 24.1 ± 1.6 years | Strength |
| h: 176.7 ± 3.0 cm |
| w: 76.0 ± 3.9 kg |
| Koshida et al. (2008)[10] | Trained | 20 (M) | a: 21.3 ± 1.5 years | Power |
| Strength |
| h: 167.7 ± 7.7 cm | Power | w: 75.9 ± 17.5 kg | Speed |
| FAIR |
| w: 75.9 ± 17.5 kg | Speed | Saeterbakken & Fimland (2013)[11] | Trained | 15 (M) | a: 23.3 ± 2.7 |
| Saeterbakken & Fimland (2013) | Strength |
| [11] | h: 181 ± 0.09 cm |
| w: 80.5 ± 8.5 kg |
| Trained | 15 (M) | a: 23.3 ± 2.7 | Strength | Sannicandro et al. (2015)[12] | No previous experience in strength or instability is indicated | 24 (M) | a: 17.8 ± 0.8 years |
| h: 181 ± 0.09 cm | Strength |
| w: 80.5 ± 8.5 kg |
| Power |
| Sannicandro et al. (2015)[12] | No previous experience in strength or instability is indicated | 24 (M) | a: 17.8 ± 0.8 years | Strength
Power | h: 179.1 ± 5.6 cm |
| w: 73 ± 4.9 kg |
| Zemkova (2012) |
| h: 179.1 ± 5.6 cm | [ |
| w: 73 ± 4.9 kg | 13] |
| Zemkova (2012) | Trained in strength, no experience in instability | [13] | 16 (M) | a: 23.4 ± 1.9 years | Power |
| Trained in strength, no experience in instability | 16 (M) | a: 23.4 ± 1.9 years | Power | h: 181.5 ± 6.1cm |
| - |
| h: 181.5 ± 6.1cm | w: 75.1 ± 6.1 kg |
| w: 75.1 ± 6.1 kg | Zemkova et al. (2017)[14] | Trained in strength, no experience in instability | 24 (M) | a: 22.1 ± 1.8 years | Power |
| Zemkova et al. (2017)[14] | Trained in strength, no experience in instability | 24 (M) | a: 22.1 ± 1.8 years | h: 184.5 ± 8.3 cm |
| w: 79.8 ± 9.4 kg |
Table 2.
Scientific evidence on exercise and variation (F, G) device used (H) volume and intensity training (I, J).
|
| Power |
|
|
| h: 184.5 ± 8.3 cm |
|
|
| w: 79.8 ± 9.4 kg |
|
By sections, the highest scores were located in ‘introduction’ (100%) and ‘discussion’ (80%) and among the highest quality studies, items no. 2 (background/rationale); no. 3 (‘Objectives—State specific objectives and/or any pre-specified hypothesis’); no. 6 (participants); no. 7 (variables); no. 8 (‘data source—procedure for determining performance measurement’), no. 11 (‘descriptive results—the number (absolute frequency) or percentage (relative frequency) of participants found in each grouping category and subcategory’); no. 12 (statistical methods); no. 15 (outcome data); no. 16 (main results); no. 18 (‘key results—a summary of key results concerning study objectives’); no. 20 (interpretation) and no. 21 (generalisability) were considered complete (100%), while the most commonly absent or incomplete item (0 points) was found in items no. 9, 10, 13 and 14 (‘main results—a measure of effect size’). The lowest scores were found in the ‘funding’ section (20%).
3. Discussion
This is the first systematic review of the literature to examine the effects of instability on measures of muscle strength, power, and speed, administered in the form of quasi-experimental studies in healthy individuals during adulthood.
About the production of force, for the exercises of the upper limb, high decreases in values have been observed for the unstable condition. These differences have ranged from 20 to 75% loss in force development in unstable conditions. According to Kornecki, Kebel, and Siemieński[15], the stabilising function of the skeletal muscles is necessary for the coordinated performance of any voluntary movement, and it significantly influences muscle coordination patterns. Therefore, significant reductions in muscle production probably occurred because the muscles around the shoulder complex needed to give priority to stability over force production. Furthermore, under conditions of instability, the stiffness of the joints that act can limit gains in strength, power and speed of movement[16].
About the production of force, for the exercises of the upper limb, high decreases in values have been observed for the unstable condition. These differences have ranged from 20 to 75% loss in force development in unstable conditions. According to Kornecki, Kebel, and Siemieński [87], the stabilising function of the skeletal muscles is necessary for the coordinated performance of any voluntary movement, and it significantly influences muscle coordination patterns. Therefore, significant reductions in muscle production probably occurred because the muscles around the shoulder complex needed to give priority to stability over force production. Furthermore, under conditions of instability, the stiffness of the joints that act can limit gains in strength, power and speed of movement [88].
However, in the data evidenced in the study by Koshida et al. [10], the losses in force values are much lower than in the rest of the studies (5.9% loss in instability) compared with 59.4% loss in Anderson and Behm [6] and in the case of Goodman et al. [9], no significant differences are observed. This inconsistency between the previous research can be attributed to the type of muscle contraction, the degree of instability during the recorded task and the equipment. In Koshida et al. [10] the bench press movement was performed in a supine position with the Swiss ball placed in the thoracic area, which provided a broader support base than for other activities performed in a sitting or standing position. Therefore, the instability imposed on the trunk stabilising muscles would probably be less significant than in previous research. Besides, both studies used dynamic contractions with an Olympic bar with weight plates, while Anderson and Behm [6] used isometric contractions with two independent handles held by straps to force the transducers into the ground. The difference in equipment could impose different levels of instability on the shoulder joint and trunk muscles. Although bilateral contractions were performed in both studies, the independence of each hand in the study by Anderson and Behm[6] may have increased the effort required to maintain balance and the need for the muscles to stabilize during maximum isometric contractions, therefore reducing the net force output. In the case of Goodman et al. [9], where no differences were found, it could be due to the use of different loads, since 1RM was used while in Anderson and Behm[6] 75% of 1RM loads were used and in Goodman et al. [
However, in the data evidenced in the study by Koshida et al. [82], the losses in force values are much lower than in the rest of the studies (5.9% loss in instability) compared with 59.4% loss in Anderson and Behm [13] and in the case of Goodman et al. [81], no significant differences are observed. This inconsistency between the previous research can be attributed to the type of muscle contraction, the degree of instability during the recorded task and the equipment. In Koshida et al. [82] the bench press movement was performed in a supine position with the Swiss ball placed in the thoracic area, which provided a broader support base than for other activities performed in a sitting or standing position. Therefore, the instability imposed on the trunk stabilising muscles would probably be less significant than in previous research. Besides, both studies used dynamic contractions with an Olympic bar with weight plates, while Anderson and Behm [13] used isometric contractions with two independent handles held by straps to force the transducers into the ground. The difference in equipment could impose different levels of instability on the shoulder joint and trunk muscles. Although bilateral contractions were performed in both studies, the independence of each hand in the study by Anderson and Behm [13] may have increased the effort required to maintain balance and the need for the muscles to stabilize during maximum isometric contractions, therefore reducing the net force output. In the case of Goodman et al. [81], where no differences were found, it could be due to the use of different loads, since 1RM was used while in Anderson and Behm [13] 75% of 1RM loads were used and in Goodman et al. [ 81
] were used 50% of 1RM. These data could indicate that the percentage of external load can influence the effect of the instability in the training.
In the case of force production in the lower limbs, there have been notable decreases when comparing tasks performed in instability concerning stable conditions. These decreases ranged from 10% to 19%, so it seems that instability affects the upper body more than the lower. With the use of the semi-sphere ball, analysed in terms of strength, with the performance of a dominant hip exercise such as deadweight the decrease in strength was 10.2%[8] while with a dominant knee exercise such as squat it was 19% [11]. This could indicate that certain movements could be affected to a lesser extent depending on the instability. However, as detailed above the methods and loads used were very different. It is noteworthy that many of the studies to check force production in the lower limb using isometric contractions. However, isometric contractions are not usually used in strength training. Despite this, results obtained under isometric contractions have reported that conditions are strongly correlated with dynamic mobility performance[17]. However, due to the isometric test mode, subjects could gradually build up strength while stabilizing and maintaining balance on different surfaces. During the 3 s of maximum effort, the subjects may have been able to stabilize the limbs and trunk and therefore be able to exert a considerable amount of force in unstable conditions. We only know of one study that investigates the production of maximum force in squats on a stable and unstable surface[18]. These researchers used an inflatable balance disk and reported a decrease of approximately 46% in peak force. Although there was a greater decrease in force in that study, it could be attributed to the lack of a familiarization session, which the rest of the studies did consider appropriate.
In the case of force production in the lower limbs, there have been notable decreases when comparing tasks performed in instability concerning stable conditions. These decreases ranged from 10% to 19%, so it seems that instability affects the upper body more than the lower. With the use of the semi-sphere ball, analysed in terms of strength, with the performance of a dominant hip exercise such as deadweight the decrease in strength was 10.2% [80] while with a dominant knee exercise such as squat it was 19% [83]. This could indicate that certain movements could be affected to a lesser extent depending on the instability. However, as detailed above the methods and loads used were very different. It is noteworthy that many of the studies to check force production in the lower limb using isometric contractions. However, isometric contractions are not usually used in strength training. Despite this, results obtained under isometric contractions have reported that conditions are strongly correlated with dynamic mobility performance [89]. However, due to the isometric test mode, subjects could gradually build up strength while stabilizing and maintaining balance on different surfaces. During the 3 s of maximum effort, the subjects may have been able to stabilize the limbs and trunk and therefore be able to exert a considerable amount of force in unstable conditions. We only know of one study that investigates the production of maximum force in squats on a stable and unstable surface [3]. These researchers used an inflatable balance disk and reported a decrease of approximately 46% in peak force. Although there was a greater decrease in force in that study, it could be attributed to the lack of a familiarization session, which the rest of the studies did consider appropriate.
In terms of strength, there seems to be a differentiation in the data concerning the devices used. When the main movement involves the muscles of the upper body the Swiss ball has been used and in the case of the main movement being performed on the lower body, the semi-sphere ball has been used. The exceptional case was the execution of an exercise such as squatting where the instability with the device in suspension was placed in the upper body. In the case of the Swiss ball, it seems to have a greater influence on the decrease of the force values (differences of 20–75%) compared to the semi-sphere ball (differences between the and 10% and 19%) suspended device (detriments between 12–47%). However, in some cases, the Swiss ball did not produce any differences between the conditions. So, it does not seem to be a determining factor in the case of muscle strength.
Concerning the production of muscular power in the upper limb, decreases in the unstable condition have been observed. Decreases with the unstable condition ranged from 7% to 17%. The data found in the studies that analyzed the bench press with Swiss ball reported a very similar percentage decrease in terms of average power (10.3% [13]; 9.9% [10]; and 12.9% [14]). These small deviations found may again be due to the different percentage of load used (50% vs. 75%) and the different volume of training applied. However, in some situations, instability has produced better power data than a stable condition. These better data have been produced in the last repetitions of the executions with high numbers of repetitions in the exercise (22–25 repetitions). The improvements observed in average power were 5.6% and in the concentric phase 13.2%.
Concerning the production of muscular power in the upper limb, decreases in the unstable condition have been observed. Decreases with the unstable condition ranged from 7% to 17%. The data found in the studies that analyzed the bench press with Swiss ball reported a very similar percentage decrease in terms of average power (10.3% [85]; 9.9% [82]; and 12.9% [86]). These small deviations found may again be due to the different percentage of load used (50% vs. 75%) and the different volume of training applied. However, in some situations, instability has produced better power data than a stable condition. These better data have been produced in the last repetitions of the executions with high numbers of repetitions in the exercise (22–25 repetitions). The improvements observed in average power were 5.6% and in the concentric phase 13.2%.
The increase in power observed could be due to previous evidence that has shown that producing a high power output with a light to moderate load would be more effective in developing maximum power than using a heavy load [19][20]. Thus, it appears that such a low rate of reduction may still allow muscle power to be gained from strength exercise in the unstable condition. The mechanism of energy production using the stretch-shortening cycle employs the energy storage capacity of several elastic components and the stimulation of the stretching reflex to facilitate muscle contraction for a minimum period. The concentric muscle action does not occur immediately after the eccentric, the stored energy is dissipated and lost in the form of heat and also the strengthening stretching reflex is not activated. Resistance to instability exercise can compromise the three phases of the stretching-shortening cycle, including the amortization phase. Around this turning point, where the eccentric phase becomes concentric, the maximum force is produced. At the same time, the subjects must stabilize the torso on an unstable surface to provide firm support for the contracted muscles. This additional task can compromise the contraction of the muscles acting on the bar. Their less intense contraction not only prolongs the change of direction of movement but, due to the lower maximum force, impairs the accumulation of elastic energy. The consequence is less speed and power in the subsequent concentric phase[21][22]. However, the subjects of the study by Zemkova et al. [14] were able to produce greater power during the executions on an unstable surface than on a stable one. This higher energy production can be attributed to the so-called ball bounce effect.
The increase in power observed could be due to previous evidence that has shown that producing a high power output with a light to moderate load would be more effective in developing maximum power than using a heavy load [90,91]. Thus, it appears that such a low rate of reduction may still allow muscle power to be gained from strength exercise in the unstable condition. The mechanism of energy production using the stretch-shortening cycle employs the energy storage capacity of several elastic components and the stimulation of the stretching reflex to facilitate muscle contraction for a minimum period. The concentric muscle action does not occur immediately after the eccentric, the stored energy is dissipated and lost in the form of heat and also the strengthening stretching reflex is not activated. Resistance to instability exercise can compromise the three phases of the stretching-shortening cycle, including the amortization phase. Around this turning point, where the eccentric phase becomes concentric, the maximum force is produced. At the same time, the subjects must stabilize the torso on an unstable surface to provide firm support for the contracted muscles. This additional task can compromise the contraction of the muscles acting on the bar. Their less intense contraction not only prolongs the change of direction of movement but, due to the lower maximum force, impairs the accumulation of elastic energy. The consequence is less speed and power in the subsequent concentric phase [92,93]. However, the subjects of the study by Zemkova et al. [86] were able to produce greater power during the executions on an unstable surface than on a stable one. This higher energy production can be attributed to the so-called ball bounce effect.
In terms of power, there seems to be a differentiation in the data about the devices used. The use of semi-sphere ball seems to have a greater influence on the decrease in power since the detriments with this device varied between 15% and 22%. In the case of the Swiss ball, the decrease in power oscillated between 7% and 14%, with better power data being found in unstable conditions with this device (5–13%). However, when the instability was placed where there was no movement, as in the case of the device in suspension and the squat, improvements of between 5% and 10% in power production were shown. Therefore, placing the instability where the main movement does not occur seems to be a good option for power improvement.
Finally, about the speed of execution, a decrease in the values in unstable conditions in comparison to stable conditions is observed, but the analysis of this variable has hardly been studied. According to Adkin et al. [23], a postural threat in a subject (fear of falling) will lead to a reduction in the magnitude and speed of voluntary movements. Thus, muscle stabilization seems to compromise gains in strength, power and speed of movement[24]. It should also be noted that new patterns of movement are generally learned at low speed, while sport-specific motor actions are executed at high speed[25].
Finally, about the speed of execution, a decrease in the values in unstable conditions in comparison to stable conditions is observed, but the analysis of this variable has hardly been studied. According to Adkin et al. [94], a postural threat in a subject (fear of falling) will lead to a reduction in the magnitude and speed of voluntary movements. Thus, muscle stabilization seems to compromise gains in strength, power and speed of movement [95]. It should also be noted that new patterns of movement are generally learned at low speed, while sport-specific motor actions are executed at high speed [26].
The great heterogeneity in terms of volume and intensity of the load is remarkable. Also, instability seems to affect the force variable to a higher degree, but with intensities close to 1RM no differences are observed. As for power, a greater number of repetitions seems to benefit the production of this variable in instability in the upper limb. Finally, speed has barely been analysed and seems to show losses of speed in instability but not excessively so.