You're using an outdated browser. Please upgrade to a modern browser for the best experience.
Please note this is a comparison between Version 3 by Jason Zhu and Version 2 by Jason Zhu.
The temperature field and chemiluminescence measurements of axisymmetric flame are obtained simultaneously in only one image. Digital Laser Speckle Displacement measures temperature fields, and direct image flame determines chemiluminescence values. Applying the Abel transform of axisymmetric objects for volume visualization requires smooth intensity profiles. Due to the nature of the experimental setup, direct image flame is corrupted with speckle noise and a crosstalk effect. These undesirable effects deteriorate the measurement results. Then, experimental data need crosstalk correction and speckle noise reduction to improve the measurements.
- chemiluminescence
- optics
- fluid flow
- premixed flames
1. Introduction
Studying a combustion process is a very complex task [1]. However, the characterization of a flame is essential to improve the combustion process’s efficiency and reduce pollutant emissions. Measuring several variables in a flame is used to monitor the efficiency of combustion processes. Generally, some measured variables are flame temperature, soot concentration, radical intensities, flame size, luminosity, and flickering [2][3][4][5][6][7][8][9][10][11][12][13][14][15][16][17][18][19][20][21][22]. Full-field optical techniques are usually used to make this kind of measurement because they give a global description of the process and do not disturb the sample. On the other hand, simultaneously measuring several variables is preferable because this avoids the lack of correlation between them [9][16][17][18][19]. However, a complex optical setup is usually employed for measurement in such cases, making the task costly and challenging [16][18].
The flame equivalence ratio (𝛷) is an adimensional variable used to determine if a fuel-oxidizer mixture is rich, lean, or stoichiometric [1]. When 𝛷>1, it denotes a fuel-rich mixing process; on the other hand, 𝛷<1 is related to fuel-lean mixtures, and the last 𝛷=1 in the ratio relationship is the value for stoichiometric mixing. This relation is intrinsically linked to determining a system’s performance. This study, as in others, corroborates that the maximum temperature value is obtained at a value of 𝛷=1.05 for LPG fuel. Other variables that can be connected directly to the flame equivalence ratio are the temperature and radical intensities (chemiluminescence) [10][11][12][13][14][15][16][17][18][19]. In a combustion process, some released species are OH, CH, CH2O, and C2, which have a specific emission spectrum [10][11][12][13][14][15][16][17][18][19]. In these combustion flames, the presence of radicals C∗2 and CH∗ are inherent in the reaction zone. These two chemical species are most abundant within the flame. Furthermore, these radicals emit in the visible region of the electromagnetic spectrum, centered at wavelengths 430 nm and 525 nm, respectively [14]. For this reason, digital color cameras play an essential role in chemiluminescence measurements [11][12][13][14][15][16][17][18]. In this analysis, each color channel contributes specific information about the object under study. However, most digital color cameras suffer from the crosstalk effect; this occurs when sensors have overlapping sensibilities, contaminating the recorded data and needing correction [15][17].
There are few works in which chemiluminescence and flame temperature are measured [3][16][17][18]. In one of the works, the optical system is simple; they only use a camera, and the temperature is measured using two-color pyrometry. However, temperature measurement is applicable only for sooty flames [3]. In other research, two-color pyrometry is also used to determine the flame’s temperature; however, the optical arrangement is complex [16][18]. In [17], the optical configuration is simple to implement, and Digital Laser Speckle Displacement (DLSD) is used to determine the temperature fields. However, the chemiluminescence measurements are contaminated with speckle noise, making determining these values difficult.
2. Theoretical Development of Intensity Profiles on Flames
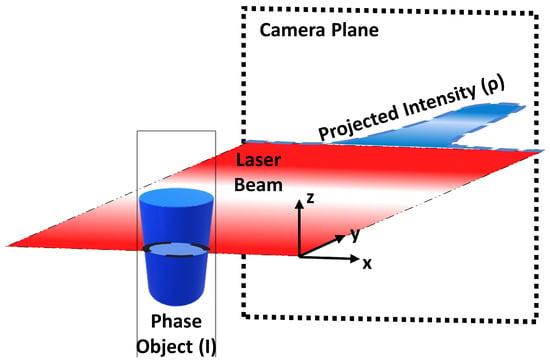
Figure 1. Intensity profile projected on the camera plane from the phase object.
3. Algorithm for Profile Intensity Noise Reduction
3. Abel Reconstruction
4. Crosstalk Correction
𝐼𝑟̂=𝐴𝑟0𝐼0+𝐴𝑟1𝐼1+𝐴𝑟2𝐼2
𝐼𝑔̂=𝐴𝑔0𝐼0+𝐴𝑔1𝐼1+𝐴𝑔2𝐼2
𝐼𝑏̂=𝐴𝑏0𝐼0+𝐴𝑏1𝐼1+𝐴𝑏2𝐼2
𝐼𝑔̂≈𝐴𝑔𝐺𝐼𝐺+𝐴𝑔𝐵𝐼𝐵
𝐼𝑏̂≈𝐴𝑏𝐺𝐼𝐺+𝐴𝑏𝐵𝐼𝐵
References
- Günther, R. Flames—Their Structure, Radiation and Temperature. Von A. G. Gaydon und H. G. Wolfhard. Chapman and Hall Ltd., London 1979 4. Aulf., XIII, 449 S., zhalr. Abb. u. Tab., Ln., £18.00. Chem. Ing. Tech. 1979, 51, 765.
- Zhou, Y.; Zheng, D. Study on Measurement of Flame Temperature Using a Linear CCD. In Proceedings of the International Technology and Innovation Conference 2006 (ITIC 2006), London, UK, 11–12 October 2006; IEEE: Hangzhou, China, 2006; Volume 2006, pp. 1647–1650.
- Toro, N.C.; Arias, P.L.; Torres, S.; Sbarbaro, D. Flame Spectra-Temperature Estimation Based on a Color Imaging Camera and a Spectral Reconstruction Technique. Appl. Opt. 2014, 53, 6351.
- Zhao, H.; Feng, H.; Xu, Z.; Li, Q. Research on Temperature Ditribution of Combustion Flames Based on High Dynamic Range Imaging. Opt. Laser Technol. 2007, 39, 1351–1359.
- Alvarez-Herrera, C.; Moreno-Hernández, D.; Barrientos-García, B. Temperature Measurement of an Axisymmetric Flame by Using a Schlieren System. J. Opt. A Pure Appl. Opt. 2008, 10, 104014.
- Farrell, P.V.; Hofeldt, D.L. Temperature Measurement in Gases Using Speckle Photography. Appl. Opt. 1984, 23, 1055.
- Fujisawa, N.; Aiura, S.; Ohkubo, M.; Shimizu, T. Temperature Measurement of Dilute Hydrogen Flame by Digital Laser-Speckle Technique. J. Vis. 2009, 12, 57–64.
- Wang, Y.; Chung, S.H. Soot Formation in Laminar Counterflow Flames. Prog. Energy Combust. Sci. 2019, 74, 152–238.
- Legros, G.; Wang, Q.; Bonnety, J.; Kashif, M.; Morin, C.; Consalvi, J.-L.; Liu, F. Simultaneous Soot Temperature and Volume Fraction Measurements in Axis-Symmetric Flames by a Two-Dimensional Modulated Absorption/Emission Technique. Combust. Flame 2015, 162, 2705–2719.
- Hardalupas, Y.; Orain, M.S.; Panoutsos, C.; Taylor, A.M.K.P.; Olofsson, J.; Seyfried, H.; Richter, M.; Hult, J.; Aldén, M.; Hermann, F.; et al. Chemiluminescence Sensor for Local Equivalence Ratio of Reacting Mixtures of Fuel and Air (FLAMESEEK). Appl. Therm. Eng. 2004, 24, 1619–1632.
- Kojima, J.; Ikeda, Y.; Nakajima, T. Basic Aspects of OH(A), CH(A), and C2(d) Chemiluminescence in the Reaction Zone of Laminar Methane–Air Premixed Flames. Combust. Flame 2005, 140, 34–45.
- Huang, H.-W.; Zhang, Y. Flame Colour Characterization in the Visible and Infrared Spectrum Using a Digital Camera and Image Processing. Meas. Sci. Technol. 2008, 19, 085406.
- Huang, H.W.; Zhang, Y. Digital Colour Image Processing Based Measurement of Premixed CH4+air and C2H4+air Flame Chemiluminescence. Fuel 2011, 90, 48–53.
- Trindade, T.; Ferreira, A.; Fernandes, E. Characterization of Combustion Chemiluminescence: An Image Processing Approach. Procedia Technol. 2014, 17, 194–201.
- Yang, J.; Ma, Z.; Zhang, Y. Improved Colour-Modelled CH* and C2* Measurement Using a Digital Colour Camera. Measurement 2019, 141, 235–240.
- Cai, W.; Kaminski, C.F. A Tomographic Technique for the Simultaneous Imaging of Temperature, Chemical Species, and Pressure in Reactive Flows Using Absorption Spectroscopy with Frequency-Agile Lasers. Appl. Phys. Lett. 2014, 104, 034101.
- Zamarripa-Ramírez, J.C.I.; Moreno-Hernández, D.; Martinez-Gonzalez, A. Simultaneous Measurement of Temperature and Color Spectrum of Axisymmetric Premixed Flames Using Digital Laser Speckle Photography and an Image Processing Approach. Meas. Sci. Technol. 2021, 32, 105903.
- Chorey, D.; Jagadale, V.; Prakash, M.; Hanstorp, D.; Andersson, M.; Deshmukh, D.; Mishra, Y.N. Simultaneous Imaging of CH*, C2*, and Temperature in Flames Using a DSLR Camera and Structured Illumination. Appl. Opt. 2023, 62, 3737.
- Hardalupas, Y.; Orain, M. Local Measurements of the Time-Dependent Heat Release Rate and Equivalence Ratio Using Chemiluminescent Emission from a Flame. Combust. Flame 2004, 139, 188–207.
- Lundberg, J.; Henriksen, M.; Gaathaug, A. Using Image Processing for Flame Diagnostics. In Linköping Electronic Conference Proceedings, Proceedings of the 58th Conference on Simulation and Modelling (SIMS 58) Reykjavik, Iceland, 25–27 September 2017; Linköping University Electronic Press: Linköping, Sweden, 2017; pp. 168–173.
- Al-Tayyar, M.A.; AlKhafaji, D.; Shahad, H.A. A Review on Flame Height and Structure and Pollutants Formation. Neuro Quantology 2022, 20, 2946–2968.
- Tanoue, K.; Ogura, Y.; Takayanagi, M.; Nishimura, T. Measurement of Temperature Distribution for the Flickering Phenomenon around the Premixed Flame by Using Laser Speckle Method. J. Vis. 2010, 13, 183–185.
- Dreyer, J.A.H.; Slavchov, R.I.; Rees, E.J.; Akroyd, J.; Salamanca, M.; Mosbach, S.; Kraft, M. Improved Methodology for Performing the Inverse Abel Transform of Flame Images for Color Ratio Pyrometry. Appl. Opt. 2019, 58, 2662.
- Rosa-Miranda, E.D.L.; Berriel-Valdos, L.R.; Gonzalez-Ramirez, E.; Alaniz-Lumbreras, D.; Saucedo-Anaya, T.; De La Rosa-Vargas, J.I.; Villa-Hernandez, J.J.; Torres-Arguelles, V.; Castano, V.M. An Alternative Approach to the Tomographic Reconstruction of Smooth Refractive Index Distributions. J. Eur. Opt. Soc.-Rapid Publ. 2013, 8, 13036.
- Fomin, N.A. Speckle Photography for Fluid Mechanics Measurements; Springer: Berlin/Heidelberg, Germany, 1998.
More
